GMAT Exam > GMAT Notes > Quantitative for GMAT > Important Formulas: Arithmetic Progression
Important Formulas: Arithmetic Progression | Quantitative for GMAT PDF Download
Logarithm
- If a is a positive real number, other than 1 and am = x, then we write: m = loga x and we say that the value of log x to the base a is m.
- Examples: (i) 103 1000 ⇒ log10 1000 = 3.
(ii) 34 = 81 ⇒ log3 81 = 4.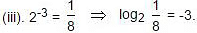
(iv) (. 1)2 = 01 ⇒ log(.1) .01 = 2.
Properties of Logarithms
1. log a (xy) = loga x + loga y
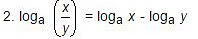
3. logx x = 1
4. loga 1 = 0
5. loga (xn) = n(loga x)
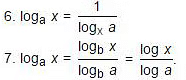
Common Logarithms
- Logarithms to the base 10 are known as common logarithms.
- The logarithm of a number contains two parts, namely 'characteristic' and 'mantissa'.
1. Characteristic
The internal part of the logarithm of a number is called its characteristic.
Case I: When the number is greater than 1.
- In this case, the characteristic is one less than the number of digits in the left of the decimal point in the given number.
Case II: When the number is less than 1.
- In this case, the characteristic is one more than the number of zeros between the decimal point and the first significant digit of the number and it is negative.
- Instead of -1, -2 etc. we write (two bar), etc.
Examples: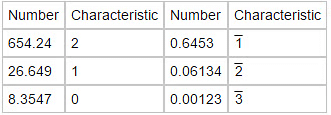
(two bar), etc.
2. Mantissa:
The decimal part of the logarithm of a number is known is its mantissa. For mantissa, we look through log table.
Question 1: If log2X + log4X = log0.25√6 and x > 0, then x is:
A. 6-1/6
B. 61/6
C. 3-1/3
D. 61/3
Correct Answer is Option (A).
- log2x + log4x = log0.25√6
We can rewrite the equation as: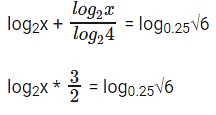
⇒ log2x * 3 = 2log0.25√6
⇒ log2x3 = -log46
⇒⇒
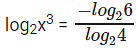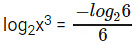
⇒ 2log2x3 = -log26 - 2log2x3 + log26 = 0
log26X6 = 0 - 6x6 = 1
x6 = 1/6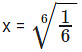 - The question is "If log2X + log4X = log0.25 √6 and x > 0, then x is"
- Hence, the answer is "6-1/6".
⇒
Question 2: log9 (3log2 (1 + log3 (1 + 2log2x))) = 1/2. Find x.
A. 4
B. 1/2
C. 1
D. 2
Correct Answer is Option (D).
log9 (3log2 (1 + log3 (1 + 2log2x)) = 1/2
3log2(1 + log3(1 + 2log2x)) = 91/2 = 3
log2(1 + log3(1 + 2log2x) = 1
1 + log3(1 + 2log2x) = 2
log3(1 + 2log2x) = 1
1 + 2log2x = 3
2log2x = 2
log2x = 1
x = 2
The question is "Find x."
Hence, the answer is "2".
Question 3: If 22x+4 – 17 × 2x+1 = –4, then which of the following is true?
A. x is a positive value
B. x is a negative value
C. x can be either a positive value or a negative value
D. None of these
Correct Answer is Option (C).
2x+4 – 17 * 2x+1 = – 4
=> 2x+1 = y
22x+2 = y2
22(22x+2) – 17 * 2x+1 = –4
4y2 – 17y + 4 = 0
4y2 – 16y – y + y = 0
4y (y – 4) – 1 (y – 4) = 0
y = 1/4 or 4
2x+1 = 1/4 or 4
⇒ x + 1 = 2 or – 2
x = 1 or – 3
The question is "which of the following is true?"
Hence, the answer is "x can be either a positive value or a negative value".
Logarithmic Series
Definition
An expansion for loge (1 + x) as a series of powers of x which is valid only, when |x|<1.
Expansion of logarithmic series
Expansion of loge (1 + x) if |x|<1 then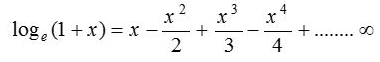
Replacing x by −x in the logarithmic series, we get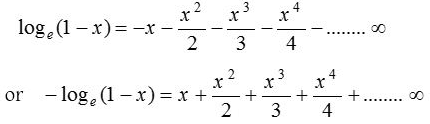
Some Important results from logarithmic series
(1) 
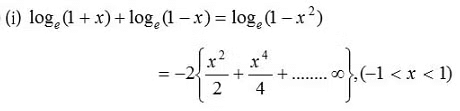
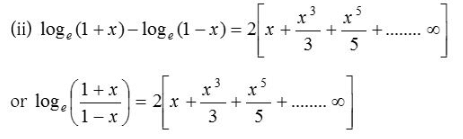
(2) The series expansion of loge (1 + x) may fail to be valid, if |x| is not less than 1. It can be proved that the logarithmic series is valid for x = 1. Putting x = 1 in the logarithmic series.
We get,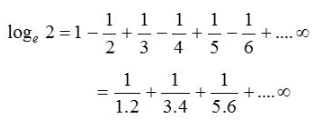
(3) When x = −1, the logarithmic series does not have a sum. This is in conformity with the fact that log(1 – 1) is not a finite quantity.
Difference between the exponential and logarithmic series
- In the exponential series 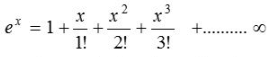all the terms carry positive signs whereas in the logarithmic series 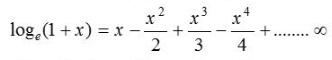the terms are alternatively positive and negative
- In the exponential series the denominator of the terms involve factorial of natural numbers. But in the logarithmic series the terms do not contain factorials.
- The exponential series is valid for all the values of x. The logarithmic series is valid, when |x|< 1.
all the terms carry positive signs whereas in the logarithmic series 
the terms are alternatively positive and negative
The document Important Formulas: Arithmetic Progression | Quantitative for GMAT is a part of the GMAT Course Quantitative for GMAT.
All you need of GMAT at this link: GMAT
|
108 videos|103 docs|114 tests
|
FAQs on Important Formulas: Arithmetic Progression - Quantitative for GMAT
| 1. What are the properties of logarithms? |  |
Ans. The properties of logarithms are as follows:
- Product Rule: logₐ(xy) = logₐ(x) + logₐ(y)
- Quotient Rule: logₐ(x/y) = logₐ(x) - logₐ(y)
- Power Rule: logₐ(xⁿ) = n * logₐ(x)
- Change of Base Rule: logₐ(x) = logᵦ(x) / logᵦ(a)
- Inverse Rule: If logₐ(x) = y, then aⁿ = x
| 2. What are the important formulas related to arithmetic progression? | |
Ans. The important formulas related to arithmetic progression (AP) are:
- nth term of an AP: aₙ = a + (n-1)d
- Sum of first n terms of an AP: Sₙ = (n/2)(2a + (n-1)d)
- Sum of first n terms of an AP using the last term: Sₙ = (n/2)(a + l)
- Sum of squares of first n natural numbers: Sₙ = (n/6)(n+1)(2n+1)
| 3. How do you use the product rule of logarithms? | |
Ans. To use the product rule of logarithms, you can split a product inside a logarithm into separate logarithms. For example, if you have logₐ(xy), you can rewrite it as logₐ(x) + logₐ(y). This allows you to simplify the calculation by evaluating the logarithms of the individual factors separately and then adding them.
| 4. How do you find the nth term of an arithmetic progression? | |
Ans. To find the nth term (aₙ) of an arithmetic progression (AP), you can use the formula aₙ = a + (n-1)d, where a is the first term and d is the common difference between consecutive terms. By substituting the values of a and d into the formula, you can calculate the desired term of the AP.
| 5. How do you find the sum of the first n terms of an arithmetic progression? | |
Ans. To find the sum (Sₙ) of the first n terms of an arithmetic progression (AP), you can use the formula Sₙ = (n/2)(2a + (n-1)d), where a is the first term, d is the common difference, and n is the number of terms. By plugging in the values of a, d, and n into the formula, you can calculate the sum of the desired terms.
About this Document
|
4.68/5 Rating |
|
Nov 24, 2024 Last updated |
Document Description: Important Formulas: Arithmetic Progression for GMAT 2024 is part of Quantitative for GMAT preparation.
The notes and questions for Important Formulas: Arithmetic Progression have been prepared according to the GMAT exam syllabus. Information about Important Formulas: Arithmetic Progression covers topics
like Logarithm, Properties of Logarithms, Logarithmic Series and Important Formulas: Arithmetic Progression Example, for GMAT 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Important Formulas: Arithmetic Progression.
Introduction of Important Formulas: Arithmetic Progression in English is available as part of our Quantitative for GMAT
for GMAT & Important Formulas: Arithmetic Progression in Hindi for Quantitative for GMAT course.
Download more important topics related with notes, lectures and mock test series for GMAT
Exam by signing up for free. GMAT: Important Formulas: Arithmetic Progression | Quantitative for GMAT
Description
Full syllabus notes, lecture & questions for Important Formulas: Arithmetic Progression | Quantitative for GMAT - GMAT | Plus excerises question with solution to help you revise complete syllabus for Quantitative for GMAT | Best notes, free PDF download
Information about Important Formulas: Arithmetic Progression
In this doc you can find the meaning of Important Formulas: Arithmetic Progression defined & explained in the simplest way possible. Besides explaining types of
Important Formulas: Arithmetic Progression theory, EduRev gives you an ample number of questions to practice Important Formulas: Arithmetic Progression tests, examples and also practice GMAT
tests
|
Explore Courses for GMAT exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
video lectures
,MCQs
,Important Formulas: Arithmetic Progression | Quantitative for GMAT
,practice quizzes
,Important Formulas: Arithmetic Progression | Quantitative for GMAT
,Objective type Questions
,Sample Paper
,Important questions
,past year papers
,Exam
,Summary
,mock tests for examination
,study material
,shortcuts and tricks
,Previous Year Questions with Solutions
,Viva Questions
,Important Formulas: Arithmetic Progression | Quantitative for GMAT
,ppt
,Extra Questions
,Semester Notes
,Free
;
Additional Information about Important Formulas: Arithmetic Progression for GMAT Preparation
Important Formulas: Arithmetic Progression Free PDF Download
The Important Formulas: Arithmetic Progression is an invaluable resource that delves deep into the core of the GMAT exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Important Formulas: Arithmetic Progression now and kickstart your journey towards success in the GMAT exam.
Importance of Important Formulas: Arithmetic Progression
The importance of Important Formulas: Arithmetic Progression cannot be overstated, especially for GMAT aspirants.
This document holds the key to success in the GMAT exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Important Formulas: Arithmetic Progression Notes
Important Formulas: Arithmetic Progression Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Important Formulas: Arithmetic Progression.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Important Formulas: Arithmetic Progression Notes on EduRev are your ultimate resource for success.
Important Formulas: Arithmetic Progression GMAT Questions
The "Important Formulas: Arithmetic Progression GMAT Questions" guide is a valuable resource for all aspiring students preparing for the
GMAT exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Important Formulas: Arithmetic Progression on the App
Students of GMAT can study Important Formulas: Arithmetic Progression alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Important Formulas: Arithmetic Progression,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Important Formulas: Arithmetic Progression is prepared as per the latest GMAT syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup