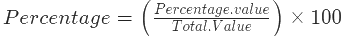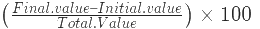Practice Questions: Percentages | Quantitative Aptitude for SSC CGL PDF Download
Definition
The term "Percentage" originates from the Latin word "per centum," signifying "by the hundred." It is symbolized by %. For instance, if we express 5%, it is equivalent to 5/100, which simplifies to 0.05.

Finding the Percentage of a Number
- Explain how to calculate a percentage of a given number using the formula:

- Provide examples and step-by-step explanations of solving percentage problems.
Percentage Increase and Decrease
- Discuss how to determine the percentage increase or decrease between two values using the formula: Percentage Change =

- Provide practical examples, such as price changes, population growth rates, or test score improvements.
Applications of Percentages in Real Life
- Examine diverse situations where percentages find common application, including scenarios involving discounts and sales, interest rates, inflation, and fluctuations in the stock market.
- Elaborate on the significance of comprehending percentages in the realm of personal finance and decision-making.
Calculating Percentages in Statistics
- Examine the involvement of percentages in statistics, encompassing tasks like determining proportions, relative frequencies, and percentages in surveys or data analysis.
- Emphasize the importance of percentages in depicting data accurately and facilitating meaningful comparisons.
Solving Percentage Problems
- Present a set of exercises that span various percentage scenarios, incorporating tasks such as calculating percentages, solving for original values, and determining percentage changes.
- Furnish detailed solutions and explanations in a step-by-step manner to assist users in comprehending the problem-solving approach.
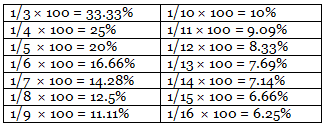
Fraction to Percentage Conversion
Examples on Percentages
Example1: Luaa spends her monthly salary in the following manner: 20% on house rent, 20% on food, 5 % on transportation, 10% on the education, and 20% on other household expenses. She saves the remaining amount of ₨. 5000 at the end of the month. Find out her monthly salary?
(a) Rs. 35000
(b) Rs. 25000
(c) Rs. 20000
(d) Rs. 30000
Ans: (c)
Let the Monthly Salary = 100%
Monthly Expenditure = 20% + 20% + 5% + 10% + 20% = 75%
Monthly Savings = 100% – 75% = 25%
Now, 25% of salary saved = 5000
Let’s take 100% salary as x
25% of x = 5000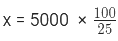
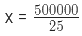
x = 20000
Therefore, her monthly salary = Rs. 20000

Example 2: Aman spends 60% of his income. Suppose his income is increased by 21% and his expenditure increases by 5%, then what is the increase in his savings (in percentage)?
(a) 60%
(b) 18%
(c) 40%
(d) 45%
Ans: (d)
Let Aman’s income = 100
Expenditure = 60 and
Savings = 40 (We get 40 by subtracting, 100 – 60)
New Income is increased by 21% = 100 + 21 = 121
Expenditure Increased by 5% = 5% more than 60 = 105% of 60 = 63
New Savings = 121 – 63 = 58
Therefore, increase in savings = 58 – 40 = 18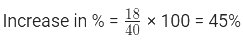
Questions based on Percentages
Q1: A man made a contribution of 7% of his income to a needy person and saved 25% of the balance. If he now has, Rs. 2100 left, then find his actual income?
(a) 9626.26
(b) 9727.27
(c) 3010.75
(d) 10000
Ans: (c)
Let's assume the man's actual income is "X" rupees.
Step 1: Contribution to the needy person Amount contributed = 7% of X = 0.07X
Remaining balance = X - 0.07X = 0.93X
Step 2: Savings from the remaining balance Amount saved = 25% of (0.93X) = 0.25 * 0.93X = 0.2325X
Remaining balance after savings = 0.93X - 0.2325X = 0.6975X
Step 3: The remaining balance is given as Rs. 2100 0.6975X = 2100
Now, solve for X: X = 2100 / 0.6975 ≈ 3010.75
So, the correct value for the man's actual income is indeed Rs. 3010.75.
Q2: In an examination, 40% are passing percentage. If a person gets 41 marks and fails by 3 marks, what are the maximum marks?
(a) 110
(b) 100
(c) 120
(d) 150
Ans: (a)
Total passing marks = 41 + 3 = 44
Let maximum marks be m
Then, 40% of m = 44
= 40m/100 = 44
40m = 4400
m = 110
Q3: Reema saves 51% of his total income of Rs. 15000 per month. Calculate his total spending.
(a) 7350
(b) 7550
(c) 6500
(d) 8560
Ans: (a)
Money spent = (100 – 51) of 15000
= 49% of 15000
= 49/100*15000 = 7350
Q4: If Jenny’s income is 30% more than that of Nikita, how much percent of Nikita’s income is less than that of Jenny?
(a) 23.08%
(b) 25.5%
(c) 27.60%
(d) 29%
Ans: (a)
Let Nikita’s income be 100
Then, Jenny’s income = 130
If Jenny’s income is 130, Nikita’s income = 100
If Jenny’s income is 100, Nikita’s income = 100/130 *100 = 76.92
Therefore, Nikita’s income is less than Jenny’s income by 100 – 76.92 = 23.08%
Q5: If the price of an article is increased by 18%, determine by how much percent must a user reduce the use, so that expenditure on it remains unchanged?
(a) 13.24%
(b) 16%
(c) 25%
(d) 15.3%
Ans: (d)
Decrease in consumption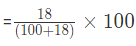
= 15.3%
|
314 videos|170 docs|185 tests
|
FAQs on Practice Questions: Percentages - Quantitative Aptitude for SSC CGL
| 1. What is the definition of percentages? |  |
| 2. Can you provide some examples of percentages in real life? |  |
| 3. How are percentages used in solving mathematical problems? |  |
| 4. How can percentages be converted into decimals or fractions? |  |
| 5. What are some common questions related to percentages in exams or interviews? |  |