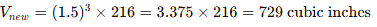Overview: Cubes and Cube Roots

Table of Contents
1. What is a Cube?
2. What is a Cube Root?
3. The Cube Root Symbol
4. You Can Also Cube Negative Numbers
5. Perfect Cubes
6. Examples
View more

Cubes and cube roots often appear in various real-world contexts, from calculating the volume of objects to understanding growth patterns and exponential increases. For example, determining the volume of a box or calculating a growth rate over time relies on these principles. 
In GMAT, mastering cubes and cube roots can provide you with quick shortcuts and insights to solve problems involving large numbers, measurements, and mathematical operations.

What is a Cube?

• The cube of a number is the result of multiplying that number by itself three times.
•  In other words, for any number y, the cube of $\mathrm{<br\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:20px;letter-spacing:inherit"><br>}x$y is given by y x y x y = y³  
• Cube of 3 = 3 x 3 x 3 = 27
  Example: What is 3 Cubed?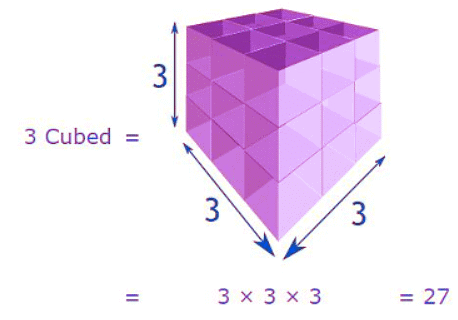
  Note: we write "3 Cubed" as 3³
  (The 3 in superscript means the number appears three times in multiplying)

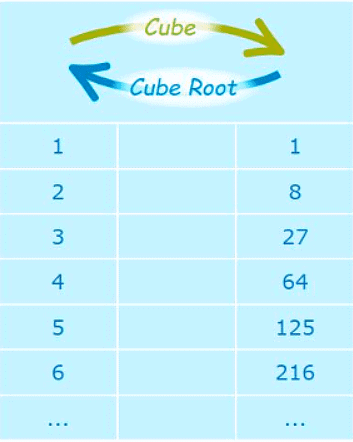
Cubes From 0³ to 6³

• 0 ³ = 0 × 0 × 0 = 0
• 1³  = 1 × 1 × 1 = 1
• 2³ =  2 × 2 × 2 = 8
• 3³ = 3 × 3 × 3 = 27
• 4³ = 4 × 4 × 4 = 64
• 5³ = 5 × 5 × 5 = 125
• 6³ = 6 × 6 × 6 = 216

What is a Cube Root?

• The cube root of a number is a value that, when multiplied thrice, gives back the original number. If we have a number 27$x$, the cube root of 27 is a number 3 such that: 3 x 3 x 3  = 27
• A cube root goes the other direction: 3 cubed is 27, so the cube root of 27 is 3.
• Some of the cubes and cube roots are given below
• Example: Find the cube root of 343?
  Sol: 343 = 7 x7 x 7
  Therefore , cuberoot of 343 = 7

The Cube Root Symbol

• The symbol of cube root is given below
• This is the special symbol that means "cube root", it is the "radical" symbol (used for square roots) with a little three to mean cube root.
• You can use it like this

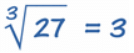
You Can Also Cube Negative Numbers

Have a look at this:
When we cube +5 we get +125: +5 × +5 × +5 = +125
When we cube -5 we get -125: -5 × -5 × -5 = -125
So the cube root of -125 is -5

Perfect Cubes

The Perfect Cubes are the cubes of the whole numbers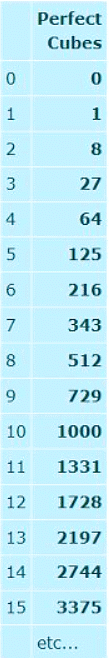
It is easy to work out the cube root of a perfect cube, but it is really hard to work out other cube roots.

Examples

Example 1: If $xx$ and $yy$ are positive integers such that $x\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup>}+y\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup>}=1000x^3\; +\; y^3\; =\; 1000$ and $x>yx\; >\; y$, find the values of $xx$ and $yy$

$\mathrm{a.\; x}=10,y=0x\; =\; 10,\; y\; =\; 0$

$\mathrm{b.\; x}=9,y=7x\; =\; 9,\; y\; =\; 7$

$\mathrm{c.\; x}=8,y=6x\; =\; 8,\; y\; =\; 6$

$\mathrm{d.\; x}=10,y=5x\; =\; 10,\; y\; =\; 5$

Sol: We are given $x\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup>}+y\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup><br\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:20px;letter-spacing:inherit"><br>}=1000$  Start by checking possible integer values of $xx$ and $yy$:
For $x=10$$\mathrm{ ,\; 1}0\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup><br\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:20px;letter-spacing:inherit"><br>}=100010^3\; =\; 1000$  and $y=0y\; =\; 0$ would give $0\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup><br\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:20px;letter-spacing:inherit"><br>}=00^3\; =\; 0$. 
So, $10\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup>}+0\mathrm{<sup\; style="word-break:break-word;line-height:1.8;font-family:lato,sans-serif;font-size:13px\; !important;letter-spacing:inherit">3</sup>}=100010^3\; +\; 0^3\; =\; 1000$+0=1000, which works.
Therefore, the solution is x=10 and $y\; =\; 0$y=0.

Answer: Option 1

Example 2: A rectangular solid has a volume of 216 cubic inches. If the length of each edge is increased by 50%, what will be the new volume of the solid?

a. 324 cubic inches

b. 486 cubic inches

c. 512 cubic inches

d. 648 cubic inches

Sol: The volume of a rectangular solid is the product of its length, width, and height. If the edge length is increased by 50%, the new edge length is 1.5 times the original.
If the original volume is V=216 cubic inches, the new volume will be