Introduction to Algebra | Quantitative for GMAT PDF Download
GMAT algebra questions cover almost 16.3% of thequantitative reasoning section.Approximately, 15 questions appear from algebra, so you need to prepare for this section with regular practice sessions.
Basic GMAT algebra concepts include Inequalities, Functions, Quadratic, and Linear Equations. Remember that you are not allowed to use any form of calculator to answer your questions.
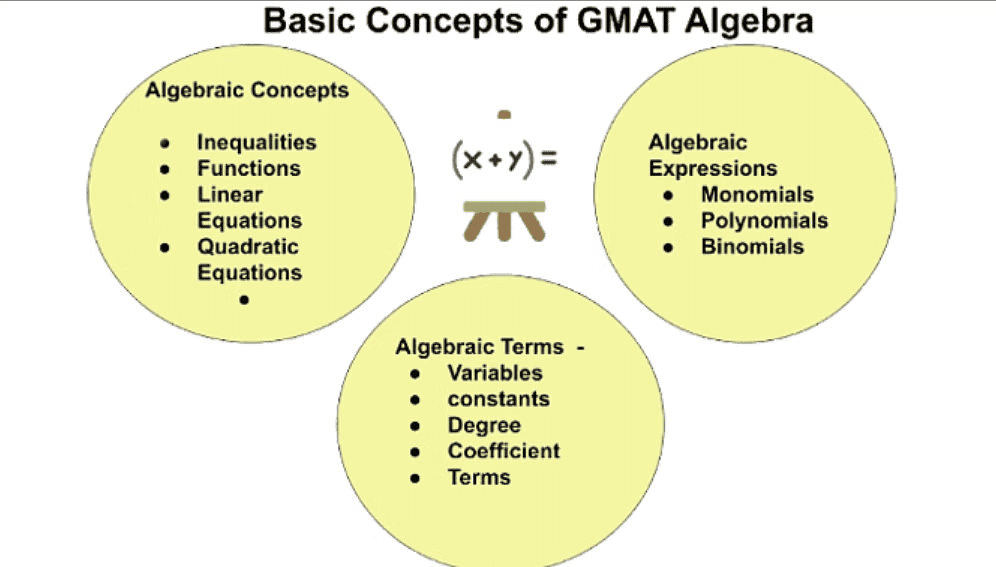
Now, we will first study Algebraic terms, Algebraic expressions, and Algebraic concepts respectively to build a good conceptual knowledge.
Basics of Algebra
GMAT algebra syllabus contains the basics of algebraic terms that you need to get familiarized with before digging deeper into algebraic expressions. The basic algebra terminologies to solve the GMAT algebra questions are given below- Variables
They are the symbols that represent the variable integers in GMAT algebra.
x and y are variables in the equation 9x + 4y + 2 as their value is not defined. - Constants
Constants are the values that do not change in algebraic problems.
2 is a constant term in equation 9x + 4y + 2 as it has one unique and defined value. - Terms
These are formed when both the variables and constants are combined in the algebraic concepts.
For example, in the expression 9x + 4y + 2: 9x, 4y, and 2 are three terms. - Algebraic Expression
GMAT algebra formulas like (x + 2), (x – 3c), and (2x – 3y) are all examples of algebraic expressions, which have terms jumbled together by addition or deduction. - Degree
The power of the variables is measured by its degrees.
The highest power of the variable is called a degree. - Coefficient
It refers to a number or a symbol that is used to indicate a property or a set of properties.
In the expression 9x + 4y + 2, Coefficient of x = 9 and Coefficient of y = 4
Algebraic Expressions
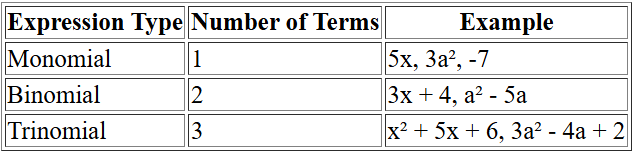
There will be many instances where we have to figure out whether the given expression is a monomial, Binomial or a polynomial.
Now we will look into this topic in depth.
1. Monomial
- Definition: A monomial is an algebraic expression that consists of a single term.
- It is made up of a constant, a variable, or the product of constants and variables raised to non-negative integer powers.
- A monomial has no addition or subtraction involved.
- Example:
5x (This is a monomial with one term: 5x)
3a² (This is a monomial with one term: 3a²)
-7 (This is a monomial because it is just a constant term) - Characteristics:
1. Contains only one term.
2. The powers of variables are non-negative integers (like x², y³, etc.).
3. There is no addition or subtraction within the expression.
2. Binomial
- Definition: A binomial is an algebraic expression that consists of two distinct terms connected by an addition (+) or subtraction (-) sign.
- Binomials can involve variables, constants, or a combination of both.
- Example:
3x + 4 (This is a binomial with two terms: 3x and 4)
a² - 5a (This is a binomial with two terms: a² and -5a)
2x³ + 3x² (This is a binomial with two terms: 2x³ and 3x²) - Characteristics:
1. Contains exactly two terms.
2. The terms are connected by either an addition (+) or a subtraction (-) sign. - Binomials are often used in factoring and solving equations.
3. Trinomial
- Definition: A trinomial is an algebraic expression that consists of three terms connected by addition (+) or subtraction (-) signs.
- Trinomials are common in quadratic equations, factoring problems, and polynomial expressions.
- Example:
x² + 5x + 6 (This is a trinomial with three terms: x², 5x, and 6)
3a² - 4a + 2 (This is a trinomial with three terms: 3a², -4a, and 2)
4x³ - 2x² + x (This is a trinomial with three terms: 4x³, -2x², and x) - Characteristics:
Contains exactly three terms.
The terms are connected by addition (+) or subtraction (-).
Trinomials are common in algebraic expressions such as quadratic equations, which are key for GMAT problem-solving.
Key Difference at a Glance
Concepts of Algebra
GMAT Algebra question types include linear and quadratic equations, functions, inequalities, exponents, and solving linear equations with different variables. All these sections evaluate your problem-solving and analytical skills in the GMAT test. GMAT scores in the quantitative section carry much weightage in algebra.
1. Inequalities
- GMAT algebra concepts include inequalities that work with non-equal comparisons between 2 numbers or other mathematical concepts.
- The fundamental concepts are - Mathematical operations with inequalities, Functioning with ranges of numbers, Transitive property, and Addition of like Qualities.
- The four signs of GMAT inequality are -
Inequality sign
Variables x and y
interpretations
>
x > y
x is greater than y
<
X < y
x is lesser than y
≥
x ≥ y
x is greater than or equal to y
≤
x ≤ y
x is lesser than or equal to y
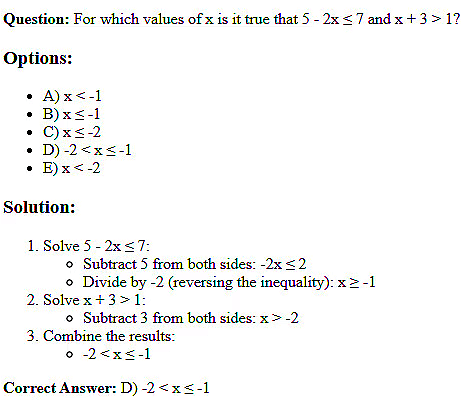
- One of the sample questions from inequalities for your understanding is given below –
- Example:

2. Functions
A function (f(x) is defined by an equation or rule that takes a value (often denoted as ( x )) as input and produces a unique result, or output, based on that input. The notation ( f(x) is read as "f of x" and represents the output value when the input is ( x ).
Types of functions that are commonly encountered in GMAT are written below
1. Linear Functions
Represented by f(x) = mx + b , where ( m ) is the slope and ( b ) is the y-intercept.
These functions graph as straight lines and describe relationships with a constant rate of change.
Example: f(x) = 3x + 52. Quadratic Functions
Represented by ( f(x) = ax2 + bx + c ), where ( a ), ( b ), and ( c ) are constants.
Quadratic functions graph as parabolas and are common in GMAT questions involving areas, velocities, or maximizing/minimizing values.
Example: f(x) = x2 - 4x + 33. Exponential Functions
Represented by f(x) = a bx, where ( a ) and ( b ) are constants.
Exponential functions show rapid increases or decreases and often appear in growth or decay problems.
Example: f(x) = 2 .3x4. Absolute Value Functions
Represented by f(x) = |x|
Absolute value functions measure distance from zero and are always non-negative.
Example: f(x) = |x - 5|
3. Quadratic Equations
Quadratic equations are another concept in the GMAT algebra syllabus and are considered one of the complex parts of algebraic expressions.
It deals with the factoring method of quadratic equations, determining solutions for the Difference of the Perfect squares, Root, and quadratic formula.
Roots of a quadratic equation
The roots of the quadratic equation are nothing but the solutions of the equations. A quadratic equation can have a maximum of two roots - it can have 0 to 1 or 2 roots.The roots can be found by applying two methods - the factoring method and the quadratic formula.
By the factor method
To factor a quadratic equation,
Step 1) - We need to take two numbers such that their sum/difference is and product is
Step 2) - Write the middle term as the sum or difference of the two numbers.
Step 3) - Factor the first two terms and last two terms.
Step 4) - Now both the terms should have a common factor. Take that common factor out.
So, this complete process is the factor method.
Hence, the quadratic equation becomes
4. Linear Equation
Example: If 5x + 2 = 3x + 18, what is the value of x?
a) 4
b) 6
c) 8
d) 10
e) 12Sol: Start with the equation:
5x + 2 = 3x + 18
Move all terms involving x to one side by subtracting 3x from both sides:
5x - 3x + 2 = 18
2x + 2 = 18
Move the constant term to the other side by subtracting 2 from both sides:
2x = 16
Divide both sides by 2 to solve for x:
x = 8
Algebraic Formulas
There are three important algebra formulas that you need to mug up to solve the GMAT algebra questions. The formulas are given below -
- The difference in Two Squares: a2–b2=(a−b)(a+b)
- Squaring a Binomial: (a±b)2=a2±2ab+b2
- The Discriminant: D=b2–4ac
Example: If x2 - 25 = 0, what is the value of x?
a) 5
b) -5
c) ±5
d) 25
e) 0
Sol: Recognize that x2 - 25 is a difference of squares, which can be factored as:
x2 - 25 = (x - 5)(x + 5) = 0
Set each factor equal to zero:
x - 5 = 0 → x = 5
x + 5 = 0 → x = -5
So, x = ±5.
|
115 videos|106 docs|113 tests
|
FAQs on Introduction to Algebra - Quantitative for GMAT
| 1. What is an algebraic expression? |  |
| 2. How do you simplify an algebraic expression? |  |
| 3. What are like terms in algebra? |  |
| 4. Can you explain what a variable is in algebra? |  |
| 5. What is the difference between an equation and an expression? |  |