Class 10 Exam > Class 10 Notes > Mathematics for GCSE/IGCSE > Converting between FDP
Converting between FDP | Mathematics for GCSE/IGCSE - Class 10 PDF Download
FDP Conversions
How do I convert from a percentage to a decimal?
To convert a percentage to a decimal, divide by 100, which involves moving the digits two places to the right.
- For instance, 6% as a decimal would be 6 ÷ 100 = 0.06
- Similarly, 40% as a decimal would be 40 ÷ 100 = 0.4
- Another example is 350% as a decimal, which would be 350 ÷ 100 = 3.5
- Lastly, 0.2% as a decimal would be 0.2 ÷ 100 = 0.002
How do I convert from a decimal to a percentage?
To convert a decimal to a percentage, multiply by 100, shifting the digits two places to the left and adding a % sign.
- For example, 0.35 as a percentage would be 0.35 × 100 = 35%
- Likewise, 1.32 as a percentage would be 1.32 × 100 = 132%
- Additionally, 0.004 as a percentage would be 0.004 × 100 = 0.4%
How do I convert from a decimal to a fraction?
- If it has one decimal place, write the digits over 10
- 0.3 is 3/10
- 1.1 is 11/10
- If it has two decimal places, write the digits over 100
- 0.07 is 7/100
- 0.13 is 13/100
- 30.01 is 3001/100
- If it has n decimal places, write the digits over 10^n
- 0.513 is 513/1000
- 0.0007 is 7/10000
- Learn simple recurring decimals as fractions
- 0.33333... = 1/3
- 0.66666... = 2/3
- Whole numbers can be written as fractions (by writing them over 1)
- 5 is 5/1
How do I convert from a percentage to a fraction?
Write the percentage over 100
- 37% is 37/100
How do I convert from a fraction to a decimal?
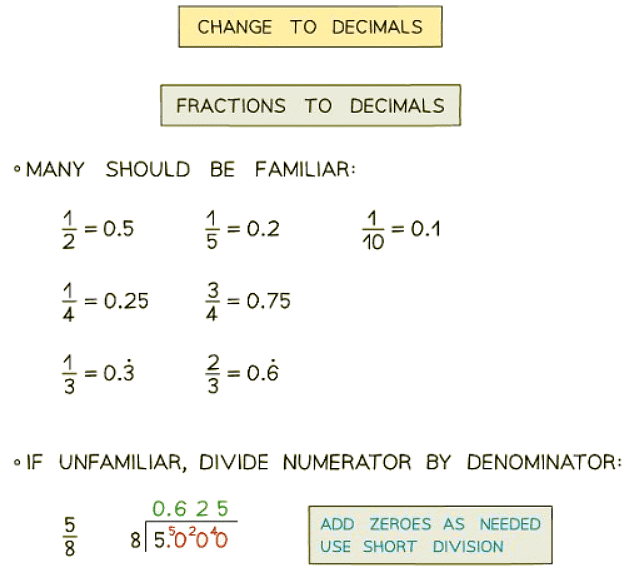
- Fractions written over powers of 10 are quicker
- 3/5 = 6/10 which is 0.6
- 7/20 = 35/100 which is 0.35
- 1/500 = 2/1000 which is 0.002
How do I convert from a fraction to a percentage?
Change fractions into decimals (see above), then multiply by 100
- 4/5 = 8/10 which is 0.8 as a decimal, which is 0.8 × 100 = 80%.
Recurring Decimals
What are recurring decimals?
- A rational number is any number that can be written as an integer (whole number) divided by another integer
- A number written as p/q in its simplest form, where p and q are integers is rational
- When you write a rational number as a decimal, you either get a decimal that stops (e.g. ¼ = 0.25), called a "terminating" decimal, or one that repeats with a pattern (e.g. ⅓ = 0.333333…), called a "recurring" decimal
- The recurring part can be written with a dot (or dots on the first and last recurring digit)
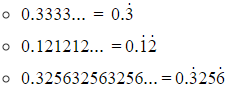
How do I write recurring decimals as fractions?
When dealing with recurring decimals, we can convert them into fractions following a systematic approach:
- Write out the recurring decimal as f and identify the repeating pattern.
- Multiply both sides of the equation by 10 successively until the recurring part aligns.
- Subtract the two lines to eliminate the recurring part.
- Divide both sides to express f as a fraction in its simplest form.
Question for Converting between FDPTry yourself: How do you convert a decimal to a percentage?View Solution
The document Converting between FDP | Mathematics for GCSE/IGCSE - Class 10 is a part of the Class 10 Course Mathematics for GCSE/IGCSE.
All you need of Class 10 at this link: Class 10
|
42 videos|395 docs|19 tests
|
FAQs on Converting between FDP - Mathematics for GCSE/IGCSE - Class 10
| 1. How do you convert a recurring decimal into a fraction? |  |
Ans. To convert a recurring decimal into a fraction, you can set up an equation where the repeating part of the decimal represents a variable. Solve for the variable to find the fraction form.
| 2. What is the difference between a terminating decimal and a recurring decimal? |  |
Ans. A terminating decimal has a finite number of digits after the decimal point, while a recurring decimal has a pattern of digits that repeats indefinitely.
| 3. Can all recurring decimals be converted into fractions? |  |
Ans. Yes, all recurring decimals can be converted into fractions by using methods like long division or algebraic manipulation.
| 4. How can you determine if a decimal is recurring or terminating? |  |
Ans. If a decimal has a repeating pattern of digits after the decimal point, it is considered a recurring decimal. If the digits stop and do not repeat, it is a terminating decimal.
| 5. Are recurring decimals rational numbers? |  |
Ans. Yes, recurring decimals are rational numbers because they can be expressed as a ratio of two integers.
Related Searches
















