Notes: Mensuration | Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET PDF Download
| Table of contents |

|
| Area |

|
| Perimeter |

|
| Area of Trapezium |

|
| Area of a General Quadrilateral |

|
| Area of a Polygon |

|
| Solid Shapes |

|
| Volume |

|
| Volume and Capacity |

|
Mensuration is a branch of mathematics that deals with the measurement of geometric figures and their properties such as length, area, and volume. It's an important topic for the CTET and other educational exams. Here's a brief overview:
Mensuration involves calculating the dimensions, area, and volume of various geometric shapes and figures. It is applicable to both two-dimensional (2D) and three-dimensional (3D) shapes.
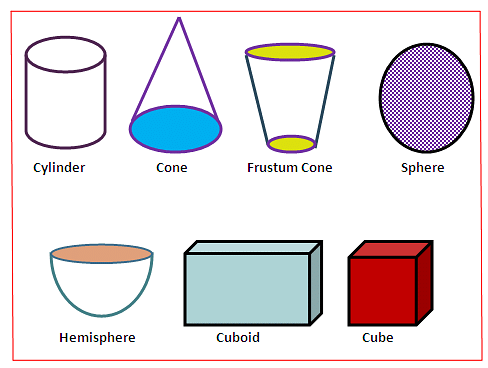
It is all about the measurement of area, perimeter and volume of the plane(2D) and solid(3D) figures.
Area
The surface covered by the border line of the figure is the area of the plain shape.
Unit of the area is square of the length unit.
Perimeter
The perimeter is the length of the boundary of the plane shape.
The unit of the perimeter is same as the length unit.
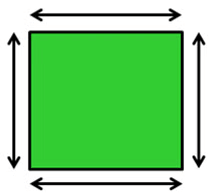
The green part is the area of the square and the distance all the way around the outside is the perimeter.
Area and Perimeter of Some 2D Shapes
| Shape | Image | Area | Perimeter |
| Square |  | (Side)2 | 4 × Side |
| Rectangle |  | Length × Breadth | 2(Length + Breadth) |
| Triangle |  | (1/2) × Base × Height | a + b + c (where a, b and c are the three sides of the triangle) |
| Parallelogram |  | Base × Height | 2(sum of adjacent sides) |
| Circle |  | πr2 | 2πr (where, r = radius of the circle) |
Area of Trapezium
A trapezium is a quadrilateral whose two sides are parallel. And if its non-parallel sides are equal then it is said to be an isosceles trapezium.
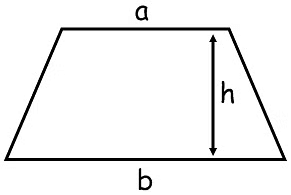
We can find the Area of Trapezium by following these steps:
1. By Splitting the figure
One way to find the Area of trapezium is to divide it into two or three plane figures and then find the area.
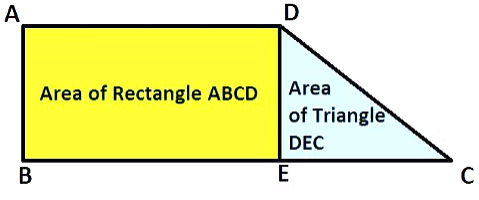
In the trapezium ABCD,
It can be divided into two parts i.e. a rectangle and a triangle.
Area of ABCD = Area of ABED + Area of DEC
2. By using formula
Another way is to calculate the area by using formula.
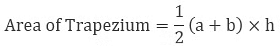
Area of trapezium is half of the product of the summation of the parallel sides and the perpendicular distance between them.
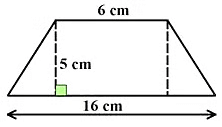
Example: Find the area of the trapezium whose parallel sides are 6 cm and 16 cm, with a height of 5 cm. Calculate the area using both the methods.
Solution:
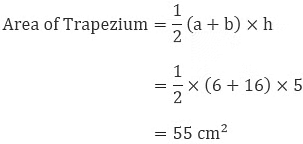
Splitting the trapezium we get –
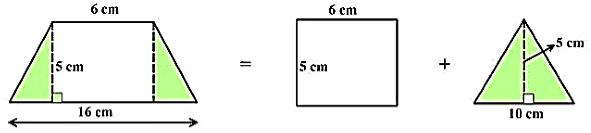
Area of the trapezium = Area of rectangle + Area of triangle
= (6 x 5) + (1/2) x 5 x 10
= 30 + 25
= 55 cm2
Remark: We should use the formula most of the time if possible as it is the quick and easy method.
Area of a General Quadrilateral
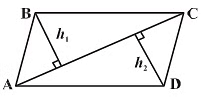
To find the area of any quadrilateral:
We can divide it into two triangles and then the area can be easily calculated by calculating the area of both the triangles separately.
Area of ABCD = Area of ∆ABC + Area of ∆ACD
= (1/2) × AC × h1 + (1/2) × AC× h2
The formula for the Area of a General Quadrilateral

Where h1 and h2 are the height of both the triangles and d is the length of the common diagonal i.e. AC.
Example: Find the area of quadrilateral ABCD.
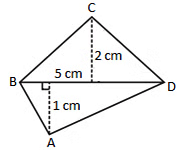
Solution:
In the quadrilateral ABCD,
BD is the common diagonal so d = 5 cm.
Height of the two triangles are h1 = 2 cm and h2 = 1 cm.
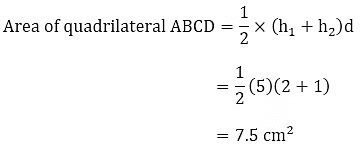
Area of Special Quadrilaterals (Rhombus)
A rhombus is a quadrilateral with all the sides are equal and parallel but not making the right angle like in square. Its two diagonals are the perpendicular bisector to each other.
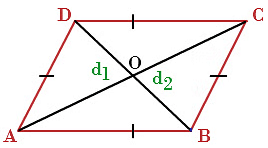
In this also we can split the rhombus into two triangles and can find the area of rhombus easily.
Formula of Area of Rhombus
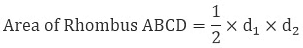
Area of rhombus is half of the product of its two diagonals.
Area of a Polygon
There is no particular formula for the area of the polygon so we need to divide it into a possible number of figures like a triangle, rectangle, trapezium and so on. By adding the area of all the split figures we will get the area of the required polygon.
Example: Find the area of the given octagon.
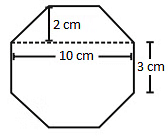
Solution:
We can divide the given octagon into three parts.
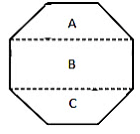
Two trapezium A and B and one rectangle shown by part B.
Two trapezium A and B and one rectangle shown by part B.
Area of A = Area of B = (1/2) × (a + b) × h
= (1/2) x (10 + 3) × 2
= 13 cm2.
Area of B = Length x Breadth
= 10 x 3
= 30 cm2.
So, the area of Octagon = 2A + B
= 2 × 13 + 30
= 56 cm2.
Solid Shapes
The 3-dimensional shapes which occupy some space are called solid shapes. Example: Cube, Cylinder, Sphere etc.
Surface Area
If we draw the net of the solid shape then we can see it’s all the faces clearly and if we add the areas of all the faces then we get the total surface area of that solid shape. The unit of surface area is a square unit.
Lateral or Curved Surface Area
If we leave the top and bottom faces of the solid shape then the area of the rest of the figure is the lateral surface of the shape. The unit of lateral surface area is a square unit.
Surface Area of Cube, Cuboid and Cylinder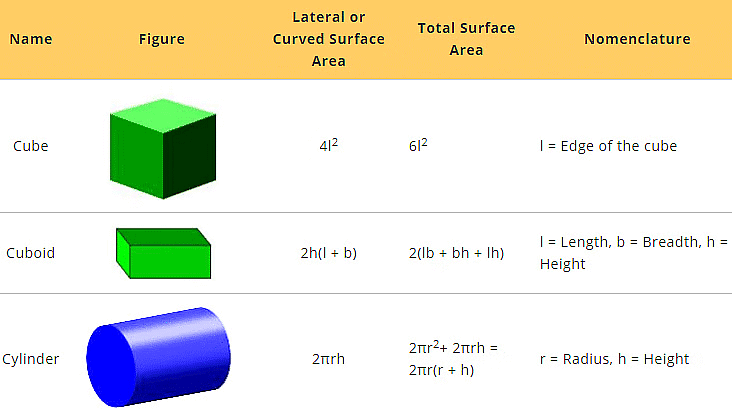
Volume
Volume is the space occupied by any solid figure i.e. the amount of capacity to carry something is the volume of that solid shape. The unit of volume is a cubic unit.
Volume of Cube, Cuboid and Cylinder
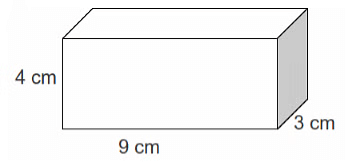
Example : There is a shoe box whose length, breadth and height is 9 cm, 3 cm and 4 cm respectively. Find the surface area and volume of the shoe box.
Solution:
Given,
length = 9 cm
Breadth = 3 cm
Height = 4 cm
Area of cuboid = 2(lb + bh + lh)
= 2(9 × 3 + 3×4 + 9 × 4)
= 2(27 + 12 + 36)
= 2(75)
= 150 cm2
Volume of cuboid = lbh
= 9 × 3 × 4
= 108 cm3
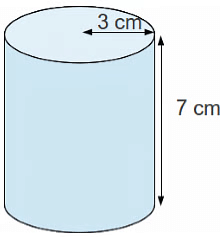
Example : If there is a cold drink can whose height is 7 cm and the radius of its round top is 3 cm then what will be the lateral surface area and volume of that cylinder? (π = 3.14)
Solution:
Given,
radius = 3 cm
Height = 7 cm
Lateral surface area of cylinder = 2πrh
= 2 × 3.14 × 3 × 7
= 131.88 cm2
Volume of cylinder = πr2h
= 3.14 × 3 × 3 × 7
= 197.82 cm3
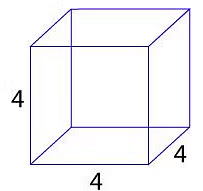
Example : If there is a box of cube shape with the length of 4 cm then what will be the capacity of this box. Also, find the surface area of the box if it is open from the top.
Solution:
Given, side = 4 cm
Capacity or volume of the box = s3
= 43 = 64 cm3
The total surface area of the box = 6s2
But, if the box is open from the top then the surface area will be total surface area minus the area of one face of the cube.
Surface Area = Total Surface Area - Area of one face
= 6s2 – s2
= 5s2 = 5 × 42
= 80 cm2
Volume and Capacity
- Volume and capacity are one and the same thing.
- Volume is the amount of space occupied by a shape.
- Capacity is the quantity that a container can hold.
- Capacity can be measured in the form of liters.
We can see the relation between liter and cm3 as,
1 L = 1000 mL
1 mL = 1 cm3,
1 L = 1000 cm3.
Thus, 1 m3 = 1000000 cm3 = 1000 L.
|
82 videos|273 docs|69 tests
|
FAQs on Notes: Mensuration - Mathematics & Pedagogy Paper 2 for CTET & TET Exams - CTET & State TET
| 1. What is the formula for the area of a trapezium? |  |
| 2. How do you calculate the area of a general quadrilateral? |  |
| 3. What is the formula to find the volume of solid shapes like cubes and cylinders? |  |
| 4. How can I determine the area of a polygon? |  |
| 5. What is the difference between volume and capacity in mensuration? |  |















