UPSC Exam > UPSC Notes > Civil Engineering Optional Notes for UPSC > Euler’s Equation: The Equation of Motion of an Ideal Fluid
Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC PDF Download
Euler’s Equation: The Equation of Motion of an Ideal Fluid
This section is not a mandatory requirement. One can skip this section (if he/she does not like to spend time on Euler's equation) and go directly to Steady Flow Energy Equation.Using the Newton's second law of motion the relationship between the velocity and pressure field for a flow of an inviscid fluid can be derived. The resulting equation, in its differential form, is known as Euler’s Equation. The equation is first derived by the scientist Euler.
Derivation:
Let us consider an elementary parallelopiped of fluid element as a control mass system in a frame of rectangular cartesian coordinate axes as shown in Fig. 12.3. The external forces acting on a fluid element are the body forces and the surface forces.
Fig 12.2 A Fluid Element appropriate to a Cartesian Coordinate System used for the derivation of Euler's Equation
Let Xx, Xy, Xz be the components of body forces acting per unit mass of the fluid element along the coordinate axes x, y and z respectively. The body forces arise due to external force fields like gravity, electromagnetic field, etc., and therefore, the detailed description of Xx, Xy and Xz are provided by the laws of physics describing the force fields. The surface forces for an inviscid fluid will be the pressure forces acting on different surfaces as shown in Fig. 12.3. Therefore, the net forces acting on the fluid element along x, y and z directions can be written as
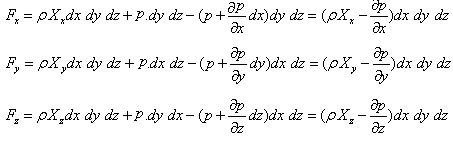
Since each component of the force can be expressed as the rate of change of momentum in the respective directions, we have
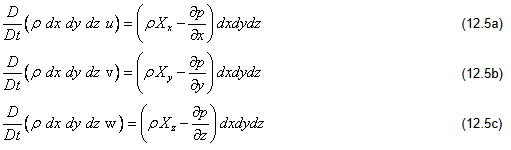
the mass of a control mass system does not change with time,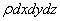 
is constant with time and can be taken common. Therefore we can write Eqs (12.5a to 12.5c) as
Expanding the material accelerations in Eqs (12.6a) to (12.6c) in terms of their respective temporal and convective components, we get
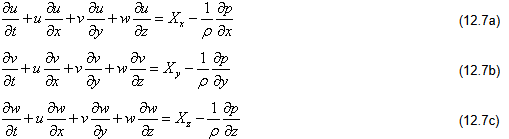
The Eqs (12.7a, 12.7b, 12.7c) are valid for both incompressible and compressible flow. By putting u = v = w = 0, as a special case, one can obtain the equation of hydrostatics .
Equations (12.7a), (12.7b), (12.7c) can be put into a single vector form as
where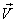 
the velocity vector and the body force vector per unit volume 
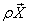are defined as
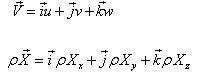
Equation (12.7d) or (12.7e) is the well known Euler’s equation in vector form, while Eqs (12.7a) to (12.7c) describe the Euler’s equations in a rectangular Cartesian coordinate system.
The document Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC is a part of the UPSC Course Civil Engineering Optional Notes for UPSC.
All you need of UPSC at this link: UPSC
|
350 videos|464 docs|2 tests
|
FAQs on Euler’s Equation: The Equation of Motion of an Ideal Fluid - Civil Engineering Optional Notes for UPSC
| 1. What is Euler's Equation and how is it related to the motion of an ideal fluid? |  |
Ans. Euler's Equation is a fundamental equation in fluid dynamics that describes the motion of an ideal fluid. It relates the pressure distribution, velocity field, and density of the fluid to the acceleration of the fluid particles.
| 2. What are the key components of Euler's Equation? | |
Ans. The key components of Euler's Equation include the convective acceleration term, pressure gradient term, gravitational acceleration term, and any external force acting on the fluid.
| 3. How is Euler's Equation different from Navier-Stokes Equation? | |
Ans. Euler's Equation is a simplified form of the Navier-Stokes Equation, which includes viscosity and other dissipative effects. Euler's Equation is applicable to ideal fluids, while the Navier-Stokes Equation is used for real fluids.
| 4. How can Euler's Equation be used in practical applications? | |
Ans. Euler's Equation is used in various engineering applications, such as aircraft design, water flow in pipes, and weather prediction models. It helps in understanding the behavior of fluids in various scenarios.
| 5. What are the assumptions made in deriving Euler's Equation for ideal fluids? | |
Ans. The assumptions made in deriving Euler's Equation for ideal fluids include the fluid being inviscid (no viscosity), incompressible, and irrotational. These assumptions simplify the equations and make them easier to solve for ideal fluid flow scenarios.
Related Exams
About this Document
|
Dec 22, 2024 Last updated |
Document Description: Euler’s Equation: The Equation of Motion of an Ideal Fluid for UPSC 2024 is part of Civil Engineering Optional Notes for UPSC preparation.
The notes and questions for Euler’s Equation: The Equation of Motion of an Ideal Fluid have been prepared according to the UPSC exam syllabus. Information about Euler’s Equation: The Equation of Motion of an Ideal Fluid covers topics
like Euler’s Equation: The Equation of Motion of an Ideal Fluid and Euler’s Equation: The Equation of Motion of an Ideal Fluid Example, for UPSC 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Euler’s Equation: The Equation of Motion of an Ideal Fluid.
Introduction of Euler’s Equation: The Equation of Motion of an Ideal Fluid in English is available as part of our Civil Engineering Optional Notes for UPSC
for UPSC & Euler’s Equation: The Equation of Motion of an Ideal Fluid in Hindi for Civil Engineering Optional Notes for UPSC course.
Download more important topics related with notes, lectures and mock test series for UPSC
Exam by signing up for free. UPSC: Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC
Description
Full syllabus notes, lecture & questions for Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC - UPSC | Plus excerises question with solution to help you revise complete syllabus for Civil Engineering Optional Notes for UPSC | Best notes, free PDF download
Information about Euler’s Equation: The Equation of Motion of an Ideal Fluid
In this doc you can find the meaning of Euler’s Equation: The Equation of Motion of an Ideal Fluid defined & explained in the simplest way possible. Besides explaining types of
Euler’s Equation: The Equation of Motion of an Ideal Fluid theory, EduRev gives you an ample number of questions to practice Euler’s Equation: The Equation of Motion of an Ideal Fluid tests, examples and also practice UPSC
tests
|
350 videos|464 docs|2 tests
|
Download as PDF
|
Explore Courses for UPSC exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
past year papers
,practice quizzes
,video lectures
,Important questions
,study material
,Sample Paper
,Semester Notes
,ppt
,Objective type Questions
,MCQs
,Extra Questions
,Free
,Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC
,mock tests for examination
,shortcuts and tricks
,Previous Year Questions with Solutions
,Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC
,Summary
,Exam
,Viva Questions
,Euler’s Equation: The Equation of Motion of an Ideal Fluid | Civil Engineering Optional Notes for UPSC
;
Additional Information about Euler’s Equation: The Equation of Motion of an Ideal Fluid for UPSC Preparation
Euler’s Equation: The Equation of Motion of an Ideal Fluid Free PDF Download
The Euler’s Equation: The Equation of Motion of an Ideal Fluid is an invaluable resource that delves deep into the core of the UPSC exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Euler’s Equation: The Equation of Motion of an Ideal Fluid now and kickstart your journey towards success in the UPSC exam.
Importance of Euler’s Equation: The Equation of Motion of an Ideal Fluid
The importance of Euler’s Equation: The Equation of Motion of an Ideal Fluid cannot be overstated, especially for UPSC aspirants.
This document holds the key to success in the UPSC exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Euler’s Equation: The Equation of Motion of an Ideal Fluid Notes
Euler’s Equation: The Equation of Motion of an Ideal Fluid Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Euler’s Equation: The Equation of Motion of an Ideal Fluid.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Euler’s Equation: The Equation of Motion of an Ideal Fluid Notes on EduRev are your ultimate resource for success.
Euler’s Equation: The Equation of Motion of an Ideal Fluid UPSC Questions
The "Euler’s Equation: The Equation of Motion of an Ideal Fluid UPSC Questions" guide is a valuable resource for all aspiring students preparing for the
UPSC exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Euler’s Equation: The Equation of Motion of an Ideal Fluid on the App
Students of UPSC can study Euler’s Equation: The Equation of Motion of an Ideal Fluid alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Euler’s Equation: The Equation of Motion of an Ideal Fluid,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Euler’s Equation: The Equation of Motion of an Ideal Fluid is prepared as per the latest UPSC syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup