Year 6 Exam > Year 6 Notes > Year 6 Mathematics > Angles in polygons
Angles in polygons | Year 6 Mathematics PDF Download
What is a Polygon?
- Polygons are 2D shapes characterized by straight sides. When polygons are regular, it means that all their sides and angles are of equal measure.
- For instance, squares and equilateral triangles are examples of regular polygons.
Working out the Sum of Internal Angles
To determine the sum of internal angles in a polygon with more than four sides, you can use a formula that applies universally for all polygons: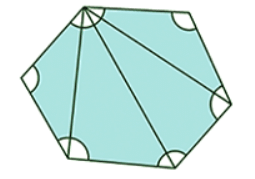
- Sum of internal angles = (number of sides - 2) x 180°.
- After subtracting 2 from the number of sides, you essentially find out how many triangles the polygon can be divided into from a single vertex.
- Subsequently, you multiply by 180°, which represents the sum of internal angles within a triangle.
- This formula can be adapted for various polygons by adjusting the number of sides accordingly.
Regular Polygons: Understanding Internal Angles
Pentagon
A pentagon has five sides. Using the formula, you can calculate the total of the internal angles:
- Sum of internal angles = (5 - 2) x 180°
- Therefore, for a pentagon, the sum of internal angles would be (5 - 2) x 180° = 3 x 180° = 540°.
Hexagon
Since a hexagon has 6 sides, let’s substitute that amount into the formula:
- Sum of internal angles = (6 - 2) x 180
- 720° = 4 x 180
- What would one angle be in a regular hexagon?
- 720° ÷ 6 = 120°
 |
Download the notes
Angles in polygons
|
Download as PDF |
Download as PDF
Heptagon
All heptagons have 7 sides, so the formula to work out the internal angles would be:
- Sum of internal angles = (7 - 2) x 180
- 900° = 5 x 180°
- What would one angle in a regular heptagon be?
- 900° ÷ 7 = 128. 57° (rounded to 2 decimal places)
Octagon
Octagons have 8 sides so again, we need to adjust the formula accordingly:
- Sum of internal angles = (8 - 2) x 180°
- 1080° = 6 x 180°
- In a regular octagon, one angle would be worth:
- 1080° ÷ 8 = 135°
 |
Take a Practice Test
Test yourself on topics from Year 6 exam
|
Practice Now |
Practice Now
Irregular polygons
In irregular polygons, the total sum of the interior angles remains constant, but the value of each individual angle varies since they are of different sizes.
The document Angles in polygons | Year 6 Mathematics is a part of the Year 6 Course Year 6 Mathematics.
All you need of Year 6 at this link: Year 6
|
54 videos|54 docs|17 tests
|
Related Exams
About this Document
Apr 21, 2025
Last updated
Document Description: Angles in polygons for Year 6 2025 is part of Year 6 Mathematics preparation.
The notes and questions for Angles in polygons have been prepared according to the Year 6 exam syllabus. Information about Angles in polygons covers topics
like What is a Polygon?, Working out the Sum of Internal Angles, Regular Polygons: Understanding Internal Angles and Angles in polygons Example, for Year 6 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Angles in polygons.
Introduction of Angles in polygons in English is available as part of our Year 6 Mathematics
for Year 6 & Angles in polygons in Hindi for Year 6 Mathematics course.
Download more important topics related with notes, lectures and mock test series for Year 6
Exam by signing up for free. Year 6: Angles in polygons | Year 6 Mathematics
Description
Full syllabus notes, lecture & questions for Angles in polygons | Year 6 Mathematics - Year 6 | Plus excerises question with solution to help you revise complete syllabus for Year 6 Mathematics | Best notes, free PDF download
Information about Angles in polygons
In this doc you can find the meaning of Angles in polygons defined & explained in the simplest way possible. Besides explaining types of
Angles in polygons theory, EduRev gives you an ample number of questions to practice Angles in polygons tests, examples and also practice Year 6
tests
Related Searches
Free
,Summary
,MCQs
,study material
,shortcuts and tricks
,video lectures
,past year papers
,practice quizzes
,Angles in polygons | Year 6 Mathematics
,Previous Year Questions with Solutions
,Exam
,Angles in polygons | Year 6 Mathematics
,Semester Notes
,Important questions
,Extra Questions
,ppt
,mock tests for examination
,Angles in polygons | Year 6 Mathematics
,Objective type Questions
,Sample Paper
,Viva Questions
,
Additional Information about Angles in polygons for Year 6 Preparation
Angles in polygons Free PDF Download
The Angles in polygons is an invaluable resource that delves deep into the core of the Year 6 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Angles in polygons now and kickstart your journey towards success in the Year 6 exam.
Importance of Angles in polygons
The importance of Angles in polygons cannot be overstated, especially for Year 6 aspirants.
This document holds the key to success in the Year 6 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Angles in polygons Notes
Angles in polygons Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Angles in polygons.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Angles in polygons Notes on EduRev are your ultimate resource for success.
Angles in polygons Year 6 Questions
The "Angles in polygons Year 6 Questions" guide is a valuable resource for all aspiring students preparing for the
Year 6 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Angles in polygons on the App
Students of Year 6 can study Angles in polygons alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Angles in polygons,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Angles in polygons is prepared as per the latest Year 6 syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup