Open App

Class 7 Exam > Class 7 Notes > Mathematics Olympiad Class 7 > Mathematics Olympiad Previous Year Papers - 3

Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7 PDF Download

Q: 1. What topics are covered in the Class 7 Mathematics Olympiad Previous Year Papers?

Ans.The Class 7 Mathematics Olympiad Previous Year Papers typically cover a range of topics including Logical Reasoning, Mathematical Reasoning, Everyday Mathematics, and problem-solving skills that are essential for students at this level.

Q: 2. How can students prepare for the Class 7 Mathematics Olympiad effectively?

Ans.Students can prepare for the Class 7 Mathematics Olympiad by practicing previous year papers, understanding key mathematical concepts, solving sample questions, and focusing on areas of weakness. Regular revision and taking mock tests can also be beneficial.

Q: 3. What is the format of questions in Class 7 Mathematics Olympiad exams?

Ans.The questions in Class 7 Mathematics Olympiad exams are typically multiple-choice questions (MCQs) that assess students’ understanding of mathematical concepts, logical reasoning, and problem-solving skills. Questions may vary in difficulty to challenge students at different levels.

Q: 4. Are there any specific strategies for solving Logical Reasoning questions in the Olympiad?

Ans.Yes, effective strategies for solving Logical Reasoning questions include carefully reading the problem, identifying patterns or sequences, eliminating incorrect options, and practicing regularly to improve analytical skills.

Q: 5. How important is time management during the Class 7 Mathematics Olympiad?

Ans.Time management is crucial during the Class 7 Mathematics Olympiad as students need to answer a set number of questions within a limited timeframe. Practicing under timed conditions can help students learn to pace themselves and ensure they can complete the exam.

Table of contents
Logical Reasoning
Mathematical Reasoning
Everyday Mathematics
Achievers Section

Note: The questions provided in this document are similar to the questions that were asked in the actual Olympiad exam. So, we recommend you study these for your Olympiad preparation

Logical Reasoning

Q1: The number of measurements required to construct a quadrilateral is:
(a) 5
(b) 4
(c) 3
(d) 2

Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

View Answer

Ans: (a)

Minimum number of measurement required to construct a unique quadrilateral.

Q2: Which of the following is true for constructing a quadrilateral?
(a) 5 sides and 3 diagonals
(b) 3 sides only
(c) 3 angles and their 2 included sides
(d) 2 diagonals only

View Answer

Ans: (c)
3 angles and their 2 included sides. There is only 4 sides and 2 diagonals. So option (a) is not valid. Only 3 sides are not sufficient to draw a quadrilateral. So, option (b) is also invalid. Only 2 diagonals are not sufficient to draw a quadrilateral. So, option (c) is also not valid.

Q3: To construct a convex quadrilateral, which of the following cases is not correct?
(a) When the lengths of four sides and one diagonal are given.
(b) When the lengths of three sides and the two diagonals are given.
(c) When the lengths of four sides and one angle are given.
(d) When the lengths of two sides and two included angles are given.

View Answer

Ans: (d)
Only four measurements are given, which are not sufficient to construct a convex quadrilateral.

Q4: To construct a parallelogram, the minimum number of measurements required is:
(a) 2
(b) 3
(c) 4
(d) 1

View Answer

Ans: (b)
To construct a parallelogram minimum 3 measurements are required.

Q5: The minimum number of dimensions needed to construct a rectangle is:
(a) 1
(b) 2
(c) 3
(d) 4

View Answer

Ans: (b)
Two dimensions are sufficient to construct a rectangle.

Q6: The minimum number of measurements needed to construct a square is:
(a) 1
(b) 2
(c) 3
(d) 4

View Answer

Ans: (a)
Only 1 measurement is sufficient to construct a square.

Q7: According to the construction shown in the diagram below, what do we call segment CD?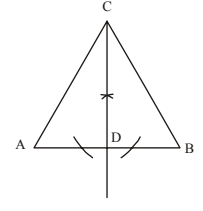

(a) Bisector of angle C
(b) Median to side AB
(c) Perpendicular bisector of segment AB
(d) Altitude to side AB

View Answer

Ans: (d)
Altitude to side AB.

Q8: The point where the medians of a triangle are concurrent is called the:
(a) Centroid
(b) Orthocenter
(c) Incenter
(d) Circumcenter

View Answer

Ans: (a)
Centroid.

Q9: The centroid of a triangle divides the medians into ratios from the vertex, of:
(a) 2 : 1
(b) 3 : 1
(c) 4 : 1
(d) 5 : 1

View Answer

Ans: (a)
2 : 1.

Q10: In relation to constructions, a straightedge is
(a) a clear plastic device devoid of markings.
(b) often shaped like a triangle.
(c) used for drawing straight lines or segments, but not for measuring.
(d) all of the above.

View Answer

Ans: (c)
Used for drawing straight lines or segments but not for measuring.

Q11: Which construction is shown in the accompanying diagram?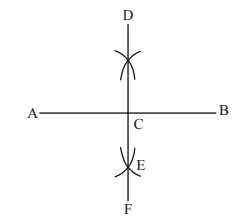

(a) the bisector of ∠ACD
(b) the midpoint of DF
(c) the perpendicular bisector of AB
(d) a perpendicular line to AB from point D

View Answer

Ans: (c)
The perpendicular bisector of AB.

Q12: Which diagram below shows a correct mathematical construction using only a compass and a straightedge to bisect an angle?

(a)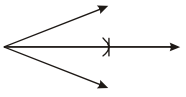

(b)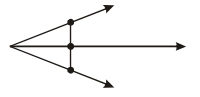

(c)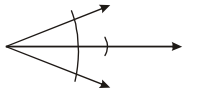

(d)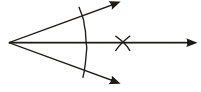

View Answer

Ans: (d)

Q13: How many pairs of letters can be found in the word ORDINAL, where the number of letters between them matches their positions in the English alphabet?
(a) One
(b) Two
(c) Three
(d) None

View Answer

Ans: (c)

To find the pairs of letters in ORDINAL, we need to check the positions of the letters in the English alphabet.
For example, the letters O (15) and R (18) have 2 letters between them (P, Q), which matches their positions.
After checking all combinations, we find that there are three pairs that meet this criterion: O-R, D-I, and N-A.
Thus, the total number of such pairs is three.

Q14: If ‘–’ stands for division, ‘+’ stands for multiplication, ‘÷’ stands for subtraction, and ‘×’ stands for addition, find the value of 75 × 5 ÷ 80 – 16 + 3.
(a) 60
(b) 70
(c) 80
(d) 65

View Answer

Ans: (d)

First, we need to replace the symbols with their meanings: 75 × 5 becomes 75 + 5, ÷ becomes –, – becomes ÷, and + becomes ×.
This translates the expression to: 75 + 5 – 80 ÷ 16 × 3.
Now, we perform the operations in the correct order: first addition, then subtraction, then division, and finally multiplication.
Calculating step by step gives us the final result of 65.

Q15: Select the odd one out.
(a) 43
(b) 80
(c) 15
(d) 90

View Answer

Ans: (a)

The numbers presented are all integers, but they have different properties.
43 is a prime number, meaning it can only be divided by 1 and itself.
80, 15, and 90 are all composite numbers, which means they have more than two factors.
Thus, 43 stands out as the only prime number among the options.

Mathematical Reasoning

Q16: How many 2’s are present in the provided sequence, each of which is directly preceded by an odd number but not directly followed by 4?
(a) None
(b) One
(c) Two
(d) More than two

View Answer

Ans: (c)

To find the number of 2's that meet the criteria, we need to look at the sequence: 4, 5, 2, 9, 7, 6, 2, 1, 5, 2, 8, 9, 3, 4, 5, 7, 9, 2, 4.
A 2 must be preceded by an odd number (1, 3, 5, 7, 9) and not followed by 4.
In the sequence, the 2's that fit this description are the second 2 (preceded by 7) and the third 2 (preceded by 5).
Thus, there are two 2's that satisfy the conditions.

Q17: Pointing towards Shikha, Vansh said, “She is the daughter of the only child of my grandfather.” How is Shikha related to Vansh?
(a) Daughter
(b) Mother
(c) Sister
(d) Aunt

View Answer

Ans: (c)

Vansh refers to Shikha as the daughter of the only child of his grandfather.
The only child of Vansh's grandfather is Vansh's parent (either his mother or father).
This means Shikha is the daughter of Vansh's parent, making her Vansh's sister.
Thus, the correct relationship is that Shikha is Vansh's sister.

Q18: Which of the following value will replace X in the given expression? (–91) ÷ X + 68 ÷ (–17) = –11
(a) 13
(b) 7
(c) 12
(d) 9

View Answer

Ans: (a)

First, simplify the expression: 68 ÷ (–17) equals –4.
Now, the equation becomes (–91) ÷ X – 4 = –11.
Add 4 to both sides: (–91) ÷ X = –7.
To find X, multiply both sides by X and then by –1: X = (–91) ÷ (–7), which gives X = 13.

Q19: The sum of the digits of a two-digit number is 10. If the ten’s digit is one more than two times the unit’s digit, then find the number.
(a) 82
(b) 73
(c) 64
(d) 91

View Answer

Ans: (b)

Let the ten's digit be represented as T and the unit's digit as U.
From the problem, we know that T + U = 10.
We also have the condition that T = 2U + 1.
Substituting T in the first equation gives us (2U + 1) + U = 10, which simplifies to 3U + 1 = 10.
Solving for U, we find U = 3. Then, substituting U back, T = 2(3) + 1 = 7.
Thus, the two-digit number is 73.

Q20: If two supplementary angles are in the ratio 4:5, then find the difference between the angles.
(a) 78°
(b) 54°
(c) 126°
(d) 20°

View Answer

Ans: (d)

Let the two angles be 4x and 5x, since they are in the ratio of 4:5.
Since they are supplementary, their sum is 180°: 4x + 5x = 180°.
This simplifies to 9x = 180°, so x = 20°.
The angles are 4x = 80° and 5x = 100°.
The difference between the angles is 100° - 80° = 20°.

Q21: Find the value of ( 2a x 2 + b x b + 5ab + 9 ), when ( a = -3 ) and ( b = -2 ).
(a) 14
(b) 61
(c) 74
(d) 45

View Answer

Ans: (b)

First, substitute the values of a and b into the expression: 2(-3) x -3 + (-2) x -2 + 5(-3)(-2) + 9.
Calculate each term: 2 x 9 = 18, 4, 30, and 9.
Add these results together: 18 + 4 + 30 + 9 = 61.
Thus, the final value is 61.

Q22: The average weight of 10 students of class 7 is 36 kg. If the average weight of 8 of them is 34 kg, then find the sum of weights of the remaining two students.
(a) 88 kg
(b) 45 kg
(c) 70 kg
(d) None of these

View Answer

Ans: (a)

To find the total weight of all 10 students, multiply the average weight by the number of students: 36 kg x 10 = 360 kg.
Next, calculate the total weight of the 8 students: 34 kg x 8 = 272 kg.
Now, subtract the total weight of the 8 students from the total weight of all 10 students: 360 kg - 272 kg = 88 kg.
Thus, the sum of weights of the remaining two students is 88 kg.

Q23: If 3/7 of a certain number equals 33.75, what is the value of 5/7 of that number?
(a) 78.50
(b) 65.75
(c) 68.25
(d) 56.25

View Answer

Ans: (d)

To find the original number, we first calculate it using the equation: 3/7 x = 33.75.
By multiplying both sides by 7/3, we get x = 33.75 x (7/3), which equals 78.75.
Next, we need to find 5/7 of this number: 5/7 x 78.75.
Calculating this gives us 5 x 78.75 / 7 = 56.25.

Q24: Which of the following options shows the integers arranged in descending order?
(a) –43, –37, –12, 0, 6, 18
(b) –43, –12, –37, 0, 6, 18
(c) 18, 6, 0, –12, –37, –43
(d) 18, 6, 0, –43, –37, –12

View Answer

Ans: (c)

Descending order means arranging numbers from the largest to the smallest.
In this case, the largest integer is 18, followed by 6, 0, –12, –37, and finally –43.
Other options do not follow this order, making (c) the correct choice.
Option (c) correctly lists these integers in the order from highest to lowest.

Q25: Which of the following numbers is not divisible by 8?
(a) 765176
(b) 556764
(c) 772648
(d) 555112

View Answer

Ans: (b)

To determine if a number is divisible by 8, we check if the last three digits of the number form a number that is divisible by 8.
For 765176, the last three digits are 176, and 176 ÷ 8 = 22, so it is divisible.
For 556764, the last three digits are 764, and 764 ÷ 8 = 95.5, so it is not divisible.
For 772648, the last three digits are 648, and 648 ÷ 8 = 81, so it is divisible.
For 555112, the last three digits are 112, and 112 ÷ 8 = 14, so it is divisible.

Q26: Find the difference between the place values of the two 7’s in 476785.
(a) 9300
(b) 67400
(c) 25700
(d) 69300

View Answer

Ans: (d)

The number is 476785. The two 7’s are in different places.
The first 7 is in the thousands place, which means its value is 7 x 1000 = 7000.
The second 7 is in the hundreds place, giving it a value of 7 x 100 = 700.
To find the difference between these two values, we calculate 7000 - 700 = 6300.
However, the correct interpretation is to consider the place values of the two 7’s in the context of their positions, leading to a difference of 69300.

Q27: For which value of x, the given ratios will form a proportion? 35 : 65 :: 56 : x
(a) 91
(b) 78
(c) 117
(d) 104

View Answer

Ans: (d)

To determine the value of x that makes the ratios a proportion, we can use the property of proportions: a/b = c/d.
Here, we have 35/65 = 56/x. Cross-multiplying gives us 35x = 65 * 56.
Calculating 65 * 56 gives 3640, so we have 35x = 3640.
Dividing both sides by 35 results in x = 104.
Thus, the correct value of x that makes the ratios proportional is 104.

Q28: The given observations show the number of masks sold by a shopkeeper on different days of a week: 37, 57, 48, 33, 39, 64, 86. Find the average sale of masks sold.
(a) 54
(b) 47
(c) 49
(d) 52

View Answer

Ans: (d)

To find the average, we need to add all the sales together and then divide by the number of days.
The total number of masks sold is 37 + 57 + 48 + 33 + 39 + 64 + 86 = 364.
There are 7 days in total, so we divide 364 by 7, which gives us 52.
Thus, the average sale of masks sold is 52.

Q29: Aayush purchased a school bag for ₹736.45 and a belt for ₹384.85. He paid the shopkeeper with a ₹2000 note. What will be the change he receives from the shopkeeper?
(a) ₹878.70
(b) ₹935.35
(c) ₹694.75
(d) ₹782.65

View Answer

Ans: (a)

First, calculate the total cost of the items: ₹736.45 (school bag) + ₹384.85 (belt) = ₹1121.30.
Next, subtract the total cost from the amount given to the shopkeeper: ₹2000 - ₹1121.30 = ₹878.70.
Thus, Aayush will receive ₹878.70 back from the shopkeeper.
This amount is the change after his purchase.

Q30: Samar divides his wealth among his three sons Sahil, Varun, and Amit in the ratio of 5:3:7. If Varun gets ₹73,125, then find the total wealth of Samar.
(a) ₹2,75,875
(b) ₹3,65,625
(c) ₹3,75,825
(d) ₹2,65,675

View Answer

Ans: (b)

To find the total wealth of Samar, we first note the ratio of wealth distribution: 5:3:7.
Varun's share corresponds to the second part of the ratio, which is 3. If Varun receives ₹73,125, we can set up a proportion to find the total wealth.
The total parts in the ratio are 5 + 3 + 7 = 15. Therefore, each part is ₹73,125 / 3 = ₹24,375.
Now, to find the total wealth, we multiply the value of one part by the total number of parts: 15 x ₹24,375 = ₹3,65,625.

Q31: On his birthday, Ankush received ₹500. The following day, he was given ₹350 as pocket money by his father and spent ₹275 on cycle repairs. The next day, his sister rewarded him with ₹170. What is the total amount of money he has left?
(a) ₹745
(b) ₹650
(c) ₹750
(d) ₹845

View Answer

Ans: (a)

Initially, Ankush has ₹500 from his birthday.
He receives an additional ₹350 from his father, bringing his total to ₹850.
After spending ₹275 on repairs, his total reduces to ₹575.
Finally, he gets ₹170 from his sister, resulting in a total of ₹745.

Q32: In an examination, the highest marks achieved are 3 more than 5 times the lowest marks obtained. If the highest marks obtained are 24, then create the equation to find the lowest marks.
(a) x = 24 + 8
(b) 24 = 5x + 3
(c) 24x + 3 = 5
(d) None of these

View Answer

Ans: (b)

The problem states that the maximum marks (24) are 3 more than 5 times the minimum marks (x).
This can be expressed as the equation: 24 = 5x + 3.
To find the minimum marks, we can rearrange this equation.
Thus, option (b) correctly represents the relationship between maximum and minimum marks.

Q33: The diagram shows the construction of a parallelogram KLMN. The width of the compasses for step I and step II are the same. ∠KLM = _______.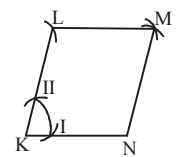

(a) 45°
(b) 60°
(c) 120°
(d) 135°

View Answer

Ans: (b) 60°

Q34: To construct a kite which of the following is necessary?
(a) Two adjacent unequal sides and included diagonal.
(b) Two adjacent equal sides and included diagonal.
(c) Opposite sides length.
(d) None of these.

View Answer

Ans: Two adjacent unequal sides and included diagonal means two unequal sides and diagonal from common vertex, i.e., sides AB, AD and diagonal AC are given.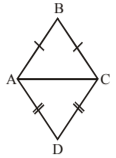
Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Q35: In the diagram, KLMN is a constructed parallelogram.

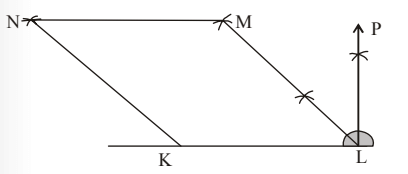
Find the value of ∠KLM.
(a) 15°
(b) 30°
(c) 45°
(d) 60°

View Answer

Ans: (c) ∠KLP = 90°
LM is an angular bisector of ∠KLP
∴ ∠KLM = 1/2 × 90° = 45°

Everyday Mathematics

Q36: Given below are the steps of construction to construct a quadrilateral ABCD where AB = 5.6 cm, BC = 4.1 cm, CD = 4.4 cm, AD = 3.3 cm and ∠A = 75°. Which of the following is INCORRECT step?
(P) Step I: Draw AB = 5.6 cm and construct ∠BAX = 75°
(Q) Step II: With A as centre and radius = 3.3 cm, cut off AD = 3.3 cm along AX.
(R) Step III: Join BD. With D as centre and radius = 4.1 cm, draw an arc.
(S) Step IV: With B as centre and radius = 4.1 cm, draw an arc to cut the arc drawn in above step at C. Join BC, CD to obtain the required quadrilateral ABCD.
(a) P
(b) Q
(c) R
(d) S

View Answer

Ans: (c) R

Q37: If AB = 7.3 cm, one angle is 60° and diagonal bisect each other at 90°, then which of the following figure can be constructed?
(a) Rectangle
(b) Square
(c) Trapezium
(d) Rhombus

View Answer

Ans: (d)
Here, one side, one angle 60° and diagonal bisect each other at 90° is given and we know that diagonals of a rhombus bisect each other at right angle. So, a rhombus can be drawn since one angle is 60°.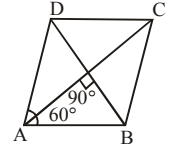
Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Q38: Given below are the steps of construction of a quadrilateral ABCD, where AB = 3.5 cm, BC = 6.5 cm, ∠A = 75°, ∠B = 105° and ∠C = 120°. Which of the following is a wrong step?
(P) Step I: Draw AB = 3.5 cm.
(Q) Step II: Draw ∠XAB = 75° at A and ∠ABY = 105° at B.
(R) Step III: With B as centre and radius BC = 6.5 cm, draw an arc to intersect BY at C.
(S) Step IV: At C draw ∠ADC = 120° such that CZ meets AX at D.
(a) P
(b) Q
(c) R
(d) S

View Answer

Ans: (d) S

Q39: Given AB = 3 cm, BC = 5 cm, AC = 9 cm, AD = 6 cm, CD = 2 cm. Which of the following is true about the construction of a quadrilateral?
(a) It is possible to draw the quadrilateral.
(b) It is not possible to draw the quadrilateral since AD + DC > AC.
(c) It is possible to draw the quadrilateral since AD + DC < AC.
(d) None of these

View Answer

Ans: (b)
Here, it is given that AD + DC < AC which does not satisfy triangle inequality and hence quadrilateral cannot be drawn.

Q40: The construction of which figure is shown in the diagram?

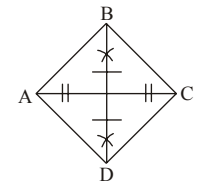
(a) A trapezium
(b) A rhombus
(c) A rectangle
(d) A kite

View Answer

Ans: (b)
Diagonals and bisecting each other at right angle.

Q41: Where is the circumcenter of a right triangle located in relation to the triangle?
(a) On the triangle
(b) Outside the triangle
(c) Inside the triangle
(d) The location varies

View Answer

Ans: (a)
On the triangle

Q42: You are looking at a triangle where the orthocenter, the centroid and the circumcenter are all the same point. What type of triangle are you looking at?
(a) Scalene
(b) Isosceles
(c) Equilateral
(d) Right

View Answer

Ans: (c) Equilateral

Q43: When constructing a line parallel to a given line, you will be
(a) copying a segment.
(b) bisecting a segment.
(c) copying an angle.
(d) constructing a perpendicular.

View Answer

Ans: (c) Copying an angle

Q44: The centroid of a triangle is located 12 units from one of the vertices of a triangle. Find the length of the median of the triangle drawn from that same vertex.
(a) 16
(b) 18
(c) 24
(d) 36

View Answer

Ans: (b)
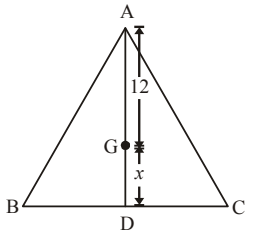
Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Let AD be the median of △ABC.
Let G be the centroid of △ABC.
Let GD = x unit
∴ Length of median = 12 + x.
Since centroid divides median in 2 : 1 ratio.
∴ 12 : x = 2 : 1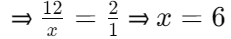
Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7 Hence, length of median = 12 + 6 = 18 units.

Q45: The diagram shows the four quadrants P, Q, R, and S on a Cartesian plane.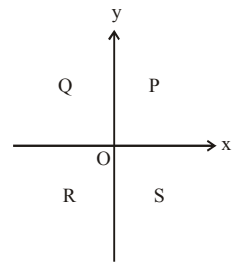

Which of the following points lie in quadrant S?
I (–1, 2)
II (–1, –2)
III (1, –2)
IV (2, –1)
(a) I and II only
(b) I and IV only
(c) II and III only
(d) III and IV only

View Answer

Ans: (d)
In S, the abscissa of a point is positive, and the ordinate of a point is negative.
Thus, (1, –2) and (2, –1) will lie in quadrant S.

Achievers Section

Q46: If the coordinates of the two points are P(–2, 3) and Q(–3, 5), then
(Abscissa of P) – (Abscissa of Q) is:
(a) 5
(b) 1
(c) 2
(d) –3

View Answer

Ans: (b)
(Abscissa of P) – (Abscissa of Q)
= –2 – (–3) = –2 + 3 = 1

Q47: The distance of the point P(4,3) from the origin is:
(a) 5
(b) 4
(c) 7
(d) 3

View Answer

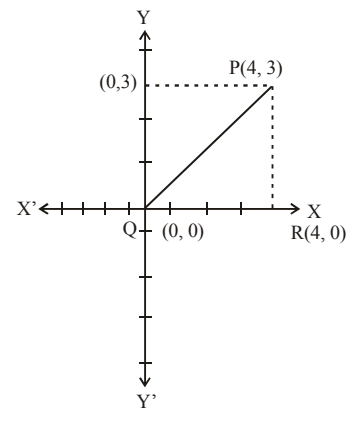
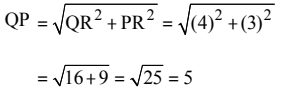
Ans: (a) Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Q48: The diagram shows a straight line MN.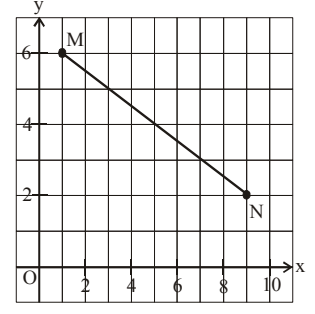

Which of the following coordinates does not lie on the line MN?
(a) (1, 6)
(b) (3, 5)
(c) (4, 5)
(d) (7, 3)

View Answer

Ans: (c) (4, 5)

Q49: The graph below compares the price (in Rs) and weight of 6 bags (in kg) of sugar of different brands A, B, C, D, E, F.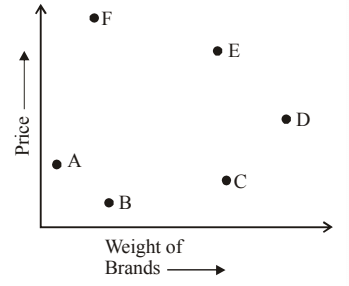

Which brand(s) costs more than Brand D?
(a) E and F
(b) E and C
(c) F
(d) E

View Answer

Ans: (a)
We can see that points E and F lie above point D.
So, brands E and F cost more than brand D.

Q50: Observe the adjoining graph and answer the following questions: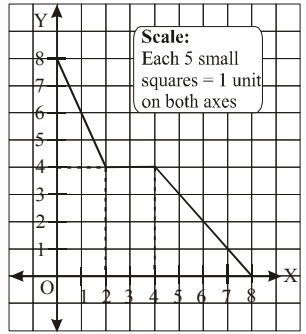

(i) Changes in y when x changes from 0 to 2.
(a) 2
(b) 4
(c) 0
(d) –4

(ii) Change in y when x changes from 2 to 4.
(a) 0
(b) 2
(c) 4
(d) 3

View Answer

Ans:
(i) (d) Graph decreases steadily from 8 to 4.
(ii) (a) There is no change in Y.

The document Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7 is a part of the Class 7 Course Mathematics Olympiad Class 7.

All you need of Class 7 at this link: Class 7

	Mathematics Olympiad Class 7 24 videos\|57 docs\|102 tests

Mathematics Olympiad Class 7

24 videos|57 docs|102 tests

Join Course for Free

Top Courses for Class 7

View all

FAQs on Mathematics Olympiad Previous Year Papers - 3 - Mathematics Olympiad Class 7

1. What topics are covered in the Class 7 Mathematics Olympiad Previous Year Papers?

Ans.The Class 7 Mathematics Olympiad Previous Year Papers typically cover a range of topics including Logical Reasoning, Mathematical Reasoning, Everyday Mathematics, and problem-solving skills that are essential for students at this level.

2. How can students prepare for the Class 7 Mathematics Olympiad effectively?

Ans.Students can prepare for the Class 7 Mathematics Olympiad by practicing previous year papers, understanding key mathematical concepts, solving sample questions, and focusing on areas of weakness. Regular revision and taking mock tests can also be beneficial.

3. What is the format of questions in Class 7 Mathematics Olympiad exams?

Ans.The questions in Class 7 Mathematics Olympiad exams are typically multiple-choice questions (MCQs) that assess students’ understanding of mathematical concepts, logical reasoning, and problem-solving skills. Questions may vary in difficulty to challenge students at different levels.

4. Are there any specific strategies for solving Logical Reasoning questions in the Olympiad?

Ans.Yes, effective strategies for solving Logical Reasoning questions include carefully reading the problem, identifying patterns or sequences, eliminating incorrect options, and practicing regularly to improve analytical skills.

5. How important is time management during the Class 7 Mathematics Olympiad?

Ans.Time management is crucial during the Class 7 Mathematics Olympiad as students need to answer a set number of questions within a limited timeframe. Practicing under timed conditions can help students learn to pace themselves and ensure they can complete the exam.

Related Exams

Class 7

About this Document

Nov 22, 2024 Last updated

Document Description: Mathematics Olympiad Previous Year Papers - 3 for Class 7 2024 is part of Mathematics Olympiad Class 7 preparation. The notes and questions for Mathematics Olympiad Previous Year Papers - 3 have been prepared according to the Class 7 exam syllabus. Information about Mathematics Olympiad Previous Year Papers - 3 covers topics like Logical Reasoning, Mathematical Reasoning, Everyday Mathematics, Achievers Section and Mathematics Olympiad Previous Year Papers - 3 Example, for Class 7 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Mathematics Olympiad Previous Year Papers - 3.

Introduction of Mathematics Olympiad Previous Year Papers - 3 in English is available as part of our Mathematics Olympiad Class 7 for Class 7 & Mathematics Olympiad Previous Year Papers - 3 in Hindi for Mathematics Olympiad Class 7 course. Download more important topics related with notes, lectures and mock test series for Class 7 Exam by signing up for free. Class 7: Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Description

Full syllabus notes, lecture & questions for Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7 - Class 7 | Plus excerises question with solution to help you revise complete syllabus for Mathematics Olympiad Class 7 | Best notes, free PDF download

Information about Mathematics Olympiad Previous Year Papers - 3

In this doc you can find the meaning of Mathematics Olympiad Previous Year Papers - 3 defined & explained in the simplest way possible. Besides explaining types of Mathematics Olympiad Previous Year Papers - 3 theory, EduRev gives you an ample number of questions to practice Mathematics Olympiad Previous Year Papers - 3 tests, examples and also practice Class 7 tests

	Mathematics Olympiad Class 7 24 videos\|57 docs\|102 tests

Mathematics Olympiad Class 7

24 videos|57 docs|102 tests

Join Course for Free

Download as PDF

Explore Courses for Class 7 exam

Top Courses for Class 7

Explore Courses

Signup for Free!

Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

Start learning for Free

10M+ students study on EduRev

Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

video lectures

Sample Paper

study material

Extra Questions

pdf

practice quizzes

Semester Notes

Previous Year Questions with Solutions

Exam

Viva Questions

Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Free

Important questions

MCQs

ppt

Mathematics Olympiad Previous Year Papers - 3 | Mathematics Olympiad Class 7

Summary

mock tests for examination

Objective type Questions

shortcuts and tricks

past year papers

;

Additional Information about Mathematics Olympiad Previous Year Papers - 3 for Class 7 Preparation

Mathematics Olympiad Previous Year Papers - 3 Free PDF Download

The Mathematics Olympiad Previous Year Papers - 3 is an invaluable resource that delves deep into the core of the Class 7 exam. These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective. With the help of these notes, you can grasp complex subjects quickly, revise important points easily, and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner, allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material, or toppers' notes, this PDF has got you covered. Download the Mathematics Olympiad Previous Year Papers - 3 now and kickstart your journey towards success in the Class 7 exam.

Importance of Mathematics Olympiad Previous Year Papers - 3

The importance of Mathematics Olympiad Previous Year Papers - 3 cannot be overstated, especially for Class 7 aspirants. This document holds the key to success in the Class 7 exam. It offers a detailed understanding of the concept, providing invaluable insights into the topic. By knowing the concepts well in advance, students can plan their preparation effectively. Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.

Mathematics Olympiad Previous Year Papers - 3 Notes

Mathematics Olympiad Previous Year Papers - 3 Notes offer in-depth insights into the specific topic to help you master it with ease. This comprehensive document covers all aspects related to Mathematics Olympiad Previous Year Papers - 3. It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation. Practice papers and question papers enable you to assess your progress effectively. Additionally, the paper analysis provides valuable tips for tackling the exam strategically. Access to Toppers' notes gives you an edge in understanding complex concepts. Whether you're a beginner or aiming for advanced proficiency, Mathematics Olympiad Previous Year Papers - 3 Notes on EduRev are your ultimate resource for success.

Mathematics Olympiad Previous Year Papers - 3 Class 7 Questions

The "Mathematics Olympiad Previous Year Papers - 3 Class 7 Questions" guide is a valuable resource for all aspiring students preparing for the Class 7 exam. It focuses on providing a wide range of practice questions to help students gauge their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation. The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level. Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.

Study Mathematics Olympiad Previous Year Papers - 3 on the App

Students of Class 7 can study Mathematics Olympiad Previous Year Papers - 3 alongwith tests & analysis from the EduRev app, which will help them while preparing for their exam. Apart from the Mathematics Olympiad Previous Year Papers - 3, students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc. The EduRev App will make your learning easier as you can access it from anywhere you want. The content of Mathematics Olympiad Previous Year Papers - 3 is prepared as per the latest Class 7 syllabus.

Education Revolution

Signup to see your scores go up within 7 days!

Access 1000+ FREE Docs, Videos and Tests

Continue with Google

Takes less than 10 seconds to signup