Lines and Angles Class 7 Notes Maths Chapter 6
| Table of contents |

|
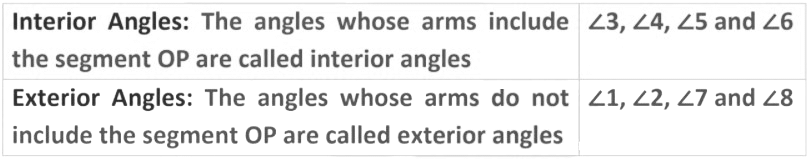
| Important Terms Related to Lines and Angles |

|
| What is an Angle? |

|
| Types of Angles |

|
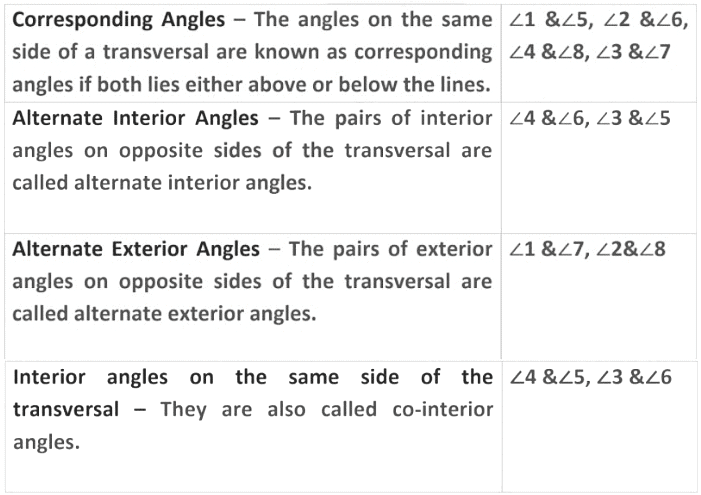
| Related Angles |

|
| Pairs of Lines |

|
| Checking for Parallel Lines |

|
Take a moment to look around the room you're in. What do you notice? Maybe a table, a chair, a wall clock, a laptop, or even a staircase. Now, glance outside—there are trees, roads, buildings, and electric poles. Did you know that all these things are made up of simple shapes like lines and angles? Let's dive into the world of lines and angles to understand how they form the very objects we see every day.
Let's dive into the world of lines and angles to understand how they form the very objects we see every day.
Important Terms Related to Lines and Angles
Some important terms related to lines and angles are:
- Point: An exact location that has no size, i.e., no length, no width, no depth, the only position is called a point. A point is denoted by a dot (.) If you take an ink pen and put a dot on paper using that, then that dot represents a point.
- Line segment: A collection of points with two fixed endpoints is called a line segment. A line segment AB is denoted by
 . The length of a line segment is fixed.
. The length of a line segment is fixed.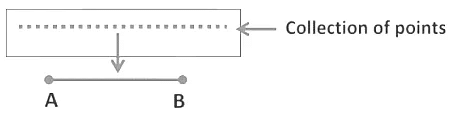
- Ray: A part of a line with one fixed point and extends endlessly from the other end is called a ray. Ray AB is denoted by
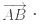 The length of a ray is infinite.
The length of a ray is infinite. Ray
Ray - Line: A line is a collection of points going endlessly in both directions along a straight path. Line AB is denoted by
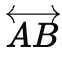 . The length of a line is infinite.
. The length of a line is infinite. Straight Line
Straight Line
What is an Angle?
When two rays originate from a common point, then the turn between two rays around the common point or vertex is called the angle between the two rays.
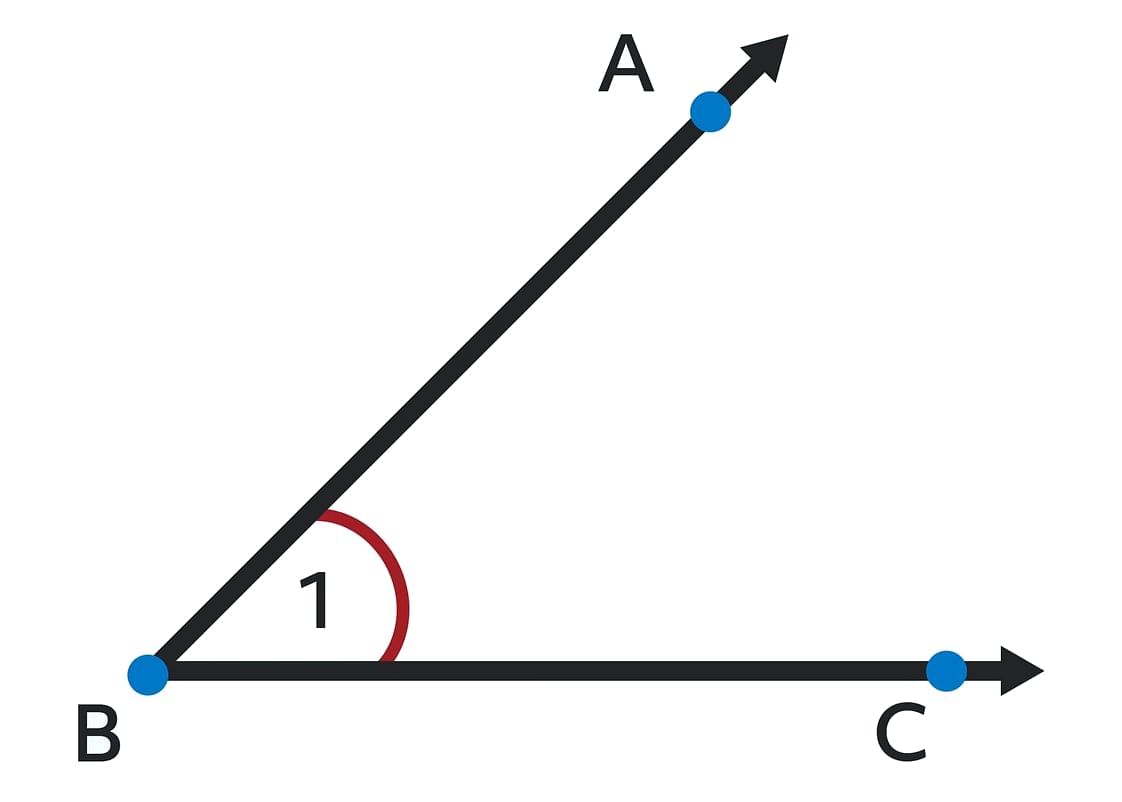 Formation of Angle
Formation of Angle
- The two rays joining to form an angle are called arms of an angle and the point at which two rays meet to form an angle is called the vertex of the angle.
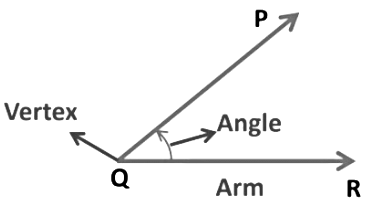
In the above figure, two rays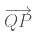 and
and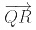 are the arm of an angle that meet at a common initial point Q (vertex)and form a ∠PQR. The measure of angle PQR is written as ∠PQR.
are the arm of an angle that meet at a common initial point Q (vertex)and form a ∠PQR. The measure of angle PQR is written as ∠PQR.
Types of Angles
Understanding the different types of angles is crucial in geometry, as they are fundamental to many geometric concepts and real-world applications.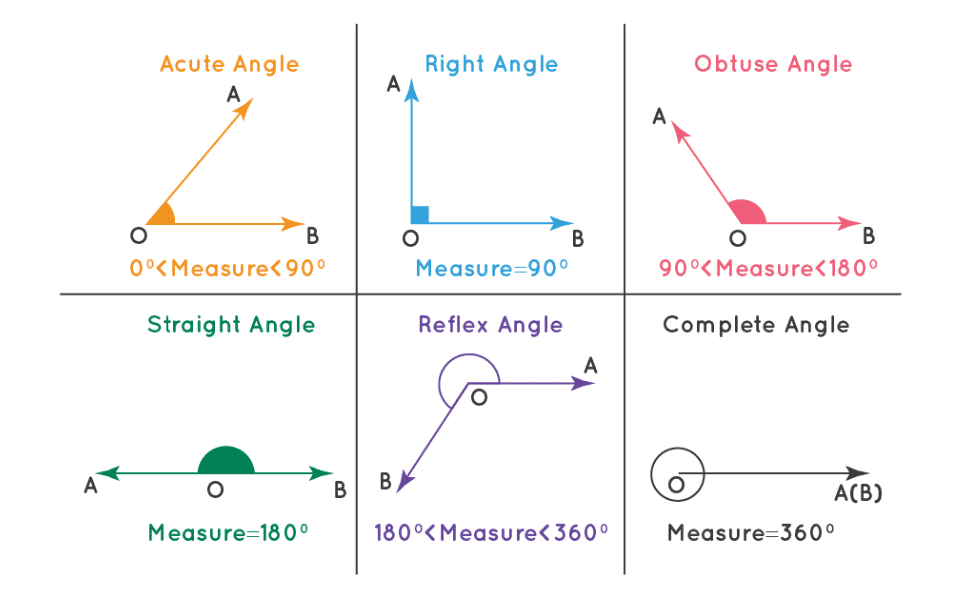 Types of Angles
Types of Angles
Related Angles
1. Complementary Angles
- When the sum of the measures of two angles is 90°, the angles are called complementary angles.
- Whenever two angles are complementary, each angle is said to be the complement of the other angle.
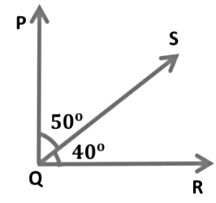 Complementary Angles
Complementary Angles
Here,
∠PQS + ∠SQR= 50° + 40° = 90°
In the above figure, we see that the sum of the two angles is 90°.
Hence, ∠PQS and ∠SQR are complementary angles. And ∠PQS and ∠SQR are said to be complements of each other.
Example: Are the given angles complementary?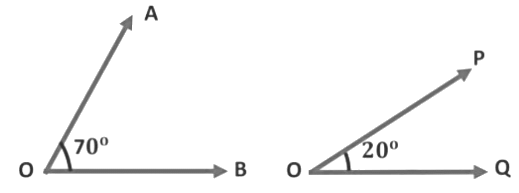 In the given figure,
In the given figure,
∠AOB = 70°and ∠POQ = 20°
∠AOB + ∠POQ = 70° + 20° = 90°
Therefore, ∠AOB and ∠POQ are complementary angles.
2. Supplementary Angles
- Two angles are said to be supplementary if the sum of their measure is equal to 180°.
- When two angles are supplementary, each angle is said to be the supplement of the other
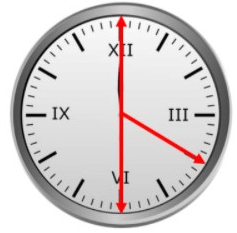 Clock
Clock
Example: Clock: The two angles formed by the hands of the above clock are supplementary.
- The measure of two angles 120° and 60° are given and when we add up that angles we get 180°.
120° + 60° = 180°. - Hence, we can say that they are supplementary angles or supplements of each other.
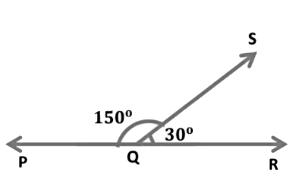 Supplementary Angle
Supplementary Angle
Here,
∠PQS + ∠SQR = 150° + 30° = 180°
In the above figure, we see that the sum of the two angles is 180°.
Hence, ∠PQS and ∠SQR are supplementary angles, and ∠PQS and ∠SQR are said to be supplements of each other.
Example: Are the following ∠AOB and ∠POQ supplementary angles or not?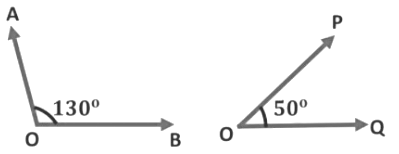 Supplementary Angle
Supplementary Angle
Sol: In the given figure,
∠AOB + ∠POQ = 130° + 50° = 180°
∠AOB + ∠POQ = 180°
∴ ∠AOB and ∠POQ are supplementary angles. Or
∠AOB and ∠POQ are said to be supplements of each other.
Pairs of Lines
1. Intersecting Lines
Two lines are said to be intersecting when they cross each other at one point only and the point at which they intersect is called the point of intersection.
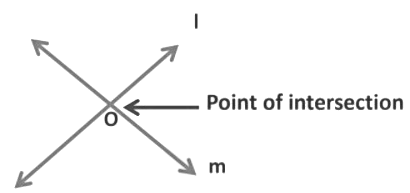 Here, two lines l and m intersect each other at point O, and point O is called the point of intersection.
Here, two lines l and m intersect each other at point O, and point O is called the point of intersection.
2. Transversal Line
A line that intersects two or more lines in a plane at distinct points is called a transversal line.
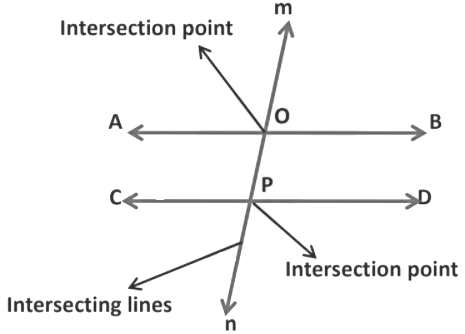
- Here, line mn intersects two lines AB and CD at two distinct points, O and P, respectively.
- Hence, line mn is called the transversal line, and points O and P are called the points of intersection.
3. Angles made by a Transversal
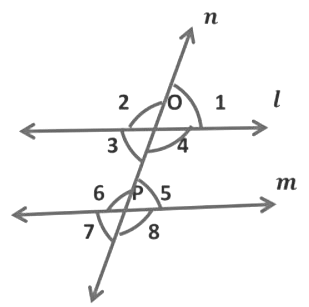
Here, the two lines l and m are intersected by a transversal n at points O and ,P respectively. We see that four angles are formed at each point O and P, namely ∠1, ∠2, ∠3, ∠4, ∠5, ∠6, ∠7, and ∠8.
Example: In the figure given below, l || m and ∠1 = 53°. Find ∠6 and ∠7.
We have,
∠1 = ∠3 [Vertically opposite angles]
∠3 = ∠7 [Corresponding angles]
∴ ∠1 = ∠7
→ ∠7 = 53° ∵ ∠1 = 53° (Given)
∠6 + ∠7 = 180° [Linear pair]
∠6 + 53° = 180°
∠6 + 53° − 53° = 180° − 53°
∠6 = 127°
Thus, ∠6 = 127° and ∠7 = 53°
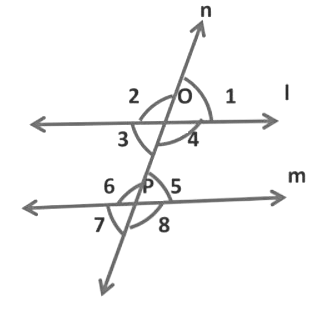
4. Transversal of Parallel Lines
If two lines lying in the same plane do not intersect when produced on either side, then such lines are said to be parallel to each other.

Here, lines l and m are parallel to each other, and transversal n intersects line l and m at points O and ,P respectively.
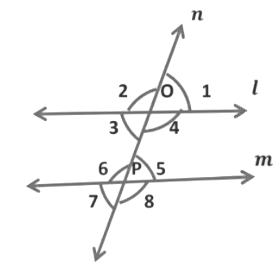
When the two parallel lines l and m are cut by a transversal n, then the following relations are obtained:
(i) When a transversal intersects two parallel lines, then each pair of alternate interior angles is equal.
When line n intersects two parallel lines l and m, then we see that each pair of alternate interior angles is equal.
∴ ∠ 3 = ∠5, ∠4 = ∠6
(ii) When a transversal intersects two parallel lines, each pair of alternate exterior angles is equal.
When line n intersects two parallel lines l and m, then we see that each pair of alternate exterior angles is equal.
∴ ∠ 2 = ∠8, ∠1 = ∠7
(iii) When a transversal intersects two parallel lines, each pair of corresponding angles is equal.
When line n intersects two parallel lines l and m, then we see that each pair of corresponding angles is equal.
∴ ∠3 = ∠7, ∠2 = ∠6, ∠ 1 = ∠5, and ∠4 = ∠8
(iv) When a transversal intersects two parallel lines, then each pair of interior angles on the same side of the transversal is supplementary
When line n intersects two parallel lines l and m, then we see that each pair of interior angles on the same side of the transversal is supplementary.
In the above figure, ∠ 3 = ∠4 .....Linear pair of angles
We know the sum of the linear pair of angles is 180° ∴ ∠ 3 + ∠4 = 180°
But, ∠4 = ∠6 ...Pair of alternate interior angles. Therefore, we can say that ∴ ∠ 3 + ∠6 = 180°
Similarly, ∠4 + ∠5 = 180°
Example: In the given figure l || m, ∠1 = 55°. Find ∠5, ∠6, and ∠7.
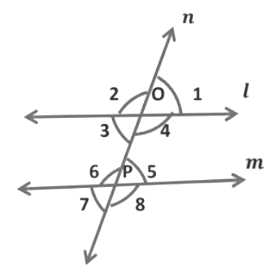
We have,
∠ 1 = ∠5 .....Corresponding angles
∴ ∠ 5 = 55° [∵ ∠1 = 55°]
∠5 = ∠7 .....Vertically opposite angles
∴ ∠7 = 55° [∵ ∠5 = 55°]
Now,
∠ 6 + ∠7 = 180°.....Linear pair of angles
∠ 6 + 55° = 180°
∠ 6 + 55° − 55° = 180° − 55°
∠ 6 = 180° − 55°
∠ 6 = 125°
Thus, ∠ 5 = 55°, ∠ 6 = 125°and ∠7 = 55°
Checking for Parallel Lines
Some special pairs of angles can be used to test if the lines are parallel or not.
(i) When a transversal intersects two parallel lines, such that if any pair of corresponding angles are equal, then the lines are parallel.
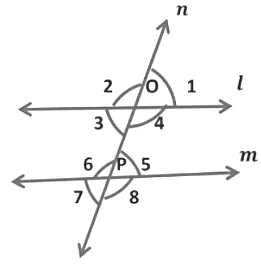
In the given figure, transversal n intersects two lines l and m in such a way that,
∠3 = ∠7, ∠2 = ∠6, ∠ 1 = ∠5, and ∠4 = ∠8 ...(Pairs of corresponding angles are equal)
Hence, we can say that lines are parallel.
(ii) When a transversal intersects two parallel lines, such that if any pair of alternate interior angles are equal, the lines have to be parallel.
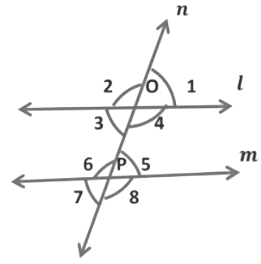
In the given figure, transversal n intersects two lines l and m in such a way that,
∠ 3 = ∠5, ∠4 = ∠6 ... (Alternate interior angles are equal)
Hence, we can say that lines are parallel.
(iii) When transversal intersects two parallel lines, such that if any pair of alternate exterior angles are equal, the lines have to be parallel.
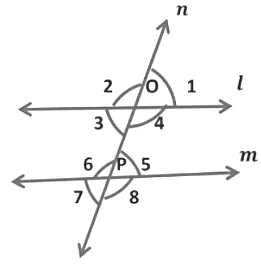
In the given figure, transversal n intersects two lines l and m in such a way that,
∠1 = ∠7, ∠2 = ∠8 ... (Alternate exterior angles are equal)
Hence, we can say that lines are parallel.
(v) When transversal intersects two parallel lines, such that if any pair of interior angles on the same side of the transversal are supplementary, the lines have to be parallel.
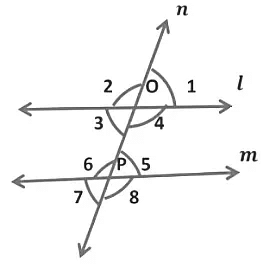
In the given figure, transversal n intersects two lines l and m in such a way that ∠ 3, ∠6, and ∠4, ∠5 ...Pairs of co-interior angles or angles on the same sides of the transversal
Hence,
∠ 3 + ∠6 = 180° and
∠4 + ∠5 = 180°
Hence, we can say that lines are parallel.
Example: Find whether AB || CD.
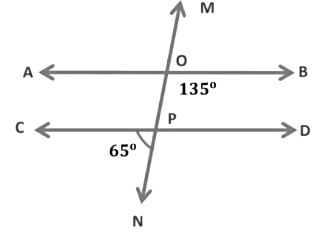
In the given figure,
∠CPN = ∠OPD = 65°...Vertically opposite angles
For AB || CD: ∠BOP + ∠OPD = 180°
The sum of the co-interior angles is 180°
So,
∠BOP+ ∠OPD
135° + 65°=200°
Since the sum of the co-interior angles is not equal to 180°
Therefore, AB is not parallel to CD.
Hope you have understood the topic well. Understand in detail the entire chapter through this video:
|
77 videos|386 docs|39 tests
|
FAQs on Lines and Angles Class 7 Notes Maths Chapter 6
| 1. What is an angle and how is it measured? |  |
| 2. What are related angles and what types are there? |  |
| 3. What are pairs of lines, and how can they be classified? |  |
| 4. How can you check if two lines are parallel? |  |
| 5. What role do transversal lines play in understanding angles? |  |
















