Decimal Fractions: Notes and Important Formulas | Quantitative Aptitude for SSC CGL PDF Download

A decimal is a number that includes a whole part and a fractional part, separated by a decimal point. It is based on the base-10 system, where each place value represents a power of 10.

A decimal fraction is a type of decimal where the fraction’s denominator is a power of 10 (such as 10, 100, or 1000) but is written using a decimal point instead of a fraction bar.

For example:

• 0.5 (which is the same as 5/10)

• 3.75 (which is the same as 375/100)

Decimals and decimal fractions are widely used in measurements, money, and everyday calculations.

Let's explore decimal fractions in detail.

What Are Decimal Fractions?

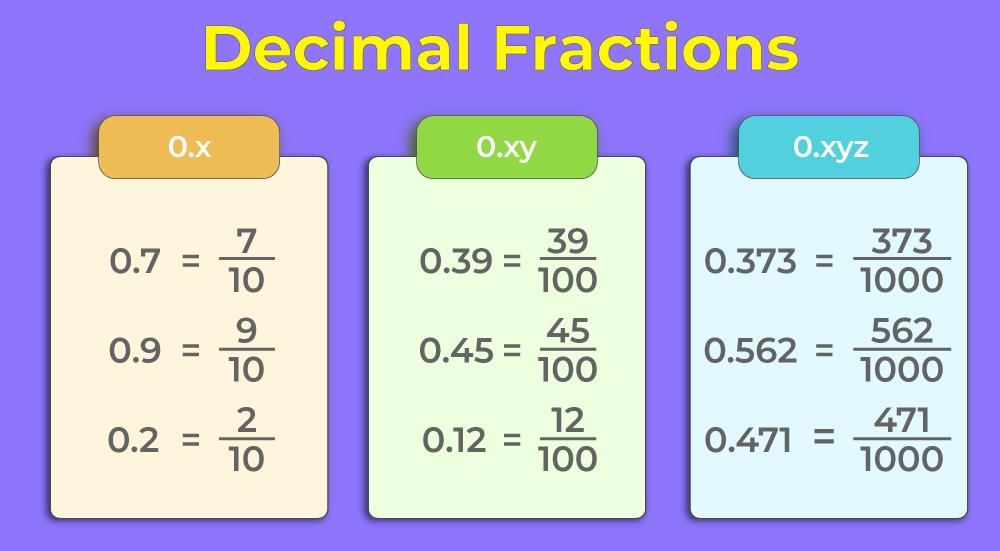
A decimal fraction is a fraction expressed in the base-10 number system, where the denominator is a power of 10, such as 10, 100, 1000, etc.

They are written using a decimal point, which separates the whole number part from the fractional part.

Examples

• 0.1 = 1/10 (one-tenth)

• 0.25 = 25/100 (twenty-five hundredths)

• 0.008 = 8/1000 (eight thousandths)

• 1.75 = 1 + 75/100 (mixed number)

EduRev Tip: Always be ready to convert between decimals and fractions—this is frequently tested in Exam.

Clear all your SSC CGL doubts with EduRev Join for Free

Join for Free

Place Value in Decimal Fractions

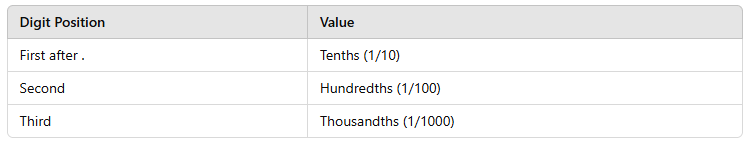
Each digit to the right of the decimal point has a place value:

Example
0.473

• 4 = tenths
• 7 = hundredths
• 3 = thousandths

Converting Decimals to Vulgar Fractions

To convert a decimal into a vulgar fraction:

1. Put 1 in the denominator under the decimal point and add zeros equal to the number of decimal places.
   
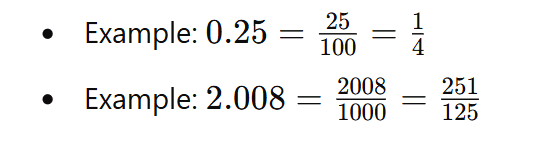
2. Annexing Zeros and Removing Decimal Signs:
   • Annexing zeros to the right of a decimal fraction doesn't change its value. For example, $0.8=0.80=0.8000.8\; =\; 0.80\; =\; 0.800$0.8 = 0.80 =0.800.
   • When the numerator and denominator have the same number of decimal places, the decimal point can be removed.
     

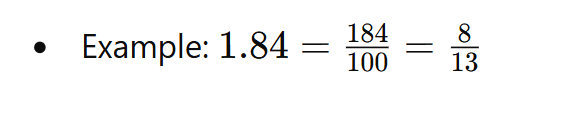
Download the notes Decimal Fractions: Notes and Important Formulas

Download as PDF

Download as PDF

Operations on Decimal Fractions

Addition and Subtraction: Align decimals and perform as usual.

• Example: $5.9632+0.073=6.03625.9632\; +\; 0.073\; =\; 6.0362$5.9632+0.073=6.0362
  

Multiplication: Multiply without the decimal point, then place the decimal in the product according to the total decimal places.

• Example: $0.2\times 0.02\times 0.002=0.0000080.2\; \backslash times\; 0.02\; \backslash times\; 0.002\; =\; 0.000008$0.2×0.02×0.002=0.000008

Division: Divide as normal and place the decimal in the quotient corresponding to the decimal places in the dividend.

• Example: $0.0204÷17=0.00120.0204\; \backslash div\; 17\; =\; 0.0012$0.0204÷17=0.0012

Recurring Decimals: If figures or sets of figures repeat indefinitely, it's a recurring decimal.

• Example: $\mathrm{0.333...}=0.3‾0.333...\; =\; 0.\backslash overline\{3\}$0.333...=0.3, $\mathrm{3.142857142857...}=3.1428573.142857142857...\; =\; 3.142857$3.142857142857...=3.142857

Pure Recurring Decimal: All figures after the decimal point repeat.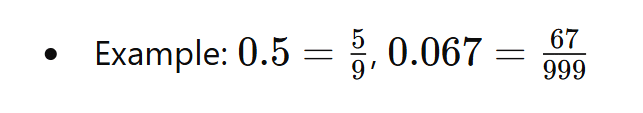
Mixed Recurring Decimal: Some figures repeat while others don't.

• Example: $\mathrm{0.173333...}=0.1730.173333...\; =\; 0.173$0.173333...=0.173

Decimal fractions are essential in mathematics for precise representation of values less than one. They're used in everyday calculations, finance, science, and more. Understanding their conversion, operations, and recurring nature is crucial for mastering mathematical concepts and applications.

Take a Practice Test Test yourself on topics from SSC CGL exam

Practice Now

Practice Now

Practice Questions on Decimal Fractions

Question 1: What is the product of 0.003 and 0.4?
Answer: 0.003 × 0.4 = 0.0012

• Multiply as if there were no decimals:
  3 × 4 = 12.

• Count the decimal places:

  • 0.003 has 3 decimal places.

  • 0.4 has 1 decimal place. Total decimal places = 3 + 1 = 4.

• Place the decimal in the product:
  12 → 0.0012.

Question 2: Convert 0.173333... to a fraction.
Answer: 0.173333... = 13/75

1. Let x = 0.173333...

2. Multiply both sides by 10:
   10x = 1.73333...

3. Subtract the original equation from the new equation:
   10x - x = 1.73333... - 0.17333...
   9x = 1.56
   ⇒ x = 1.56/9 = 156/900 = 13/75.

Question 3: What is the product of 0.003 and 0.4?
Answer: 0.003 × 0.4 = 0.0012

• Multiply as if there were no decimals:
  3 × 4 = 12.

• Count the decimal places:

  • 0.003 has 3 decimal places.

  • 0.4 has 1 decimal place. Total decimal places = 3 + 1 = 4.

• Place the decimal in the product:
  12 → 0.0012.

Question 4: What is 3.257 + 4.836?

Align the decimals and add:

Answer: 8.093

Question 5:
What is 9.462 - 3.218?

​Align the decimals and subtract:

Answer: 6.244