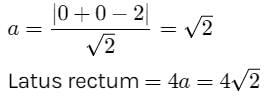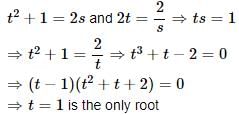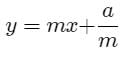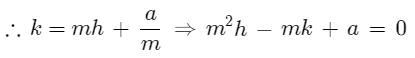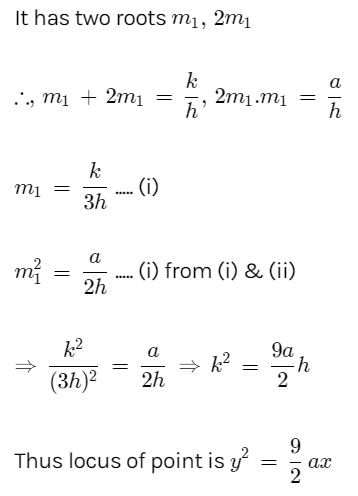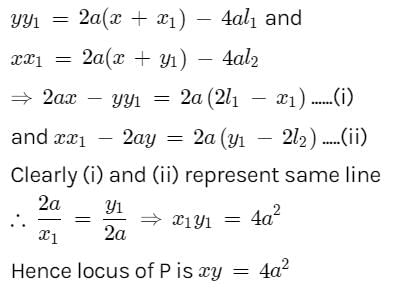Explore Exams
Login Signup
Sign in Open App
JEE Exam  >  JEE Test  >  Mathematics (Maths) Main & Advanced  >  Test: Parabola - 2 - JEE MCQ

Parabola - 2 - Free MCQ Practice Test with solutions, JEE Maths


MCQ Practice Test & Solutions: Test: Parabola - 2 (20 Questions)

You can prepare effectively for JEE Mathematics (Maths) for JEE Main & Advanced with this dedicated MCQ Practice Test (available with solutions) on the important topic of "Test: Parabola - 2". These 20 questions have been designed by the experts with the latest curriculum of JEE 2026, to help you master the concept.

Test Highlights:

  • - Format: Multiple Choice Questions (MCQ)
  • - Duration: 40 minutes
  • - Number of Questions: 20

Sign up on EduRev for free to attempt this test and track your preparation progress.

Test: Parabola - 2 - Question 1
Save

If the line x + y – 1 = 0 touches the parabola y2 = kx , then the value of k is

Detailed Solution: Question 1

If y = -x + 1 is tangent to y2 = kx, then
c = a / m

c = (k / 4) / (-1)

1 = (k / 4) / (-1)
k = -4

Test: Parabola - 2 - Question 2
Save

Directrix of a parabola is x + y = 2. If it's focus is origin, then latus rectum of the parabola is equal to

Detailed Solution: Question 2

 Distance from focus (0,0) to directrix x + y = 2 is |0 + 0 – 2|/√2 = 2/√2 = √2.
Thus 2a = √2,
so a = √2/2.
Latus rectum = 4a = 4 · (√2/2) = 2√2.

Clear all your doubts with EduRev
Test: Parabola - 2 - Question 3
Save

Which one of the following equations represents parametrically, parabolic profile ?

Detailed Solution: Question 3

x2 − 2 = −2cost
⇒ x2 = 2 − 2cost
⇒x2 = 2(1−cost)
⇒x2 = 2(1−(1−2sin2 t/2))
⇒x2 = 4sin2 t/2
We have y = 4cos2 t/2
⇒cos2 t/2= y/4
We know the identity, sin2 t/2 + cos2 t/2 = 1
⇒ x2/4 + y/4 = 1
⇒ x2 = 4−y represents a parabolic profile.

Test: Parabola - 2 - Question 4
Save

If (t2, 2t) is one end of a focal chord of the parabola y2 = 4x then the length of the focal chord will be

Detailed Solution: Question 4

Given (t2 , 2t) be one end of focal chord then other end be (1/t2 , −2t )

Length of focal chord = [(t2 - 1/t2)2 + (2t + t/2)2]½

= ( t + 1/t)2

Test: Parabola - 2 - Question 5
Save

From the focus of the parabola y2 = 8x as centre, a circle is described so that a common chord of the curves is equidistant from the vertex and focus of the parabola. The equation of the circle is

Detailed Solution: Question 5

Focus of parabola y2 = 8x is (2,0). Equation of circle with centre (2,0) is (x−2)2 + y2 = r2
Let AB is common chord and Q is mid point i.e. (1,0)
AQ2 = y2 = 8x
= 8×1 = 8
∴ r2 = AQ2 + QS2
= 8 + 1 = 9
So required circle is (x−2)2 + y2 = 9

Test: Parabola - 2 - Question 6
Save

The point of intersection of the curves whose parametric equations are x = t2 + 1, y = 2t and x = 2s, y = 2/s is given by

Detailed Solution: Question 6

For intersection of both the curve we must have,

Therefore, the point of intersection is (2,2)(2,2)
Hence, option 'B' is correct.

Test: Parabola - 2 - Question 7
Save

PN is an ordinate of the parabola y2 = 4ax. A straight line is drawn parallel to the axis to bisect NP and meets the curve in Q. NQ meets the tangent at the vertex in a point T such that AT = kNP, then the value of k is (where A is the vertex)

Detailed Solution: Question 7

The equation of parabola be y2=4ax
let the point P be (at2,2at)
PN is ordinate ⇒N(at2,0)
Equation of straight line bisecting NP is
y=at
substituting y in equation of parabola
a2t2 = 4ax
⇒x = 4at2
So the coordinates of Q are (4at2 ,at)
Equation of NQ is y−0 = (at−0)/(at2/4 - at2)(x−at2)
y= −4/3t(x−at2)
Put x=0
y = −4/3t(0−at^2)
⇒y=4at/3
⇒AT = 4at/3​
NP = 2at
AT/NP = (4at/3)/2at
= ⅔
AT = 2/3NP

Test: Parabola - 2 - Question 8
Save

The tangents to the parabola x = y2 + c from origin are perpendicular then c is equal to

Detailed Solution: Question 8

Test: Parabola - 2 - Question 9
Save

The locus of a point such that two tangents drawn from it to the parabola y2 = 4ax are such that the slope of one is double the other is

Detailed Solution: Question 9

Let the point be (h, k)

Now equation of tangent to the parabola y2 = 4ax whose slope is m is

as it passes through (h, k)]

Test: Parabola - 2 - Question 10
Save

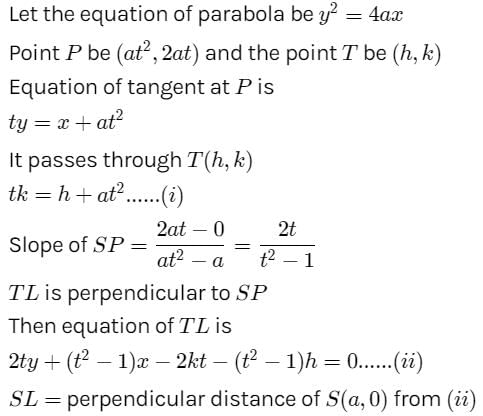
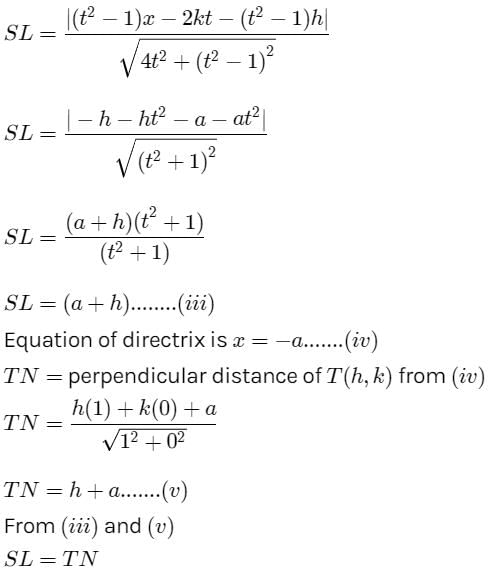
T is a point on the tangent to a parabola y2 = 4ax at its point P. TL and TN are the perpendiculars on the focal radius SP and the directrix of the parabola respectively. Then

Detailed Solution: Question 10


Test: Parabola - 2 - Question 11
Save

The equation of the circle drawn with the focus of the parabola (x – 1)2 – 8y = 0 as its centre and touching the parabola at its vertex is

Test: Parabola - 2 - Question 12
Save

The equation of the tangent at the vertex of the parabola x2 + 4x + 2y = 0 is

Detailed Solution: Question 12

The Equation of tangent at vertex to parabola x2+4x+2y=0 is :
x2+4x+2y+4−4=0
(x+2)2 = −2(y−2)
x2=−2y
Equation of tangent at vertex is y=0
y−2=0
y=2

Test: Parabola - 2 - Question 13
Save

Locus of the point of intersection of the perpendicular tangents of the curve y2 + 4y – 6x – 2 = 0 is

Detailed Solution: Question 13

Given parabola is, y2+4y−6x−2=0
⇒ y2+4y+4=6x+6=6(x+1)
⇒ (y+2)2 = 6(x+1)
shifting origin to (−1,−2)
Y2 = 4aX  where a = 3/2
We know locus of point of intersection of perpendicular tangent is directrix of the parabola itself
Hence required locus is X=−a ⇒ x+1=−3/2
⇒ 2x+5=0

Test: Parabola - 2 - Question 14
Save

Tangents are drawn from the points on the line x – y + 3 = 0 to parabola y2 = 8x. Then the variable chords of contact pass through a fixed point whose coordinates are

Detailed Solution: Question 14

Let (k,k+3) be the point on the line x−y+3=0
Equation of chord of contact is S1=0
⇒yy1=4(x+x1)
⇒y(k+3)=4(x+k)
⇒4x−3y−k(y−4)=0
Therefore, straight line passes through fixed point (3,4)

Test: Parabola - 2 - Question 15
Save

The line 4x – 7y + 10 = 0 intersects the parabola, y2 = 4x at the points A & B. The co-ordinates of the point of intersection of the tangents drawn at the points A & B are

Detailed Solution: Question 15

ky=[4(x+h)]/2
=> 2ky=2(x+h)
2ky=4x+4h  =>4x−2ky+4h=0
4x−7y+10=0
4h=10  => h=5/2
2k=7 => k=7/2
point of intersection of tan⁡gent at p and q is (5/2,7/2)

Test: Parabola - 2 - Question 16
Save

If (3t12-6t1) represents the feet of the normals to the parabola y2 = 12x from (1, 2), then Σ1/t1 is

Test: Parabola - 2 - Question 17
Save

TP & TQ are tangents to the parabola, y2 = 4ax at P & Q. If the chord PQ passes through the fixed point (–a, b) then the locus of T is

Test: Parabola - 2 - Question 18
Save

If the tangent at the point P (x1, y1) to the parabola y2 = 4ax meets the parabola y2 = 4a (x + b) at Q & R, then the mid point of QR is

Test: Parabola - 2 - Question 19
Save

Let PSQ be the focal chord of the parabola, y2 = 8x. If the length of SP = 6 then, l(SQ) is equal to(where S is the focus)

Detailed Solution: Question 19

Since the semi latus rectum of a parabola is the harmonic mean between the segment of any focal chord of a parabola, therefore,SP,4,SQ are in H.P.
⇒4=2(SP.SQ)/(SP+SQ)
⇒4=2*6.SQ/(6+SQ)
⇒SQ=3

Test: Parabola - 2 - Question 20
Save

Two parabolas y2 = 4a(x – l1) and x2 = 4a(y – l2) always touch one another, the quantities l1 and l2 are both variable. Locus of their point of contact has the equation

Detailed Solution: Question 20

 Let P(x1 , y1) be point of contact of two parabola. tangents at P of the two parabolas are

173 videos|510 docs|154 tests
Join Course
Information about Test: Parabola - 2 Page
In this test you can find the Exam questions for Test: Parabola - 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Parabola - 2, EduRev gives you an ample number of Online tests for practice
Download as PDF
;

About this JEE Practice Test

This test contains 20 MCQs on Parabola - 2 with detailed solutions for each question. It is part of the Mathematics (Maths) for JEE Main & Advanced course on EduRev, designed to align with the current JEE syllabus. Each solution explains not just the correct answer but also gives you an understanding of the topic — not just to attempt the question but also help you prepare for the exam with practice.

Explore more JEE Study Resources

If you found this Parabola - 2 MCQ Test helpful, you can further enhance your JEE preparation with in-depth courses on EduRev, for all the subjects and topics available in Mathematics (Maths) for JEE Main & Advanced course. The course offers:
  • Video Lectures: covering all the topics of Mathematics (Maths) for JEE Main & Advanced
  • Notes for Revision: offering revision notes, important questions, summaries, flashcards, cheatsheets & more for JEE preparation
  • MCQ Test: variety of questions as per the pattern of JEE exam
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup