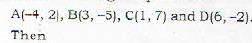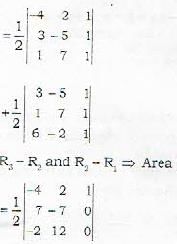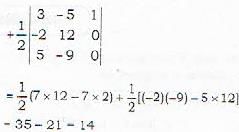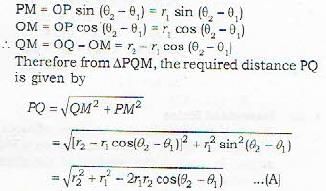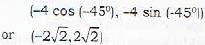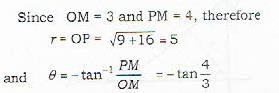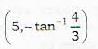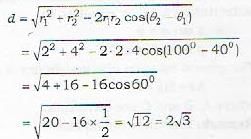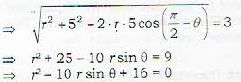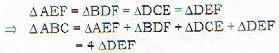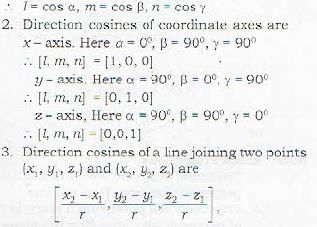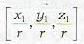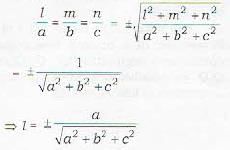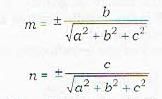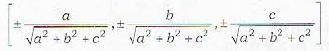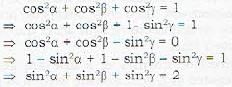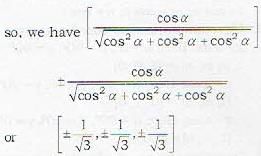Test: Dimensional Geometry - 2 - Mathematics MCQ
20 Questions MCQ Test - Test: Dimensional Geometry - 2
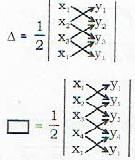
The area of a quadrilateral, whose vertices are given by (x1, y1), (x2, y2), (x3, y3) and (x4, y4) respectively, is
The area of the quadrilateral ABCD with coordinates of A, B, C and D as (-4, 2), (3, -5), (1, 7) and (6, -2) respectively is
The system of coordinate known as the Cartesian system of coordinates was first introduced by
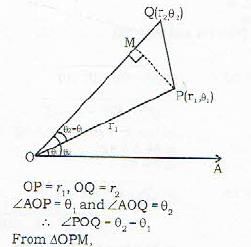
The distance between two points, whose coordinates are given by (r1, θ1) and (r2, θ2) respectively, is given by
The polar equation of a circle with radius c and centre (a, α) is given by
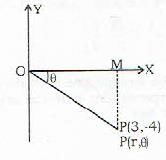
The rectangular coordinates of the point (-4, -π/4) are
The distance between the points whose poar coordinates are (2,40°) & (4,100°) respectively, is
The locus of a point whose distance is 3 from the point (5,π/2) is given by
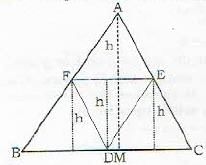
Let ABC be any triangle. Let D, E, F be the mid points of the sides BC,CA,AB respectively, Then
In a tetrahedron, each of the four vertices is the intersection of
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?
If α, β, γ are the angles that a line makes with the positive direction of the coordinate axis, then the direction cosines of the line are
If l,m,n are the direction cosines of a line, then
If the direction cosines l, m, n of a line are proportional to a. b, c, then
Let α, β, γ be the angles that a line makes with x-axis and y-axis, z-axis respectively. Which of the following relations does not hold
The direction cosine of a line that makes equal angles with the axes are given by
The projection of line segment joining (0,0,0) and (1,1,1) on the line 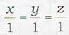 will be
will be