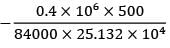All Courses
Get the App Signup / Login
Sign in Open App
Mechanical Engineering Exam  >  Mechanical Engineering Tests  >  Test: Torsion Level - 3 - Mechanical Engineering MCQ

Test: Torsion Level - 3 - Mechanical Engineering MCQ


Test Description

10 Questions MCQ Test - Test: Torsion Level - 3

Test: Torsion Level - 3 for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Test: Torsion Level - 3 questions and answers have been prepared according to the Mechanical Engineering exam syllabus.The Test: Torsion Level - 3 MCQs are made for Mechanical Engineering 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Torsion Level - 3 below.
Solutions of Test: Torsion Level - 3 questions in English are available as part of our course for Mechanical Engineering & Test: Torsion Level - 3 solutions in Hindi for Mechanical Engineering course. Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free. Attempt Test: Torsion Level - 3 | 10 questions in 30 minutes | Mock test for Mechanical Engineering preparation | Free important questions MCQ to study for Mechanical Engineering Exam | Download free PDF with solutions
Test: Torsion Level - 3 - Question 1
Save

Two shafts are made of the same material and same outer diameter. With one having a circular section, and the other a hollow circular section with inner diameter, half that of outer diameter. The torque that can be transmitted by the solid section is N times that of the hollow section, where N is

Detailed Solution for Test: Torsion Level - 3 - Question 1
Given d = D /2

Ts =

N = 16/15

Test: Torsion Level - 3 - Question 2
Save

A solid phosphor bronze shaft 60 mm in diameter is rotating at 800 rpm and transmitting power. It is subjected to torsion only. An electrical resistance strain gauge mounted on the surface of the shaft, gives the strain reading as 3.98 × 10−4 . If the modulus of elasticity for bronze is 105 GN/m2 and Poisson’s ratio 0.3. The power transmitted by the shaft will be (Bending effect may be neglected) (Gage is mounted at 45o to the shaft axis).

Detailed Solution for Test: Torsion Level - 3 - Question 2

p1 = +τ, p1 = −τ strain gages

Internal at + 45° to axis.

Strain, ϵ =

= 3.98 × 10−4

= 3.98 × 10−4

τ, maximum shear stress =

=32.146 N/mm2

Torque,

= 1363366 Nmm = 1363.36 Nm

rpm, N = 800 Angular speed,

ω =

= 83.78 rad/sec

Power = ωT = 83.78 × 1363.36 Nm/s = 114216.6 W

= 114.216 kW

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Torsion Level - 3 - Question 3
Save

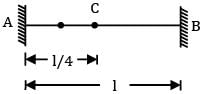
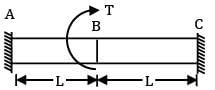
A round shaft of diameter 'd' and length 'l' fixed at both ends 'A' and 'B' is subjected to a twisting moment ‘T’ at 'C', at a distance of l/4 from A (see figure). The torsional stresses in the parts AC and CB will be

Detailed Solution for Test: Torsion Level - 3 - Question 3

τACLAC = τCBLCB

Test: Torsion Level - 3 - Question 4
Save

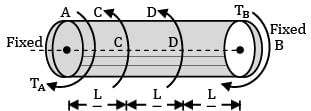
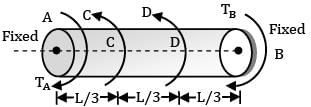
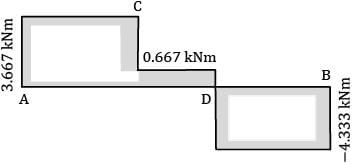
A horizontal shaft, L m long, is rigidly fixed at both the ends. At section C and D, torques 3 kNm (ccw) and 5 kNm (ccw) looking from the side A are applied. What are the fixing couples TA and TB?
Detailed Solution for Test: Torsion Level - 3 - Question 4

There are 3 portions AC, CD and DB, each of length L/3

Torque at A, say TA

Torque between CD = TA − 3kNm

Torque between DB = TA − 3 − 5

= TA − 8 kNm

Angular twist θAB = 0, since both the ends are fixed

θAC + θCD + θDB = 0

or TA + TA − 3 + TA − 8 = 0

3TA = 11

TA = 11/3 = 3.667 kNm

TA − 3 = 0.667 kNm

TA − 8 = −4.333 kNm = TB

Torque distribution is shown in figure

Test: Torsion Level - 3 - Question 5
Save

In a torsion test, the specimen is a hollow shaft with 50 mm external diameter and 30 mm internal diameter. An applied torque of 1.6 kNm is found to produce an angular twist of 0.40° measured on a length of 0.2 m of the shaft. Young’s modulus of elasticity obtained from the elastic test has been found to be 200 GPa.

Then the Poisson’s ratio will be
Detailed Solution for Test: Torsion Level - 3 - Question 5
Strain T = 1.6 kNm = 1.6 × 106 Nmm θ = 0.40 = = 6.98 × 10−3 rad

L = 200 mm d = 50 mm Polar moment,

= 61.38 × 104mm4

Shear modulus,

G =

= 74.7 × 103 N/mm2 (modulus of rigidity)

= 74.7 GPa

E = 2G[1 + ν] ν, Poisson’s ratio

=

= 0.338

Test: Torsion Level - 3 - Question 6
Save

Two beams are of same length and same weight. One beam is of solid circular section and the other is a hollow section. The internal diameter is half of the external diameter. The ratio of the strength of hollow section to that of solid section is
Detailed Solution for Test: Torsion Level - 3 - Question 6
Solid and hollow sections have same weight Wh = Ws γ × Vh = γ × Vs

Ah = As

D2 − d2 = d12

Assume k = d / D

D2(1 − k2 ) = d12

D √(1 − k2) = d1 ⋯ ①

=

=

=

=

Test: Torsion Level - 3 - Question 7
Save

A circular section rod ABC is fixed at ends A and C. It is subjected to torque T at B. !B = BC = L and the polar moment of inertia of portions AB and BC are 2 J and J respectively. If G is the modulus of rigidity, what is the angle of twist at point B?
Detailed Solution for Test: Torsion Level - 3 - Question 7

θAB = θBC

or

= 2TBC

TAB + TBC = T or TBC = T/3

or QB = QAB =

Test: Torsion Level - 3 - Question 8
Save

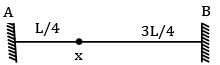
A solid circular rod AB of diameter D and length L is fixed at both ends. A torque T is applied at a section x such that !X = L/4 and BX = 3L/4. What is the maximum shear stress developed in the rod?
Detailed Solution for Test: Torsion Level - 3 - Question 8

θAX = θXB and TA + TB = T

or

or

τmax =

Test: Torsion Level - 3 - Question 9
Save

A hollow shaft having an inside diameter 60% of its outside diameter is to replace a solid shaft transmitting the same power at the same speed. If the material is the same, the percentage saving in the materials is
Detailed Solution for Test: Torsion Level - 3 - Question 9
Torque transmitted by solid shaft

T =

Torque transmitted by hollow shaft

T =

=

=

Torque transmitted is the same equate ① and ②

D = 0.9548 D0

Area of solid shaft. As =

= 0.716 D02

Area of hollow shaft,

AH =

=

= 0.502 D02

For the same material, the weight of the shaft is proportional to the areas Saving in material = saving in area

=

=

= 29.88%

Test: Torsion Level - 3 - Question 10
Save

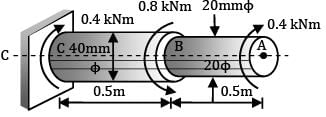
A stepped shaft ABC, 1m long made of steel is of diameter 20 mm from A to B = 0.5m, 40 mm from B to C = 0.5 m. It is subjected to a twisting moment of 0.4 kNm (cw) at A, 0.8 kNm (ccw) at B and 0.4 kNm (cw) at C. If G = 84 N/mm2, the angular twist between A and C. (in degrees) is ______________

 
Detailed Solution for Test: Torsion Level - 3 - Question 10

!B Torque = 0.4 kNm = 0.4 × 106 Nmm L = 500 mm

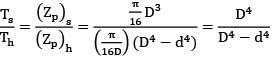
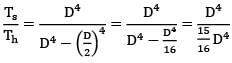
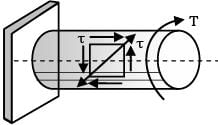
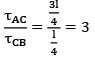
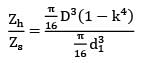
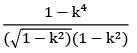
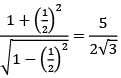
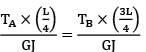
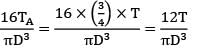
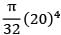J = = 1.5708 × 104 mm4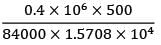

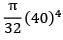θAB =

= 0.15158 rad = +8.685°

BC Torque = −0.4 kNm = −0.4 × 106 Nmm L = 500 mm

J = = 25.132 × 104 mm4

θBC =

= −0.9511 rad = −0.545o

Total angular twist, θAC = 8.685° − 0.545° = 8.14o

Information about Test: Torsion Level - 3 Page
In this test you can find the Exam questions for Test: Torsion Level - 3 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Torsion Level - 3, EduRev gives you an ample number of Online tests for practice

Top Courses for Mechanical Engineering

Download as PDF

Top Courses for Mechanical Engineering

Important Questions for Torsion Level - 3

Find all the important questions for Torsion Level - 3 at EduRev.Get fully prepared for Torsion Level - 3 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Torsion Level - 3 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Torsion Level - 3 resources.

Torsion Level - 3 MCQs with Answers

Prepare for the Torsion Level - 3 within the Mechanical Engineering exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Torsion Level - 3

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Torsion Level - 3 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup