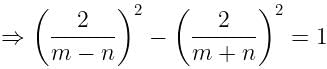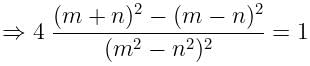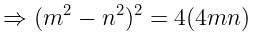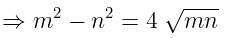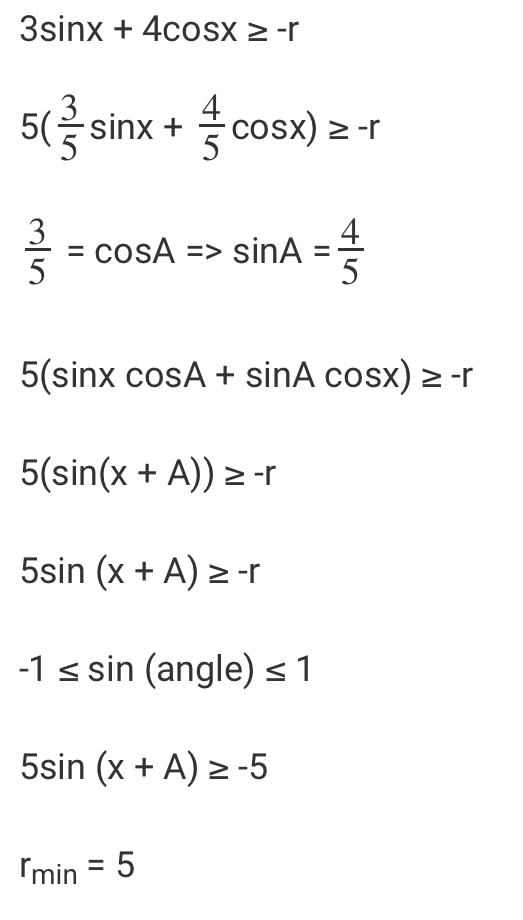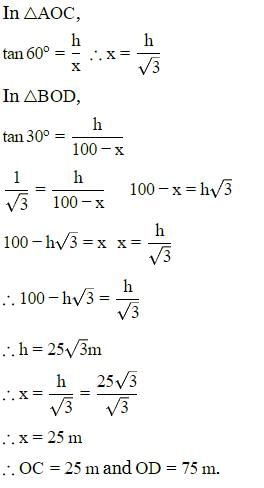Test: Trigonometry- 1 - Railways MCQ
10 Questions MCQ Test - Test: Trigonometry- 1
A student is standing with a banner at the top of a 100 m high college building. From a point on the ground, the angle of elevation of the top of the student is 60° and from the same point, the angle of elevation of the top of the tower is 45°. Find the height of the student.
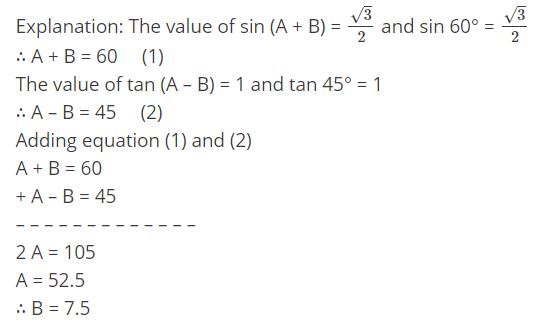
If sin (A + B) = √3 / 2 and tan (A – B) = 1. What are the values of A and B?
If tanØ + sinØ = m, tanØ - sinØ = n, find the value of m2 - n2.
If cos A + cos2 A = 1 and a sin12 A + b sin10 A + c sin8 A + d sin6 A - 1 = 0. Find the value of a+b / c+d
3sinx + 4cosx + r is always greater than or equal to 0. What is the smallest value ‘r’ can to take?
A right angled triangle has a height ‘p’, base ‘b’ and hypotenuse ‘h’. Which of the following value can h2 not take, given that p and b are positive integers?
If Cos x – Sin x = √2 Sin x, find the value of Cos x + Sin x:
Two poles of equal height are standing opposite to each other on either side of a road which is 100 m wide. Find a point between them on road, angles of elevation of their tops are 30∘ and 60∘. The height of each pole in meter, is:
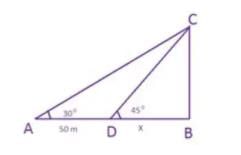
Anil looked up at the top of a lighthouse from his boat and found the angle of elevation to be 30 degrees. After sailing in a straight line 50 m towards the lighthouse, he found that the angle of elevation changed to 45 degrees. Find the height of the lighthouse.
The tops of two poles of height 30 m and 14 m are connected by a string. If the wire makes an angle of 30° with the horizontal, find the length of the wire.