Olympiad Test: Algebraic Expressions - Grade 7 MCQ
20 Questions MCQ Test - Olympiad Test: Algebraic Expressions
The constant term in the expression 1 + x2+ x is
The coefficient of y3 in the expression y − y3+ y2is
Get the algebraic expressions for subtraction of z from y.
When terms have the same algebraic factor, they are called __________.
An expression which contains two unlike terms is called _______.
Simplify these expressions and find their values, if x = 3, a = – 1, b = – 2.
3x – 5a – x2 + 9b
Simplify these expressions and find their values, if x = 3, a = – 1, b = – 2.
2b – 8x +4x2 + 4a
Simplify combining like terms: 3a – 2b – ab – (a – b + ab) + 3ab + b – a
The number of terms in the expression 1.2ab – 2.4 b + 3.6a is
A taxi charges $27 per km and a fixed charge of $45. If the taxi is hired for z km, which of the following is an algebraic expression to find the total fare?
What is the numerical coefficient of y2in the expression 2x2y - 15xy2 + 7y
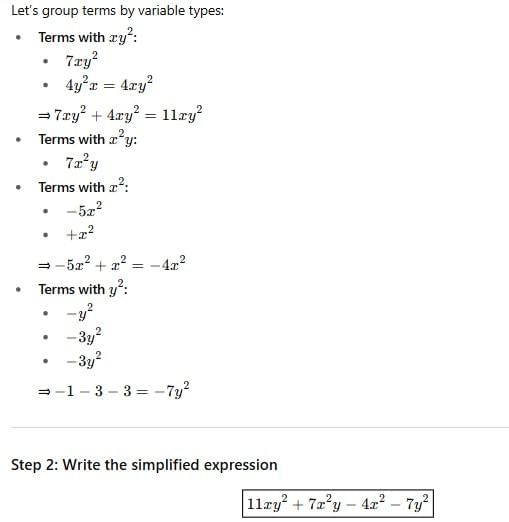
Simplify the following: 7xy2 − y2 + 7x2y − 5x2 − 3y2 + 4y2x − 3y2 + x2
Sita is y years old this year. Her brother is twice as old as her. How old was her brother 2 years ago?
From the following expressions 10pq, 7p, 8q, −p2q2, -7pq, -23, ab, 3a, b.The like terms are
The expression "twice the product of 3 and x" is the same as which of the following?
When a = 0, b = -1, find the value of 2a²b + 2ab² + ab.