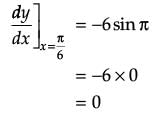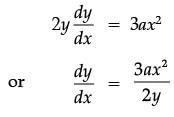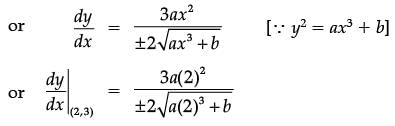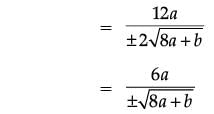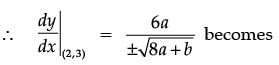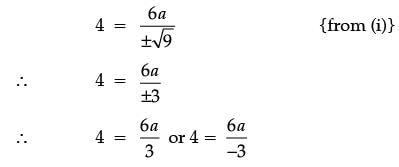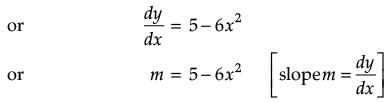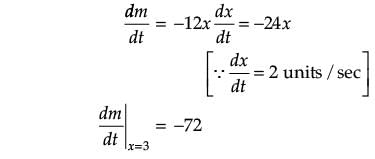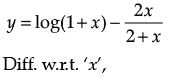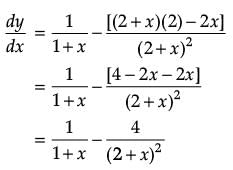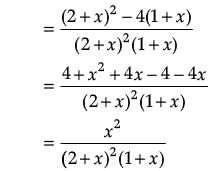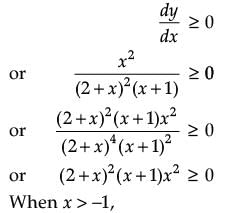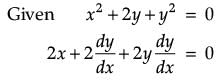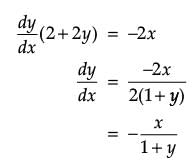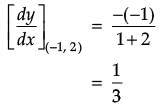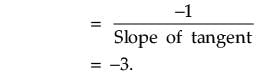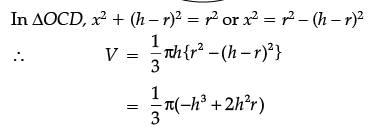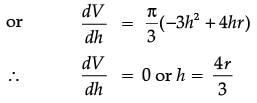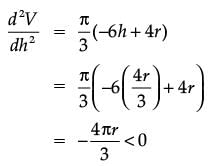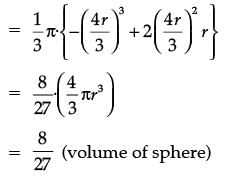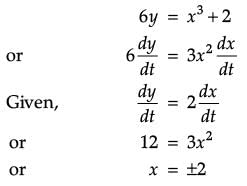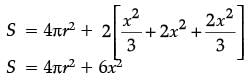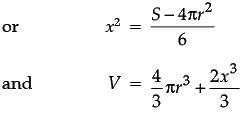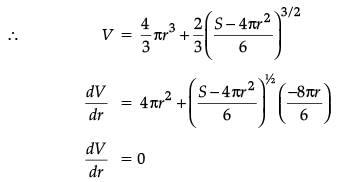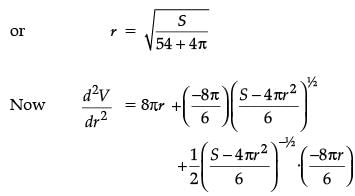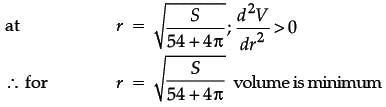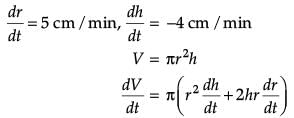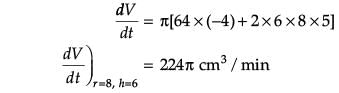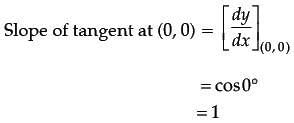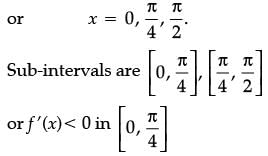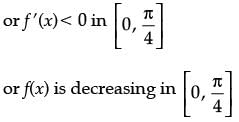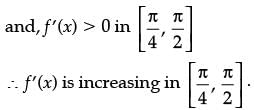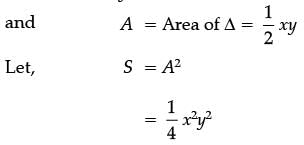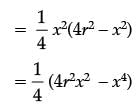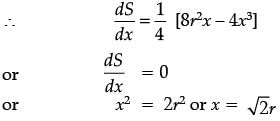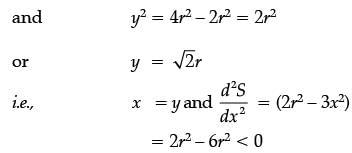All Courses
Get the App Signup / Login
Sign in Open App
Commerce Exam  >  Commerce Tests  >  Test: Application of Derivatives- Assertion & Reason Type Questions - Commerce MCQ

Test: Application of Derivatives- Assertion & Reason Type Questions - Commerce MCQ


Test Description

15 Questions MCQ Test - Test: Application of Derivatives- Assertion & Reason Type Questions

Test: Application of Derivatives- Assertion & Reason Type Questions for Commerce 2024 is part of Commerce preparation. The Test: Application of Derivatives- Assertion & Reason Type Questions questions and answers have been prepared according to the Commerce exam syllabus.The Test: Application of Derivatives- Assertion & Reason Type Questions MCQs are made for Commerce 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Application of Derivatives- Assertion & Reason Type Questions below.
Solutions of Test: Application of Derivatives- Assertion & Reason Type Questions questions in English are available as part of our course for Commerce & Test: Application of Derivatives- Assertion & Reason Type Questions solutions in Hindi for Commerce course. Download more important topics, notes, lectures and mock test series for Commerce Exam by signing up for free. Attempt Test: Application of Derivatives- Assertion & Reason Type Questions | 15 questions in 30 minutes | Mock test for Commerce preparation | Free important questions MCQ to study for Commerce Exam | Download free PDF with solutions
Test: Application of Derivatives- Assertion & Reason Type Questions - Question 1
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): At x = π/6, the curve y = 2cos2 (3x) has a vertical tangent.

Reason (R): The slope of tangent to the curve y = 2cos2 (3x) at x = π/6 is zero.

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 1

Given y = 2cos2(3x)

dy/dx = 2 x 2 x cos(3x) x (- sin 3x) x 3

dy/dx = -6 sin 6x dx

∴ R is true.

Since the slope of tangent is zero, the tangent is parallel to the X-axis. That is the curve has a horizontal tangent at x = π/6. Hence A is false.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 2
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): The function y = [x(x – 2)]2 is increasing in (0, 1) ∪ (2, ∞)

Reason (R): dy/dx = 0, when x = 0, 1, 2

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 2

y = [x(x-2]2

= [x2 - 2x]2

∴ dy/dx = 2(x2 - 2x) (2x - 2)

or dy/dx = 4x(x-1)(x-2)

On equating dy/dx = 0

4x(x - 1)(x - 2) = 0 ⇒ x = 0, x = 1, x = 2

∴ Intervals are (-∞=, 0), (0,1), (1,2), (2,∞)

Since, dy/dx > 0 in (0,1) or (2, ∞)

∴ f(x) is increasing in (0,1) ∪ (2, ∞)

Both A and R are true. But R is not the correct explanation of A.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Application of Derivatives- Assertion & Reason Type Questions - Question 3
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

The equation of tangent at (2, 3) on the curve y2 = ax3 + b is y = 4x – 5.

Assertion (A): The value of a is ±2

Reason (R): The value of b is ±7

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 3

y2 = ax3 + b

Differentiate with respect to x,

Since (2, 3) lies on the curve

y2 = ax3 + b

Or 9 = 8a + b …. (i)

Also from equation of tangent

y = 4x – 5

slope of the tangent = 4

either, a = 2 or a = - 2

For a = 2,

9 = 8(2) + b

or b = - 7

∴ a = 2 and b = - 7 and

for a = - 2,

9 = 8(- 2 )+b or b = 25

or a = - 2 and b = 25

Hence A is true and R is false.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 4
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

The total revenue received from the sale of x units of a product is given by R(x) = 3x2 + 36x + 5 in rupees.

Assertion (A): The marginal revenue when x = 5 is 66.

Reason (R): Marginal revenue is the rate of change of total revenue with respect to the number of items sold at an instance.
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 4

Marginal revenue is the rate of change of total revenue with respect to the number of items sold at an instance. Therefore R is true.

R’(x) = 6x + 36

R’(5) = 66

∴ A is true.

R is the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 5
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): The function f(x) = x3 – 3x2 + 6x – 100 is strictly increasing on the set of real numbers.

Reason (R): A strictly increasing function is an injective function.
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 5

f(x) = x3 - 3x2+ 6x - 100

f(x) = 3x2 - 6x + 6

= 3[x2 - 2x + 2]

= 3[(x-1)2+1]

Since f’(x) > 0; x ∊ R

f(x) is strictly increasing on R.

Hence A is true.

For a strictly increasing function,

x1 > x2

⇒ f(x1) > f(x2)

i.e.) x1 = x2

⇒ f(x1) — f(x2)

Hence, a strictly increasing function is always an injective function.

So R is true.

But R is not the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 6
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): For the curve y = 5x – 2x3, if x increases at the rate of 2 units/sec, then at x = 3 the slope of curve is decreasing at 36 units/sec.

Reason (R): The slope of the curve is dy/dx
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 6

The slope of the curve y = f(x) is dy/dx. R is true

Given

Curve is y = 5x – 2x3

Rate of Change of the slope is decreasing by 72 units/s.

A is false.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 7
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): The function is a decreasing function of x throughout its domain.

Reason (R): The domain of the function
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 7

log (1 + x) is defined only when x + 1 > 0 or x > -1

Hence R is true.

For increasing function,

dy/dx is always greater than zero.

Is always increasing throughout its domain.

Hence A is false.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 8
Save

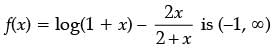
Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): The slope of normal to the curve x2 + 2y + y2 = 0 at (–1, 2) is –3.

Reason (R): The slope of tangent to the curve x2 + 2y + y2 = 0 at (–1, 2) is 1/3
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 8

Slope of tangent at (-1,2)

Hence R is true.

Slope of normal at (-1,2)

Hence A is true.

R is the correct explanation for A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 9
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

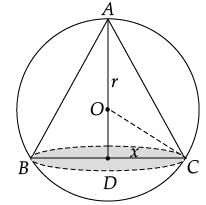
Assertion (A): The altitude of the cone of maximum volume that can be inscribed in a sphere of radius r is 4r/3.

Reason (R): The maximum volume of the cone is 8/27 of the volume of the sphere.
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 9

Let radius of cone be x and its height be h.

∴ OD = (h – r)

Volume of cone

∴ at h = 4r/3, Volume is maximum

Maximum volume

Hence both A and R are true.

R is not the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 10
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

A particle moves along the curve 6y = x3 + 2.

Assertion (A): The curve meets the Y axis at three points.

Reason (R): At the points (2,5/3) and (–2, –1) the ordinate changes two times as fast as the abscissa.
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 10

On Y axis, x = 0. The curve meets the Y axis at only one point, i.e., (0,⅓)

Hence A is false.

Put x = 2 and –2 in the given equation to get y

∴ The points are (2, 5/3), (-2,-1)

R is true.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 11
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

The sum of surface areas (S) of a sphere of radius ‘r’ and a cuboid with sides x/3, x and 2x is a constant.

Assertion (A): The sum of their volumes (V) is minimum when x equals three times the radius of the sphere.

Reason (R): V is minimum when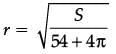

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 11

Given

i.e., r2(54 + 4p) = S

or r2(54 + 4p) = 4?r2 + 6x2

or 6x2 = 54r2

or x2 = 9r2

or x = 3r

Hence both A and R are true.

R is the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 12
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

The radius r of a right circular cylinder is increasing at the rate of 5 cm/min and its height h, is decreasing at the rate of 4 cm/min.

Assertion (A): When r = 8 cm and h = 6 cm, the rate of change of volume of the cylinder is 224p cm3/min

Reason (R): The volume of a cylinder is

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 12

The volume of a cylinder is V=?r2h.

So R is false.

∴ Volume is increasing at the rate of 224? cm3/min.

∴ A is true.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 13
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Assertion (A): The equation of tangent to the curve y = sin x at the point (0, 0) is y = x.

Reason (R): If y = sin x, then dy/dx at x = 0 is 1
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 13

Given y = sin x

dy/dx = cos x

∴ R is true.

Equation of tangent at (0, 0) is y – 0 = 1(x – 0)

⇒ y = x.

Hence A is true.

R is the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 14
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

Consider the function f(x) = sin4x + cos4x.

Assertion (A): f(x) is increasing in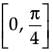

Reason (R): f(x) is decreasing in

Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 14

f(x) = sin4 x + cos4 x

or

f ’(x) = 4sin3 xcos x – 4cos3 xsin x

= – 4sin xcos x [– sin2 x + cos2 x]

= – 2sin 2x cos 2x

= – sin 4x

On equating,

f ’(x) = 0

or – sin 4x = 0

or 4x = 0, ?, 2?, ...........

Both A and R are true. But R is not the correct explanation of A.

Test: Application of Derivatives- Assertion & Reason Type Questions - Question 15
Save

Directions : In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as.

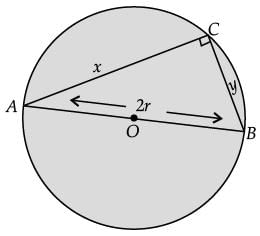
AB is the diameter of a circle and C is any point on the circle.

Assertion (A): The area of DABC is maximum when it is isosceles.

Reason (R): DABC is a right-angled triangle.
Detailed Solution for Test: Application of Derivatives- Assertion & Reason Type Questions - Question 15

Let the sides of rt.? ABC be x and y

∴ x2 + y2 = 4r2

or Area is maximum, when ? is isosceles.

Hence A is true.

Angle in a semicircle is a right angle. ∠C = 90°

⇒ ?ABC is a right-angled triangle.

∴ R is true.

R is the correct explanation of A.

Information about Test: Application of Derivatives- Assertion & Reason Type Questions Page
In this test you can find the Exam questions for Test: Application of Derivatives- Assertion & Reason Type Questions solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Application of Derivatives- Assertion & Reason Type Questions, EduRev gives you an ample number of Online tests for practice

Top Courses for Commerce

Download as PDF

Top Courses for Commerce

Important Questions for Application of Derivatives- Assertion & Reason Type Questions

Find all the important questions for Application of Derivatives- Assertion & Reason Type Questions at EduRev.Get fully prepared for Application of Derivatives- Assertion & Reason Type Questions with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Application of Derivatives- Assertion & Reason Type Questions syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Application of Derivatives- Assertion & Reason Type Questions resources.

Application of Derivatives- Assertion & Reason Type Questions MCQs with Answers

Prepare for the Application of Derivatives- Assertion & Reason Type Questions within the Commerce exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Application of Derivatives- Assertion & Reason Type Questions

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Application of Derivatives- Assertion & Reason Type Questions with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup