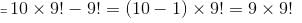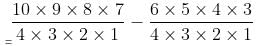Test Level 1: Permutation & Combination - 2 - CAT MCQ
20 Questions MCQ Test - Test Level 1: Permutation & Combination - 2
The number of squares in a board having 10 rows and 9 columns is
Find the last digit of the sum 1! + 2! + 3! + ………… + 100!.
Two packs of 52 cards are shuffled together. The number of ways in which a man can be dealt 26 cards, so that he does not get two cards of the same suit and same denominations is
In how many ways will a particular person be found among those six if six persons are to be selected out of ten?
A 7-character code is such that only even digits occur at even places and only odd digits occur at odd places, e.g. 1436789. How many such codes can be made from digits 1-9, if repetition of digits is allowed?
Five boxes are numbered from 1 to 5. A ball is to be placed in each box and balls of three different colours Red, Blue and Green are available. After this, a code is generated as *****, where the first * represents the first letter of colour of the ball in that box, and so on so forth (e.g. RBBGR and RBBRG are two different codes). How many different codes will result?
If 10P5 × 4P3 = 6P5 × X × 2, then X is
What is the difference between total number of arrangements when 10 members are arranged in a row and in a circle?
How many numbers lying between 600 and 700 can be formed with the digits 0-9, where 7 occurs at exactly one of the positions (repetition is allowed)?
In how many ways can 6 awards be distributed among 9 actors, if each actor can get any number of awards?
There are 6 black balls and 4 white balls in a box. In how many ways can 4 balls be selected, in which there is at least one white ball?
An apparel shop has 15 different shades of T-shirts and 35 different shades of shorts. In how many different ways can one buy two items containing only one shade of T-shirt and one shade of shorts?
How many numbers of 3 digits can be formed with the digits 1, 2, 3, 4, 5 (repetition of digits not allowed)?
In how many ways can a person send invitation cards to 6 of his friends if he has four servants to distribute the cards?
In how many ways can 7 Indians, 5 Pakistanis and 6 Dutch be seated in a row so that all persons of the same nationality sit together?
There are 4 qualifying examinations to enter into Oxford University: RAT, BAT, SAT, and PAT. An Engineer cannot go to Oxford University through BAT or SAT. A CA on the other hand can go to the Oxford University through the RAT, BAT & PAT but not through SAT. Further there are 3 ways to become a CA(viz., Foundation, Inter & Final). Find the ratio of number of ways in which an Engineer can make it to Oxford University to the number of ways a CA can make it to Oxford University.
In how many ways can the letters of the word PATNA be rearranged?
How many numbers of four digits can be formed with the digits 0, 1, 2, 3 (repetition of digits being allowed?