Test: Linear Equations in One Variable - 2 - ACT MCQ
15 Questions MCQ Test - Test: Linear Equations in One Variable - 2
The sum of three consecutive number is 126. Find the highest number?
In a group of 100 students, each student offers exactly 8 subjects, and exactly 10 students take each subject. Find the total number of different subjects.
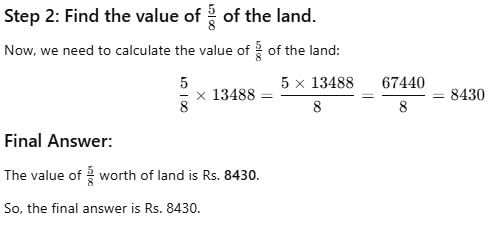
Find the 5/8 worth of land whose 3/4 worth be Rs. 10116.
A total of 324 notes comprising of Rs. 20 and Rs. 50 denominations make a sum of Rs. 12450. The number of Rs. 20 notes is
If 1/3rd of the first of the three consecutive odd numbers is 2 more than 1/5th of the third number, then the second number is?
If y2 = y + 7, then what is the value of y3?
Jane won a lottery and gets 1/3rd of the winning amount and donates Rs. 6000 which is 1/6th of amount he got, find how much the lottery was worth.
Rajeev was to earn Rs. 500 and a free holiday for seven weeks’ work. He worked for only 5 weeks and earned Rs. 50 and a free holiday. What was the value of the holiday?
If 80% of my age 6 years ago is the same as 60% of my age after 10 years. What is the product of digits of my present age?
Find the product of two consecutive numbers where four times the first number is 10 more than thrice the second number.
A woman had only 50 paisa and 1 rupee coins in her purse. The total number of coins were 120 and the total amount was Rs.100. So the number of 1 rupee coins are_______.
A is 5 years older than B and 10 years younger than C. If the sum of ages of A, B and C together after 5 years is 80 years. Find the present age of B.
In 10 years, a father will be twice as old as his son. Five years ago, the father was three times as old as his son. Find their current ages.