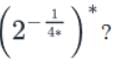Test: Functions- 2 - Railways MCQ
20 Questions MCQ Test - Test: Functions- 2
If [x] denotes the greatest integer less than or equal to x, what is the value of [x]?
(1) When x2 is divided by 9, the quotient is zero.
(2) 2x2+9x+10=0
For the positive integers k, m and n, k(m)n means that the remainder when m is divided by n is k. If k(13766)9 and p(137)k, where p is a positive integer, then p is equal to.
If f(z) = 2z+1, then which of the following can be the value of x for which f(1)∗f(2x)=f(x−1)∗{f(4)+f(−1)}−f(1)?
For a positive integer x, the function f(x) is defined as f(x)=2x2+3x+4
. Is the value of the function f(x) an even number?
- x is a multiple of 3
- x is a prime number
For all positive integers x, when x is even and
when x is odd. What is the value of positive integer x?
(1) The highest power of 2 that divides &(4x) completely is 36
(2) The highest power of 2 that divides (&4)*x completely is 8
The function PF is defined as PF(a) = k, where k is the number of prime factors of positive integer a. If PF(x) = PF(2x)=PF(3x) = 2 and PF(y) = PF(5y) = PF(7y) = 2, where x and y are positive integers, what is the value of PF(least common multiple of x and y)?
For any integers x and y, min(x, y) and max(x, y) denote the minimum and the maximum of x and y, respectively. For example, min(2, 1) = 1 and max(2,1) = 2. If a, b, c and d are distinct positive integers, is max(a, max(b, min(c, d))) = max(d, max(a, min(b, c))) ?
(1) b, c and d are factors of a
(2) a – 2d = b + c
The function f(x) is defined as where x is not equal to -1. If a is not equal to -1 or 0, which of the following expressions must be true for all values of a?
If % denotes one of the arithmetic operations, addition, subtraction, multiplication or division, and if z is an integer, what is the value of 2z%z?
(1) 3%(2z)=−(z%1)
(2) (3z)%(z−1)=5
For all positive numbers a and b, the function {a, b} = k, where k is the multiple of b nearest to a.. If x and y are positive integers such that x/y= 15.25 and {xy,y} = 16, what is the value of y?
The distance travelled (D) by a car in miles when it uses more than 1 liter of fuel is given by D= x +kv, where v is the liters of fuel used, and x, and k are constants. If the car uses 4 liters of fuel to travel 53 miles and 8 liters of fuel to travel 101 miles, how much fuel in litres will the car require to travel 149 miles?
If [z] denotes the least integer greater than or equal to z and [z2] = 2, which of the following could be the value of [z]?
I. 2
II. 1
III. -2
For distinct positive integers x and y, where x < y, the function FP(x, y) returns the smallest prime number between x and y, exclusive, or the text string ‘NULL’ if no such number is found. If FP(a, b) +FP(c, d) = FP(e, f), where a, b, c, d, e and f are distinct positive integers, what is the value of ca ?
(1) FP(g, h) = a, where g and h are distinct positive integers
(2) c is less than the minimum possible value of the function FP(x,y).
A function f(x) is defined as f(x) = x + x2 for an integer x. If a is an integer, is f(a) a prime number?
1. f(a)+f(a+1) is a prime number
2. The greatest common divisor of f(3) & f(4) is f(a)*2.
The function Ro rounds a positive decimal number to its nearest integer starting from its rightmost digit. What is the value of Ro(64.4ab5), where a and b are single digit positive integers?
(1) Ro(36.4a7) = 37
(2) Ro(87.b6) = 88
The operation @ is defined for all distinct and nonzero a and b by
The symbols @ and # each denote one of the four arithmetic operations: addition, subtraction, multiplication and division. If the symbols denote different arithmetic operations such thatand a@b#c = c#b@a, where a, b and c are distinct positive integers, what is the value of 4@5#4?
A function f(x) is defined as f(x)=3x2−20x+c, where c is a constant. Also given f(1) = -16. What is the value of f(c) + f(-c) ?