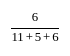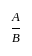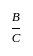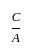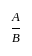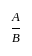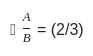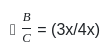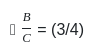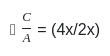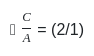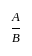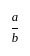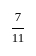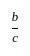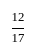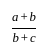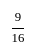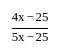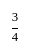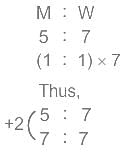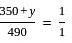CTET & State TET Exam > CTET & State TET Tests > Mathematics Paper 2 (Ratio and Proportion) - CTET & State TET MCQ
Mathematics Paper 2 (Ratio and Proportion) - CTET & State TET MCQ
Test Description
10 Questions MCQ Test - Mathematics Paper 2 (Ratio and Proportion)
Mathematics Paper 2 (Ratio and Proportion) for CTET & State TET 2025 is part of CTET & State TET preparation. The Mathematics Paper 2 (Ratio and Proportion) questions and answers have been prepared
according to the CTET & State TET exam syllabus.The Mathematics Paper 2 (Ratio and Proportion) MCQs are made for CTET & State TET 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Mathematics Paper 2 (Ratio and Proportion) below.
Solutions of Mathematics Paper 2 (Ratio and Proportion) questions in English are available as part of our course for CTET & State TET & Mathematics Paper 2 (Ratio and Proportion) solutions in
Hindi for CTET & State TET course.
Download more important topics, notes, lectures and mock test series for CTET & State TET Exam by signing up for free. Attempt Mathematics Paper 2 (Ratio and Proportion) | 10 questions in 10 minutes | Mock test for CTET & State TET preparation | Free important questions MCQ to study for CTET & State TET Exam | Download free PDF with solutions
Mathematics Paper 2 (Ratio and Proportion) - Question 1
The salary of A, B and C are in the ratio of 5 : 11 : 6. If their total salary is Rs.2750, find the salary received by C?(in Rs)
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 1
Mathematics Paper 2 (Ratio and Proportion) - Question 2
The sum of the three numbers is 370. The first number is 1/4th of the third number, and the ratio of the second number to the third is 3 : 5. Find the third number
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 2
Mathematics Paper 2 (Ratio and Proportion) - Question 3
If A ∶ B ∶ C = 2 ∶ 3 ∶ 4 then 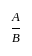 ∶
∶ 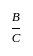 ∶
∶ 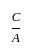 is equal to -
is equal to -
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 3
Mathematics Paper 2 (Ratio and Proportion) - Question 4
In a box, there are ₹10 notes, ₹20 notes and ₹50 in a ratio of 3 ∶ 5 ∶ 7. The total amount of notes is ₹3,360. Find the number of ₹20 notes and ₹50 notes taken together.
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 4
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 5
Mathematics Paper 2 (Ratio and Proportion) - Question 6
Ravi, Ramesh and Suresh can work together for ₹1,680. If Ravi and Ramesh together are to do 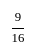 of the work, then the share (in ₹) of the Suresh should be:
of the work, then the share (in ₹) of the Suresh should be:
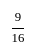 of the work, then the share (in ₹) of the Suresh should be:
of the work, then the share (in ₹) of the Suresh should be:
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 6
Mathematics Paper 2 (Ratio and Proportion) - Question 7
What is the number of girls if the total number of students is 2400 and the ratio of boys to girls is 7:5?
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 7
Mathematics Paper 2 (Ratio and Proportion) - Question 8
x varies directly as the square of y and inversely as the cube root of z and x = 2, when y = 4, z= 8. What is the value of y when x = 3, and z = 27?
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 8
Mathematics Paper 2 (Ratio and Proportion) - Question 9
Two numbers are in ratio of 4 ∶ 5 respectively. If each number is reduced by 25, then the ratio becomes 3 ∶ 4. Find the largest number.
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 9
Mathematics Paper 2 (Ratio and Proportion) - Question 10
The ratio of number of men and women in a ice-cream factory of 840 workers is 5 : 7. How many more men should be joined to make the ratio 1 : 1?
Detailed Solution for Mathematics Paper 2 (Ratio and Proportion) - Question 10
Information about Mathematics Paper 2 (Ratio and Proportion) Page
In this test you can find the Exam questions for Mathematics Paper 2 (Ratio and Proportion) solved & explained in the simplest way possible.
Besides giving Questions and answers for Mathematics Paper 2 (Ratio and Proportion), EduRev gives you an ample number of Online tests for practice
Download as PDF