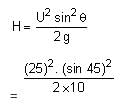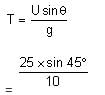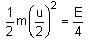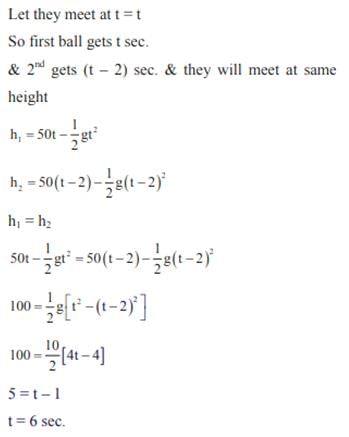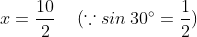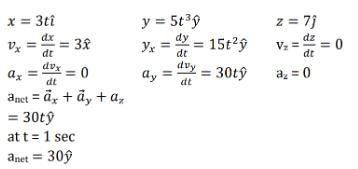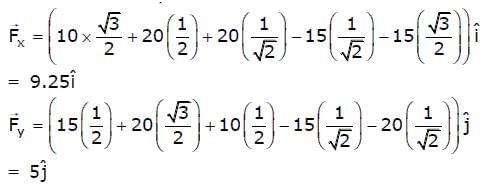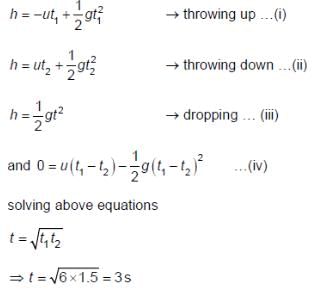Test: Motion in a Plane (May 21) - JEE MCQ
15 Questions MCQ Test - Test: Motion in a Plane (May 21)
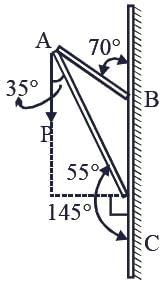
Consider a frame that is made up of two thin massless rods AB and AC as shown in the figure.
A vertical force 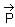 of magnitude 100 N is applied at point A of the frame.
of magnitude 100 N is applied at point A of the frame.
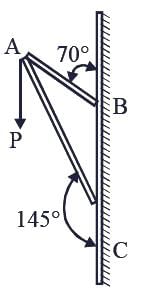
Suppose the force is 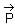 resolved parallel to the arms AB and AC of the frame. The magnitude of the resolved component along the arm AC is xN. The value of x, to the nearest integer, is _________.
resolved parallel to the arms AB and AC of the frame. The magnitude of the resolved component along the arm AC is xN. The value of x, to the nearest integer, is _________.
[Given: sin (35°) = 0.573, cos (35°) = 0.819 sin (110°) = 0.939, cos (110°) = -0.342]
A vertical force
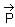 of magnitude 100 N is applied at point A of the frame.
of magnitude 100 N is applied at point A of the frame.
Suppose the force is
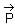 resolved parallel to the arms AB and AC of the frame. The magnitude of the resolved component along the arm AC is xN. The value of x, to the nearest integer, is _________.
resolved parallel to the arms AB and AC of the frame. The magnitude of the resolved component along the arm AC is xN. The value of x, to the nearest integer, is _________.[Given: sin (35°) = 0.573, cos (35°) = 0.819 sin (110°) = 0.939, cos (110°) = -0.342]
A player kicks a football with an initial speed of 25 ms-1 at an angle of 45° from the ground. What are the maximum height and the time taken by the football to reach the highest point during motion? (Take g = 10 ms-2)
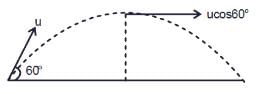
A ball is projected with kinetic energy E, at an angle of 60° to the horizontal. The kinetic energy of this ball at the highest point of its flight will become:
A ball is projected from the ground with a speed 15 ms-1 at an angle θ with horizontal so that its range and maximum height are equal, then tan θ will be equal to
A ball is projected vertically upward with an initial velocity of 50 ms-1 at t = 0 s. At t = 2 s, another ball is projected vertically upward with same velocity. At t = _______ s, second ball will meet the first ball (g = 10 ms-2). (in integers)
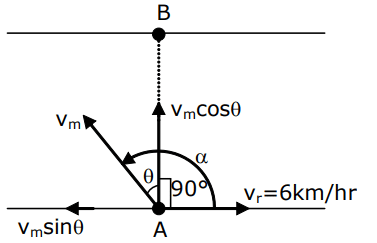
A swimmer can swim with velocity 12 km/h in still water. Water flowing in a river has velocity 6 km/h. The direction with respect to the direction of flow of river water he should swim in order to reach the point on the other bank just opposite to his starting point is __________ (in degrees).
(Round off to the nearest integer)
A person is swimming with a speed of 10 m/s at an angle of 120° with the flow and reaches a point directly opposite on the other side of the river. The speed of the flow is 'x' m/s. The value of 'x' to the nearest integer is ______.
A swimmer wants to cross a river from point A to point B. Line AB makes an angle of 30° with the flow of river. Magnitude of velocity of the swimmer is same as that of the river. The angle θ with the line AB should be _____ °, so that the swimmer reaches point B. (in integers)
At time t = 0, a particle starts travelling from a height 7 cm in a plane keeping z coordinate constant. At any instant of time, its position along the
 and
and  directions is defined as 3t and 5t3, respectively. At t = 1 s, acceleration of the particle will be
directions is defined as 3t and 5t3, respectively. At t = 1 s, acceleration of the particle will be
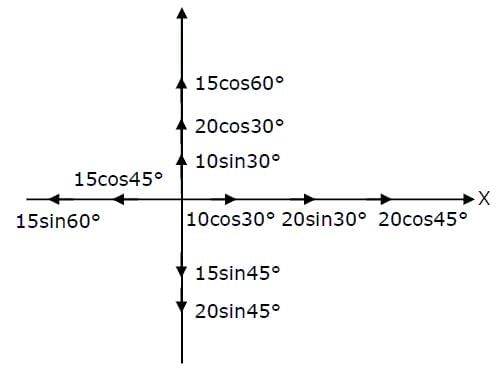
The resultant of these force and
is approximately ___________ N.
[Take = 1.7,
= 1.4 Given
and
unit vector along x, y axis]
Two vectors and
have equal magnitudes. If magnitude of
is equal to two times the magnitude of
, then the angle between
and
will be:
A body of mass 2 kg moves under a force of N. It starts from rest and was at the origin initially. After 4s, its new coordinates are (8, b, 20). The value of b is ________. (Round off to the nearest integer)
Statement I: Two forces and
where
, when act at an angle
to each other, the magnitude of their resultant is
, when they act at an angle
, the magnitude of their resultant becomes
. This is possible only when
.
Statement II: In the situation given above.
= 60° and
= 90°
In the light of the above statements, choose the most appropriate answer from the options given below
A particle of mass m is projected with a speed u from the ground at an angle θ = π / 3 w.r.t. horizontal axis (x-axis). When it has reached its maximum height, it collides completely inelastically with another particle of the same mass and velocity u. The horizontal distance covered by the combined mass before reaching the ground is:
From the top of a tower, a ball is thrown vertically upward which reaches the ground in 6 s. A second ball thrown vertically downward from the same position with the same speed reaches the ground in 1.5 s. A third ball released, from the rest from the same location, will reach the ground in ____ s.(In Integers)