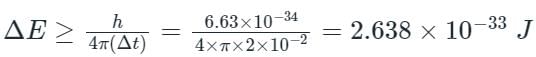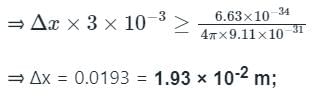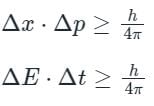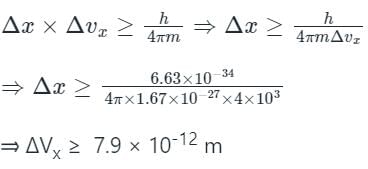Test: Heisenberg's Uncertainty Principle - EmSAT Achieve MCQ
10 Questions MCQ Test - Test: Heisenberg's Uncertainty Principle
The error in the measurement of lifetime of an atom is 2 × 10-2 sec. What is the minimum uncertainty in its energy in eV?
The particles that are indistingishable and obey both Heisenberg's uncertainty principle and Pauli's exclusion principle obey
Which law found the corelation between the lifetime of the half-life of the alpha emitter and the alpha energies?
Uncertainty in the position of an electron moving with a velocity 300 m/s accurate up to 0.001%, will be
Which of the following statements are correct?
The uncertainty in measuring velocity of a proton is 4×103 m/s. The minimum uncertainty involved in measuring the position of proton will be?
Which other variable pairs (other than momentum and position) are important observables in Heisenberg’s uncertainty principle?
A ball of mass 0.5kg is moving with velocity 6.626 m/s. What’s the wavelength of that ball?
Mass of a photon is given by 3.313 x 10-34 kg. Find it’s wavelength.