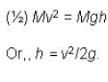All Courses
Get the App Signup / Login
Sign in Open App
JEE Exam  >  JEE Tests  >  Test: Rolling Motion - JEE MCQ

Test: Rolling Motion - JEE MCQ


Test Description

10 Questions MCQ Test - Test: Rolling Motion

Test: Rolling Motion for JEE 2024 is part of JEE preparation. The Test: Rolling Motion questions and answers have been prepared according to the JEE exam syllabus.The Test: Rolling Motion MCQs are made for JEE 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Rolling Motion below.
Solutions of Test: Rolling Motion questions in English are available as part of our course for JEE & Test: Rolling Motion solutions in Hindi for JEE course. Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt Test: Rolling Motion | 10 questions in 10 minutes | Mock test for JEE preparation | Free important questions MCQ to study for JEE Exam | Download free PDF with solutions
Test: Rolling Motion - Question 1
Save

A spherical solid ball rolls on a table. What fraction of its total K.E. is rotational K.E. ?

Detailed Solution for Test: Rolling Motion - Question 1

https://edurev.gumlet.io/ApplicationImages/Temp/540824_9230a68a-782f-4f14-a007-3719b083800f_lg.png

Test: Rolling Motion - Question 2
Save

A body is rolling down an inclined plane without slipping. How does the acceleration of the rolling body depend on its radius?

Detailed Solution for Test: Rolling Motion - Question 2

The acceleration of a rolling body is independent of its mass and radius.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Rolling Motion - Question 3
Save

Acceleration (a) of a body of radius R rolling down an inclined plane of inclination is given by

Test: Rolling Motion - Question 4
Save

What is the moment of inertia of a solid sphere about its diameter?
Detailed Solution for Test: Rolling Motion - Question 4

We have to consider solid sphere contribution of infinite small solid cylinder.
Icylinder = ½ MR²
dI = ½ dmr²
dm = pdV
dV = πr²dx
By substituting dV into dm
dm = pπr²dx
Now dm into dI
dI = ½ r²pπr²dx = ½ pπr⁴dx
Now, r² = R²-x²
dI = ½ pπ(R²-x²)²dx
Integrate both sides
I = ½ pπ 16/15 R²
P = M/V
P = M/4/3πR³ (density of sphere)
After substituting
I = 2/5MR2

Test: Rolling Motion - Question 5
Save

What is the coefficient of friction when a cylinder rolls down without slipping on an inclined plane?
Detailed Solution for Test: Rolling Motion - Question 5

Test: Rolling Motion - Question 6
Save

If a solid cylinder rolls up along a inclined plane with an initial velocity v. It will rise up to a height h equal to
Test: Rolling Motion - Question 7
Save

What will be the speed of center of mass, if a solid sphere of radius 20 cm which rotates at 2 rps along a straight line?
Detailed Solution for Test: Rolling Motion - Question 7

V = rw
V = 20 × 10-2 × 2π(2)
V = 2.51 m/s

Test: Rolling Motion - Question 8
Save

Two discs A and B are mounted on a vertical axle. Suppose the discs have moment of inertia as I and 2 I respectively about an axis. Disc A is given angular velocity ω, using the potential energy of a spring compressed by x1. Similarly the potential energy of a spring compressed by x2 gives an angular velocity of 2ω to Disc B. Considering that both the discs rotate in same direction .Find the ratio x1/ x2 :
Test: Rolling Motion - Question 9
Save

A small cylinder rolling with a velocity v along a horizontal surface encounters a smooth inclined surface. The height ‘h’ up to which the cylinder will ascend is
Detailed Solution for Test: Rolling Motion - Question 9

A body can roll along a surface only if the surface is rough. The body will roll up to the foot of the inclined smooth surface. It will continue to spin with the angular speed it has acquired, and will slide up to a certain height, maintaining its spin motion throughout the smooth surface. Its translational kinetic energy alone is responsible for its upward motion along the smooth incline so that the height up to which it will rise is given by

Test: Rolling Motion - Question 10
Save

The kinetic energy of a rolling wheel is
Detailed Solution for Test: Rolling Motion - Question 10

The total KE is the sum of translational KE and rotational KE.

Information about Test: Rolling Motion Page
In this test you can find the Exam questions for Test: Rolling Motion solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Rolling Motion, EduRev gives you an ample number of Online tests for practice

Top Courses for JEE

Download as PDF

Top Courses for JEE

Important Questions for Rolling Motion

Find all the important questions for Rolling Motion at EduRev.Get fully prepared for Rolling Motion with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Rolling Motion syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Rolling Motion resources.

Rolling Motion MCQs with Answers

Prepare for the Rolling Motion within the JEE exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Rolling Motion

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Rolling Motion with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup