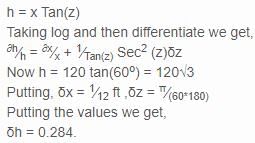Explore Exams
Login Signup
Sign in Open App
JEE Exam  >  JEE Test  >  Mathematics (Maths) Main & Advanced  >  Test: Rate of Change And Approximations - JEE MCQ

Rate of Change And Approximations - Free MCQ Practice Test with solutions,


MCQ Practice Test & Solutions: Test: Rate of Change And Approximations (10 Questions)

You can prepare effectively for JEE Mathematics (Maths) for JEE Main & Advanced with this dedicated MCQ Practice Test (available with solutions) on the important topic of "Test: Rate of Change And Approximations". These 10 questions have been designed by the experts with the latest curriculum of JEE 2026, to help you master the concept.

Test Highlights:

  • - Format: Multiple Choice Questions (MCQ)
  • - Duration: 10 minutes
  • - Number of Questions: 10

Sign up on EduRev for free to attempt this test and track your preparation progress.

Test: Rate of Change And Approximations - Question 1
Save

The radius of air bubble is increasing at the rate of 0. 25 cm/s. At what rate the volume of the bubble is increasing when the radius is 1 cm.​

Test: Rate of Change And Approximations - Question 2
Save

The total revenue in Rupees received from the sale of x units of a product is given by R(x) = 5x2 + 22x + 35. Find the marginal revenue, when x = 7, where by marginal revenue we mean the rate of change of total revenue with respect to the number of items sold at an instant

Detailed Solution: Question 2

Clear all your doubts with EduRev
Test: Rate of Change And Approximations - Question 3
Save

Find the approximate change in total surface area of a cube of side x metre caused by increase in side by 1%.​

Test: Rate of Change And Approximations - Question 4
Save

The volume of cube is increasing at the constant rate of 3 cm3/s. Find the rate of change of edge of the cube when its edge is 5 cm.​

Detailed Solution: Question 4

Let V be the instantaneous volume of the cube.
dV/dt = 3 cm3/s
Let x be the side of the cube.
V = x3
dV/dt = 3x2 * (dx/dt)
3 = 3*(52)*(dx/dt)
So, dx/dt = 1/25 cm/s

Test: Rate of Change And Approximations - Question 5
Save

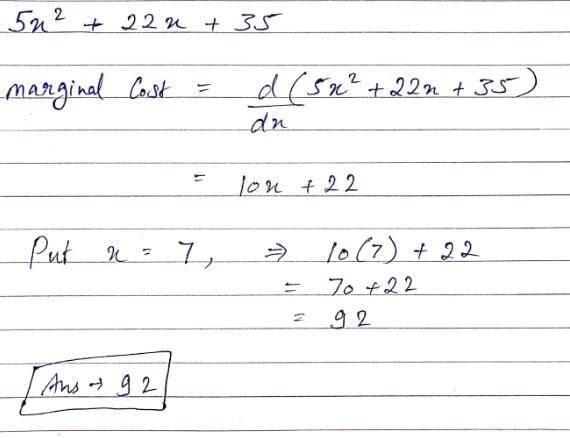
The total cost associated with the production of x units of a product is given by C(x) = 5x2 + 14x + 6. Find marginal cost when 5 units are produced

Detailed Solution: Question 5

C(x) = 5x2 + 14x + 6
Marginal Cost M(x) = C’(x)
M(x) = 10x +14
So, M(5) = 50 + 14
= 64 Rs

Test: Rate of Change And Approximations - Question 6
Save

At a distance of 120ft from the foot of a tower the elevation of its top is 60o. If the possible error in measuring the distance and elevation are 1 inch and 1 minute, find approximate error in calculated height.

Detailed Solution: Question 6

Tan(z) = h⁄x

Test: Rate of Change And Approximations - Question 7
Save

A stone is dropped into a quiet lake and waves move in circles at a speed of 2cm per second. At the instant, when the radius of the circular wave is 12 cm, how fast is the enclosed area changing ?​

Detailed Solution: Question 7

Rate of increase of radius dr/dt = 2 cm/s
Area of circle A = πr2
dA/dt = π*(2r)*(dr/dt)
= π*(24)*2
= 48π cm2/s
Rate of increase of area is 48π cm2/s (increasing as it is positive).

Test: Rate of Change And Approximations - Question 8
Save

Using approximation find the value of  ​

Detailed Solution: Question 8

Let x=4, Δx=0.01

y=x^½ = 2

y+Δy = (x+ Δx)^½ = (4.01)^½

Δy = (dy/dx) * Δx

Δy = (x^(-1/2))/2 * Δx

Δy = (½)*(½) * 0.01

Δy = 0.25 * 0.01

Δy = 0.0025

So, (4.01)^½ = 2 + 0.0025 = 2.0025

Test: Rate of Change And Approximations - Question 9
Save

Find the approximate value of f(10.01) where f(x) = 5x2 +6x + 3​

Detailed Solution: Question 9

f(x) = 5x2 +6x + 3
f(10.01) = 5*(10.01)2 + 6*(10.01) + 3
To find (10.01)2
Let p=10, Δp=0.01
y=p2 = 100
y+Δy = (p+ Δp)2 = (10.01)2
Δy = (dy/dp) * Δp
Δy = 2*p* Δx
Δy = 2*10* 0.01
Δy = 20 * 0.01
Δy = 0.2
So, (10.01)2 = y + Δy
= 100.2
So,
f(10.01) = 5*(100.2) + 6*(10.01) + 3
= 501 + 60.06 + 3
= 564.06

Test: Rate of Change And Approximations - Question 10
Save

Given a function y = f(x) . Let Δx be the very small change in the value of x , then the corresponding change in the value of y that is Δy is approximately given by

173 videos|510 docs|154 tests
Join Course
Information about Test: Rate of Change And Approximations Page
In this test you can find the Exam questions for Test: Rate of Change And Approximations solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Rate of Change And Approximations, EduRev gives you an ample number of Online tests for practice
Download as PDF
;

About this JEE Practice Test

This test contains 10 MCQs on Rate of Change And Approximations with detailed solutions for each question. It is part of the Mathematics (Maths) for JEE Main & Advanced course on EduRev, designed to align with the current JEE syllabus. Each solution explains not just the correct answer but also gives you an understanding of the topic — not just to attempt the question but also help you prepare for the exam with practice.

Explore more JEE Study Resources

If you found this Rate of Change And Approximations MCQ Test helpful, you can further enhance your JEE preparation with in-depth courses on EduRev, for all the subjects and topics available in Mathematics (Maths) for JEE Main & Advanced course. The course offers:
  • Video Lectures: covering all the topics of Mathematics (Maths) for JEE Main & Advanced
  • Notes for Revision: offering revision notes, important questions, summaries, flashcards, cheatsheets & more for JEE preparation
  • MCQ Test: variety of questions as per the pattern of JEE exam
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup