Test: Introduction To Conditional Probability - Commerce MCQ
10 Questions MCQ Test - Test: Introduction To Conditional Probability
If A and B are two events of sample space S, then
If E, F and G are events with P(G) ≠≠ 0 then P ((E ∪ F)|G) given by
If A, B and C are three events of sample space S, then
If A and B are two events such that P(A) ≠ O and P(A) ≠ 1, then
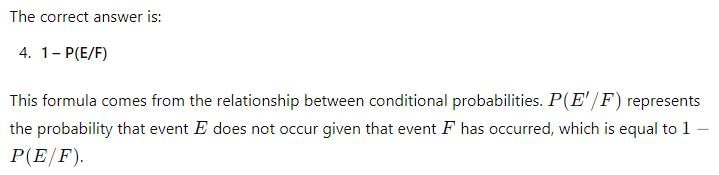
Let E and F be events of a sample space S of an experiment, then P(E’/F) = …
A fair six-sided die is rolled twice. What is the probability of getting 2 on the first roll and not getting 4 on the second roll?
In a box containing 100 Ipods, 10 are defective. The probability that out of a sample of 5 Ipods, exactly 1 is defective is:
Three coins are tossed. If at least two coins show head, the probability of getting one tail is:
If A and B are two events such that P(A) = 0.3 and P(B) = 0.9 and P(B|A) = 0.6,then P(A|B) = ……
A bag contains 25 tickets numbered from 1 to 25. Two tickets are drawn one after another without replacement. The probability that both tickets will show even numbers is: