Test: Mean And Variance Of A Random Variable - Commerce MCQ

Here, mean = [na+n(−a)]/2n=0
Hence variance = [na²+n(−a)²]2n − (mean)²
⇒ 2 = a² − (0)²
⇒ a² = 2

The sum of all the probabilities is equal to 1. 
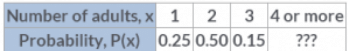
Therefore, the probability that four or more adults reside in a home = 1 - (0.25 + 0.50 + 0.15) 
= 0.10.

Here, X represents the number of sixes obtained when two dice are thrown simultaneously. Therefore, X can take the value of 0, 1, or 2.
∴ P(X=0)=P(not getting six on any of the dice) = 25/36
P(X=1)=P(sixo n first die and no six on second die) + P(no six on firstdie and six on second die)
=2(1/6 × 5/6)=10/36
P(X=2)=P(sixonboththedice)= 1/36
Therefore, the required probability distribution is as follows.
Then, expectation of X=E(X)=∑Xi
​P(Xi)= 0(25/36)+1(10/36)+2(1/36)
= 1/3 

 The sample space of the experiment is S = {1, 2, 3, 4, 5, 6}. 
 Let X denote the number obtained on the throw. 
Then X is a random variable which can take values X = 1, 2, 3, 4, 5, or 6.  Also P(1) = P(2) = P(3) = P(4) = P(5) = ⅙

Let X denotes the number of tails, then probability distribution is
X         0      1      2     3
P(X)    1/8  3/8  3/8  1/8
Mean (xipi) = 0(⅛) + 1(⅜) + 2(⅜) + 3(⅛)
= 3/2