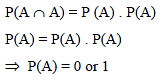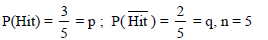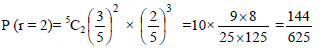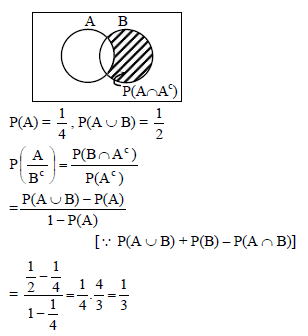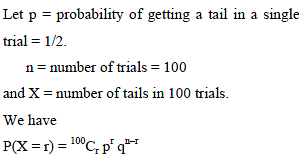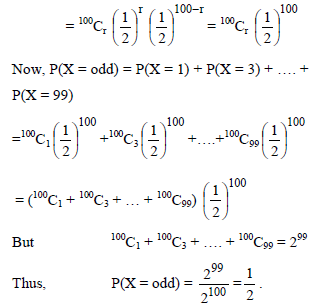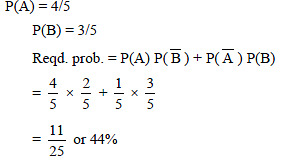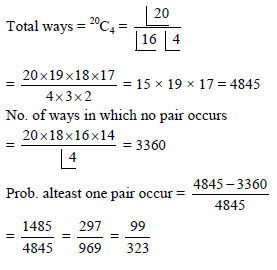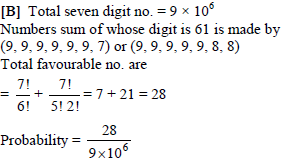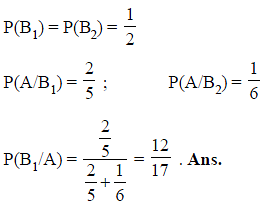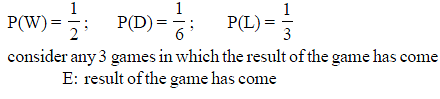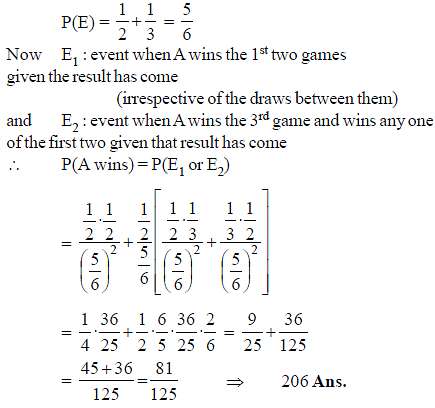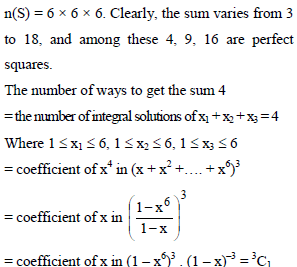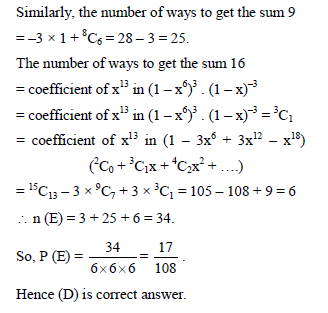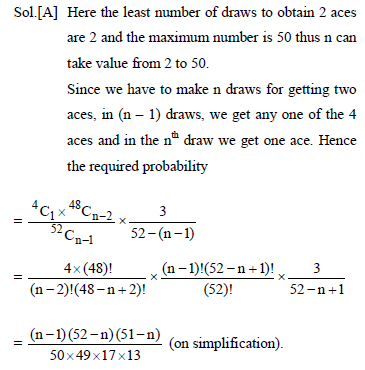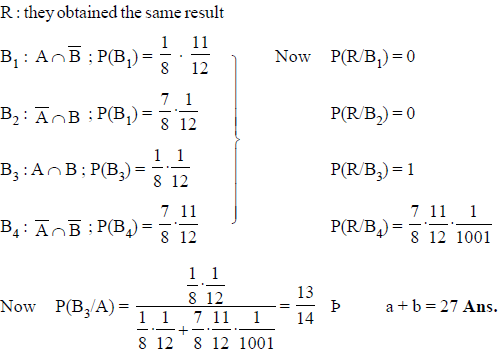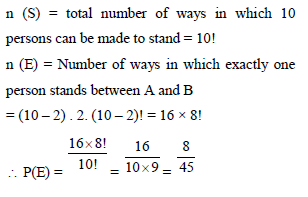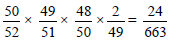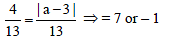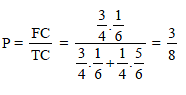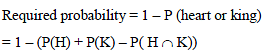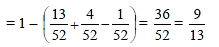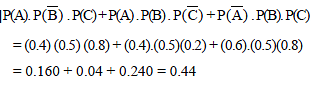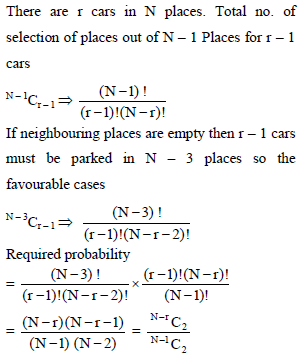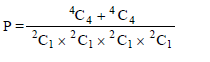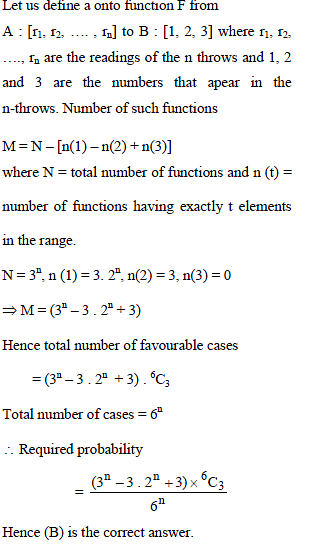Probability - 2 - JEE MCQ

Consider a set 'P' containing 'n' elements. A subset 'A' of 'P' is drawn and there after set 'P' is reconstructed. Now one more subset 'B' of 'P' is drawn. Probability of drawing sets A and B so that A ∩B has exactly one element –

A fair coin is tossed 100 times. The probability of getting tails an odd number of times is-

A speaks truth in 80% cases and B in 60% cases. In what percentage of case are they likely to contradict each other in starting the same fact : 

There are 10 pairs of shoes in a cupboard from which 4 shoes are picked at radndom. The probability that there is at least one pair is

Four numbers are multiplied together. Then the probability that the product will be divisible by 5 or 10 is -

Seven digit numbers are made from using all digits repetition of digits is allowed. Then the probability that the sum of digits of number is 61, is

A letter has either come from LONDON or CLIFTON, but on the post mark 2 consecutive letters 'ON' are found to be visible. The probability that the letter has come from LONDON is

A match between two players A and B is won by the player who first wins two games. A's chance of winning, drawing or losing any particular games are 1/2, 1/6 or 1/3 respectively. If the probability of A's winning the match can be expressed in the form p/q, find (p + q).

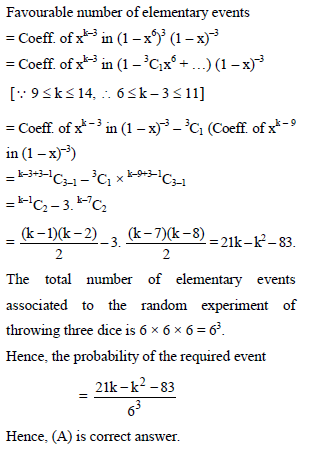
Three six-faced dice are thrown together. The probability that the sum of the numbers appearing on the dice is k (9 ≤ k ≤ 14), is -

Three dice are thrown. The probability of getting a sum which is a perfact square, is -

A, B are two inaccurate arithmeticians whose chance of solving a given question correctly are (1/8) and (1/12) respectively. They solve a problem and obtained the same result. If it is 1000 to 1 against their making the same mistake. If the chance that the result is correct is expressed as a/b , find the least value of (a + b).

There are 10 persons among whom are A and B who are made to stand in a row in random order. The probability that there is exactly one person between A and B is-

From a well shuffled pack of 52 playing cards, if cards are drawn one by one without replacement till the black ace comes, then probability that the black aces comes in the 4^th draw is -

A man is known to speak truth in 75% cases. He throws a dice & reports that it is a six. The probability that it is actually a six is -

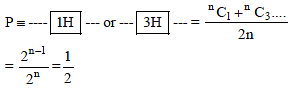
A coin is tossed ‘n’ times. The probability that head will turn up an odd no. of times is :

A card is drawn at random from a pack of 52 cards. The probability of drawing a card which is neither a heart nor a king is-

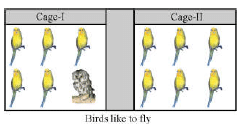
Shalu bought two cages of birds : Cage-I contains 5 parrots and 1 owl, and Cage-II contains 6 parrots, as shown.

One day Shalu forgot to lock both cages and two birds flew from Cage-I to Cage-II. Then two birds flew back from Cage-II to Cage-I. Assume that all birds have equal chance of flying, the probability that the Owl is still in Cage-I, is

Three rifle man take one shot each at the same target. The probability of the first rifle man hitting the target is 0.4, the probability of the 2^rd rifle man hitting target is 0.5 and the probability of third rifle man hitting the target is 0.8. Then the probability that exactly two of them hit the target is

A car is parked among N cars standing in a row, but not at either end. On his return, the owner finds that exactly 'r' of the N placed are still occupied. The probability that both the places neighbouring his car empty is -

From each of the four married couples, one of the partners is selected at random. The probability that those selected are of the same sex is-

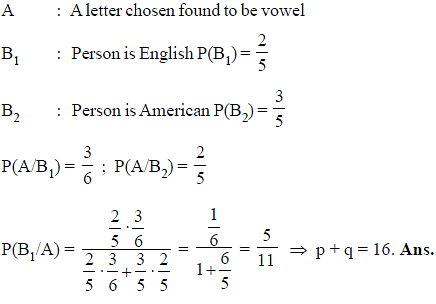
In an hotel International, 40% English and 60% Americans people are staying. English and American spellings for the same word are 'RIGOUR' and 'RIGOR', respectively. A randomly chosen man staying at a Nigerian hotel writes this word, and a letter taken at random from his spelling was found to be a vowel. Then the probability that the writer is an Englishman, can be expressed as (p/q), p, q ∈ N, find the least value of (p + q).

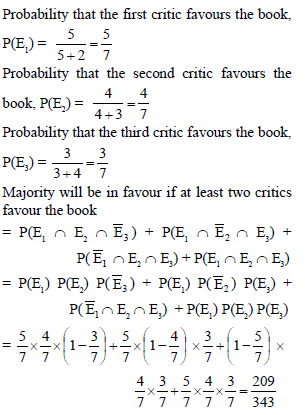
Three critics review a book. Odds in favour of the book are 5 : 2, 4 : 3 and 3 : 4 respectively for the three critics. The probability that majority are in favour of the book is

An unbiased die, with faces numbered 1, 2, 3, 4, 5, 6, is thrown n times and the list of n numbers shown up is noted. Then the probability that, among the numbers 1, 2, 3, 4, 5, 6, only three numbers appear in this list, is -

A permutation of 5 digits from the set {1, 2, 3, 4, 5} where each digit is used exactly once, is chosen randomly. Let p/q expressed as rational in lowest form be the probability that the chosen permutation changes from increasing to decreasing, or decreasing to increasing at most once e.g. the strings like 1 2 3 4 5, 5 4 3 2 1, 1 2 5 4 3 and 5 3 2 1 4 are acceptable but strings like 1 3 2 4 5 or 5 3 2 4 1 are not, find (p + q).