RRB NTPC Mathematics Test - 2 - RRB NTPC/ASM/CA/TA MCQ
30 Questions MCQ Test - RRB NTPC Mathematics Test - 2
The amount of simple interest on a deposit of Rs. 8,500 for 3 years is Rs. 2,040. Find the rate of interest per annum.
The following pie chart gives the percentages of the number of students studying in different streams in an engineering college. There are 5000 students in the college in all.
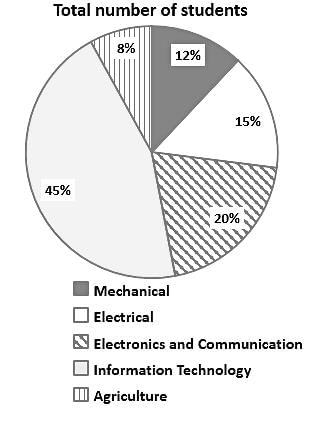
The college decided to give ₹300 per student to each stream for the purchase of teaching materials. How much will the Electronics and Communication stream get?

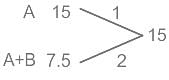
A can do a certain work in 15 days, while Working together, A and B can do the same work in 7.5 days. How many days will B alone take to do the same work?
Before 6 years, the ratio between the age of Radha and Ram is 5 : 8. The difference between the age of Ram and Radha is 6 years. After how many years, the ratio of their age will become 13 : 16?
The following bar graph shows the number of votes for favourite pizza toppings.
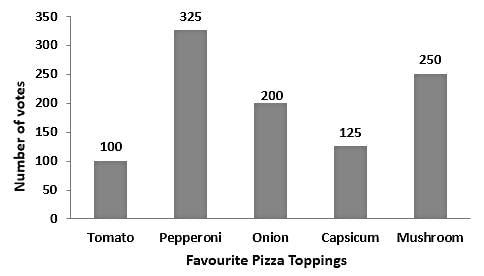
Of all the votes, what is the percentage of the votes for pepperoni?
Two trains of lengths 120 m and 180 m are traveling in opposite directions at the uniform speed of 34 km/h and 32 km/h. The time taken by the two trains to pass each other is:
The ratio of candidates passing and failing within the State.
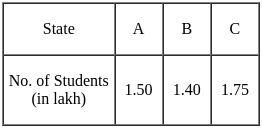
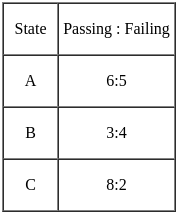
What is the number of passing students in State B?
Vishnu left place A at 10 am. for place B, and Sudeep left place B at 2 pm. for place A. The distance between the two places is 556 km. If Vishnu's speed is 42 km/h and Sudeep's speed is 55 km/h, then at what time will they meet each other?
Priya borrowed a certain sum at the rate of 12% per annum. If she paid Rs. 10176 at the end of 2 years as interest compounded annually. What was the sum she borrowed?
The radius of a wheel is 7 cm. If the wheel revolves from point A to B with a speed of 220 revolutions per minute and reaches B after 20 minutes, then find the distance between A and B.
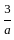 ?
?
A man buys 30 mangoes for a rupee (cost price of all mangoes is same). How many mangoes can be sold for a rupee (selling price of all mangoes is same) so that there is a loss of 25 percent?
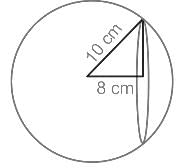
If a sphere of radius 10 cm is intersected by a plane at a distance 8 cm from its centre, what is the radius of the curve of intersection of the plane and the sphere?
Study the table given below and answer the question that follows.
The table shows the total number of employees in different departments of an organisation and percentage of females and males.
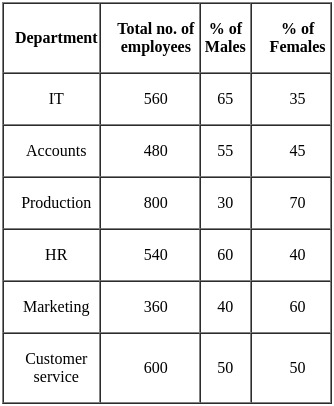
What is the total number of male employees in the accounts and marketing department together?
Suresh can do a work in 15 days. Suresh and Ramesh together do the same work in 10 days. If they are paid Rs. 1500 for the work, how should the money (in Rs.) be divided between them?
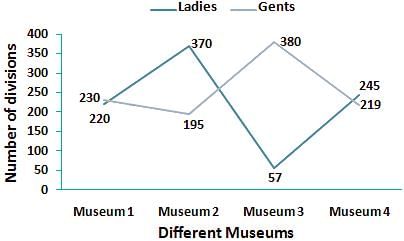
The graph shows number of visitors (ladies and gents) in four different museums namely 1, 2, 3 and 4. Which museum had the maximum number of visitors?
Shivani covered a certain distance in her vehicle. If she had travelled 6 km/h faster, she would have taken 30 minutes less. If she had travelled 5 km/h slower, she would have taken 30 minutes more. The distance is:
A bus travels at an average of 40 miles per hour for 2 hours and then travels at a speed of 60 miles per hour for 3 hours. How far did the bus travel in the entire 5 hours?
A person borrowed some money for three years at 8% simple interest. He lends the money at 5% compound interest for 6 six years. If he earns a total profit of Rs 5000, how much money did he borrow? (Given (1.05)6 = 1.34)



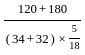 = 300 ×
= 300 × 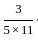 = 180/11 sec
= 180/11 sec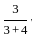
 ×140000
×140000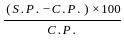 or
or 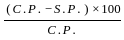
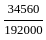 × 100 % = 18 %
× 100 % = 18 %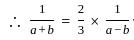
 then what is (x + y) equal to?
then what is (x + y) equal to?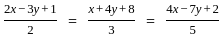
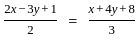
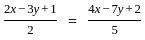
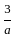
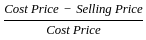 × 100
× 100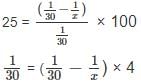
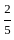 ∶
∶ 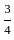 ∶
∶ 
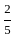 = 16, 40 ×
= 16, 40 × 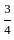 = 30, 40 ×
= 30, 40 × 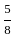 = 25
= 25
















