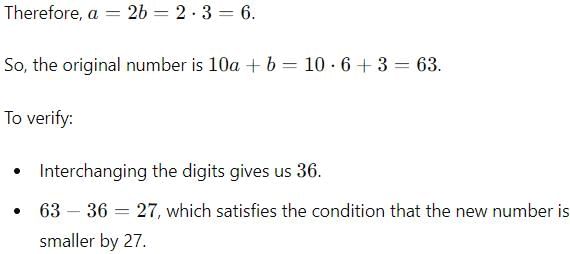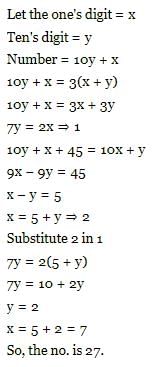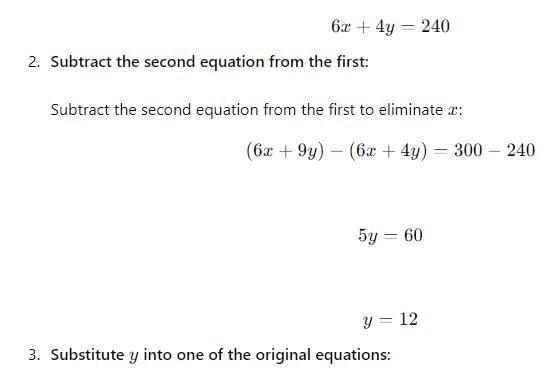Test: Number System- 1 - Railways MCQ
20 Questions MCQ Test - Test: Number System- 1
In a three digit number, the digit in the unit’s place is twice the digit in the ten’s place and 1.5 times the digit in the hundred’s place. If the sum of all the three digits of the number is 13, what is the number?
In a two digit positive number, the digit at the units place is equal to the square of the digit in ten’s place and the difference between the number and the number obtained by interchanging the digits is 54. What is 40% of the original number?
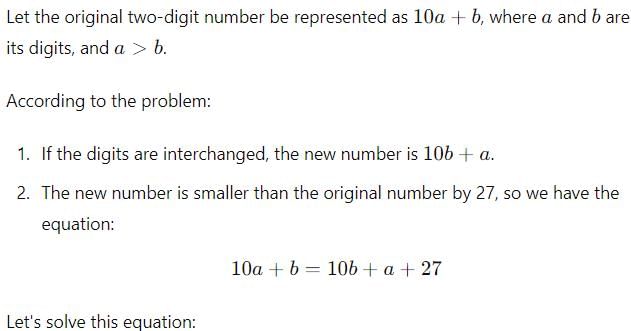
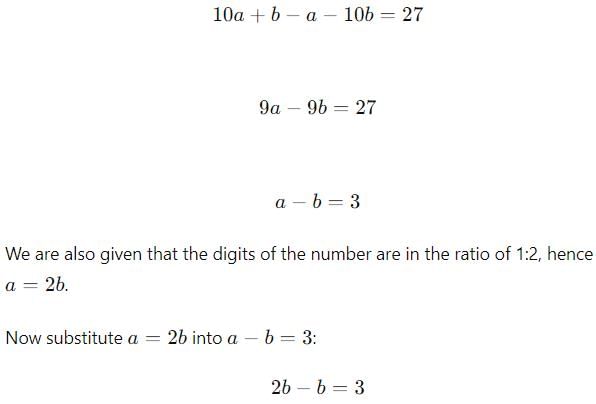
If the positions of the digits of a two digit number are interchanged, the number obtained is smaller than the original number by 27. If the digits of the number are in the ratio of 1:2, what is the original number?
A certain number of two digits is three times the sum of its digits. If 45 is added to it, the digits are reversed. The number is _______
The number obtained by interchanging the two digits of a two digit number is less than the original number by 27. If the difference between the two digits of the number is 3, then what is the original number?
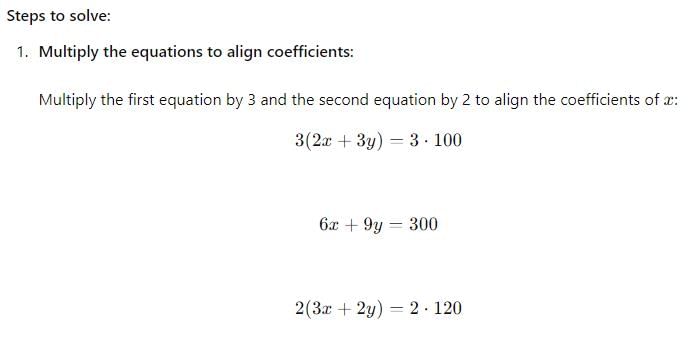
Two numbers such that the sum of twice the first number and thrice the second number is 100 and the sum of thrice the first number and twice the second number is 120. Which is larger number?
The ratio of the two numbers is 11 : 4 and the H.C.F is 16, then find the sum of the two numbers.
A number is divided by 2, 3, 4, 5 or 6, reminder in each case is one. But the number is exactly divisible by 7. The number lies between 250 and 350, the sum of digits of the number will be
Sum of three consecutive odd numbers & three consecutive even numbers together is 231. Difference between the smallest odd number and the smallest even number is 11. What is the sum of the largest even number and largest odd number?
Sum of eight consecutive odd numbers is 656. Average of four consecutive even numbers is 87. What is the sum of the largest even number and largest odd number?
The sum of the digits of a two-digit number is 6. If the digits are reversed, the number is decreased by 36. Find the number?
If the places of last two-digits of a three digit number are interchanged, a new number greater than the original number by 36 is obtained. What is the difference between the last two digits of that number?
A number when divided by 143 leaves 31 as the remainder. What will be the remainder when the same number is divided by 13?
The numerator of a rational number is 4 less than the denominator. If the numerator is increased by 15 and denominator is decreased by 4, we get 6. Find the rational number?
When a number is added to 20 percent of the second number, we get 150 percent of the second number. Find the ratio between the first and second number?
If two numbers are each divided by the same divisor, the remainders are respectively 3 and 4. If the sum of the two numbers be divided by the same divisor, the remainder is 2. The divisor is
A number gets reduced to its two-third when 24 is subtracted from it. Find oneeighth of the number?
Three numbers are in the ratio 4:3:5. If the difference between thrice the third number and the sum of first and second number is 64. Find the difference between the first and third number?
One-fifth of a number when subtracted from one – third of the number gives 24. Find the square of the number.
25% of a number is 2 times 65% of another number. Find the ratio of the second no to the first number?