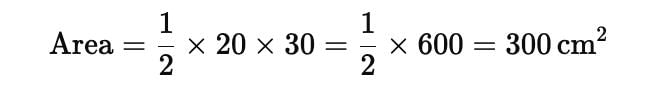All Exams >
Class 7 >
Mathematics (Maths) Class 7 >
All Questions
All questions of Perimeter and Area for Class 7 Exam
The base in the area of parallelogram is- a)area / height
- b)area × base
- c)area / base
- d)area × height
Correct answer is option 'A'. Can you explain this answer?
The base in the area of parallelogram is
a)
area / height
b)
area × base
c)
area / base
d)
area × height
|
|
Ananya Das answered |
Since area of parallelogram is base × height
So to find Base of parallelogram the formula is = Area of parallelogram / Height
So option A is the correct answer.
The height in the area of parallelogram is- a)area / base
- b)area / height
- c)area × base
- d)area × height
Correct answer is option 'A'. Can you explain this answer?
The height in the area of parallelogram is
a)
area / base
b)
area / height
c)
area × base
d)
area × height
|
|
Avantika Desai answered |
Since Area of parallelogram is base × height
So Height = Area of parallelogram / Base
So option A is the correct answer.
A door frame of dimensions 4m x 5m is fixed on the rectangular wall of dimensions 11m x 11m. Find the total labor charges for painting the wall if the labor charges for painting 1m2 of the wall is Rs 2.50.- a)Rs. 252.50
- b)Rs. 300
- c)Rs. 20
- d)Rs. 350
Correct answer is option 'A'. Can you explain this answer?
A door frame of dimensions 4m x 5m is fixed on the rectangular wall of dimensions 11m x 11m. Find the total labor charges for painting the wall if the labor charges for painting 1m2 of the wall is Rs 2.50.
a)
Rs. 252.50
b)
Rs. 300
c)
Rs. 20
d)
Rs. 350

|
Nagaveni Devar answered |
How
What is the circumference of a circle of diameter 10 cm?- a)35 cm
- b)30 cm
- c)31.4 cm
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
What is the circumference of a circle of diameter 10 cm?
a)
35 cm
b)
30 cm
c)
31.4 cm
d)
None of these

|
Tutorpedia Coaching answered |
The formula for the circumference of a circle is:
Circumference = π × diameter
Circumference = 3.14 ×10 = 31.4cm
If the ratio of circumferences of two circles is 4:9, what is the ratio of their areas?
- a)9:4
- b)16:81
- c)4:9
- d)2:3
Correct answer is option 'B'. Can you explain this answer?
If the ratio of circumferences of two circles is 4:9, what is the ratio of their areas?
a)
9:4
b)
16:81
c)
4:9
d)
2:3

|
Shiksha Academy answered |
2πr1/2πr2 = 4/9
r1/r2 = 4/9
πr12/πr22 = r12/r22 = 16/81
r1/r2 = 4/9
πr12/πr22 = r12/r22 = 16/81
The length and the breadth of a rectangular piece of land are 400 m and 250 m respectively. What is the cost of the land at Rs. 1000 per square metre?- a)Rs.10 lakhs
- b)Rs.1 crore
- c)Rs.10 crores
- d)Rs.10 thousands
Correct answer is option 'C'. Can you explain this answer?
The length and the breadth of a rectangular piece of land are 400 m and 250 m respectively. What is the cost of the land at Rs. 1000 per square metre?
a)
Rs.10 lakhs
b)
Rs.1 crore
c)
Rs.10 crores
d)
Rs.10 thousands

|
Tanishq Kulkarni answered |
∴ Area = 400 × 250m2
= 100000 m2
Cost of the land per square metre
= Rs.1000
∴ Cost of total land
100000 × Rs.1000 = Rs.10 crores
= 100000 m2
Cost of the land per square metre
= Rs.1000
∴ Cost of total land
100000 × Rs.1000 = Rs.10 crores
Find the perimeter of a triangle with sides 4 cm, 6 cm and 10 cm
- a)20cm
- b)24cm
- c)9cm
- d)18cm
Correct answer is option 'A'. Can you explain this answer?
Find the perimeter of a triangle with sides 4 cm, 6 cm and 10 cm
a)
20cm
b)
24cm
c)
9cm
d)
18cm

|
Jaya Mukherjee answered |
Perimeter = Sum of all the sides
Perimeter of a triangle = a+b+c
Here a = 4cm , b = 6cm , c =10cm
(6+4+5)cm=15cm
So option A is the correct answer.
How many plants (approximately) will be there in a circular bed whose outer edge measures 30 cm allowing 4 cm2 for each plant?- a)18
- b)750
- c)24
- d)120
Correct answer is option 'A'. Can you explain this answer?
How many plants (approximately) will be there in a circular bed whose outer edge measures 30 cm allowing 4 cm2 for each plant?
a)
18
b)
750
c)
24
d)
120

|
Srishti Reddy answered |
Circumference = 30 cm Area
= C2/4π = 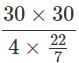 =71.6cm2
=71.6cm2
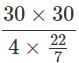 =71.6cm2
=71.6cm2Number of plants = A4 = 71.64 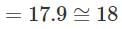
The difference between the circumference and radius of a circle is37 m. What is the circumference of the circle?- a)7m
- b)44m
- c)154m
- d)88m
Correct answer is option 'B'. Can you explain this answer?
The difference between the circumference and radius of a circle is37 m. What is the circumference of the circle?
a)
7m
b)
44m
c)
154m
d)
88m

|
Anmol Iyer answered |
2πr − r = 37
r = 37/2π−1 = 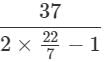 = 7m
= 7m
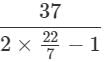 = 7m
= 7mCircumference = 2πr = 2 × 22/7 × 7 = 44m
A rectangular garden has a length of 12 meters and a width of 8 meters. What is the perimeter of the garden?- a)16 meters
- b)40 meters
- c)18 meters
- d)56 meters
Correct answer is option 'B'. Can you explain this answer?
A rectangular garden has a length of 12 meters and a width of 8 meters. What is the perimeter of the garden?
a)
16 meters
b)
40 meters
c)
18 meters
d)
56 meters

|
Wizius Careers answered |
Perimeter of a rectangle = 2 * (Length + Width)
Given: Length = 12 meters Width = 8 meters
Perimeter = 2 * (12 meters + 8 meters) Perimeter = 2 * (20 meters) Perimeter = 40 meters
So, the perimeter of the garden is 40 meters.
Which of the following has the formula : Base × Height- a)area of parallelogram
- b)area of square
- c)area of quadrilateral
- d)area of trapezium
Correct answer is option 'A'. Can you explain this answer?
Which of the following has the formula : Base × Height
a)
area of parallelogram
b)
area of square
c)
area of quadrilateral
d)
area of trapezium
|
|
Yashvi Singh answered |
Understanding the Area of a Parallelogram
The formula for calculating the area of a shape is crucial in geometry, especially for various polygons. One prominent formula is:
Area = Base × Height
This formula specifically applies to the area of a parallelogram. Here’s a detailed breakdown:
1. Definition of Parallelogram
- A parallelogram is a four-sided figure (quadrilateral) where opposite sides are parallel and equal in length.
2. Components of the Formula
- Base: The length of one side of the parallelogram, which is considered the bottom side.
- Height: The perpendicular distance from the base to the opposite side. It is important that height is measured at a right angle to the base.
3. Why Other Options Are Incorrect
- Area of Square: The area is calculated using the formula Side × Side.
- Area of Quadrilateral: There isn't a single formula for all quadrilaterals; the area can vary based on the shape.
- Area of Trapezium: The formula is (Base1 + Base2) × Height / 2, which is different from the parallelogram's formula.
Conclusion
Thus, the correct answer is option 'A' as the formula Base × Height accurately represents the area of a parallelogram. Understanding these distinctions helps in accurately calculating areas of different shapes in geometry.
The formula for calculating the area of a shape is crucial in geometry, especially for various polygons. One prominent formula is:
Area = Base × Height
This formula specifically applies to the area of a parallelogram. Here’s a detailed breakdown:
1. Definition of Parallelogram
- A parallelogram is a four-sided figure (quadrilateral) where opposite sides are parallel and equal in length.
2. Components of the Formula
- Base: The length of one side of the parallelogram, which is considered the bottom side.
- Height: The perpendicular distance from the base to the opposite side. It is important that height is measured at a right angle to the base.
3. Why Other Options Are Incorrect
- Area of Square: The area is calculated using the formula Side × Side.
- Area of Quadrilateral: There isn't a single formula for all quadrilaterals; the area can vary based on the shape.
- Area of Trapezium: The formula is (Base1 + Base2) × Height / 2, which is different from the parallelogram's formula.
Conclusion
Thus, the correct answer is option 'A' as the formula Base × Height accurately represents the area of a parallelogram. Understanding these distinctions helps in accurately calculating areas of different shapes in geometry.
A wire bent in the form of a circle of radius 42 cm is again bent in the form of a square. What is the ratio of the regions enclosed by the circle and the square?- a)11:12
- b)21:33
- c)22:33
- d)14:11
Correct answer is option 'D'. Can you explain this answer?
A wire bent in the form of a circle of radius 42 cm is again bent in the form of a square. What is the ratio of the regions enclosed by the circle and the square?
a)
11:12
b)
21:33
c)
22:33
d)
14:11

|
Prateek Sharma answered |
Length of wire = 2π × 42 = 84πcm
Let x be the side of the square. We have, 4x = 84π ⇒ x = 21π
Area of the circle: Area of the square
= π(42)2:(21π)2
= 4:π = 4:22/7 = 14:11
Let x be the side of the square. We have, 4x = 84π ⇒ x = 21π
Area of the circle: Area of the square
= π(42)2:(21π)2
= 4:π = 4:22/7 = 14:11
The ______ is the distance around a given two-dimensional object.- a)perimeter
- b)area
- c)volume
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
The ______ is the distance around a given two-dimensional object.
a)
perimeter
b)
area
c)
volume
d)
none of these

|
Ankit Jain answered |
The Concept of Perimeter
The perimeter is a fundamental concept in geometry that refers to the total distance around a two-dimensional shape. Understanding the perimeter is essential for various applications, including architecture, landscaping, and even in banking when calculating land or property boundaries.
Definition of Perimeter
- The perimeter is the sum of all the sides of a polygon.
- For regular shapes like squares and rectangles, specific formulas can be used to calculate the perimeter easily.
How to Calculate Perimeter
- For a rectangle:
- Perimeter = 2 * (length + width)
- For a square:
- Perimeter = 4 * side
- For a triangle:
- Perimeter = side1 + side2 + side3
- For a circle (circumference):
- Perimeter = 2 * π * radius
Importance of Perimeter
- Real-World Applications: Knowing the perimeter helps in planning and design, such as fencing a yard or laying out a garden.
- Measurement Skills: Understanding perimeter reinforces basic mathematical skills and promotes spatial awareness.
- Banking Relevance: In banking, accurate perimeter measurements can influence property valuations and investment decisions.
In summary, the perimeter is the total distance around a two-dimensional object, making option 'A' the correct answer. Understanding this concept is crucial across various fields, emphasizing its practical importance in both daily life and professional contexts.
The perimeter is a fundamental concept in geometry that refers to the total distance around a two-dimensional shape. Understanding the perimeter is essential for various applications, including architecture, landscaping, and even in banking when calculating land or property boundaries.
Definition of Perimeter
- The perimeter is the sum of all the sides of a polygon.
- For regular shapes like squares and rectangles, specific formulas can be used to calculate the perimeter easily.
How to Calculate Perimeter
- For a rectangle:
- Perimeter = 2 * (length + width)
- For a square:
- Perimeter = 4 * side
- For a triangle:
- Perimeter = side1 + side2 + side3
- For a circle (circumference):
- Perimeter = 2 * π * radius
Importance of Perimeter
- Real-World Applications: Knowing the perimeter helps in planning and design, such as fencing a yard or laying out a garden.
- Measurement Skills: Understanding perimeter reinforces basic mathematical skills and promotes spatial awareness.
- Banking Relevance: In banking, accurate perimeter measurements can influence property valuations and investment decisions.
In summary, the perimeter is the total distance around a two-dimensional object, making option 'A' the correct answer. Understanding this concept is crucial across various fields, emphasizing its practical importance in both daily life and professional contexts.
The area of triangle is- a)(1/2) × base × height
- b)(1/2)× (base + height)
- c)base ´ height
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
The area of triangle is
a)
(1/2) × base × height
b)
(1/2)× (base + height)
c)
base ´ height
d)
None of these

|
Shraddha Chopra answered |
Area of Triangle Calculation:
To calculate the area of a triangle, you can use the formula:
(1/2) × base × height
This formula is derived by multiplying the base of the triangle by the height of the triangle and then dividing the result by 2. This is because the area of a triangle is always half of the product of its base and height.
Example:
Let's say you have a triangle with a base of 6 units and a height of 4 units.
Using the formula: (1/2) × 6 × 4 = 12 square units.
Therefore, the area of the triangle is 12 square units.
Conclusion:
The correct formula for calculating the area of a triangle is:
(1/2) × base × height
This formula is widely used and accepted for determining the area of a triangle in various mathematical and practical applications.
If we cut a square along one of its diagonals, two triangles are obtained. Area of each triangle obtained = __________.- a)(1/2)×Area of the square
- b)(1/4)×Area of the square
- c)Area of the square
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
If we cut a square along one of its diagonals, two triangles are obtained. Area of each triangle obtained = __________.
a)
(1/2)×Area of the square
b)
(1/4)×Area of the square
c)
Area of the square
d)
None of these

|
Geetika Kulkarni answered |
The area of each triangle obtained by cutting a square along one of its diagonals is equal to half the area of the square.
So, the correct answer is:
a) (1/2)
So, the correct answer is:
a) (1/2)
The height in the area of a triangle- a)(2×area)/base
- b)base/(2×area)
- c)(2×area) / height
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
The height in the area of a triangle
a)
(2×area)/base
b)
base/(2×area)
c)
(2×area) / height
d)
None of these

|
Aspire Academy answered |
we know that:
area=(1/2) × base × height
so, height=(2×area)/base
so, height=(2×area)/base
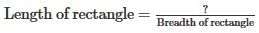
- a)breadth of rectangle
- b)Perimeter of rectangle
- c)Area of rectangle
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
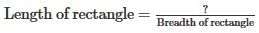
a)
breadth of rectangle
b)
Perimeter of rectangle
c)
Area of rectangle
d)
None of these
|
|
Shreya answered |
Becoz area of rectangle=length×breadth..so,if we have to find length of a rectangle then the equation is Length=Area/breadth (by cross-multiplication).....
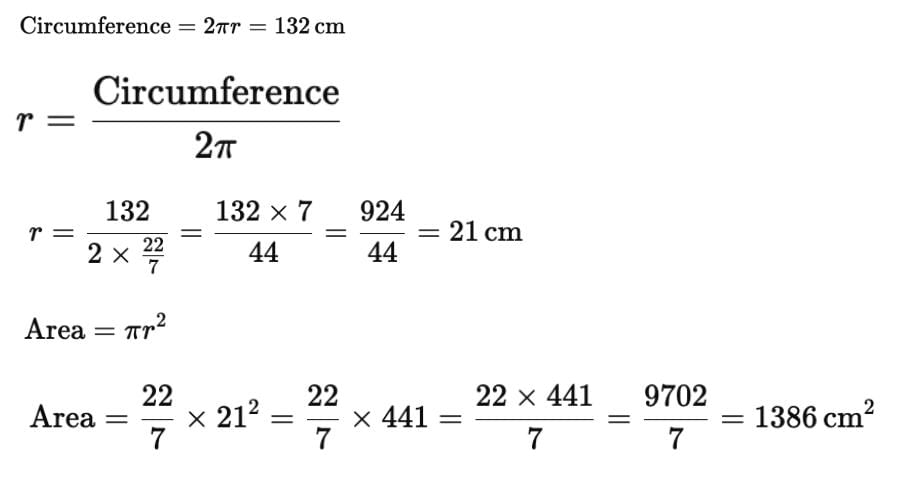
The circular grass lawn of radius 35 m has a path of width 7 m around it on the outside. What is the area of the path?- a)1496m2
- b)1450m2
- c)1576m2
- d)1694m2
Correct answer is option 'D'. Can you explain this answer?
The circular grass lawn of radius 35 m has a path of width 7 m around it on the outside. What is the area of the path?
a)
1496m2
b)
1450m2
c)
1576m2
d)
1694m2

|
Indu Gupta answered |
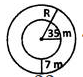
r = 35 m; R = 35+7=42 m
Formula for area of a circle:
Area = πr²
Area of inner circle:
Area = 22/7 × 35²
35² = 1225
Area = 22/7 × 1225 = 22 × 175 = 3850 m²
Area of outer circle:
Area = 22/7 × 42²
42² = 1764
Area = 22/7 × 1764 = 22 × 252 = 5544 m²
Area of path = Area of outer circle -Area of inner circle
Area of path = 5544 − 3850 = 1694m2
If the area of a circle is 2464 m2, find its diameter,- a)56m
- b)154m
- c)176m
- d)206m
Correct answer is option 'A'. Can you explain this answer?
If the area of a circle is 2464 m2, find its diameter,
a)
56m
b)
154m
c)
176m
d)
206m

|
Tutorpedia Coaching answered |
To find the diameter of a circle with an area of 2464 m2:
We start with the formula for the area of a circle:
- Area = π r2, where r is the radius.
- We can express the area in terms of diameter d: Area = π (d/2)2.
Given the area:
- Area = 2464 m2
- Using the formula: π (d/2)2 = 2464
Substituting π with 22/7:
- 22/7 * (d/2)2 = 2464
- Multiply both sides by 4: d2 = 2464 * 4 * 7 / 22
Calculating:
- d2 = 2464 * 4 * 7 / 22
- After calculating, we find d = 56 m.
Thus, the diameter of the circle is 56 m.
Find the area of following traingle: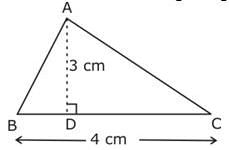
- a)6 cm2
- b)5 cm2
- c)4 cm2
- d)3 cm2
Correct answer is option 'A'. Can you explain this answer?
Find the area of following traingle:

a)
6 cm2
b)
5 cm2
c)
4 cm2
d)
3 cm2
|
|
Rohan Mehta answered |
Area of triangle= ½ *base*height
=½*4*3=6cm2
=½*4*3=6cm2
In the given figure, ABCD is a parallelogram. DL⊥AB and DM⊥BC. If AB = 18cm,BC = 12cm and DM = 10cm,find DL
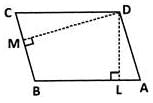
- a)
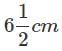
- b)6cm
- c)
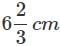
- d)
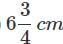
Correct answer is option 'C'. Can you explain this answer?
In the given figure, ABCD is a parallelogram. DL⊥AB and DM⊥BC. If AB = 18cm,BC = 12cm and DM = 10cm,find DL


a)
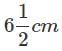
b)
6cm
c)
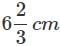
d)
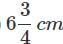

|
Anmol Iyer answered |
Here we equate the areas, i.e., AB×DL = BC×DM
⇒ 18×DL = 12×10
⇒ DL = (12×10)/18 = 20/3 =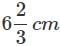
⇒ DL = (12×10)/18 = 20/3 =
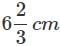
The distance around a circular region is known as its_______.- a)Circumference
- b)Area
- c)Volume
- d)None of the above
Correct answer is option 'A'. Can you explain this answer?
The distance around a circular region is known as its_______.
a)
Circumference
b)
Area
c)
Volume
d)
None of the above

|
Praveen Kumar answered |
- The distance around a circular region is known as its circumference.
- Circumference is the term used to describe the perimeter of a circle.
- Circumference is the term used to describe the perimeter of a circle.
The length and breadth of a rectangular hall in a model are 0.4 m and 30 cm respectively. What is the distance between the diagonally opposite corners of the wall in the model?- a)34.16 m
- b)50 m
- c)34.16 cm
- d)50 cm
Correct answer is option 'D'. Can you explain this answer?
The length and breadth of a rectangular hall in a model are 0.4 m and 30 cm respectively. What is the distance between the diagonally opposite corners of the wall in the model?
a)
34.16 m
b)
50 m
c)
34.16 cm
d)
50 cm

|
Rainbow Rise Classes answered |
Distance between two opposite corners
= 
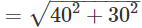 = 50 cm
= 50 cm

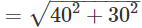 = 50 cm
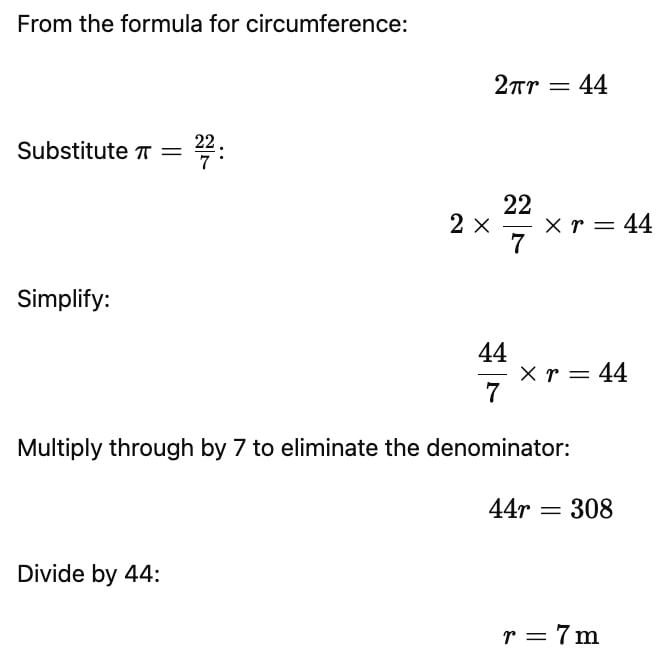
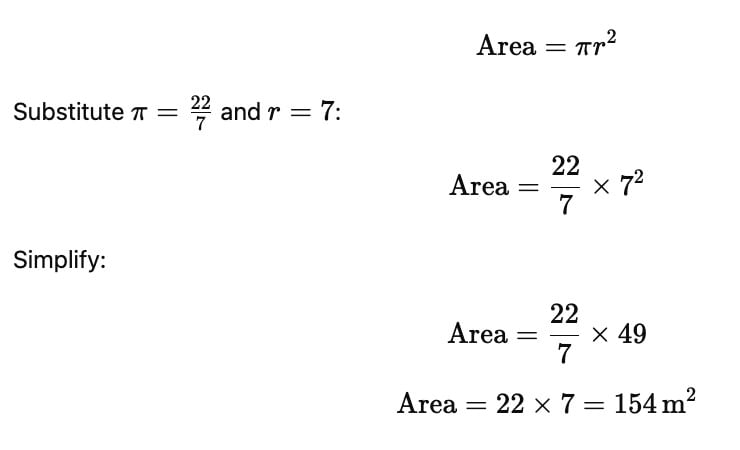
= 50 cmThe circumference of a circle is 44 m. Find the area of the circle?- a)6084.5 m2
- b)276.5 m2
- c)154 m2
- d)44 m2
Correct answer is option 'C'. Can you explain this answer?
The circumference of a circle is 44 m. Find the area of the circle?
a)
6084.5 m2
b)
276.5 m2
c)
154 m2
d)
44 m2

|
Rainbow Rise Classes answered |
Given:
- Circumference of the circle: C=44 m
- Formula for circumference: C = 2 π r
Find the area of a triangle with a base of 10 cm and a height of 30 cm- a)150
- b)100
- c)400
- d)600
Correct answer is option 'A'. Can you explain this answer?
Find the area of a triangle with a base of 10 cm and a height of 30 cm
a)
150
b)
100
c)
400
d)
600

|
Praveen Kumar answered |
We know Area of a triangle is bxh/2
where b is base and h is height
Given that base is 10 cm and height is 30 cm
Area of a triangle 10 cm x 30 cm / 2
= 300 cm2/2
= 150 cm2
So option A is the correct answer.
What is the circumference of a circle of diameter 10 cm?- a)35 cm
- b)30 cm
- c)31.4 cm
- d)None of these
Correct answer is option 'C'. Can you explain this answer?
What is the circumference of a circle of diameter 10 cm?
a)
35 cm
b)
30 cm
c)
31.4 cm
d)
None of these

|
Learning Education answered |
use the formula of circumference: 2πr
given: diameter=2r=10cm
so, circumference 2πr=(3.14)10=31.4 cm
A wall hanging is in the shape given in the figure. Find its perimeter. 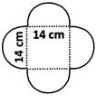
- a)176 cm
- b)146 cm
- c)44cm
- d)88cm
Correct answer is option 'D'. Can you explain this answer?
A wall hanging is in the shape given in the figure. Find its perimeter.

a)
176 cm
b)
146 cm
c)
44cm
d)
88cm

|
Abhay Menon answered |
The perimeter of the wall hanging is given by the sum of circumferences of the 4 semicircles −4 x diameter. Clearly, the diameter of each semicircle is 14 cm. The required perimeter
= 2 × circumference of circle of radius 7 cm.
= 2×2 × 22/7 × 7 = 88cm
In the following figure, ABCD is a parallelogram. DL⊥AB and AB =13 cm = AD . If the area of parallelogram is 156 cm2, find AL. 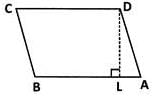
- a)5cm
- b)6cm
- c)7cm
- d)8cm
Correct answer is option 'A'. Can you explain this answer?
In the following figure, ABCD is a parallelogram. DL⊥AB and AB =13 cm = AD . If the area of parallelogram is 156 cm2, find AL.

a)
5cm
b)
6cm
c)
7cm
d)
8cm

|
Abhay Menon answered |
We have, area =156 cm2
⇒ b×h = 156
AB×DL=156
DL = 156/AB =156/13 = 12cm
In right ΔADL, AD2=DL2+LA2
AL2=AD2−DL2=132−122 = 25
∴ AL =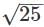 =5cm
=5cm
⇒ b×h = 156
AB×DL=156
DL = 156/AB =156/13 = 12cm
In right ΔADL, AD2=DL2+LA2
AL2=AD2−DL2=132−122 = 25
∴ AL =
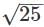 =5cm
=5cmFind the breadth of a rectangular plot of land, if its area is 440 m2 and the length is 22 m.- a)20 m
- b)5 m
- c)15 m
- d)10 m
Correct answer is option 'A'. Can you explain this answer?
Find the breadth of a rectangular plot of land, if its area is 440 m2 and the length is 22 m.
a)
20 m
b)
5 m
c)
15 m
d)
10 m

|
Wizius Careers answered |
The formula for the area of a rectangle is given by:
Area=Length×Breadth
In this case, the area is given as 440 m² and the length is given as 22 m. Let's denote the breadth as B. The formula can be rearranged to solve for the breadth:
Breadth=Area/Length
Substitute the given values:
Breadth=440 m2/22 m
Breadth=20 m
Chapter doubts & questions for Perimeter and Area - Mathematics (Maths) Class 7 2025 is part of Class 7 exam preparation. The chapters have been prepared according to the Class 7 exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Class 7 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Perimeter and Area - Mathematics (Maths) Class 7 in English & Hindi are available as part of Class 7 exam.
Download more important topics, notes, lectures and mock test series for Class 7 Exam by signing up for free.
Mathematics (Maths) Class 7
77 videos|388 docs|39 tests
|
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Forgot Password
OR
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily