All Exams >
ACT >
Mathematics for ACT >
All Questions
All questions of Plane Geometry for ACT Exam
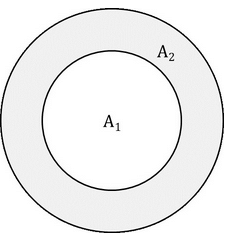 In the given figure, the area of the smaller circle is A1 and the area of the bigger circle is (A1 + A2). If the radius of the smaller circle is 6cm and A2 = 3A1, find the radius of the bigger circle.
In the given figure, the area of the smaller circle is A1 and the area of the bigger circle is (A1 + A2). If the radius of the smaller circle is 6cm and A2 = 3A1, find the radius of the bigger circle.- a)7 cm
- b)6√2 cm
- c)6√3 cm
- d)12 cm
- e)12√2 cm
Correct answer is option 'D'. Can you explain this answer?
In the given figure, the area of the smaller circle is A1 and the area of the bigger circle is (A1 + A2). If the radius of the smaller circle is 6cm and A2 = 3A1, find the radius of the bigger circle.
a)
7 cm
b)
6√2 cm
c)
6√3 cm
d)
12 cm
e)
12√2 cm
|
|
Aditya Kumar answered |
Let, Radius of smaller circle = r
and, Radius of Bigger circle = R
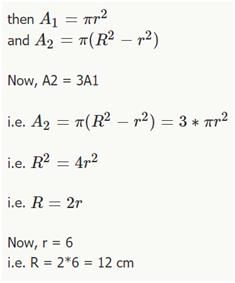
and, Radius of Bigger circle = R
The complement angle of 80° is- a)18 / π radian
- b)5π / 9 radian
- c)π / 18 radian
- d)9 / 5π radian
Correct answer is option 'C'. Can you explain this answer?
The complement angle of 80° is
a)
18 / π radian
b)
5π / 9 radian
c)
π / 18 radian
d)
9 / 5π radian
|
|
Rahul Kapoor answered |
Complementary angles: Complementary angles are angle pairs whose measures sum to one right angle (90°).
So, the required angle will be 10°
180° = π radian
1º = (π / 180)
∴ 10º = {(π × 10) / 180} = (π / 18)
Hence, option C is correct.
180° = π radian
1º = (π / 180)
∴ 10º = {(π × 10) / 180} = (π / 18)
Hence, option C is correct.
In triangle PQR length of the side QR is less than twice the length of the side PQ by 2 cm. Length of the side PR exceeds the length of the side PQ by 10 cm. The perimeter is 40 cm. The length of the smallest side of the triangle PQR is :- a)6 cm
- b)8 cm
- c)7 cm
- d)10 cm
- e)9 cm
Correct answer is option 'B'. Can you explain this answer?
In triangle PQR length of the side QR is less than twice the length of the side PQ by 2 cm. Length of the side PR exceeds the length of the side PQ by 10 cm. The perimeter is 40 cm. The length of the smallest side of the triangle PQR is :
a)
6 cm
b)
8 cm
c)
7 cm
d)
10 cm
e)
9 cm

|
Nandita Yadav answered |
In Δ PQR,
QR +2 = 2PQ
QR = 2PQ - 2 ------- (2)
PR = PQ + 10 ----- (2)
Perimeter = 40 m
PQ + QR + Rp = 40
Putting the value of PQ and QR from equation (1) and (2),
Pq + 2PQ - 2 + PQ +10 = 40
4PQ = 32
PQ = 8 cm which is the smallest side of the triangle.
QR +2 = 2PQ
QR = 2PQ - 2 ------- (2)
PR = PQ + 10 ----- (2)
Perimeter = 40 m
PQ + QR + Rp = 40
Putting the value of PQ and QR from equation (1) and (2),
Pq + 2PQ - 2 + PQ +10 = 40
4PQ = 32
PQ = 8 cm which is the smallest side of the triangle.
The point of intersection of the altitudes of a triangle is called its:- a)Incentre
- b)Excentre
- c)Orthocentre
- d)Centroid
- e)none of these
Correct answer is option 'C'. Can you explain this answer?
The point of intersection of the altitudes of a triangle is called its:
a)
Incentre
b)
Excentre
c)
Orthocentre
d)
Centroid
e)
none of these
|
|
Alok Verma answered |
The three (possibly extended) altitudes intersect in a single point, called the orthocenter of the triangle, usually denoted by H. The orthocenter lies inside the triangle if and only if the triangle is acute (i.e. does not have an angle greater than or equal to a right angle).
In a triangle ABC, the internal bisector of the angle A meets BC at D. If AB = 4, AC = 3 and ∠ A = 600, then length of AD is :- a)2√3
- b)(12√3) / 7
- c)(15√3) / 8
- d)(6√3) / 7
- e)None of these
Correct answer is option 'B'. Can you explain this answer?
In a triangle ABC, the internal bisector of the angle A meets BC at D. If AB = 4, AC = 3 and ∠ A = 600, then length of AD is :
a)
2√3
b)
(12√3) / 7
c)
(15√3) / 8
d)
(6√3) / 7
e)
None of these
|
|
Lavanya Menon answered |

Let BC = x and Ad = y, then as per bisector theorem,
BD /DC = AB /AC = 4 /3
Hence, BD = 4x/7 and DC = 3x/7
Now, in Δ ABD using cosine rule,
cos 300 = [{42 +y2 - (16x2/49)} /2*3*y]
Or, 4√3y =[{16 +y2 - (16x2/49)}] --------- (i)
Similarly in Δ ADC,
cos 300 = [{32 +y2 - (9x2/49)} /2*3*y]
Or, 3√3y =[{9 +y2 - (9x2/49)}] --------- (ii)
From equation (i) and (ii), we get
y = 12√3 /7.
In the figure given below, ABC is a triangle. BC is parallel to AE. If BC = AC, then what is the value of ∠CAE?
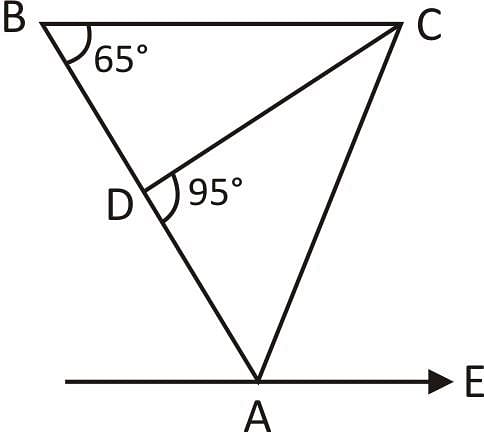
- a)20°
- b)30°
- c)40°
- d)50°
Correct answer is option 'D'. Can you explain this answer?
In the figure given below, ABC is a triangle. BC is parallel to AE. If BC = AC, then what is the value of ∠CAE?


a)
20°
b)
30°
c)
40°
d)
50°

|
Pioneer Academy answered |
Given that, BC || AE
∠CBA + ∠EAB = 180°
⇒ ∠EAB = 180° – 65° = 115°
∵ BC = AC
Hence, ΔABC is an isosceles triangle.
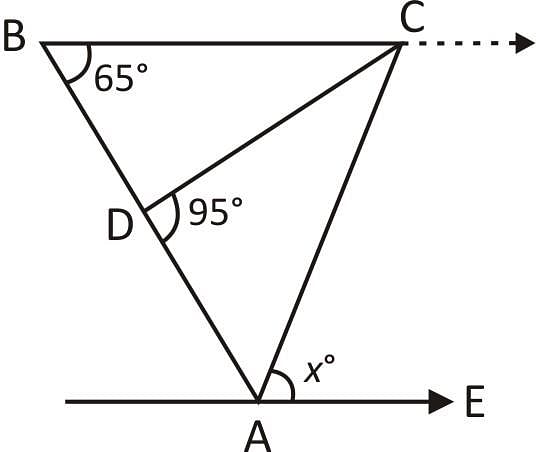
⇒ ∠CBA = ∠CAB = 65°
Now, ∠EAB = ∠EAC + ∠CAB
⇒ 115° = x + 65° ⇒ x = 50°.
Hence, option D is correct.
∠CBA + ∠EAB = 180°
⇒ ∠EAB = 180° – 65° = 115°
∵ BC = AC
Hence, ΔABC is an isosceles triangle.

⇒ ∠CBA = ∠CAB = 65°
Now, ∠EAB = ∠EAC + ∠CAB
⇒ 115° = x + 65° ⇒ x = 50°.
Hence, option D is correct.
If one arm of an angle is respectively parallel to the arm of another angle formed with a common line between them, then the two angles are- a)Neither equal nor supplementary
- b)not equal but supplementary
- c)equal but not supplementary
- d)Either equal or supplementary
Correct answer is option 'B'. Can you explain this answer?
If one arm of an angle is respectively parallel to the arm of another angle formed with a common line between them, then the two angles are
a)
Neither equal nor supplementary
b)
not equal but supplementary
c)
equal but not supplementary
d)
Either equal or supplementary

|
Mihir Nambiar answered |
Explanation:
When two angles have one arm parallel to the arm of another angle, and they are formed with a common line between them, they are called corresponding angles. In this case, the two angles are not equal, but they are supplementary.
Corresponding Angles:
When two lines are intersected by a transversal, the angles formed on the same side of the transversal and in the same relative position are called corresponding angles.
Parallel Lines:
Parallel lines are lines that are always the same distance apart and never intersect. When two lines are parallel, the corresponding angles formed by the transversal are equal.
Supplementary Angles:
Supplementary angles are two angles that add up to 180 degrees. In other words, if the sum of two angles is 180 degrees, they are supplementary.
Explanation of the Answer:
In this question, it is given that one arm of an angle is parallel to the arm of another angle formed with a common line between them. This means that the corresponding angles formed by the transversal are equal. However, since the two angles are not equal, they cannot be congruent.
The angles are formed on the same side of the transversal and in the same relative position, so they are corresponding angles. Since corresponding angles are equal, the two angles are not equal but supplementary. This means that the sum of the two angles is 180 degrees.
Therefore, the correct answer is option B: the two angles are not equal but supplementary.
When two angles have one arm parallel to the arm of another angle, and they are formed with a common line between them, they are called corresponding angles. In this case, the two angles are not equal, but they are supplementary.
Corresponding Angles:
When two lines are intersected by a transversal, the angles formed on the same side of the transversal and in the same relative position are called corresponding angles.
Parallel Lines:
Parallel lines are lines that are always the same distance apart and never intersect. When two lines are parallel, the corresponding angles formed by the transversal are equal.
Supplementary Angles:
Supplementary angles are two angles that add up to 180 degrees. In other words, if the sum of two angles is 180 degrees, they are supplementary.
Explanation of the Answer:
In this question, it is given that one arm of an angle is parallel to the arm of another angle formed with a common line between them. This means that the corresponding angles formed by the transversal are equal. However, since the two angles are not equal, they cannot be congruent.
The angles are formed on the same side of the transversal and in the same relative position, so they are corresponding angles. Since corresponding angles are equal, the two angles are not equal but supplementary. This means that the sum of the two angles is 180 degrees.
Therefore, the correct answer is option B: the two angles are not equal but supplementary.
ABC is a triangle in which AB = 4 cm and BC = 7cm. What is the possible range of values of CA?- a)-3< CA < 3
- b)-3< CA <11
- c)3< CA<11
- d)5<CA<12
- e)5<CA<17
Correct answer is option 'C'. Can you explain this answer?
ABC is a triangle in which AB = 4 cm and BC = 7cm. What is the possible range of values of CA?
a)
-3< CA < 3
b)
-3< CA <11
c)
3< CA<11
d)
5<CA<12
e)
5<CA<17
|
|
Lavanya Menon answered |
Possible range of values of CA will be |AB - BC| < CA < |AB + BC|
So possible range of values of CA will be 3 < CA <11
In the given figure AB || CD, ∠A = 128°, ∠E = 144°. Then, ∠FCD is equal to :

- a)72°
- b)64°
- c)136°
- d)92°
Correct answer is option 'D'. Can you explain this answer?
In the given figure AB || CD, ∠A = 128°, ∠E = 144°. Then, ∠FCD is equal to :


a)
72°
b)
64°
c)
136°
d)
92°

|
Pioneer Academy answered |
As per the given figure,
Through E draw EE’ || AB || CD.
Then, ∠AEE’ = 180° ‒ ∠BAE = (180° ‒ 128°) = 52°.
(Interior angles on the same side of the transversal are supplementary.)
Now, ∠E’EC = (144° ‒ 52°) = 92°.
∠FCD = ∠E’EC = 92° (Corr. ∠s).
Hence, option D is correct.
Through E draw EE’ || AB || CD.
Then, ∠AEE’ = 180° ‒ ∠BAE = (180° ‒ 128°) = 52°.
(Interior angles on the same side of the transversal are supplementary.)
Now, ∠E’EC = (144° ‒ 52°) = 92°.
∠FCD = ∠E’EC = 92° (Corr. ∠s).
Hence, option D is correct.
If two supplementary angles differ by 44°, then one of the angle is:- a)72°
- b)102°
- c)65°
- d)68°
Correct answer is option 'D'. Can you explain this answer?
If two supplementary angles differ by 44°, then one of the angle is:
a)
72°
b)
102°
c)
65°
d)
68°

|
Hridoy Desai answered |
If two supplementary angles differ by 44 degrees, we can set up the equation:
x + (x + 44) = 180
Simplifying this equation, we have:
2x + 44 = 180
Subtracting 44 from both sides, we get:
2x = 136
Dividing both sides by 2, we find:
x = 68
So, one angle is 68 degrees, and the other angle (which differs by 44 degrees) is 112 degrees.
x + (x + 44) = 180
Simplifying this equation, we have:
2x + 44 = 180
Subtracting 44 from both sides, we get:
2x = 136
Dividing both sides by 2, we find:
x = 68
So, one angle is 68 degrees, and the other angle (which differs by 44 degrees) is 112 degrees.
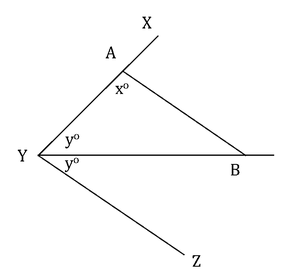 If yo = 45o, is xo = 90o?I. AB is parallel to YZII. AB = AY
If yo = 45o, is xo = 90o?I. AB is parallel to YZII. AB = AY- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficien
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'D'. Can you explain this answer?
If yo = 45o, is xo = 90o?
I. AB is parallel to YZ
II. AB = AY
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficien
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.

|
Rhea Gupta answered |
Steps 1 & 2: Understand Question and Draw Inferences
In ΔABY,
xo + yo + Angle ABY = 180o
- xo + 45o + Angle ABY = 180o
- xo + Angle ABY = 135o
We need to find if x is a right angle or not, i.e. if x = 90o.
For this we need the value of Angle ABY.
Step 3: Analyze Statement 1
Given that AB is parallel to YZ.
- xo + 2yo = 180 (Since both of them are consecutive interior angles).
Given that y = 45o
- x = 90o
Therefore, statement 1 is sufficient to arrive at a unique answer.
Step 4: Analyze Statement 2
Given AB = AY.
Therefore, Angle ABY = yo = 45o (given)
Therefore, xo + 45o = 135o
- x = 90o
Therefore, statement 2 is sufficient to arrive at a unique answer.
Step 5: Analyze Both Statements Together (if needed)
Since we arrived at a unique answer in at least one of step 3 and step 4, this step is not necessary.
Correct Answer: D
What is the area of an obtuse angled triangle whose two sides are 8 and 12 and the angle included between two sides is 150o?- a)24 sq units
- b)48 sq units
- c)24√3
- d)48√3
- e)Such a triangle does not exist
Correct answer is option 'A'. Can you explain this answer?
What is the area of an obtuse angled triangle whose two sides are 8 and 12 and the angle included between two sides is 150o?
a)
24 sq units
b)
48 sq units
c)
24√3
d)
48√3
e)
Such a triangle does not exist

|
Navya Yadav answered |
If two sides of a triangle and the included angle 'y' is known, then the area of the triangle = 1/2 * (Product of sides) * sin y
Substituting the values in the formula, we get 1/2 * 8 * 12 * sin 150o
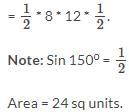
Substituting the values in the formula, we get 1/2 * 8 * 12 * sin 150o
A wheel of a car of radius 21 cms is rotating at 600 RPM. What is the speed of the car in km/hr?- a)79.2 km/hr
- b)47.52 km/hr
- c)7.92 km/hr
- d)39.6 km/hr
- e)3.96 km/hr
Correct answer is option 'B'. Can you explain this answer?
A wheel of a car of radius 21 cms is rotating at 600 RPM. What is the speed of the car in km/hr?
a)
79.2 km/hr
b)
47.52 km/hr
c)
7.92 km/hr
d)
39.6 km/hr
e)
3.96 km/hr

|
Janani Sharma answered |
The radius of the wheel measures 21 cm.
In one rotation, the wheel will cover a distance which is equal to the circumference of the wheel.
∴ in one rotation this wheel will cover 2 * π * 21 = 132 cm.
In one rotation, the wheel will cover a distance which is equal to the circumference of the wheel.
∴ in one rotation this wheel will cover 2 * π * 21 = 132 cm.
In a minute, the distance covered by the wheel = circumference of the wheel * rpm
∴ this wheel will cover a distance of 132 * 600 = 79200 cm in a minute.
∴ this wheel will cover a distance of 132 * 600 = 79200 cm in a minute.
In an hour, the wheel will cover a distance of 79200 * 60 = 4752000 cm.
Therefore, the speed of the car = 4752000 cm/hr = 47.52 km/hr
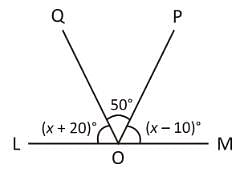
In the figure given above LOM is a straight line. What is the value of x°?- a)45°
- b)60°
- c)70°
- d)80°
Correct answer is option 'B'. Can you explain this answer?

In the figure given above LOM is a straight line. What is the value of x°?
a)
45°
b)
60°
c)
70°
d)
80°

|
Pioneer Academy answered |
From the given figure,
∠LOQ + ∠QOP + ∠POM = 180°
(straight line)
∴ (x° + 20°) + 50° + (x° – 10°) = 180°
⇒ 2x° + 60° = 180° ⇒ 2x° = 120°
∴ x° = 60°
Hence, optuion B is correct.
∠LOQ + ∠QOP + ∠POM = 180°
(straight line)
∴ (x° + 20°) + 50° + (x° – 10°) = 180°
⇒ 2x° + 60° = 180° ⇒ 2x° = 120°
∴ x° = 60°
Hence, optuion B is correct.
If the sum of the interior angles of a regular polygon measures 1440o, how many sides does the polygon have- a)10 sides
- b)8 sides
- c)12 sides
- d)9 sides
- e)None of these
Correct answer is option 'A'. Can you explain this answer?
If the sum of the interior angles of a regular polygon measures 1440o, how many sides does the polygon have
a)
10 sides
b)
8 sides
c)
12 sides
d)
9 sides
e)
None of these

|
Athul Joshi answered |
Sum of all interior angles of a convex polygon = (n - 2)* 180
So, (n - 2) * 180 = 1440
n - 2 = 8
n = 10
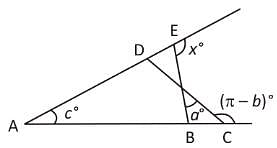
The angles x°, a°, c° and (π – b)° are indicated in the figure given below. Which one of the following is correct?
- a)x° = a° + c° – b°
- b)x° = b° – a° – c°
- c)x° = a° + b° + c°
- d)x° = a° – b° + c°
Correct answer is option 'C'. Can you explain this answer?
The angles x°, a°, c° and (π – b)° are indicated in the figure given below. Which one of the following is correct?


a)
x° = a° + c° – b°
b)
x° = b° – a° – c°
c)
x° = a° + b° + c°
d)
x° = a° – b° + c°

|
Notes Wala answered |
∠PCT + ∠PCB = π
(Linear pair)
(Linear pair)
∠PCB = π – (π – b°) = b° ..... (i)

In ΔBPC,

In ΔBPC,
∠PCB + ∠BPC + ∠PBC = π
∠PBC = π – ∠PCB – ∠BPC = π – b° – a° ..... (ii)
∵ ∠ABE + ∠EBC = π
(∵ ∠PBC = ∠EBC) (linear pair)
∠ABE = π – ∠PBC = π – (π – b° – a°) = a° + b° ...(iii)
(∵ ∠PBC = ∠EBC) (linear pair)
∠ABE = π – ∠PBC = π – (π – b° – a°) = a° + b° ...(iii)
Now, in ΔABE
Sum of two interior angles = Exterior angle
∠EAB + ∠ABE = ∠BES ⇒ c° + b° + a° = x°
∴ x° = a° + b° + c°.
Hence, option C is correct.
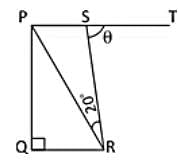
In the trapezium PQRS, QR || PS, ∠Q = 90°, PQ = QR and ∠PRS = 20°. If ∠TSR = θ, then the value of θ is:
- a)75°
- b)55°
- c)65°
- d)45°
Correct answer is option 'C'. Can you explain this answer?
In the trapezium PQRS, QR || PS, ∠Q = 90°, PQ = QR and ∠PRS = 20°. If ∠TSR = θ, then the value of θ is:


a)
75°
b)
55°
c)
65°
d)
45°

|
BT Educators answered |
In the given figure,
PQ = QR and ∠PQR = 90° ⇒ ∠QPR = ∠QRP = 45°.
∴ ∠QRS = (45° + 20°) = 65°.
∴ θ = ∠QRS = 65° (alt. ∠s)
PQ = QR and ∠PQR = 90° ⇒ ∠QPR = ∠QRP = 45°.
∴ ∠QRS = (45° + 20°) = 65°.
∴ θ = ∠QRS = 65° (alt. ∠s)
Hence, option C is correct.
Consider the following statementsI. The locus of points which are equidistant from two parallel lines is a line parallel to both of them and drawn mid way between them.
II. The perpendicular distance of any point on this locus line from two original parallel lines are equal. Further, no point outside this locus line has this property.Which of the above statements is/are correct?- a)Only I
- b)Only II
- c)Both I and II
- d)Neither I nor II
Correct answer is option 'C'. Can you explain this answer?
Consider the following statements
I. The locus of points which are equidistant from two parallel lines is a line parallel to both of them and drawn mid way between them.
II. The perpendicular distance of any point on this locus line from two original parallel lines are equal. Further, no point outside this locus line has this property.
II. The perpendicular distance of any point on this locus line from two original parallel lines are equal. Further, no point outside this locus line has this property.
Which of the above statements is/are correct?
a)
Only I
b)
Only II
c)
Both I and II
d)
Neither I nor II
|
|
Rahul Kapoor answered |
Statements I and II are both true, because the locus of points which are equidistant from two parallel lines is a line parallel to both of them and draw mid way between them.
Also, it is true that the perpendicular distances of any point on this locus line from two original parallel lines are equal. Further, no point outside this locus line has this property.
Hence, option C is correct.
Also, it is true that the perpendicular distances of any point on this locus line from two original parallel lines are equal. Further, no point outside this locus line has this property.

Hence, option C is correct.
In the given figure, AB || CD, m∠ABF = 45° and m∠CFC = 110°. Then, m∠FDC is:
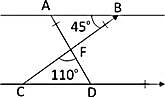
- a)25°
- b)45°
- c)35°
- d)30°
Correct answer is option 'A'. Can you explain this answer?
In the given figure, AB || CD, m∠ABF = 45° and m∠CFC = 110°. Then, m∠FDC is:


a)
25°
b)
45°
c)
35°
d)
30°

|
Pioneer Academy answered |
As in the given figure,
∠FCD = ∠FBA = 45° (alt. ∠s)
∴ ∠FDC = 180° ‒ (110° + 45°) = 25°.
Hence, option A is correct.
∠FCD = ∠FBA = 45° (alt. ∠s)
∴ ∠FDC = 180° ‒ (110° + 45°) = 25°.
Hence, option A is correct.
In the given figure, AB || CD, ∠ABO = 40° and ∠CDO = 30°. If ∠DOB = x°, then the value of x is:

- a)35°
- b)110°
- c)70°
- d)140°
Correct answer is option 'C'. Can you explain this answer?
In the given figure, AB || CD, ∠ABO = 40° and ∠CDO = 30°. If ∠DOB = x°, then the value of x is:


a)
35°
b)
110°
c)
70°
d)
140°

|
Notes Wala answered |
In the given figure,
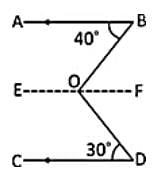
Through O draw EOF parallel to AB & so to CD.
∴ ∠BOF = ∠ABO = 40° (alt. ∠s)
Similarly, ∠FOD = ∠CDO = 30° (alt. ∠s)
∴ ∠BOD = (40° + 30°) = 70°.
So, x = 70°.

Through O draw EOF parallel to AB & so to CD.
∴ ∠BOF = ∠ABO = 40° (alt. ∠s)
Similarly, ∠FOD = ∠CDO = 30° (alt. ∠s)
∴ ∠BOD = (40° + 30°) = 70°.
So, x = 70°.
Hence, option C is correct.
A lady grows cabbages in her garden that is in the shape of a square. Each cabbage takes 1 square feet of area in her garden. This year, she has increased her output by 211 cabbages as compared to last year. The shape of the area used for growing the cabbages has remained a square in both these years. How many cabbages did she produce this year?- a)11236
- b)11025
- c)14400
- d)12696
- e)Cannot be determined
Correct answer is option 'A'. Can you explain this answer?
A lady grows cabbages in her garden that is in the shape of a square. Each cabbage takes 1 square feet of area in her garden. This year, she has increased her output by 211 cabbages as compared to last year. The shape of the area used for growing the cabbages has remained a square in both these years. How many cabbages did she produce this year?
a)
11236
b)
11025
c)
14400
d)
12696
e)
Cannot be determined

|
Mrinalini Dasgupta answered |
The shape of the area used for growing cabbages has remained a square in both the years.
Let the side of the square area used for growing cabbages this year be X ft.
Therefore, the area of the ground used for cultivation this year = X2 sq.ft.
Let the side of the square area used for growing cabbages this year be X ft.
Therefore, the area of the ground used for cultivation this year = X2 sq.ft.
Let the side of the square area used for growing cabbages last year be Y ft.
Therefore, the area of the ground used for cultivation last year = Y2 sq.ft.
Therefore, the area of the ground used for cultivation last year = Y2 sq.ft.
As the number of cabbages grown has increased by 211, the area would have increased by 211 sq ft because each cabbage takes 1 sq ft space.
Hence, X2 - Y2 = 211
(X + Y)(X - Y) = 211.
211 is a prime number and hence it will have only two factors. i.e., 211 and 1.
Therefore, 211 can be expressed as product of 2 numbers in only way = 211 * 1
i.e., (X + Y)(X - Y) = 211 * 1
So, (X + Y) should be 211 and (X - Y) should be 1.
Solving the two equations we get X = 106 and Y = 105.
(X + Y)(X - Y) = 211.
211 is a prime number and hence it will have only two factors. i.e., 211 and 1.
Therefore, 211 can be expressed as product of 2 numbers in only way = 211 * 1
i.e., (X + Y)(X - Y) = 211 * 1
So, (X + Y) should be 211 and (X - Y) should be 1.
Solving the two equations we get X = 106 and Y = 105.
Therefore, number of cabbages produced this year = X2 = 1062 = 11236.
The area in both the years are squares of two numbers.
That rules out choice D. 12696 is not the square of any number.
Check Choice A: If this year's produce is 11236, last year's produce would have been 11236 - 211 = 11025
11025 is the square of 105.
So, 11236 is the answer.
The area in both the years are squares of two numbers.
That rules out choice D. 12696 is not the square of any number.
Check Choice A: If this year's produce is 11236, last year's produce would have been 11236 - 211 = 11025
11025 is the square of 105.
So, 11236 is the answer.
Consider the triangle shown in the figure where BC = 12 cm, Db = 9 cm, CD = 6 cm and What is the ratio of the perimeter of the triangle ADC to that of the triangle BDC ?
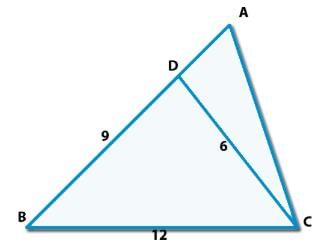
- a)7:9
- b)8:9
- c)6:9
- d)5:9
- e)None of these
Correct answer is option 'A'. Can you explain this answer?
Consider the triangle shown in the figure where BC = 12 cm, Db = 9 cm, CD = 6 cm and What is the ratio of the perimeter of the triangle ADC to that of the triangle BDC ?


a)
7:9
b)
8:9
c)
6:9
d)
5:9
e)
None of these

|
Nayanika Bajaj answered |
Here, ∠ACB = c+180-(2c-b) = 180-(b+c)
So, We can say that Δ BCD and &delta ABC will be similar.
According to property of similarity,
AB/12 = 12/9
Hence,
AB = 16
AC/6 = 12/9
AC = 8
Hence, AD = 7 and AC = 8
Now,
Perimeter of Delta; ADC / Perimeter of &delta BDC,
= (6+7+8)/(9+6+12)
= 21/27 = 7/9.
So, We can say that Δ BCD and &delta ABC will be similar.
According to property of similarity,
AB/12 = 12/9
Hence,
AB = 16
AC/6 = 12/9
AC = 8
Hence, AD = 7 and AC = 8
Now,
Perimeter of Delta; ADC / Perimeter of &delta BDC,
= (6+7+8)/(9+6+12)
= 21/27 = 7/9.
Vertices of a quadrilateral ABCD are A(0, 0), B(4, 5), C(9, 9) and D(5, 4). What is the shape of the quadrilateral?- a)Square
- b)Rectangle but not a square
- c)Rhombus
- d)Parallelogram but not a rhombus
- e)Kite
Correct answer is option 'C'. Can you explain this answer?
Vertices of a quadrilateral ABCD are A(0, 0), B(4, 5), C(9, 9) and D(5, 4). What is the shape of the quadrilateral?
a)
Square
b)
Rectangle but not a square
c)
Rhombus
d)
Parallelogram but not a rhombus
e)
Kite

|
Nikhil Khanna answered |
Shape of the Quadrilateral:
- Given Coordinates:
- A(0, 0)
- B(4, 5)
- C(9, 9)
- D(5, 4)
- Explanation:
- A rhombus is a special type of quadrilateral where all four sides are equal in length.
- In this case, the given coordinates form a quadrilateral where the distances between consecutive points are all equal:
- AB = 5 units
- BC = 5 units
- CD = 5 units
- DA = 5 units
- Conclusion:
- Since all four sides of the quadrilateral ABCD are equal in length, it meets the definition of a rhombus.
Therefore, the shape of the quadrilateral ABCD is a rhombus.
- Given Coordinates:
- A(0, 0)
- B(4, 5)
- C(9, 9)
- D(5, 4)
- Explanation:
- A rhombus is a special type of quadrilateral where all four sides are equal in length.
- In this case, the given coordinates form a quadrilateral where the distances between consecutive points are all equal:
- AB = 5 units
- BC = 5 units
- CD = 5 units
- DA = 5 units
- Conclusion:
- Since all four sides of the quadrilateral ABCD are equal in length, it meets the definition of a rhombus.
Therefore, the shape of the quadrilateral ABCD is a rhombus.
Consider the following statementsIf two straight lines intersect, then
I. vertically opposite angles are equal.
II. vertically opposite angles are supplementary.
III. adjacent angles are complementary.Which of the statements given above is/are correct?- a)Only III
- b)Only I
- c)II and III
- d)II and II
Correct answer is option 'B'. Can you explain this answer?
Consider the following statements
If two straight lines intersect, then
I. vertically opposite angles are equal.
II. vertically opposite angles are supplementary.
III. adjacent angles are complementary.
I. vertically opposite angles are equal.
II. vertically opposite angles are supplementary.
III. adjacent angles are complementary.
Which of the statements given above is/are correct?
a)
Only III
b)
Only I
c)
II and III
d)
II and II
|
|
Rahul Kapoor answered |
Here, AB and CD are two lines.
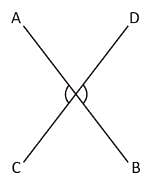
If two straight lines intersect, then opposite vertically angles are equal.
Hence, option B is correct.

If two straight lines intersect, then opposite vertically angles are equal.
Hence, option B is correct.
The floor of a rectangular room of 72 square meters area needs to be covered by a circular carpet that occupies exactly half the area of the floor. What must be the circumference of the carpet in meters?- a)3√π
- b)6√π
- c)9√π
- d)12√π
- e)15√π
Correct answer is option 'D'. Can you explain this answer?
The floor of a rectangular room of 72 square meters area needs to be covered by a circular carpet that occupies exactly half the area of the floor. What must be the circumference of the carpet in meters?
a)
3√π
b)
6√π
c)
9√π
d)
12√π
e)
15√π

|
Prashanth Chawla answered |
Given that the area of the rectangular floor = 72 square meters
And that the carpet occupies exactly half the area.
Area of carpet = 36 square meters.
Let the radius of carpet = R meters
Therefore πR2 = 36
Therefore the circumference =
In the coordinate system, the center of a circle lies at (2, 3). If point A with coordinates (-1, 7) does not lie outside the circle, which of the following points must lie inside the circle?I. (0, 7)II. (5, -1)III. (-2, 7)- a)I only
- b)II only
- c)III only
- d)I and II only
- e)None of the above
Correct answer is option 'A'. Can you explain this answer?
In the coordinate system, the center of a circle lies at (2, 3). If point A with coordinates (-1, 7) does not lie outside the circle, which of the following points must lie inside the circle?
I. (0, 7)
II. (5, -1)
III. (-2, 7)
a)
I only
b)
II only
c)
III only
d)
I and II only
e)
None of the above

|
Sreemoyee Nambiar answered |
To determine which of the given points must lie inside the circle, we need to calculate the distance between each point and the center of the circle. If the distance is less than the radius of the circle, then the point lies inside the circle.
1. Calculating the distance for point (0, 7):
The distance between two points (x1, y1) and (x2, y2) is given by the formula:
distance = sqrt((x2 - x1)^2 + (y2 - y1)^2)
Using this formula, the distance between (0, 7) and (2, 3) is:
distance = sqrt((2 - 0)^2 + (3 - 7)^2) = sqrt(4 + 16) = sqrt(20) = 2sqrt(5)
Since 2sqrt(5) is greater than the radius of the circle, point (0, 7) lies outside the circle.
2. Calculating the distance for point (5, -1):
The distance between (5, -1) and (2, 3) is:
distance = sqrt((2 - 5)^2 + (3 - (-1))^2) = sqrt(9 + 16) = sqrt(25) = 5
Since 5 is equal to the radius of the circle, point (5, -1) lies on the circumference of the circle, but not inside it.
3. Calculating the distance for point (-2, 7):
The distance between (-2, 7) and (2, 3) is:
distance = sqrt((2 - (-2))^2 + (3 - 7)^2) = sqrt(16 + 16) = sqrt(32) = 4sqrt(2)
Since 4sqrt(2) is greater than the radius of the circle, point (-2, 7) lies outside the circle.
Therefore, out of the given points, only point A (-1, 7) lies inside the circle. Hence, the correct answer is option A (I only).
1. Calculating the distance for point (0, 7):
The distance between two points (x1, y1) and (x2, y2) is given by the formula:
distance = sqrt((x2 - x1)^2 + (y2 - y1)^2)
Using this formula, the distance between (0, 7) and (2, 3) is:
distance = sqrt((2 - 0)^2 + (3 - 7)^2) = sqrt(4 + 16) = sqrt(20) = 2sqrt(5)
Since 2sqrt(5) is greater than the radius of the circle, point (0, 7) lies outside the circle.
2. Calculating the distance for point (5, -1):
The distance between (5, -1) and (2, 3) is:
distance = sqrt((2 - 5)^2 + (3 - (-1))^2) = sqrt(9 + 16) = sqrt(25) = 5
Since 5 is equal to the radius of the circle, point (5, -1) lies on the circumference of the circle, but not inside it.
3. Calculating the distance for point (-2, 7):
The distance between (-2, 7) and (2, 3) is:
distance = sqrt((2 - (-2))^2 + (3 - 7)^2) = sqrt(16 + 16) = sqrt(32) = 4sqrt(2)
Since 4sqrt(2) is greater than the radius of the circle, point (-2, 7) lies outside the circle.
Therefore, out of the given points, only point A (-1, 7) lies inside the circle. Hence, the correct answer is option A (I only).
What is the value of a?
(1) The area of an equilateral triangle of side length 3a units is 9√3 square units
(2) The area of an isosceles triangle whose sides are of length 4a, 4a and a units is 3√7 square units- a)Statement (1) ALONE is sufficient, but statement (2) alone is
not sufficient to answer the question asked. - b)Statement (2) ALONE is sufficient, but statement (1) alone is
not sufficient to answer the question asked. - c)BOTH statements (1) and (2) TOGETHER are sufficient to
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked. - d)EACH statement ALONE is sufficient to answer the question
asked. - e)Statements (1) and (2) TOGETHER are NOT sufficient to
answer the question asked, and additional data specific to the
problem are needed.
Correct answer is option 'D'. Can you explain this answer?
What is the value of a?
(1) The area of an equilateral triangle of side length 3a units is 9√3 square units
(2) The area of an isosceles triangle whose sides are of length 4a, 4a and a units is 3√7 square units
(1) The area of an equilateral triangle of side length 3a units is 9√3 square units
(2) The area of an isosceles triangle whose sides are of length 4a, 4a and a units is 3√7 square units
a)
Statement (1) ALONE is sufficient, but statement (2) alone is
not sufficient to answer the question asked.
not sufficient to answer the question asked.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is
not sufficient to answer the question asked.
not sufficient to answer the question asked.
c)
BOTH statements (1) and (2) TOGETHER are sufficient to
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked.
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked.
d)
EACH statement ALONE is sufficient to answer the question
asked.
asked.
e)
Statements (1) and (2) TOGETHER are NOT sufficient to
answer the question asked, and additional data specific to the
problem are needed.
answer the question asked, and additional data specific to the
problem are needed.

|
Pallabi Sengupta answered |
Steps 1 & 2: Understand Question and Draw Inferences
To find: the value of a
Step 3: Analyze Statement 1 independently
- Area of an equilateral Δ of side length 3a is 9√3
So, a = 2 (rejecting a = -2 because the side of a triangle, 3a, cannot be negative)
Thus, Statement 1 alone is sufficient to answer the question
Thus, Statement 1 alone is sufficient to answer the question
Step 4: Analyze Statement 2 independently
- The area of an isosceles triangle whose sides are of length 4a, 4a and a units is 3√7 square units
- Let’s draw the given triangle:
- A perpendicular on the side that measures a units from the opposite vertex will bisect this side (Property of Isosceles triangles)
- Let the height of the perpendicular be h
- So, by applying Pythagoras Theorem,
=> 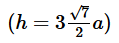 (Rejecting the negative value of h since the length of a perpendicular cannot be negative)
(Rejecting the negative value of h since the length of a perpendicular cannot be negative)
so a2 =4
That is, a = 2 (rejecting a = -2 because the side of a triangle, 3a, cannot be
negative)
negative)
Thus, Statement 2 alone is sufficient to answer the question
Step 5: Analyze both Statements together (if needed)
Since we’ve already arrived at a unique answer in each of Steps 3 and 4, this
step is not required
Since we’ve already arrived at a unique answer in each of Steps 3 and 4, this
step is not required
Answer: Option D
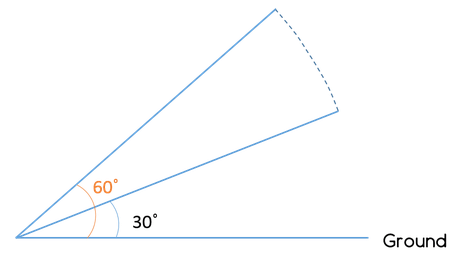 The figure above shows the position, at two instants of time, of a 10-meter-long rod as it was falling down to the ground. If one end of the rod was pinned to the ground, by what vertical distance did the rod fall between the two instants of time?
The figure above shows the position, at two instants of time, of a 10-meter-long rod as it was falling down to the ground. If one end of the rod was pinned to the ground, by what vertical distance did the rod fall between the two instants of time?- a)
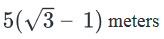
- b)

- c)

- d)
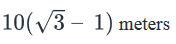
- e)

Correct answer is option 'A'. Can you explain this answer?
The figure above shows the position, at two instants of time, of a 10-meter-long rod as it was falling down to the ground. If one end of the rod was pinned to the ground, by what vertical distance did the rod fall between the two instants of time?
a)
b)
c)
d)
e)

|
Quantronics answered |
Given:
- Length of rod = 10 meters
- Angle with the ground at 1st instant = 60o
- Angle with the ground at 2nd instant = 30o
To find:
- Vertical distance covered between the 2 instants
Approach:
- We will first try to understand the question
- To do so, let us:
- Drop perpendiculars from the free end of the rod at both instants of time
- Label the triangles thus formed
- From the diagram, it is clear that we need to find AB – PQ
- (Note: if you are not sure how AB – PQ is equal to the vertical distance covered by the ladder, drop a perpendicular PX on AB. You’ll see that BQPX is a rectangle and so, PQ = BX. So, AB – PQ = AB – BX = AX. The ladder was earlier at a height of A and it is now at a height of X. So, the distance AX clearly denotes the vertical distance covered by the ladder.)
- Thus, we need to find AB – PQ
- AB is a side of right ΔOBA, which is 30-60- 90 Triangle and angle AOB = 60o
- PQ is a side of right ΔOQP, which is also a 30-60-90 Triangle with angle POQ = 30o
- In each of the 2 right triangles, we know a side and two angles.
- So, by using side ratio property of 30-60-90 triangle, we can find the required values
Working Out:
- Finding AB
- In right ΔOBA
- OB: AB: OA = 1: √3: 2
- We know OA = 10
- AB: OA = √3: 2
- Thus AB = 5√3
- In right ΔOBA
- Finding PQ
- In right ΔOQP
- PQ: OQ: PO = 1: √3: 2
- We know PO = 10
- PQ: PO = 1: 2
- Thus PQ = 5
- In right ΔOQP
- Thus, AB – PQ = 5√3 – 5 = 5 (√3 -1) meters
- Therefore, the correct answer is Option A.
The complement of 72° 40’ is :- a)107° 20’
- b)27° 20’
- c)17° 20’
- d)12° 40’
Correct answer is option 'C'. Can you explain this answer?
The complement of 72° 40’ is :
a)
107° 20’
b)
27° 20’
c)
17° 20’
d)
12° 40’
|
|
Rahul Kapoor answered |
Complement of 72° 40’ is 90° ‒ (72° 40’)
= (89° 60’) ‒ (72° 40’) {since 1° = 60’}
= 17° 20’
= (89° 60’) ‒ (72° 40’) {since 1° = 60’}
= 17° 20’
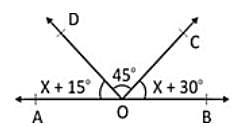
In the given figure, if AOB is a straight line, then the value of x is:
- a)90°
- b)45°
- c)22.5°
- d)150°
Correct answer is option 'B'. Can you explain this answer?
In the given figure, if AOB is a straight line, then the value of x is:


a)
90°
b)
45°
c)
22.5°
d)
150°

|
Wizius Careers answered |
As, (x + 30°) + 45° + (x + 15°) = 180°
⇒ x = 45°.
⇒ x = 45°.
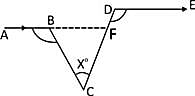
In the adjoining figure, ∠ABC = 100°, ∠EDC = 120° and AB || DE. Then, ∠BCD is equal to:
- a)80°
- b)60°
- c)40°
- d)20°
Correct answer is option 'C'. Can you explain this answer?
In the adjoining figure, ∠ABC = 100°, ∠EDC = 120° and AB || DE. Then, ∠BCD is equal to:


a)
80°
b)
60°
c)
40°
d)
20°

|
Pioneer Academy answered |
In the given figure,
Produce AB to meet CD at F.
∠BFD = ∠EDF = 120° (alt. ∠s)
∠BFC = (180° ‒ 120°) = 60°.
∠CBF = (180° ‒ 100°) = 80°.
∴ ∠BCF = 180° ‒ (60° + 80°) = 40°.
Hence, option C is correct.
Produce AB to meet CD at F.
∠BFD = ∠EDF = 120° (alt. ∠s)
∠BFC = (180° ‒ 120°) = 60°.
∠CBF = (180° ‒ 100°) = 80°.
∴ ∠BCF = 180° ‒ (60° + 80°) = 40°.
Hence, option C is correct.
Two right angled triangles are congruent if :
I.The hypotenuse of one triangle is equal to the hypotenuse of the other.
II.a side for one triangle is equal to the corresponding side of the other.
III.Sides of the triangles are equal.
IV. An angle of the triangle are equal.
Of these statements, the correct ones are combination of:- a)I and II
- b)II and III
- c)I and III
- d)IV only
- e)I and IV
Correct answer is option 'A'. Can you explain this answer?
Two right angled triangles are congruent if :
I.The hypotenuse of one triangle is equal to the hypotenuse of the other.
II.a side for one triangle is equal to the corresponding side of the other.
III.Sides of the triangles are equal.
IV. An angle of the triangle are equal.
Of these statements, the correct ones are combination of:
I.The hypotenuse of one triangle is equal to the hypotenuse of the other.
II.a side for one triangle is equal to the corresponding side of the other.
III.Sides of the triangles are equal.
IV. An angle of the triangle are equal.
Of these statements, the correct ones are combination of:
a)
I and II
b)
II and III
c)
I and III
d)
IV only
e)
I and IV

|
Advait Roy answered |
Two right angled triangle are congruent if the hypotenuse of one triangle is equal to the hypotenuse of the other and a side of one triangle is equal to the corresponding side of the other triangle
In ΔABC, point D lies on the side AB between vertices A and B.
If CD = CB, is AD > BC?
(1) ∠ADC = 110
(2) ∠ACD = 30
- a)Statement (1) ALONE is sufficient, but statement (2) alone is
not sufficient to answer the question asked. - b)Statement (2) ALONE is sufficient, but statement (1) alone is
not sufficient to answer the question asked. - c)BOTH statements (1) and (2) TOGETHER are sufficient to
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked. - d)EACH statement ALONE is sufficient to answer the question
asked. - e)Statements (1) and (2) TOGETHER are NOT sufficient to
answer the question asked, and additional data specific to the
problem are needed.
Correct answer is option 'C'. Can you explain this answer?
In ΔABC, point D lies on the side AB between vertices A and B.
If CD = CB, is AD > BC?
(1) ∠ADC = 110
(2) ∠ACD = 30
If CD = CB, is AD > BC?
(1) ∠ADC = 110
(2) ∠ACD = 30
a)
Statement (1) ALONE is sufficient, but statement (2) alone is
not sufficient to answer the question asked.
not sufficient to answer the question asked.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is
not sufficient to answer the question asked.
not sufficient to answer the question asked.
c)
BOTH statements (1) and (2) TOGETHER are sufficient to
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked.
answer the question asked, but NEITHER statement ALONE
is sufficient to answer the question asked.
d)
EACH statement ALONE is sufficient to answer the question
asked.
asked.
e)
Statements (1) and (2) TOGETHER are NOT sufficient to
answer the question asked, and additional data specific to the
problem are needed.
answer the question asked, and additional data specific to the
problem are needed.

|
Mrinalini Dasgupta answered |
step 1 & 2: Understand Question and Draw Inference
Given:
Given:
Representing the given information visually as below:
- In triangle BCD, CD = CB
- So, angles opposite to these sides will be equal. Let these angles measure Xo
- Let ∠ACD = y∘
- So, the different angles in this figure will be as under:
To find: If AD > BC
- That is, if AD > CD (since BC = CD)
- That is, if in triangle ADC,
- ∠ACD > ∠DAC (since sides opposite to greater angles are greater)
- That is, y∘ > x∘ − y∘
- That is, 2y∘ > x∘
- So, the answer to the question will be YES if
- 2y∘ > x∘
Step 3 : Analyze Statement 1 independent
Step 4 : Analyze Statement 2 independent
Step 5: Analyze Both Statements Together (if needed)
From Statement 1:x = 70∘
From Statement 2: y = 30∘
From Statement 2: y = 30∘
Since we now know the values of xo and yo we can determine if 2yo > Xo
Thus, the 2 statements together are sufficient to answer the question.
Answer: Option C
The supplement of 154° 30’ is :- a)25° 30’
- b)44° 45’
- c)158° 45’
- d)168° 30’
Correct answer is option 'A'. Can you explain this answer?
The supplement of 154° 30’ is :
a)
25° 30’
b)
44° 45’
c)
158° 45’
d)
168° 30’

|
Pioneer Academy answered |
Supplement of 154° 30’ is 180° ‒ (154° 30’)
= (179° 30’) ‒ (154° 30’) {since 1° = 60’}
= 25° 30’.
= (179° 30’) ‒ (154° 30’) {since 1° = 60’}
= 25° 30’.
The straight lines AD and BC intersect one another at the point O. If ∠AOB + ∠BOD + ∠DOC = 274°, then ∠AOC is :- a)86°
- b)90°
- c)94°
- d)137°
Correct answer is option 'A'. Can you explain this answer?
The straight lines AD and BC intersect one another at the point O. If ∠AOB + ∠BOD + ∠DOC = 274°, then ∠AOC is :
a)
86°
b)
90°
c)
94°
d)
137°
|
|
Rahul Kapoor answered |
As we know that the sum of all the angles around a point is 360°.
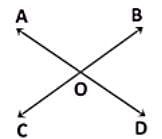
(∠AOB + ∠BOD + ∠DOC) + ∠AOC = 360°
∴ 274° + ∠AOC = 360° or ∠AOC = 86°.
Hence, option A is correct.

(∠AOB + ∠BOD + ∠DOC) + ∠AOC = 360°
∴ 274° + ∠AOC = 360° or ∠AOC = 86°.
Hence, option A is correct.
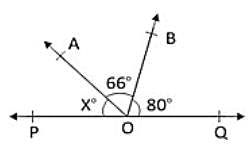
In the given figure, the value of x, that would make POQ a straight line, is :
- a)50°
- b)44°
- c)34°
- d)33°
Correct answer is option 'C'. Can you explain this answer?
In the given figure, the value of x, that would make POQ a straight line, is :


a)
50°
b)
44°
c)
34°
d)
33°
|
|
Rahul Kapoor answered |
POQ will be a straight line,
If 80° + 66° + x = 180°, i.e. x = 34°.
If 80° + 66° + x = 180°, i.e. x = 34°.
In the coordinate plane, a circle has center (-1, -3) and passes through the point (4,2). What is the circumference of the circle?- a)5√2π
- b)10√2π
- c)10√3π
- d)25π
- e)50π
Correct answer is option 'B'. Can you explain this answer?
In the coordinate plane, a circle has center (-1, -3) and passes through the point (4,2). What is the circumference of the circle?
a)
5√2π
b)
10√2π
c)
10√3π
d)
25π
e)
50π

|
Yash Rane answered |
To find the circumference of this circle, we need its radius. And the radius is the distance from its center to any point on the circle, so the radius is the distance from (-1 ,-3) to (4,2).
This distance is computed with the distance formula, which is essentially the Pythagorean Theorem. The sides of the triangle are the x and y distances, 5 and 5, so the distance is 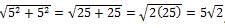
The radius of the circle is 5√2, so the circumference is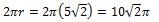
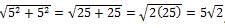
The radius of the circle is 5√2, so the circumference is
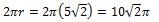
The correct answer is (B).
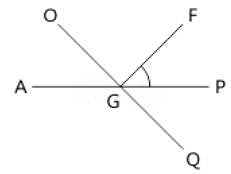
In the given figure lines AP and OQ intersect at G. If ∠AGO + ∠PGF = 70° and ∠PGQ = 40°. Find the angle value of ∠PGF.
- a)31°
- b)35°
- c)30°
- d)20°
Correct answer is option 'C'. Can you explain this answer?
In the given figure lines AP and OQ intersect at G. If ∠AGO + ∠PGF = 70° and ∠PGQ = 40°. Find the angle value of ∠PGF.


a)
31°
b)
35°
c)
30°
d)
20°
|
|
Rahul Kapoor answered |
As AP is a straight line and rays GO and GF stands on it.
∴ ∠AGO + ∠OGF + ∠PGF = 180°
∴ ∠AGO + ∠OGF + ∠PGF = 180°
⇒ (∠AGO + ∠PGF) + ∠OGF = 180°
⇒ 70° + ∠OGF = 180°
⇒ ∠OGF = 180° – 70°
⇒ ∠OGF = 110°
As, OQ is a straight line, rays GF and GP stands on it.
∠OGF + ∠PGF + ∠PGQ = 180°
Putting the value of ∠OGF & ∠PGQ
110° + ∠PGF + 40° = 180°
∠PGF = 180° – 150° = 30°
Hence, option C is correct.
Hence, option C is correct.
What is the measure of the radius of the circle that circumscribes a triangle whose sides measure 9, 40 and 41?- a)6
- b)4
- c)24.5
- d)20.5
- e)12.5
Correct answer is option 'D'. Can you explain this answer?
What is the measure of the radius of the circle that circumscribes a triangle whose sides measure 9, 40 and 41?
a)
6
b)
4
c)
24.5
d)
20.5
e)
12.5

|
Anirban Singh answered |
Solution:
Step 1: Find the semi-perimeter of the triangle
Given sides of the triangle are 9, 40, and 41.
Semi-perimeter, denoted as 's', is half of the perimeter of the triangle.
Perimeter = 9 + 40 + 41 = 90
Semi-perimeter, s = 90/2 = 45
Step 2: Apply the formula to find the radius of the circumcircle
The radius of the circumcircle of a triangle with sides a, b, and c and semi-perimeter s is given by the formula:
\(R = \frac{abc}{4 \sqrt{s(s-a)(s-b)(s-c)}}\)
Substitute the values of sides and semi-perimeter into the formula:
\(R = \frac{9*40*41}{4 \sqrt{45(45-9)(45-40)(45-41)}}\)
\(R = \frac{1440}{4 \sqrt{45*36*5*4}}\)
\(R = \frac{1440}{4*120}\)
\(R = \frac{1440}{480}\)
\(R = 3\)
Therefore, the measure of the radius of the circle that circumscribes the triangle is 20.5, which corresponds to option 'D'.
Step 1: Find the semi-perimeter of the triangle
Given sides of the triangle are 9, 40, and 41.
Semi-perimeter, denoted as 's', is half of the perimeter of the triangle.
Perimeter = 9 + 40 + 41 = 90
Semi-perimeter, s = 90/2 = 45
Step 2: Apply the formula to find the radius of the circumcircle
The radius of the circumcircle of a triangle with sides a, b, and c and semi-perimeter s is given by the formula:
\(R = \frac{abc}{4 \sqrt{s(s-a)(s-b)(s-c)}}\)
Substitute the values of sides and semi-perimeter into the formula:
\(R = \frac{9*40*41}{4 \sqrt{45(45-9)(45-40)(45-41)}}\)
\(R = \frac{1440}{4 \sqrt{45*36*5*4}}\)
\(R = \frac{1440}{4*120}\)
\(R = \frac{1440}{480}\)
\(R = 3\)
Therefore, the measure of the radius of the circle that circumscribes the triangle is 20.5, which corresponds to option 'D'.
What is the area of the 144o sector of the circle?(1) The square with one of the sides as the chord that subtends an angle of 90o on the circle measures 64 sq. cm. in area.
(2) When an isosceles triangle is constructed by taking the longest chord of the circle and the other vertex on the circumference of the circle, the length of each of the equal sides of the triangle is 4√2 cm.- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'D'. Can you explain this answer?
What is the area of the 144o sector of the circle?
(1) The square with one of the sides as the chord that subtends an angle of 90o on the circle measures 64 sq. cm. in area.
(2) When an isosceles triangle is constructed by taking the longest chord of the circle and the other vertex on the circumference of the circle, the length of each of the equal sides of the triangle is 4√2 cm.
(2) When an isosceles triangle is constructed by taking the longest chord of the circle and the other vertex on the circumference of the circle, the length of each of the equal sides of the triangle is 4√2 cm.
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.

|
Anirban Singh answered |
Steps 1 & 2: Understand Question and Draw Inferences
We need to find the area of a sector subtending an angle of 144o at the center of the circle.
For this we need either the radius of the circle or the area of a known portion of the circle.
Step 3: Analyze Statement 1
The chord that subtends an angle of 90o on the circle is the diameter itself.
(Please note that the chord here subtends an angle of 90o on the circle, and not on the center of the circle)
Area of the square with the side as the diameter is 64 sq. cm.
Therefore the length of the diameter = √(64) = 8 cm.
- Radius of the circle = 4 cm.
We can find the area of the 144o sector by using the formula
Therefore statement 1 is sufficient to arrive at a unique answer.
Step 4: Analyze Statement 2
The longest chord of the circle is the diameter itself.
We know that the diameter subtends an angle of 90o on the circle.
Therefore the isosceles triangle constructed would be an isosceles right angled triangle with the diameter as the hypotenuse.
Given that the length of the equal sides is 4√2 cm.
Let the diameter = D
Therefore, D2 = (4√2)2 + (4√2)2 (Applying Pythagoras)
⇒ D2 = 64
⇒ D = 8 cm.
⇒ Radius = 4 cm.
We can find the area of the 144o sector by using the formula 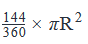
Therefore statement 2 is sufficient to arrive at a unique answer.
Step 5: Analyze Both Statements Together (if needed)
We arrived at a unique answer in both Step 3 and Step 4 above. So this step is not required.
Correct Answer: D
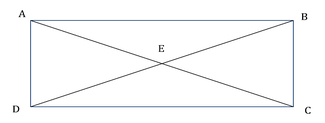 In the figure shown above, point E is the intersection point of the diagnoals AC and BD of rectangle ABCD. Is AB greater than BC?
In the figure shown above, point E is the intersection point of the diagnoals AC and BD of rectangle ABCD. Is AB greater than BC? - AE = 5 cms and AB = 7.25 cm
- The perimeter of AEB is greater than the perimeter of BEC
- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is 'D'. Can you explain this answer?
In the figure shown above, point E is the intersection point of the diagnoals AC and BD of rectangle ABCD. Is AB greater than BC?
- AE = 5 cms and AB = 7.25 cm
- The perimeter of AEB is greater than the perimeter of BEC
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.
|
|
Aisha Gupta answered |
Steps 1 & 2: Understand Question and Draw Inferences
In the given rectangle ABCD, we need to find if AB > BC, i.e., if the length is greater than the breadth.
Note that AC and BD are the diagonals of the rectangle and E is their point of intersection.
In right triangle ADC, by Pythagoras Theorem,
AD2 + DC2 = AC2 . . . (1)
Similarly, in right triangle DCB,
DC2 + BC2 = DB2 . . . (2)
Equation (1) – Equation (2):
AD2 – BC2 = AC2 – DB2
Being opposite sides of a rectangle, AD = BC
Therefore, the above equation becomes:
0 = AC2 – DB2Therefore, AC = DB.
Thus, we observe that the two diagonals of the rectangle are equal.
As we know that diagonals of a rectangle bisect each other, we can write
AE = BE = CE = DE = AC/2 = BD/2
(Now, it is also easy to notice that the rectangle is symmetrical about point E.
Therefore, point E will divide each diagonal in half. )
Step 3: Analyze Statement 1
Given AE = 5
- AC = 10
Also, given that AB = 7.25
Notice that ABC is a right angled triangle. Applying Pythagoras theorem
AC2 = AB2 + BC2
- BC2 = 102 – 7.252
Therefore, we can calculate the value of BC and so we can arrive at a unique answer on whether AB is greater than BC or not.
Statement 1 is sufficient to arrive at a unique answer.
Step 4: Analyze Statement 2
Perimeter of AEB > Perimeter of BEC
- AE + BE + AB > BE + CE + BC
- AB > BC (Since we already noted that AE = BE = CE = DE)
Statement 2 is sufficient to arrive at a unique answer.
Step 5: Analyze Both Statements Together (if needed)
We arrived at a unique answer in step 3 and step 4 above. Therefore, this step is not needed.
Correct Answer: D
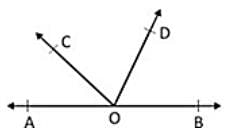
In the given figure, AOB is a straight line. If ∠AOC + ∠BOD = 85°, then ∠COD is:
- a)85°
- b)90°
- c)95°
- d)100°
Correct answer is option 'C'. Can you explain this answer?
In the given figure, AOB is a straight line. If ∠AOC + ∠BOD = 85°, then ∠COD is:


a)
85°
b)
90°
c)
95°
d)
100°
|
|
Rahul Kapoor answered |
Clearly,
∠AOC + ∠COD + ∠BOD = 180°
∴ 85° + ∠COD = 180°. So, ∠COD = (180° ‒ 85°) = 95°.
Hence, option C is correct.
∠AOC + ∠COD + ∠BOD = 180°
∴ 85° + ∠COD = 180°. So, ∠COD = (180° ‒ 85°) = 95°.
Hence, option C is correct.
In a Δ ABC, (1 / 2) ∠A + (1 / 3) ∠C + (1 / 2) ∠B = 80°, then what is the value of ∠C?
- a)35°
- b)40°
- c)60°
- d)70°
Correct answer is option 'C'. Can you explain this answer?
In a Δ ABC, (1 / 2) ∠A + (1 / 3) ∠C + (1 / 2) ∠B = 80°, then what is the value of ∠C?
a)
35°
b)
40°
c)
60°
d)
70°
|
|
Rahul Kapoor answered |
Given that,
(1 / 2) ∠A + (1 / 3) ∠C + (1 / 2) ∠B = 80�
⇒ 3∠A + 2∠C + 3∠B = 480�
⇒ 3(∠A + ∠B) + 2∠C = 480� ....(i)
Also, in ΔABC,
∠A + ∠B + ∠C = 180�
On multiplying both sides L.H.S. & R.H.S. by 3, we get
3(∠A + ∠B) + 3∠C = 540� ..... (ii)
On subtracting Eq. (i) from Eq. (ii), we get
∠C = 60�.
Hence, option C is correct.
(1 / 2) ∠A + (1 / 3) ∠C + (1 / 2) ∠B = 80�
⇒ 3∠A + 2∠C + 3∠B = 480�
⇒ 3(∠A + ∠B) + 2∠C = 480� ....(i)
Also, in ΔABC,
∠A + ∠B + ∠C = 180�
On multiplying both sides L.H.S. & R.H.S. by 3, we get
3(∠A + ∠B) + 3∠C = 540� ..... (ii)
On subtracting Eq. (i) from Eq. (ii), we get
∠C = 60�.
Hence, option C is correct.
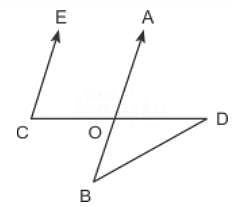
In the figure given above, EC is parallel to AB, ∠ECD = 70° and ∠BDO = 20°. What is the value of ∠OBD?
- a)20°
- b)30°
- c)40°
- d)50°
Correct answer is option 'D'. Can you explain this answer?
In the figure given above, EC is parallel to AB, ∠ECD = 70° and ∠BDO = 20°. What is the value of ∠OBD?


a)
20°
b)
30°
c)
40°
d)
50°
|
|
Rahul Kapoor answered |
Given that, EC || AB
∴ ∠ECO + ∠AOC = 180°

⇒ ∠AOC = 180° – 70° = 110°
∴ ∠ECO + ∠AOC = 180°

⇒ ∠AOC = 180° – 70° = 110°
∴ ∠BOD = ∠AOC = 110°
(alternate angle)
Now, in ΔOBD
∠BOD + ∠ODB + ∠DBO = 180°
∴ 110° + 20° + x° = 180° ⇒ x° = 50°.
Hence, option D is correct.
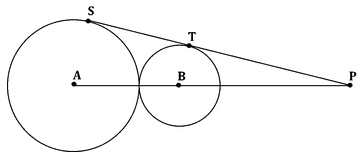 A bigger circle (with center A) and a smaller circle (with center B) are touching each other externally. PT and PS are the tangents drawn to these circles from an external point (as shown in the figure). What is the length of ST?(1) The radii of the bigger and the smaller circles are 9 cm and 4 cm respectively
A bigger circle (with center A) and a smaller circle (with center B) are touching each other externally. PT and PS are the tangents drawn to these circles from an external point (as shown in the figure). What is the length of ST?(1) The radii of the bigger and the smaller circles are 9 cm and 4 cm respectively
(2) PB = 52/5 cm- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'A'. Can you explain this answer?
A bigger circle (with center A) and a smaller circle (with center B) are touching each other externally. PT and PS are the tangents drawn to these circles from an external point (as shown in the figure). What is the length of ST?
(1) The radii of the bigger and the smaller circles are 9 cm and 4 cm respectively
(2) PB = 52/5 cm
(2) PB = 52/5 cm
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.

|
Naroj Boda answered |
Steps 1 & 2: Understand Question and Draw Inferences
Given that PT is a tangent to the small circle and PS is a tangent to the big circle.
ΔPTB and ΔPSA are right angled at T and S respectively.
We need to find the length of ST.
Step 3: Analyze Statement 1
(1) The radii of the bigger and the smaller circles are 9 cm and 4 cm respectively.
If we drop a perpendicular BD on side AS, we get:
BDST is a rectangle (since all angles of this quadrilateral are right angles).
Therefore, since opposite sides of a rectangle are equal, SD = BT = 4 cm
This means, AD = 9 – 4 = 5cm
In right triangle ADB, by applying Pythagoras Theorem, we get:
BD2 + AD2 = AB2
That is, BD2 = (9+4)2 – (5)2
BD2 = (13+5)(13-5)
BD2 = 18*8 = 24*32
Therefore, BD = 22*3 = 12
Since opposite sides of a rectangle are equal, ST = BD = 12 cm
Since we have been able to determine a unique length of ST, Statement (1) is sufficient.
Step 4: Analyze Statement 2
(2) PB = 52/5 cm
In right triangle BTP, we know the length of only one side: BP.
In order to find the lengths of the other sides of this triangle, we need one more piece of information – either one of the two unknown angles, or one of the two unknown sides.
Since we don’t have this information, we will not be able to find the lengths of the unknown sides of triangle BTP.
Due to a similar reasoning, we will not be able to find the length of the sides of triangle ASP.
So, we will not have enough information to find the length of ST.
Therefore statement 2 is not sufficient to arrive at a unique answer.
Step 5: Analyze Both Statements Together (if needed)
We have arrived at a unique answer in step 3 above. Hence this step is not required.
Correct Answer: A
In the figure given below, AB is parallel to CD. ∠ABC = 65°, ∠CDE = 15° and AB = AE. What is the value of ∠AEF?

- a)30°
- b)35°
- c)40°
- d)45°
Correct answer is option 'B'. Can you explain this answer?
In the figure given below, AB is parallel to CD. ∠ABC = 65°, ∠CDE = 15° and AB = AE. What is the value of ∠AEF?


a)
30°
b)
35°
c)
40°
d)
45°

|
Notes Wala answered |
Given that,
∠ABC = 65° and ∠CDE = 15°
Here, ∠ABC + ∠TCB = 180°
(∵ AB || CD)
∠TCB = 180° – ∠ABC
∴ ∠TCB = 180° – 65° = 115°
∵ ∠TCB + ∠DCB = 180°
(Linear pair)
∴ ∠DCB = 65°
Now, in ΔCDE
∠CED = 180° – (∠ECD + ∠EDC)
(∵ ∠ECD = ∠BCD)
= 180° – (– 65° + 15° ) = 100°

∵ ∠DEC + ∠FEC = 180°

∵ ∠DEC + ∠FEC = 180°
⇒ ∠FEC = 180° – 100° = 80°
Given that, AB = AE.
i.e. ΔABE an isosceles triangle.
∴ ∠ABE = ∠AEB = 65°
∵ ∠AEB + ∠AEF + ∠FEC = 180°
(straight line)
⇒ 65° + x° + 80° = 180°
∴ x° = 180° – 145° = 35°.
Hence, option B is correct.
A wheel makes 12 revolutions per min. The angle in radian described by a spoke of the wheel in 1 s is:- a)5π/2
- b)2π/5
- c)3π/5
- d)4π/5
Correct answer is option 'B'. Can you explain this answer?
A wheel makes 12 revolutions per min. The angle in radian described by a spoke of the wheel in 1 s is:
a)
5π/2
b)
2π/5
c)
3π/5
d)
4π/5
|
|
Rahul Kapoor answered |
= 12 × Its circumference
= 12 × 2πr
∴ In 1 s distance travelled by the wheel =
(12 × 2πr) / 60 = (2 / 5) πr
∴ Angle = (Arc / Radius)
= (2 / 5πr) / r = 2π / 5
Which is the required angle.
Hence, option B is correct.
= 12 × 2πr
∴ In 1 s distance travelled by the wheel =
(12 × 2πr) / 60 = (2 / 5) πr
∴ Angle = (Arc / Radius)
= (2 / 5πr) / r = 2π / 5
Which is the required angle.
Hence, option B is correct.
Is the perimeter of triangle ABC greater than 6 cm? (1) One side of triangle ABC measures 3 cm (2) The lengths of the three sides of triangle ABC are consecutive integers - a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'D'. Can you explain this answer?
Is the perimeter of triangle ABC greater than 6 cm?
(1) One side of triangle ABC measures 3 cm
(2) The lengths of the three sides of triangle ABC are consecutive integers
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.

|
Ananya Iyer answered |
Steps 1 & 2: Understand Question and Draw Inferences
Let’s say the sides of the triangle are a, b, and c.
Let the perimeter of the triangle be X.
Thus, X = a + b + c
We need to find whether X > 6
Since we don’t have any other information, let’s move on to the analysis of the statement 1.
Step 3: Analyse Statement 1
Statement 1 says: One side of triangle ABC measures 3 cm.
Let’s assume a = 3 cm
Now, we know that the sum of two sides of a triangle is always greater than the third side.
So, we can write:
b + c > a
Adding a to both sides of this inequality, we get:
a + b + c > 2a
That is, X > 2a
Substituting a = 3 cm in this inequality, we get:
X > 6 cm
Thus, Statement 1 alone is sufficient to answer the question.
Step 4: Analyse Statement 2
Statement 2 says: The lengths of the three sides of triangle ABC are consecutive integers.
So, the lengths of the sides are n, (n+1), (n+2) cm, where n is a positive integer.
Perimeter X = n + (n+1) + (n+2)
X = 3n + 3
X = 3(n+1)
Now, we know that the sum of two sides of a triangle is always greater than the third side.
This means, we can write:
n + (n+1) > n + 2
2n + 1 > n + 2
n > 1
Adding 1 to both sides of this inequality:
n + 1 > 2
We know that multiplying both sides of an inequality with a positive number doesn’t change the inequality. Therefore, multiplying both sides of the above inequality with 3:
3(n+1) > 6
This means, X > 6
Thus, Statement 2 alone is sufficient to determine if X > 6
Step 5: Analyse Both Statements Together (if needed)
Since we got the answer to the question in Steps 3 and 4, we don’t need to perform this step.
Answer: Option (D)
In ΔLMN, LM = |x-7|, MN = |x-4| and NL = x + 1, where x is a number whose value is not known. Is ΔLMN an acute triangle (a triangle each of whose angles measures less than 90∘)?(1) NM = 10(2) LM = 7- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient to answer the question asked.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient to answer the question asked.
- c)BOTH statements (1) and (2) TOGETHER are sufficient to answer the question asked, but NEITHER statement ALONE is sufficient to answer the question asked.
- d)EACH statement ALONE is sufficient to answer the question asked.
- e)Statements (1) and (2) TOGETHER are NOT sufficient to answer the question asked, and additional data specific to the problem are needed.
Correct answer is option 'D'. Can you explain this answer?
In ΔLMN, LM = |x-7|, MN = |x-4| and NL = x + 1, where x is a number whose value is not known. Is ΔLMN an acute triangle (a triangle each of whose angles measures less than 90∘)?
(1) NM = 10
(2) LM = 7
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient to answer the question asked.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient to answer the question asked.
c)
BOTH statements (1) and (2) TOGETHER are sufficient to answer the question asked, but NEITHER statement ALONE is sufficient to answer the question asked.
d)
EACH statement ALONE is sufficient to answer the question asked.
e)
Statements (1) and (2) TOGETHER are NOT sufficient to answer the question asked, and additional data specific to the problem are needed.

|
Sanskriti Ahuja answered |
Given: Some number x
- ΔLMN with sides as shown below:
Find: Is ΔLMN an acute triangle?
Step 3: Analyze Statement 1 independently
- NM = 10
- That is, |x -4| = 10
- So, x is a number whose distance from 4 on the number line is 10
- So, x = -6 or 14
- Rejecting the negative value since x + 1, being the length of a geometric figure, must be positive.
- So, x = 14
- Therefore, the sides of the triangle are:
- 15, 10, 7
- The longest side is 15
- 102 + 72 < 152
- 15, 10, 7
- Therefore, the triangle is obtuse.
- (Note: An obtuse triangle is a triangle one of whose angles is greater than 90∘)
- So, the answer to the given question is NO.
- Since we’ve been able to arrive at a unique answer to the question, Statement 1 alone is sufficient.
Step 4: Analyze Statement 2 independently
- LM = 7
- |x-7| = 7
- So, x is a number whose distance from 7 on the number line is 7
- So, x = 0 or 14
- Case 1: x = 0
- The sides of the triangle are:
- 1, 4, 7
- The longest side is 7
- 1, 4, 7
- In this case, the sum of 2 sides of the triangle (1 + 4) is not greater than the 3rd side
- Therefore, this triangle is not possible
- So, this value of x is not possible.
- Case 2: x = 14
- So, the sides of the triangle are:
- 15, 10, 7
- The longest side is 15
- 102 + 72 < 152
- 15, 10, 7
- Therefore, the triangle is obtuse.
- So, the answer to the given question is NO.
- Since we’ve been able to arrive at a unique answer to the question, Statement 2 alone is sufficient
- Step 5: Analyze Both Statements Together (if needed)Since we’ve already arrived at a unique answer in each of Steps 3 and 4, this step is not requiredHence the correct answer is Option D .
A circular track needs to be fenced along the inner and outer boundaries of the track to prevent stalkers from interrupting any events. What is the width of the track?(1) The fencing used for the outer boundary can actually be used to fence the inner boundary twice.
(2) The area of the circle formed by the inner boundary is 10 sq. meter.- a)Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
- b)Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
- c)BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
- d)EACH statement ALONE is sufficient.
- e)Statements (1) and (2) TOGETHER are NOT sufficient.
Correct answer is option 'C'. Can you explain this answer?
A circular track needs to be fenced along the inner and outer boundaries of the track to prevent stalkers from interrupting any events. What is the width of the track?
(1) The fencing used for the outer boundary can actually be used to fence the inner boundary twice.
(2) The area of the circle formed by the inner boundary is 10 sq. meter.
(2) The area of the circle formed by the inner boundary is 10 sq. meter.
a)
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
b)
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
c)
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
d)
EACH statement ALONE is sufficient.
e)
Statements (1) and (2) TOGETHER are NOT sufficient.

|
Prateek Gupta answered |
Steps 1 & 2: Understand Question and Draw Inferences
Assume that the inner radius, outer radius and width of the circular track are r, R and w.
Therefore R = r + w
We need to find w.
w = R - r
So we need the values of r and R to find W.
Step 3: Analyze Statement 1
This statement says that the fencing used for outer boundary (perimeter of the outer boundary) is twice the size of the fencing for inner boundary (perimeter of inner boundary).
Perimeter of outer boundary = 2πR
Perimeter of inner boundary = 2πr
Therefore,
2πR = 2*2πr
R = 2r.
However, this information is not enough to find w.
Therefore statement 1 is not sufficient to arrive at a unique answer.
Step 4: Analyze Statement 2
The area of the inner circle is 10 sq. meters.
But we still don’t know R and so we cannot calculate the value of w.
Step 5: Analyze Both Statements Together (if needed)
Combining the information we got from statements (1) and (2);
Therefore statement (1) and statement (2) together are sufficient to arrive at a unique answer.
Correct Answer: C
Chapter doubts & questions for Plane Geometry - Mathematics for ACT 2025 is part of ACT exam preparation. The chapters have been prepared according to the ACT exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for ACT 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Plane Geometry - Mathematics for ACT in English & Hindi are available as part of ACT exam.
Download more important topics, notes, lectures and mock test series for ACT Exam by signing up for free.
Mathematics for ACT
144 videos|100 docs|61 tests
|
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup










