All Exams >
Grade 12 >
Mathematics for Grade 12 >
All Questions
All questions of Statistics for Grade 12 Exam
What is the range of the following data?23, 45, 34, 21, 89, 45, 47, 91- a)70
- b)56
- c)71
- d)69
Correct answer is option 'A'. Can you explain this answer?
What is the range of the following data?
23, 45, 34, 21, 89, 45, 47, 91
a)
70
b)
56
c)
71
d)
69
|
|
Vikas Kapoor answered |
Maximum and minimum value of the data 23, 45, 34, 21, 89, 45, 47, 91 are 21 and 91.
Range = 91 – 21 = 70
Range = 91 – 21 = 70
The mean deviation of the following data 14, 15, 16, 17, 13 is:- a)4
- b)2.3
- c)3
- d)1.2
Correct answer is option 'D'. Can you explain this answer?
The mean deviation of the following data 14, 15, 16, 17, 13 is:
a)
4
b)
2.3
c)
3
d)
1.2
|
|
Vikas Kapoor answered |
Here N= 5 , sigma x = 75
so mean = 15
now taking deviation from mean,( By ignoring signs)
we get sigma deviation from mean = 6
Now applying the formula of mean deviation
M.D.= SIGMA deviation from mean/ n
so M.D.= 6/5
= 1.2
so mean = 15
now taking deviation from mean,( By ignoring signs)
we get sigma deviation from mean = 6
Now applying the formula of mean deviation
M.D.= SIGMA deviation from mean/ n
so M.D.= 6/5
= 1.2
The mean of 5 observations is 4.4 and their variance is 8.24. If three observations are 1,2 and 6 , the other two observations are- a)4 and 8
- b)5 and 7
- c)5 and 9
- d)4 and 9
Correct answer is option 'D'. Can you explain this answer?
The mean of 5 observations is 4.4 and their variance is 8.24. If three observations are 1,2 and 6 , the other two observations are
a)
4 and 8
b)
5 and 7
c)
5 and 9
d)
4 and 9
|
|
Gaurav Kumar answered |
Let the other nos. be a and b
then (x+y+1+2+6)/5 = 4.4
x + y = 13 ---------------------(1)
Variance = 8.24


41.2 = 19.88 + (x2 + 19.36 – 8.8x) + (y2 + 19.36 – 8.8y)
21.32 = x2 + y2 + 38.72 – 8.8(x + y)
x2 + y2 + 38.72 – 8.8(13) – 21.32 = 0
(using equation (1))
x2 + y2 – 97 = 0 …(2)
Squaring equation (1) both the sides,
we get (x + y)2 = (13)^2
x2 + y2 + 2xy = 169
97 + 2xy = 169
(using equation (2))
xy = 36 or x = 36/y (1)
⇒ 36/y + y = 13
y2 + 36 = 13y
y2 – 13y + 36 = 0
(y – 4)(y – 9) = 0
Either (y – 4) = 0 or (y – 9) = 0
⇒ y = 4 or y = 9
For y = 4 x = 36/y
= 36/4 = 12
For y = 9
x = 36/9
x = 4
Thus, remaining two observations are 4 and 9.
then (x+y+1+2+6)/5 = 4.4
x + y = 13 ---------------------(1)
Variance = 8.24


41.2 = 19.88 + (x2 + 19.36 – 8.8x) + (y2 + 19.36 – 8.8y)
21.32 = x2 + y2 + 38.72 – 8.8(x + y)
x2 + y2 + 38.72 – 8.8(13) – 21.32 = 0
(using equation (1))
x2 + y2 – 97 = 0 …(2)
Squaring equation (1) both the sides,
we get (x + y)2 = (13)^2
x2 + y2 + 2xy = 169
97 + 2xy = 169
(using equation (2))
xy = 36 or x = 36/y (1)
⇒ 36/y + y = 13
y2 + 36 = 13y
y2 – 13y + 36 = 0
(y – 4)(y – 9) = 0
Either (y – 4) = 0 or (y – 9) = 0
⇒ y = 4 or y = 9
For y = 4 x = 36/y
= 36/4 = 12
For y = 9
x = 36/9
x = 4
Thus, remaining two observations are 4 and 9.
A batsman scores runs in 10 innings as 38,70,48,34,42,55,63,46,54 and 44 , then the mean score is- a)4.94
- b)49.4
- c)494
- d)0.494
Correct answer is option 'B'. Can you explain this answer?
A batsman scores runs in 10 innings as 38,70,48,34,42,55,63,46,54 and 44 , then the mean score is
a)
4.94
b)
49.4
c)
494
d)
0.494
|
|
Mayuri Agrawal answered |
38+70+48+34+42+55+63+46+54 +44=494
494/10
=49.4
494/10
=49.4
The mean deviation about the mean for the following data:5, 6, 7, 8, 6, 9, 13, 12, 15 is:- a)1.5
- b)3.2
- c)2.89
- d)5
Correct answer is option 'C'. Can you explain this answer?
The mean deviation about the mean for the following data:
5, 6, 7, 8, 6, 9, 13, 12, 15 is:
a)
1.5
b)
3.2
c)
2.89
d)
5
|
|
Vikas Kapoor answered |
let, X =5,6,7,8,9,13,12,15.
(5+6+7+8+9+13+12+15)÷9 = 9.
and hence a = 9.
the mean deviation about the mean is summation of |X-a|÷ the total number
i.e , |X-a| = 4,3,2,1,3,0,4,3,6 and the total no. is 9.
hence summation of |X-a| = 26,
the mean deviation is 26 ÷ 9 = 2.89 ans
(5+6+7+8+9+13+12+15)÷9 = 9.
and hence a = 9.
the mean deviation about the mean is summation of |X-a|÷ the total number
i.e , |X-a| = 4,3,2,1,3,0,4,3,6 and the total no. is 9.
hence summation of |X-a| = 26,
the mean deviation is 26 ÷ 9 = 2.89 ans
For a given data, the standard deviation is 20.If 3 is added to each observation , what is the new variance of the resulting observations?- a)20
- b)23
- c)17
- d)60
Correct answer is option 'A'. Can you explain this answer?
For a given data, the standard deviation is 20.If 3 is added to each observation , what is the new variance of the resulting observations?
a)
20
b)
23
c)
17
d)
60
|
|
Sarita Yadav answered |
If a three, is added to each number in a set of data, the mean will be increased by 3 and the standard deviation will be unaltered (since the spread of the data will be unchanged).
Hence, variance of the new data = 20
Hence, variance of the new data = 20
The mean deviation about the mean for the following data 3, 7, 8, 9, 4, 6, 8, 13, 12, 10 is:- a)5
- b)3
- c)2
- d)2.4
Correct answer is option 'D'. Can you explain this answer?
The mean deviation about the mean for the following data 3, 7, 8, 9, 4, 6, 8, 13, 12, 10 is:
a)
5
b)
3
c)
2
d)
2.4
|
|
Gaurav Kumar answered |
Arrange data in ascending order,
3,4,6,7,8,8,9,10,12,13
No. of observations = 10
Median = n/2 => 10/2 = 5h observation.
5th observation is 8
Now we calculate mean deviation about median, i.e;
=> ∑∣xi−M∣/10
= {|3-8| +|4-8| +|6-8| +|7-8| +|8-8| +|8-8| +|9-8| +|10-8| +|12-8| +|13-8| }/10
= { 5 + 4 + 2 + 1 + 0 + 0 + 1 + 2 + 4 + 5}/10
= 24/10 => 2.4
3,4,6,7,8,8,9,10,12,13
No. of observations = 10
Median = n/2 => 10/2 = 5h observation.
5th observation is 8
Now we calculate mean deviation about median, i.e;
=> ∑∣xi−M∣/10
= {|3-8| +|4-8| +|6-8| +|7-8| +|8-8| +|8-8| +|9-8| +|10-8| +|12-8| +|13-8| }/10
= { 5 + 4 + 2 + 1 + 0 + 0 + 1 + 2 + 4 + 5}/10
= 24/10 => 2.4
The two lines of regression are 2x - 7y + 6 = 0 and 7x – 2y +1 = 0. What is correlation coefficient between x and y ?- a)4/49
- b)- 2/7
- c)2/7
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
The two lines of regression are 2x - 7y + 6 = 0 and 7x – 2y +1 = 0. What is correlation coefficient between x and y ?
a)
4/49
b)
- 2/7
c)
2/7
d)
none of these
|
|
Hansa Sharma answered |
ρ = (b(xy) * b(yx))
But sign of ρρ is same as sign of b(xy), b(yx)
Therefore, ρ = 2/7
But sign of ρρ is same as sign of b(xy), b(yx)
Therefore, ρ = 2/7
The standard deviation of first 10 multiples of 4 is:- a)7
- b)8
- c)11.5
- d)14
Correct answer is option 'C'. Can you explain this answer?
The standard deviation of first 10 multiples of 4 is:
a)
7
b)
8
c)
11.5
d)
14
|
|
Krishna Iyer answered |
First 10 multiples of 4 are 4,8,12...40.
This is an A.P.
sum=n/2(a+l)
= 10/2(4+40)
∴ sum=220.
Mean, u=sum/n
= 220/10 = 22
D1 = 4-22 = -18
D2 = 8-22 = -16
D3 = 12-22 = -10
D4 = 16- 22 = -8
Similarly we subtract multiple of 4 by 22 upto 10 terms we get
-18, -14, -10, -8………...18
S.D. = σ2 = ∑(D2)/n
=[ (-18)2 ,(-14)2, (-10)2, (-6)2 + (-2)2 +(6)2 + (10)2 + (14)2 + (18)2]/10
Solving this, we get
σ = 11.5
This is an A.P.
sum=n/2(a+l)
= 10/2(4+40)
∴ sum=220.
Mean, u=sum/n
= 220/10 = 22
D1 = 4-22 = -18
D2 = 8-22 = -16
D3 = 12-22 = -10
D4 = 16- 22 = -8
Similarly we subtract multiple of 4 by 22 upto 10 terms we get
-18, -14, -10, -8………...18
S.D. = σ2 = ∑(D2)/n
=[ (-18)2 ,(-14)2, (-10)2, (-6)2 + (-2)2 +(6)2 + (10)2 + (14)2 + (18)2]/10
Solving this, we get
σ = 11.5
The H.M. of 4,8,16 is- a)6.7
- b)7.8
- c)6.85
- d)6.4
Correct answer is option 'C'. Can you explain this answer?
The H.M. of 4,8,16 is
a)
6.7
b)
7.8
c)
6.85
d)
6.4
|
|
Vivek answered |
HM = 3*(1/4 + 1/8 + 1/16)-¹
= 6.85
KEY POINT →→ HM of n terms = n*[1/a + 1/b + (upto
n terms) ]-¹
For a given data, the variance is 15. If each observation is multiplied by 2, what is the new variance of the resulting observations?- a)15
- b)60
- c)30
- d)7.5
Correct answer is option 'B'. Can you explain this answer?
For a given data, the variance is 15. If each observation is multiplied by 2, what is the new variance of the resulting observations?
a)
15
b)
60
c)
30
d)
7.5
|
|
Poonam Reddy answered |
Variance = 15
New variance = 22*15
= 4*15
= 60
New variance = 22*15
= 4*15
= 60
If the coefficient of variation between x and y is 0.28, covariance between x and y is 7.6, and the variance of x is 9, then the S.D. of the y series is- a)10.05
- b)10.1
- c)9.05
- d)9.8
Correct answer is option 'C'. Can you explain this answer?
If the coefficient of variation between x and y is 0.28, covariance between x and y is 7.6, and the variance of x is 9, then the S.D. of the y series is
a)
10.05
b)
10.1
c)
9.05
d)
9.8
|
|
Hansa Sharma answered |
Correlation coefficient = cov (x,y)/ (std deviation (x) ×std deviation (y))
Correlation coefficient = 0.28
cov (x,y) = 7.6
variance of x is 9.
=> std deviation (x) = √variance of X = √9 = 3
=> 0.28 = 7.6 / ( 3 * std deviation (y))
=> std deviation (y) = 7.6 / ( 3 * 0.28)
=> std deviation (y) = 9.05
standard deviation of Y series = 9.05
Correlation coefficient = 0.28
cov (x,y) = 7.6
variance of x is 9.
=> std deviation (x) = √variance of X = √9 = 3
=> 0.28 = 7.6 / ( 3 * std deviation (y))
=> std deviation (y) = 7.6 / ( 3 * 0.28)
=> std deviation (y) = 9.05
standard deviation of Y series = 9.05
Mean of the squares of the deviations from mean is called the:- a)Mode
- b)Standard deviation
- c)Variance
- d)Quartile deviation
Correct answer is option 'C'. Can you explain this answer?
Mean of the squares of the deviations from mean is called the:
a)
Mode
b)
Standard deviation
c)
Variance
d)
Quartile deviation
|
|
Jyoti Kapoor answered |
Squared deviations from the mean Squared deviations from the mean (SDM) are involved in various calculations. In probability theory and statistics, the definition of variance is either the expected value of the SDM (when considering a theoretical distribution) or its average value (for actual experimental data).
The S.D. of the observations 22,26,28,20,24,30 is- a)3
- b)2.4
- c)3.42
- d)2
Correct answer is option 'C'. Can you explain this answer?
The S.D. of the observations 22,26,28,20,24,30 is
a)
3
b)
2.4
c)
3.42
d)
2
|
|
Lavanya Menon answered |
μ = (22 + 26 + 28 + 20 + 24 + 30)/6
= 150/6
= 25
x(i) = (xi - μ)2
x(22) = (22-25)2 = 9
x(26) = (26-25)2 = 1
x(28) = (28-25)2 = 9
x(20) = (20-25)2 = 25
x(24) = (24-25)2 = 1
x(30) = (30-25)2 = 25
(xi - μ)2 = 70
Standard deviation : [(xi - μ)2]/N
= (70/6)½
= 3.42
= 150/6
= 25
x(i) = (xi - μ)2
x(22) = (22-25)2 = 9
x(26) = (26-25)2 = 1
x(28) = (28-25)2 = 9
x(20) = (20-25)2 = 25
x(24) = (24-25)2 = 1
x(30) = (30-25)2 = 25
(xi - μ)2 = 70
Standard deviation : [(xi - μ)2]/N
= (70/6)½
= 3.42
If in moderately asymmetrical distribution mode and mean of the data are 6 μ and 9 μ respectively, then median is- a)8 μ
- b)6 μ
- c)5 μ
- d)7 μ
Correct answer is option 'A'. Can you explain this answer?
If in moderately asymmetrical distribution mode and mean of the data are 6 μ and 9 μ respectively, then median is
a)
8 μ
b)
6 μ
c)
5 μ
d)
7 μ
|
|
Ananya Das answered |
Median = [mode + 2(mean)]/3
= [6μ+2(9μ)]/3
= 24μ/3
= 8μ
= [6μ+2(9μ)]/3
= 24μ/3
= 8μ
A man tavels at a speed of 20 km/hour and then return at a speed of 30 km/hour. His average speed of the whole journey is- a)24 km/hour
- b)26 km/hour
- c)25 km/hour
- d)24.5 km/hour
Correct answer is option 'A'. Can you explain this answer?
A man tavels at a speed of 20 km/hour and then return at a speed of 30 km/hour. His average speed of the whole journey is
a)
24 km/hour
b)
26 km/hour
c)
25 km/hour
d)
24.5 km/hour
|
|
Nabanita Bajaj answered |
Solution:
The average speed of the whole journey is given by the formula:
Average speed = Total distance / Total time
Let's assume that the distance travelled by the man in one direction is 'd' km.
Therefore, the total distance travelled by the man in the whole journey is 2d km.
Let's also assume that the time taken by the man to travel 'd' km at a speed of 20 km/hour is 't1' hours.
Therefore, the time taken by the man to travel 'd' km at a speed of 30 km/hour is 't2' hours.
Using the formula:
Time = Distance / Speed
We can write:
t1 = d / 20 and t2 = d / 30
Therefore, the total time taken by the man in the whole journey is:
Total time = t1 + t2 = d / 20 + d / 30 = (3d + 2d) / (60) = 5d / 60 = d / 12 hours
Using the formula for average speed, we get:
Average speed = Total distance / Total time
= 2d / (d / 12)
= 24 km/hour
Therefore, the correct answer is option 'A' - 24 km/hour.
The average speed of the whole journey is given by the formula:
Average speed = Total distance / Total time
Let's assume that the distance travelled by the man in one direction is 'd' km.
Therefore, the total distance travelled by the man in the whole journey is 2d km.
Let's also assume that the time taken by the man to travel 'd' km at a speed of 20 km/hour is 't1' hours.
Therefore, the time taken by the man to travel 'd' km at a speed of 30 km/hour is 't2' hours.
Using the formula:
Time = Distance / Speed
We can write:
t1 = d / 20 and t2 = d / 30
Therefore, the total time taken by the man in the whole journey is:
Total time = t1 + t2 = d / 20 + d / 30 = (3d + 2d) / (60) = 5d / 60 = d / 12 hours
Using the formula for average speed, we get:
Average speed = Total distance / Total time
= 2d / (d / 12)
= 24 km/hour
Therefore, the correct answer is option 'A' - 24 km/hour.
A batsman scores runs in 10 innings 38,70,48,34,42,55,63,46,54 and 44 , then the mean deviation is- a)6.4
- b)9.6
- c)10.6
- d)8.6
Correct answer is option 'D'. Can you explain this answer?
A batsman scores runs in 10 innings 38,70,48,34,42,55,63,46,54 and 44 , then the mean deviation is
a)
6.4
b)
9.6
c)
10.6
d)
8.6
|
|
Jyoti Sengupta answered |
Arranging the given data in ascending order,
we have 34, 38, 42, 44, 46, 48, 54, 55, 63, 70
Here, Median M = (46+48)/2
=47
(∵ n = 10, median is the mean of 5th and 6th items)
∴ Mean deviation = ∑|xi−M|/n
=∑|xi−47|/10
= (13+9+5+3+1+1+7+8+16+23)/10
=8.6
we have 34, 38, 42, 44, 46, 48, 54, 55, 63, 70
Here, Median M = (46+48)/2
=47
(∵ n = 10, median is the mean of 5th and 6th items)
∴ Mean deviation = ∑|xi−M|/n
=∑|xi−47|/10
= (13+9+5+3+1+1+7+8+16+23)/10
=8.6
If the mean of the squares of first n natural numbers be 11, then n is equal to- a)13
- b)5
- c)- 13/2
- d)11
Correct answer is option 'B'. Can you explain this answer?
If the mean of the squares of first n natural numbers be 11, then n is equal to
a)
13
b)
5
c)
- 13/2
d)
11
|
|
Tarun Roy answered |
**Given information:**
The mean of the squares of the first n natural numbers is 11.
**To find:**
The value of n.
**Solution:**
Let's first calculate the sum of the squares of the first n natural numbers.
The sum of the squares of the first n natural numbers can be calculated using the formula:
Sum = n(n+1)(2n+1)/6
Now, we are given that the mean of these squares is 11.
Mean = Sum/n
Substituting the value of Sum, we get:
11 = n(n+1)(2n+1)/6n
Simplifying further, we get:
11 = (n+1)(2n+1)/6
Multiplying both sides by 6, we get:
66 = (n+1)(2n+1)
Expanding the equation, we get:
66 = 2n^2 + 3n + n + 1
Rearranging the equation, we get:
2n^2 + 4n - 65 = 0
Now, we can solve this quadratic equation for n using factorization or the quadratic formula.
By factoring, we can write:
2n^2 + 4n - 65 = 0
(n + 13)(2n - 5) = 0
So, n can be either -13/2 or 5.
Since n represents the number of natural numbers, it cannot be a negative or fractional value. Therefore, the only valid solution is n = 5.
Hence, the correct answer is option B.
The mean of the squares of the first n natural numbers is 11.
**To find:**
The value of n.
**Solution:**
Let's first calculate the sum of the squares of the first n natural numbers.
The sum of the squares of the first n natural numbers can be calculated using the formula:
Sum = n(n+1)(2n+1)/6
Now, we are given that the mean of these squares is 11.
Mean = Sum/n
Substituting the value of Sum, we get:
11 = n(n+1)(2n+1)/6n
Simplifying further, we get:
11 = (n+1)(2n+1)/6
Multiplying both sides by 6, we get:
66 = (n+1)(2n+1)
Expanding the equation, we get:
66 = 2n^2 + 3n + n + 1
Rearranging the equation, we get:
2n^2 + 4n - 65 = 0
Now, we can solve this quadratic equation for n using factorization or the quadratic formula.
By factoring, we can write:
2n^2 + 4n - 65 = 0
(n + 13)(2n - 5) = 0
So, n can be either -13/2 or 5.
Since n represents the number of natural numbers, it cannot be a negative or fractional value. Therefore, the only valid solution is n = 5.
Hence, the correct answer is option B.
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below: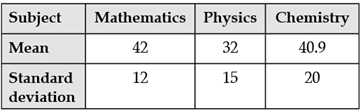
 Q. What is the coefficient of variation of marks obtained by the students in Mathematics?
Q. What is the coefficient of variation of marks obtained by the students in Mathematics?- a)28.57
- b)46.87
- c)48.89
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.
The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below:


Q. What is the coefficient of variation of marks obtained by the students in Mathematics?
a)
28.57
b)
46.87
c)
48.89
d)
none of these

|
Learners Habitat answered |
Standard deviation of Mathematics = 12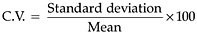
The coefficient of variation,
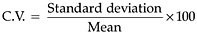
C.V. (in Mathematics) = 12/42 x 100 = 28.57
If the mean of the first n odd natural numbers be n itself, then n is equal to- a)3
- b)any natural number
- c)2
- d)1
Correct answer is option 'B'. Can you explain this answer?
If the mean of the first n odd natural numbers be n itself, then n is equal to
a)
3
b)
any natural number
c)
2
d)
1
|
|
Ujwal Ghosh answered |
The Mean of the First n Odd Natural Numbers
To solve this problem, we need to find the value of n for which the mean of the first n odd natural numbers is equal to n itself.
Understanding the Mean
The mean of a set of numbers is calculated by adding up all the numbers in the set and then dividing the sum by the total number of values in the set.
Calculating the Mean of Odd Natural Numbers
The first n odd natural numbers can be written as 1, 3, 5, 7, ..., (2n-1). To find their mean, we add up all these numbers and divide by n.
Sum of the first n odd natural numbers = 1 + 3 + 5 + 7 + ... + (2n-1)
To calculate the sum of an arithmetic series, we use the formula: Sn = (n/2)(a + l), where Sn is the sum of the series, n is the number of terms, a is the first term, and l is the last term.
In this case, the first term is 1 and the last term is (2n-1). So, the sum of the first n odd natural numbers can be represented as:
Sn = (n/2)(1 + (2n-1))
Simplifying the expression:
Sn = (n/2)(1 + 2n - 1)
= (n/2)(2n)
= n^2
The mean of the first n odd natural numbers is given by:
Mean = Sn/n = n^2/n = n
Determining the Value of n
According to the given condition, the mean of the first n odd natural numbers is equal to n itself. Therefore, we have:
n = n
This equation holds true for all natural numbers. Hence, the correct answer is option B - any natural number.
Conclusion
The mean of the first n odd natural numbers is equal to n itself for any natural number. This can be mathematically proven by calculating the sum of the series and dividing it by n. Therefore, the correct answer to the given question is option B.
To solve this problem, we need to find the value of n for which the mean of the first n odd natural numbers is equal to n itself.
Understanding the Mean
The mean of a set of numbers is calculated by adding up all the numbers in the set and then dividing the sum by the total number of values in the set.
Calculating the Mean of Odd Natural Numbers
The first n odd natural numbers can be written as 1, 3, 5, 7, ..., (2n-1). To find their mean, we add up all these numbers and divide by n.
Sum of the first n odd natural numbers = 1 + 3 + 5 + 7 + ... + (2n-1)
To calculate the sum of an arithmetic series, we use the formula: Sn = (n/2)(a + l), where Sn is the sum of the series, n is the number of terms, a is the first term, and l is the last term.
In this case, the first term is 1 and the last term is (2n-1). So, the sum of the first n odd natural numbers can be represented as:
Sn = (n/2)(1 + (2n-1))
Simplifying the expression:
Sn = (n/2)(1 + 2n - 1)
= (n/2)(2n)
= n^2
The mean of the first n odd natural numbers is given by:
Mean = Sn/n = n^2/n = n
Determining the Value of n
According to the given condition, the mean of the first n odd natural numbers is equal to n itself. Therefore, we have:
n = n
This equation holds true for all natural numbers. Hence, the correct answer is option B - any natural number.
Conclusion
The mean of the first n odd natural numbers is equal to n itself for any natural number. This can be mathematically proven by calculating the sum of the series and dividing it by n. Therefore, the correct answer to the given question is option B.
If the median = (mode + 2 mean) μ, then μ is equal to- a)3
- b)1/3
- c)2/3
- d)2
Correct answer is option 'B'. Can you explain this answer?
If the median = (mode + 2 mean) μ, then μ is equal to
a)
3
b)
1/3
c)
2/3
d)
2
|
|
Muskaan Singh answered |
If the median equals the mode plus twice the mean, we can express this relationship mathematically as:
Median = Mode + 2 * Mean
Median = Mode + 2 * Mean
Coefficient of correlation between the observations (1, 6) , (2 , 5) , (3 , 4) , (4 , 3) , (5 , 2) , (6 , 1) is- a)0
- b)1
- c)-1
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
Coefficient of correlation between the observations (1, 6) , (2 , 5) , (3 , 4) , (4 , 3) , (5 , 2) , (6 , 1) is
a)
0
b)
1
c)
-1
d)
none of these
|
|
Shanaya Shah answered |
Given observations: (1, 6), (2, 5), (3, 4), (4, 3), (5, 2), (6, 1)
To find the coefficient of correlation, we need to first calculate the mean and standard deviation of both the variables.
Let X be the first variable and Y be the second variable.
Calculating mean:
- Mean of X = (1+2+3+4+5+6)/6 = 3.5
- Mean of Y = (6+5+4+3+2+1)/6 = 3.5
Calculating standard deviation:
- Standard deviation of X = sqrt((1-3.5)^2 + (2-3.5)^2 + (3-3.5)^2 + (4-3.5)^2 + (5-3.5)^2 + (6-3.5)^2) = 1.87
- Standard deviation of Y = sqrt((6-3.5)^2 + (5-3.5)^2 + (4-3.5)^2 + (3-3.5)^2 + (2-3.5)^2 + (1-3.5)^2) = 1.87
Calculating the coefficient of correlation using the formula:
r = Σ((Xi - X̄)(Yi - Ȳ))/sqrt(Σ(Xi - X̄)^2 * Σ(Yi - Ȳ)^2)
Substituting the values,
r = ((-2.5)*4.5 + (-1.5)*2.5 + (-0.5)*0.5 + 0.5*2.5 + 1.5*4.5 + 2.5*6.5)/(1.87^2 * 1.87^2 * 6)
r = -1
Therefore, the coefficient of correlation between the given observations is -1, which indicates a perfect negative correlation.
To find the coefficient of correlation, we need to first calculate the mean and standard deviation of both the variables.
Let X be the first variable and Y be the second variable.
Calculating mean:
- Mean of X = (1+2+3+4+5+6)/6 = 3.5
- Mean of Y = (6+5+4+3+2+1)/6 = 3.5
Calculating standard deviation:
- Standard deviation of X = sqrt((1-3.5)^2 + (2-3.5)^2 + (3-3.5)^2 + (4-3.5)^2 + (5-3.5)^2 + (6-3.5)^2) = 1.87
- Standard deviation of Y = sqrt((6-3.5)^2 + (5-3.5)^2 + (4-3.5)^2 + (3-3.5)^2 + (2-3.5)^2 + (1-3.5)^2) = 1.87
Calculating the coefficient of correlation using the formula:
r = Σ((Xi - X̄)(Yi - Ȳ))/sqrt(Σ(Xi - X̄)^2 * Σ(Yi - Ȳ)^2)
Substituting the values,
r = ((-2.5)*4.5 + (-1.5)*2.5 + (-0.5)*0.5 + 0.5*2.5 + 1.5*4.5 + 2.5*6.5)/(1.87^2 * 1.87^2 * 6)
r = -1
Therefore, the coefficient of correlation between the given observations is -1, which indicates a perfect negative correlation.
The mean weight of a group of 10 items is 28 and that of another group of n items is 35.The mean of combined group of 10 + n items is found to be 30. The value of n is- a)12
- b)10
- c)4
- d)2
Correct answer is option 'C'. Can you explain this answer?
The mean weight of a group of 10 items is 28 and that of another group of n items is 35.The mean of combined group of 10 + n items is found to be 30. The value of n is
a)
12
b)
10
c)
4
d)
2

|
Sahana Joshi answered |
sum of weights of 10 items = 280
sum of weights of n items = 35n
so, sum of weights of (10 + n) items = 280 + 35n
so ,mean = (280 + 35 n) / (10 + n)
30(10 + n) = 280 + 35n
solving we get, n = 4
sum of weights of n items = 35n
so, sum of weights of (10 + n) items = 280 + 35n
so ,mean = (280 + 35 n) / (10 + n)
30(10 + n) = 280 + 35n
solving we get, n = 4
If the two lines of regression are at right angles, then ρ(X,Y) is equal to- a)- 1
- b)0
- c)1
- d)1 or - 1
Correct answer is option 'B'. Can you explain this answer?
If the two lines of regression are at right angles, then ρ(X,Y) is equal to
a)
- 1
b)
0
c)
1
d)
1 or - 1
|
|
Nabanita Bajaj answered |
Understanding Regression Lines and Correlation
When two lines of regression are at right angles, it provides critical information about the relationship between the variables X and Y.
Correlation Coefficient (ρ)
- The correlation coefficient (ρ) quantifies the degree of linear relationship between two variables.
- Its value ranges from -1 to +1:
- ρ = 1 indicates a perfect positive correlation.
- ρ = -1 indicates a perfect negative correlation.
- ρ = 0 indicates no correlation.
Right Angles and Independence
- Lines of regression are perpendicular (at right angles) when the product of their slopes equals -1.
- This condition signifies that changes in one variable do not predict changes in the other, indicating independence.
Conclusion: The Value of ρ
- When the lines of regression are at right angles, it implies that:
- There is no linear relationship between X and Y.
- Thus, ρ must equal 0.
This leads us to conclude that the correct answer to the question is option 'B', which states that ρ(X,Y) is equal to 0.
When two lines of regression are at right angles, it provides critical information about the relationship between the variables X and Y.
Correlation Coefficient (ρ)
- The correlation coefficient (ρ) quantifies the degree of linear relationship between two variables.
- Its value ranges from -1 to +1:
- ρ = 1 indicates a perfect positive correlation.
- ρ = -1 indicates a perfect negative correlation.
- ρ = 0 indicates no correlation.
Right Angles and Independence
- Lines of regression are perpendicular (at right angles) when the product of their slopes equals -1.
- This condition signifies that changes in one variable do not predict changes in the other, indicating independence.
Conclusion: The Value of ρ
- When the lines of regression are at right angles, it implies that:
- There is no linear relationship between X and Y.
- Thus, ρ must equal 0.
This leads us to conclude that the correct answer to the question is option 'B', which states that ρ(X,Y) is equal to 0.
For a symmetrical distribution Q1 = 20 and Q3. = 40. The median of the data is- a)10
- b)40
- c)20
- d)30
Correct answer is option 'D'. Can you explain this answer?
For a symmetrical distribution Q1 = 20 and Q3. = 40. The median of the data is
a)
10
b)
40
c)
20
d)
30
|
|
Gauri Rane answered |
Understanding Symmetrical Distribution
In a symmetrical distribution, the data is evenly distributed around its central point, which is the median. The key characteristics of this distribution are:
- The mean, median, and mode are all equal.
- The quartiles (Q1 and Q3) divide the data into segments.
Given Values
- Q1 (First Quartile) = 20
- Q3 (Third Quartile) = 40
In a symmetrical distribution, the median can be found using the following relationship between quartiles:
Median Calculation
- The median lies exactly halfway between Q1 and Q3 in a symmetrical distribution.
- To find the median, you can use the formula:
Median = (Q1 + Q3) / 2
Substituting the given values:
- Median = (20 + 40) / 2
- Median = 60 / 2
- Median = 30
Conclusion
Thus, the median of the data is 30, which corresponds to option 'D'. This result aligns with the principles of symmetrical distributions, confirming that the data is balanced around this central point.
In a symmetrical distribution, the data is evenly distributed around its central point, which is the median. The key characteristics of this distribution are:
- The mean, median, and mode are all equal.
- The quartiles (Q1 and Q3) divide the data into segments.
Given Values
- Q1 (First Quartile) = 20
- Q3 (Third Quartile) = 40
In a symmetrical distribution, the median can be found using the following relationship between quartiles:
Median Calculation
- The median lies exactly halfway between Q1 and Q3 in a symmetrical distribution.
- To find the median, you can use the formula:
Median = (Q1 + Q3) / 2
Substituting the given values:
- Median = (20 + 40) / 2
- Median = 60 / 2
- Median = 30
Conclusion
Thus, the median of the data is 30, which corresponds to option 'D'. This result aligns with the principles of symmetrical distributions, confirming that the data is balanced around this central point.
If the mean of 3,4,x,7,10, is 6, then the value of x is- a)7
- b)5
- c)4
- d)6
Correct answer is option 'D'. Can you explain this answer?
If the mean of 3,4,x,7,10, is 6, then the value of x is
a)
7
b)
5
c)
4
d)
6
|
|
Tanishq Singh answered |
Question:
If the mean of 3, 4, x, 7, 10 is 6, then the value of x is
Answer:
To find the value of x, we need to use the formula for the mean, which is the sum of all the numbers divided by the total number of numbers.
The formula for the mean is:
mean = (3 + 4 + x + 7 + 10)/5
Given that the mean is 6, we can substitute this value into the formula and solve for x:
6 = (3 + 4 + x + 7 + 10)/5
Simplifying the equation:
To simplify the equation, we can start by multiplying both sides of the equation by 5 to eliminate the fraction:
6 * 5 = (3 + 4 + x + 7 + 10)
30 = 3 + 4 + x + 7 + 10
Now, we can combine like terms on the right side of the equation:
30 = 24 + x
Next, we can isolate the variable x by subtracting 24 from both sides of the equation:
30 - 24 = x
6 = x
Conclusion:
Therefore, the value of x is 6. Hence, the correct answer is option D.
If the mean of 3, 4, x, 7, 10 is 6, then the value of x is
Answer:
To find the value of x, we need to use the formula for the mean, which is the sum of all the numbers divided by the total number of numbers.
The formula for the mean is:
mean = (3 + 4 + x + 7 + 10)/5
Given that the mean is 6, we can substitute this value into the formula and solve for x:
6 = (3 + 4 + x + 7 + 10)/5
Simplifying the equation:
To simplify the equation, we can start by multiplying both sides of the equation by 5 to eliminate the fraction:
6 * 5 = (3 + 4 + x + 7 + 10)
30 = 3 + 4 + x + 7 + 10
Now, we can combine like terms on the right side of the equation:
30 = 24 + x
Next, we can isolate the variable x by subtracting 24 from both sides of the equation:
30 - 24 = x
6 = x
Conclusion:
Therefore, the value of x is 6. Hence, the correct answer is option D.
For a moderately skewed distribution, quartile deviation and the standard deviation are related by- a)S.D. = 3/2 Q.D.
- b)S.D = 3/4 Q.D.
- c)S.D. = 4/3 Q.D.
- d)S.D. = 2/3 Q.D.
Correct answer is option 'A'. Can you explain this answer?
For a moderately skewed distribution, quartile deviation and the standard deviation are related by
a)
S.D. = 3/2 Q.D.
b)
S.D = 3/4 Q.D.
c)
S.D. = 4/3 Q.D.
d)
S.D. = 2/3 Q.D.
|
|
Sushant Chaudhary answered |
The relationship between the mean, quartile and the standard deviation are as follows:
Mean Deviation is the mean of all the absolute deviations of a set of data.
Quartile deviation is the difference between “first and third quartiles” in any distribution.
Standard deviation measures the “dispersion of the data set” that is relative to its mean.
Mean Deviation = 4/5 × Quartile deviation
Standard Deviation = 3/2 × Quartile deviation
Mean Deviation is the mean of all the absolute deviations of a set of data.
Quartile deviation is the difference between “first and third quartiles” in any distribution.
Standard deviation measures the “dispersion of the data set” that is relative to its mean.
Mean Deviation = 4/5 × Quartile deviation
Standard Deviation = 3/2 × Quartile deviation
If the two lines of regression of a bivariate distribution coincide, then the correlation coefficient ρρsatisfies.- a)ρ = 0
- b)ρ > 0
- c)ρ = 1 or −1
- d)ρ < 0
Correct answer is option 'C'. Can you explain this answer?
If the two lines of regression of a bivariate distribution coincide, then the correlation coefficient ρρsatisfies.
a)
ρ = 0
b)
ρ > 0
c)
ρ = 1 or −1
d)
ρ < 0
|
|
Aman Chauhan answered |
If the two lines of regression of a bivariate distribution coincide, then the correlation coefficient is +1 or -1, indicating a perfect linear relationship between the two variables.
Mean deviation from the mean for the observations – 1, 0 ,4 is- a)2/3
- b)3/2
- c)2
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
Mean deviation from the mean for the observations – 1, 0 ,4 is
a)
2/3
b)
3/2
c)
2
d)
none of these
|
|
Bhargavi Bose answered |
To calculate the mean deviation from the mean for a set of observations, follow these steps:
1. Calculate the mean of the observations by adding all the values together and dividing by the total number of observations.
2. Subtract the mean from each individual observation to find the deviation from the mean.
3. Take the absolute value of each deviation to ensure that negative values do not cancel out positive values.
4. Add up all the absolute deviations.
5. Divide the sum of the absolute deviations by the total number of observations to find the mean deviation from the mean.
Here is an example:
Observations: 5, 7, 9, 12, 15
1. Mean = (5 + 7 + 9 + 12 + 15) / 5 = 9.6
2. Deviations from the mean: -4.6, -2.6, -0.6, 2.4, 5.4
3. Absolute deviations: 4.6, 2.6, 0.6, 2.4, 5.4
4. Sum of absolute deviations: 15.6
5. Mean deviation from the mean = 15.6 / 5 = 3.12
Therefore, the mean deviation from the mean for these observations is 3.12.
1. Calculate the mean of the observations by adding all the values together and dividing by the total number of observations.
2. Subtract the mean from each individual observation to find the deviation from the mean.
3. Take the absolute value of each deviation to ensure that negative values do not cancel out positive values.
4. Add up all the absolute deviations.
5. Divide the sum of the absolute deviations by the total number of observations to find the mean deviation from the mean.
Here is an example:
Observations: 5, 7, 9, 12, 15
1. Mean = (5 + 7 + 9 + 12 + 15) / 5 = 9.6
2. Deviations from the mean: -4.6, -2.6, -0.6, 2.4, 5.4
3. Absolute deviations: 4.6, 2.6, 0.6, 2.4, 5.4
4. Sum of absolute deviations: 15.6
5. Mean deviation from the mean = 15.6 / 5 = 3.12
Therefore, the mean deviation from the mean for these observations is 3.12.
The Q.D. of the daily wages (in Rs) of 7 persons given below: 12,7,15,10,17,19,25 is- a)4.5
- b)9
- c)5
- d)14.5
Correct answer is option 'A'. Can you explain this answer?
The Q.D. of the daily wages (in Rs) of 7 persons given below: 12,7,15,10,17,19,25 is
a)
4.5
b)
9
c)
5
d)
14.5
|
|
Arshiya Shah answered |
Q.D. of Daily Wages of 7 Persons
To find the Q.D. (Quartile Deviation) of the daily wages of 7 persons given below: 12, 7, 15, 10, 17, 19, 25, we need to follow the steps given below:
Step 1: Arrange the data in ascending order.
7, 10, 12, 15, 17, 19, 25
Step 2: Find the median or the second quartile (Q2) of the data.
Median of the data = (n + 1)/2-th value = (7 + 1)/2-th value = 4th value
Hence, median = 15
Step 3: Find the first quartile (Q1) of the data.
Q1 = (n + 1)/4-th value = (7 + 1)/4-th value = 2nd value
Hence, Q1 = 10
Step 4: Find the third quartile (Q3) of the data.
Q3 = 3(n + 1)/4-th value = 3(7 + 1)/4-th value = 6th value
Hence, Q3 = 19
Step 5: Calculate the Quartile Deviation (Q.D.) using the formula:
Q.D. = (Q3 - Q1)/2
Substituting the values of Q1 and Q3, we get:
Q.D. = (19 - 10)/2 = 4.5
Therefore, the Q.D. of the daily wages of 7 persons given is 4.5.
Note: Quartile deviation is a measure of dispersion that gives an idea about the spread of data around the median. It is calculated as half of the difference between the third and first quartiles.
To find the Q.D. (Quartile Deviation) of the daily wages of 7 persons given below: 12, 7, 15, 10, 17, 19, 25, we need to follow the steps given below:
Step 1: Arrange the data in ascending order.
7, 10, 12, 15, 17, 19, 25
Step 2: Find the median or the second quartile (Q2) of the data.
Median of the data = (n + 1)/2-th value = (7 + 1)/2-th value = 4th value
Hence, median = 15
Step 3: Find the first quartile (Q1) of the data.
Q1 = (n + 1)/4-th value = (7 + 1)/4-th value = 2nd value
Hence, Q1 = 10
Step 4: Find the third quartile (Q3) of the data.
Q3 = 3(n + 1)/4-th value = 3(7 + 1)/4-th value = 6th value
Hence, Q3 = 19
Step 5: Calculate the Quartile Deviation (Q.D.) using the formula:
Q.D. = (Q3 - Q1)/2
Substituting the values of Q1 and Q3, we get:
Q.D. = (19 - 10)/2 = 4.5
Therefore, the Q.D. of the daily wages of 7 persons given is 4.5.
Note: Quartile deviation is a measure of dispersion that gives an idea about the spread of data around the median. It is calculated as half of the difference between the third and first quartiles.
The standard deviation for the following data:
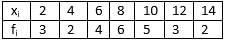
- a)5
- b)4.21
- c)3.12
- d)None of the above
Correct answer is option 'C'. Can you explain this answer?
The standard deviation for the following data:

a)
5
b)
4.21
c)
3.12
d)
None of the above
|
|
Neha Sharma answered |
Answer: C
Solution: Variance= [summation (y^2×f) /N] -[ summation (yf) /N]^2
=(296/25) -(0/25) ^2
=11.84
standard deviation=√11.84=3.12
Solution: Variance= [summation (y^2×f) /N] -[ summation (yf) /N]^2
=(296/25) -(0/25) ^2
=11.84
standard deviation=√11.84=3.12
The mean deviation about the mean for the following data: 6, 7, 10, 12, 13, 4, 8, 12.- a)9.2
- b)1.54
- c)1.89
- d)2.75
Correct answer is option 'D'. Can you explain this answer?
The mean deviation about the mean for the following data: 6, 7, 10, 12, 13, 4, 8, 12.
a)
9.2
b)
1.54
c)
1.89
d)
2.75

|
Jatin Sharma answered |
Calculating the Mean Deviation about the Mean:
To calculate the mean deviation about the mean, we first need to find the mean of the given data set.
Finding the Mean:
First, add up all the numbers in the data set:
6 + 7 + 10 + 12 + 13 + 4 + 8 + 12 = 72
Next, divide the sum by the total number of values in the data set:
72 / 8 = 9
So, the mean of the data set is 9.
Calculating the Mean Deviation:
Next, we find the deviation of each number from the mean:
|6-9| = 3
|7-9| = 2
|10-9| = 1
|12-9| = 3
|13-9| = 4
|4-9| = 5
|8-9| = 1
|12-9| = 3
Now, we calculate the mean deviation by finding the average of these deviations:
(3 + 2 + 1 + 3 + 4 + 5 + 1 + 3) / 8 = 22 / 8 = 2.75
Therefore, the correct answer is option D) 2.75.
To calculate the mean deviation about the mean, we first need to find the mean of the given data set.
Finding the Mean:
First, add up all the numbers in the data set:
6 + 7 + 10 + 12 + 13 + 4 + 8 + 12 = 72
Next, divide the sum by the total number of values in the data set:
72 / 8 = 9
So, the mean of the data set is 9.
Calculating the Mean Deviation:
Next, we find the deviation of each number from the mean:
|6-9| = 3
|7-9| = 2
|10-9| = 1
|12-9| = 3
|13-9| = 4
|4-9| = 5
|8-9| = 1
|12-9| = 3
Now, we calculate the mean deviation by finding the average of these deviations:
(3 + 2 + 1 + 3 + 4 + 5 + 1 + 3) / 8 = 22 / 8 = 2.75
Therefore, the correct answer is option D) 2.75.
If x and y are related as y – 4x = 3, then the nature of correlation between x and y is- a)perfect negative
- b)no correlation
- c)perfect positive
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
If x and y are related as y – 4x = 3, then the nature of correlation between x and y is
a)
perfect negative
b)
no correlation
c)
perfect positive
d)
none of these
|
|
Rohan Singh answered |
Positive correlation is a relationship between two variables in which both variables move in tandem. A positive correlation exists when one variable decreases as the other variable decreases, or one variable increases while the other increases.
Quartile deviation is nearly equal to- a)3/2 σ
- b)2 σ
- c)2/3 σ
- d)3 σ
Correct answer is option 'C'. Can you explain this answer?
Quartile deviation is nearly equal to
a)
3/2 σ
b)
2 σ
c)
2/3 σ
d)
3 σ
|
|
Mehul Rane answered |
It is not accurate to say that quartile deviation is nearly equal to 3/2. Quartile deviation is a measure of dispersion that is calculated as half of the difference between the third and first quartiles. It is equivalent to dividing the interquartile range (IQR) by 2.
The formula for quartile deviation is:
QD = (Q3 - Q1) / 2
where Q3 is the third quartile and Q1 is the first quartile.
The value of quartile deviation depends on the distribution of the data. In some cases, it may be close to 3/2, but in general, it can take on a wide range of values. Therefore, it is not appropriate to make a general statement that quartile deviation is nearly equal to 3/2.
The formula for quartile deviation is:
QD = (Q3 - Q1) / 2
where Q3 is the third quartile and Q1 is the first quartile.
The value of quartile deviation depends on the distribution of the data. In some cases, it may be close to 3/2, but in general, it can take on a wide range of values. Therefore, it is not appropriate to make a general statement that quartile deviation is nearly equal to 3/2.
The arithmetic mean of the numerical values of the deviations of items from some average value is called the- a)Standard deviation
- b)Range
- c)Quartile deviation
- d)Mean deviation
Correct answer is option 'D'. Can you explain this answer?
The arithmetic mean of the numerical values of the deviations of items from some average value is called the
a)
Standard deviation
b)
Range
c)
Quartile deviation
d)
Mean deviation
|
|
Maulik Majumdar answered |
Mean deviation of a data set is the average of the absolute deviations from a central point (Average value).
Least square lines of regression give best possible estimates, when ρ(X,Y) is equal to- a)- 1 or 1
- b)less than 1
- c)greater than -1
- d)1/ 2
Correct answer is option 'A'. Can you explain this answer?
Least square lines of regression give best possible estimates, when ρ(X,Y) is equal to
a)
- 1 or 1
b)
less than 1
c)
greater than -1
d)
1/ 2

|
Learners Habitat answered |
The least square lines of regression provide the best possible estimates when the correlation coefficient ρ(X,Y) is equal to 1 or -1. This is because a correlation of ρ=1 or ρ=−1 indicates a perfect linear relationship between X and Y, meaning one variable can be predicted exactly from the other using the regression line.
The Mode of the following items is 0,1,6,7,2,3,7,6,6,2,6,0,5,6,0.- a)2
- b)5
- c)0
- d)6
Correct answer is option 'D'. Can you explain this answer?
The Mode of the following items is 0,1,6,7,2,3,7,6,6,2,6,0,5,6,0.
a)
2
b)
5
c)
0
d)
6

|
Hafsat Ahmad answered |
The mode is 6 because mode means the most frequent appearing and 6 appears the most
The two lines of regression are x + 4y = 3 and 3x +y = 15. value of x for y = 3 is- a)- 4
- b)4
- c)- 9
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
The two lines of regression are x + 4y = 3 and 3x +y = 15. value of x for y = 3 is
a)
- 4
b)
4
c)
- 9
d)
none of these

|
Pragati Nair answered |
We have x + 4y = 3 and 3x + y = 15 as the lines of regression of y on x and x on y respectively.
Therefore, for y = 3 the required line is x on y
so put y= 3 in 3x + y = 15
we get, x = 4
Therefore, for y = 3 the required line is x on y
so put y= 3 in 3x + y = 15
we get, x = 4
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results: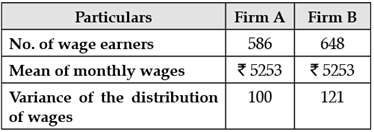
 Q. Coefficient of variation of the distribution of wages for firm A
Q. Coefficient of variation of the distribution of wages for firm A- a)0.15
- b)0.19
- c)0.21
- d)0.24
Correct answer is option 'B'. Can you explain this answer?
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:


Q. Coefficient of variation of the distribution of wages for firm A
a)
0.15
b)
0.19
c)
0.21
d)
0.24
|
|
Raghav Bansal answered |
Variance of distribution of wages,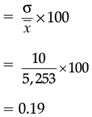
σ2 = 100
Standard deviation, σ = √σ2
= √100
= 10
coefficient of Variation
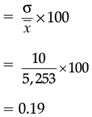
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results: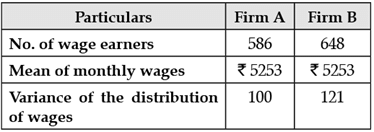
 Q. Which firm A or B shows greater variability in individual wages?
Q. Which firm A or B shows greater variability in individual wages?- a)Firm B
- b)Firm A
- c)Both
- d)Can’t Say
Correct answer is option 'A'. Can you explain this answer?
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:


Q. Which firm A or B shows greater variability in individual wages?
a)
Firm B
b)
Firm A
c)
Both
d)
Can’t Say
|
|
Geetika Shah answered |
Coefficient of variation of wages, of firm A = 0.19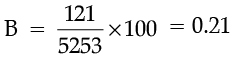
coefficient of variation of wages, of firm
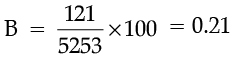
∴ Firm B shows greater variability in individual wages.
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below: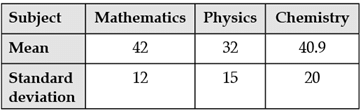
 Q. What is the coefficient of variation of marks obtained by the students in Physics?
Q. What is the coefficient of variation of marks obtained by the students in Physics?- a)28.57
- b)46.87
- c)48.89
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.
The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below:


Q. What is the coefficient of variation of marks obtained by the students in Physics?
a)
28.57
b)
46.87
c)
48.89
d)
none of these
|
|
Tanuja Kapoor answered |
Standard deviation of Physics = 15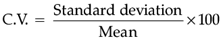
The coefficient of variation,
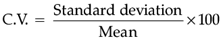
C.V. (in Physics) = 15/32 × 100 = 46.87
For a bivariate frequency distribution byx > 1, then byx is- a)negative
- b)less than 1
- c)lies between 0 and 1
- d)none of these
Correct answer is option 'D'. Can you explain this answer?
For a bivariate frequency distribution byx > 1, then byx is
a)
negative
b)
less than 1
c)
lies between 0 and 1
d)
none of these

|
KP Classes answered |
The regression coefficient b yx is defined as the change in Y per unit change in X. It is related to the correlation coefficient ρ(X,Y) and the regression coefficient b xy through the relationship:
byx⋅bxy=ρ2
Where ρ is the correlation coefficient, and ρ2 lies between 0 and 1.
Key Consideration: If byx >1, it means that the slope of the regression line predicting Y from X is greater than 1. However, b yx can still be positive or negative depending on the direction of the relationship.
The condition b yx >1 implies a steeper slope of the regression line for Y on X. It does not necessarily indicate negativity or positivity, as it depends on the data.
byx⋅bxy=ρ2
Where ρ is the correlation coefficient, and ρ2 lies between 0 and 1.
Key Consideration: If byx >1, it means that the slope of the regression line predicting Y from X is greater than 1. However, b yx can still be positive or negative depending on the direction of the relationship.
The condition b yx >1 implies a steeper slope of the regression line for Y on X. It does not necessarily indicate negativity or positivity, as it depends on the data.
In a group of students, mean weight of boys is 80 kg and mean weight of girls is 50kg.If the mean weight of all the students taken together is 60kg, then the ratio of the number of boys to that of the girls is- a)2 : 1
- b)3 : 2
- c)1 : 2
- d)2 : 3
Correct answer is option 'C'. Can you explain this answer?
In a group of students, mean weight of boys is 80 kg and mean weight of girls is 50kg.If the mean weight of all the students taken together is 60kg, then the ratio of the number of boys to that of the girls is
a)
2 : 1
b)
3 : 2
c)
1 : 2
d)
2 : 3

|
Nipuns Institute answered |
To solve this, let the number of boys be b and the number of girls be g.
Step 1: Use the formula for the combined mean
The formula for the mean of a combined group is:
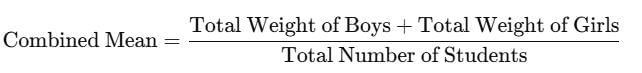
Given: Mean weight of boys = 80kg,
Mean weight of girls = 50kg,
Combined mean = 60kg.
The total weight of boys is 80b, and the total weight of girls is 50g. The total number of students is b+g. Substituting these into the formula:
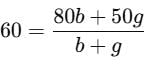
Step 2: Solve for the ratio b/g
Multiply through by b+g to eliminate the denominator:
60(b+g)=80b+50g
Expand and simplify:
60b+60g=80b+50g
60g−50g=80b−60b
10g=20b
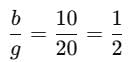
Step 3: Conclusion
The ratio of the number of boys to the number of girls is:
1:2
Step 1: Use the formula for the combined mean
The formula for the mean of a combined group is:
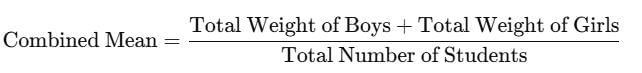
Given: Mean weight of boys = 80kg,
Mean weight of girls = 50kg,
Combined mean = 60kg.
The total weight of boys is 80b, and the total weight of girls is 50g. The total number of students is b+g. Substituting these into the formula:
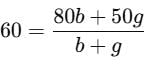
Step 2: Solve for the ratio b/g
Multiply through by b+g to eliminate the denominator:
60(b+g)=80b+50g
Expand and simplify:
60b+60g=80b+50g
60g−50g=80b−60b
10g=20b
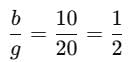
Step 3: Conclusion
The ratio of the number of boys to the number of girls is:
1:2
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below:
 Q. What is the coefficient of variation of marks obtained by the students in Chemistry?
Q. What is the coefficient of variation of marks obtained by the students in Chemistry?- a)28.57
- b)46.87
- c)48.89
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
A teacher conducted a surprise test of Mathematics, Physics and Chemistry for class XI on Monday.
The mean and standard deviation of marks obtained by 50 students of the class in three subjects are given below:


Q. What is the coefficient of variation of marks obtained by the students in Chemistry?
a)
28.57
b)
46.87
c)
48.89
d)
none of these
|
|
Ananya Das answered |
Standard deviation of Chemistry = 20
C.V. (in Chemistry) = 20/40.9 x 100 = 48.89
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:
 Q. Amount paid by firm B is
Q. Amount paid by firm B is- a)3078258
- b)3043944
- c)3708258
- d)3403944
Correct answer is option 'D'. Can you explain this answer?
An analysis of monthly wages paid to workers in two firms A and B, belonging to the same industry, gives the following results:


Q. Amount paid by firm B is
a)
3078258
b)
3043944
c)
3708258
d)
3403944
|
|
Geetika Shah answered |
No. of wage earners = 648
Mean of monthly wages, x = ₹5253
Amount paid by firm B = 648 × 5253
= ₹3403944
S.D. of a data is 6. When each observation is increased by 1, then the S.D. of new data is- a)8
- b)6
- c)5
- d)7
Correct answer is option 'B'. Can you explain this answer?
S.D. of a data is 6. When each observation is increased by 1, then the S.D. of new data is
a)
8
b)
6
c)
5
d)
7
|
|
Disha Bajaj answered |
Given:
Standard deviation (SD) of a data = 6
To find:
SD of the new data when each observation is increased by 1
Solution:
When each observation in a data set is increased by a constant value, the mean of the data set also increases by that constant value. However, the standard deviation remains unchanged.
Explanation:
Let's consider a data set with n observations: {x1, x2, x3, ..., xn}
The mean of this data set is given by:
Mean = (x1 + x2 + x3 + ... + xn) / n
When each observation is increased by 1, the new data set becomes: {x1 + 1, x2 + 1, x3 + 1, ..., xn + 1}
The mean of the new data set is given by:
New Mean = [(x1 + 1) + (x2 + 1) + (x3 + 1) + ... + (xn + 1)] / n
To find the standard deviation, we need to calculate the sum of squares of the differences between each observation and the mean, and then take the square root of the average of these squared differences.
Calculation of Standard Deviation:
SD = sqrt[((x1 - Mean)^2 + (x2 - Mean)^2 + (x3 - Mean)^2 + ... + (xn - Mean)^2) / n]
Similarly, for the new data set:
New SD = sqrt[((x1 + 1 - New Mean)^2 + (x2 + 1 - New Mean)^2 + (x3 + 1 - New Mean)^2 + ... + (xn + 1 - New Mean)^2) / n]
Comparison:
We know that the mean of the new data set is increased by 1 compared to the original mean.
New Mean = Mean + 1
Substituting this into the equation for New SD, we get:
New SD = sqrt[((x1 + 1 - (Mean + 1))^2 + (x2 + 1 - (Mean + 1))^2 + (x3 + 1 - (Mean + 1))^2 + ... + (xn + 1 - (Mean + 1))^2) / n]
= sqrt[((x1 - Mean)^2 + (x2 - Mean)^2 + (x3 - Mean)^2 + ... + (xn - Mean)^2) / n]
= SD
Hence, the standard deviation of the new data set remains unchanged, which is equal to the standard deviation of the original data set.
Therefore, the SD of the new data is 6, which is the same as the original SD.
Hence, option B is the correct answer.
Standard deviation (SD) of a data = 6
To find:
SD of the new data when each observation is increased by 1
Solution:
When each observation in a data set is increased by a constant value, the mean of the data set also increases by that constant value. However, the standard deviation remains unchanged.
Explanation:
Let's consider a data set with n observations: {x1, x2, x3, ..., xn}
The mean of this data set is given by:
Mean = (x1 + x2 + x3 + ... + xn) / n
When each observation is increased by 1, the new data set becomes: {x1 + 1, x2 + 1, x3 + 1, ..., xn + 1}
The mean of the new data set is given by:
New Mean = [(x1 + 1) + (x2 + 1) + (x3 + 1) + ... + (xn + 1)] / n
To find the standard deviation, we need to calculate the sum of squares of the differences between each observation and the mean, and then take the square root of the average of these squared differences.
Calculation of Standard Deviation:
SD = sqrt[((x1 - Mean)^2 + (x2 - Mean)^2 + (x3 - Mean)^2 + ... + (xn - Mean)^2) / n]
Similarly, for the new data set:
New SD = sqrt[((x1 + 1 - New Mean)^2 + (x2 + 1 - New Mean)^2 + (x3 + 1 - New Mean)^2 + ... + (xn + 1 - New Mean)^2) / n]
Comparison:
We know that the mean of the new data set is increased by 1 compared to the original mean.
New Mean = Mean + 1
Substituting this into the equation for New SD, we get:
New SD = sqrt[((x1 + 1 - (Mean + 1))^2 + (x2 + 1 - (Mean + 1))^2 + (x3 + 1 - (Mean + 1))^2 + ... + (xn + 1 - (Mean + 1))^2) / n]
= sqrt[((x1 - Mean)^2 + (x2 - Mean)^2 + (x3 - Mean)^2 + ... + (xn - Mean)^2) / n]
= SD
Hence, the standard deviation of the new data set remains unchanged, which is equal to the standard deviation of the original data set.
Therefore, the SD of the new data is 6, which is the same as the original SD.
Hence, option B is the correct answer.
The mean and S.D. of 1,2,3,4,5,6 is- a)3,3
- b)7/2,√3
- c)7/2,
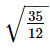
- d)3, 35/12
Correct answer is option 'C'. Can you explain this answer?
The mean and S.D. of 1,2,3,4,5,6 is
a)
3,3
b)
7/2,√3
c)
7/2,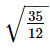
d)
3, 35/12

|
Learners Habitat answered |
We are given the data: 1, 2, 3, 4, 5, 6
Step 1: Calculate the Mean
The formula for the mean is:
Mean = (Sum of all values) / (Number of values)
Substituting the values:
Mean = (1 + 2 + 3 + 4 + 5 + 6) / 6 = 21 / 6 = 7/2
Step 2: Calculate the Variance
The formula for variance is:
Variance = Σ(xi - Mean)² / n
1. Subtract the mean (7/2) from each value and square the result:
- (1 - 7/2)² = (-5/2)² = 25/4
- (2 - 7/2)² = (-3/2)² = 9/4
- (3 - 7/2)² = (-1/2)² = 1/4
- (4 - 7/2)² = (1/2)² = 1/4
- (5 - 7/2)² = (3/2)² = 9/4
- (6 - 7/2)² = (5/2)² = 25/4
2. Sum the squared values:
(25/4) + (9/4) + (1/4) + (1/4) + (9/4) + (25/4) = 70/4 = 35/2
3. Divide by n (6):
Variance = (35/2) / 6 = 35/12
Step 3: Calculate the Standard Deviation
The standard deviation is the square root of the variance:
Standard Deviation = √(35/12)
Final Answer
The mean is 7/2, and the standard deviation is √(35/12).
Correct option: (c) 7/2, √(35/12)
Chapter doubts & questions for Statistics - Mathematics for Grade 12 2025 is part of Grade 12 exam preparation. The chapters have been prepared according to the Grade 12 exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Grade 12 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Statistics - Mathematics for Grade 12 in English & Hindi are available as part of Grade 12 exam.
Download more important topics, notes, lectures and mock test series for Grade 12 Exam by signing up for free.
Mathematics for Grade 12
99 videos|124 docs|82 tests
|
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup