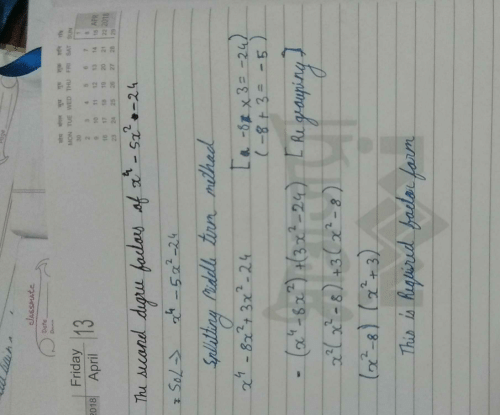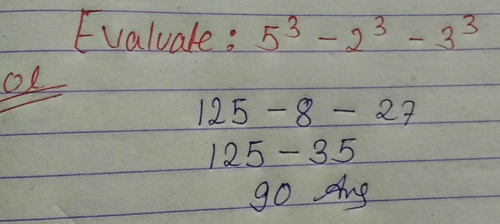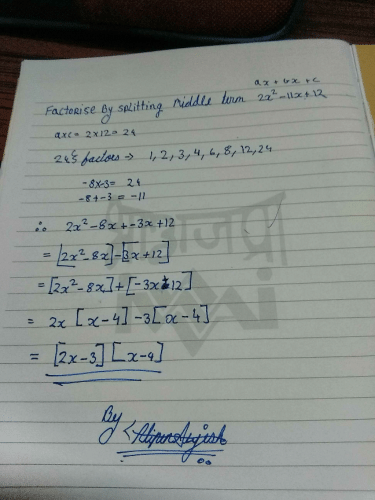All Exams >
Class 9 >
Mathematics (Maths) Class 9 >
All Questions
All questions of Polynomials for Class 9 Exam
The number of zeros of x2 + 4x + 2 - a)1
- b)2
- c)3
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
The number of zeros of x2 + 4x + 2
a)
1
b)
2
c)
3
d)
none of these
|
|
Harshit Agrawal answered |
The given equation has 2 zeros as it has degree of 2
Polynomials having only three terms are called ________- a)monomial
- b)binomial
- c)trinomials
- d)multinomial
Correct answer is option 'C'. Can you explain this answer?
Polynomials having only three terms are called ________
a)
monomial
b)
binomial
c)
trinomials
d)
multinomial
|
Mayank Jaiswal answered |
Polynomials having only three term are called Trinomial.
The degree of the polynomial x4 – 3x3 + 2x2 – 5x + 3 is:- a)2
- b)1
- c)4
- d)3
Correct answer is option 'C'. Can you explain this answer?
The degree of the polynomial x4 – 3x3 + 2x2 – 5x + 3 is:
a)
2
b)
1
c)
4
d)
3
|
Tarun Sengupta answered |
**Explanation:**
The degree of a polynomial is the highest power of the variable in that polynomial. In this case, the polynomial is:
x^4 + 3x^3 + 2x^2 + 5x - 3
To find the degree of this polynomial, we need to identify the term with the highest power of x. Let's break down each term in the polynomial:
- The term x^4 has a power of 4.
- The term 3x^3 has a power of 3.
- The term 2x^2 has a power of 2.
- The term 5x has a power of 1.
- The constant term -3 has a power of 0.
As we can see, the term with the highest power of x is x^4. Therefore, the degree of the polynomial is 4.
Therefore, option C is the correct answer.
The degree of a polynomial is the highest power of the variable in that polynomial. In this case, the polynomial is:
x^4 + 3x^3 + 2x^2 + 5x - 3
To find the degree of this polynomial, we need to identify the term with the highest power of x. Let's break down each term in the polynomial:
- The term x^4 has a power of 4.
- The term 3x^3 has a power of 3.
- The term 2x^2 has a power of 2.
- The term 5x has a power of 1.
- The constant term -3 has a power of 0.
As we can see, the term with the highest power of x is x^4. Therefore, the degree of the polynomial is 4.
Therefore, option C is the correct answer.
The remainder obtained when the polynomial p(x) is divided by (b – ax) is- a)
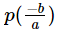
- b)
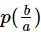
- c)
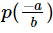
- d)
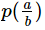
Correct answer is option 'B'. Can you explain this answer?
The remainder obtained when the polynomial p(x) is divided by (b – ax) is
a)
b)
c)
d)
|
|
Meera Rana answered |
ANSWER :- b
Solution :- b-ax=0
b=ax
b/a=x
i.e. remainder is p(b/a)
Evaluate (11)3
- a)1313
- b)1331
- c)3131
- d)3113
Correct answer is option 'B'. Can you explain this answer?
Evaluate (11)3
a)
1313
b)
1331
c)
3131
d)
3113
|
Ananya Das answered |
(a+b)3 = a3 + b3 + 3ab(a + b)
11³= (10+1)³=1000+1+30(11)=1001+330=1331
11³= (10+1)³=1000+1+30(11)=1001+330=1331
Which of the following are the factors of a2 + ab +bc + ca
- a)(a + b) (a + c)
- b)(a + b + c)
- c)(a + b) (b + c)
- d)(b + c) (c + a)
Correct answer is option 'A'. Can you explain this answer?
Which of the following are the factors of a2 + ab +bc + ca
a)
(a + b) (a + c)
b)
(a + b + c)
c)
(a + b) (b + c)
d)
(b + c) (c + a)
|
Nilotpal Unni answered |
Factors of a2 ab bc ca
To find the factors of a2 ab bc ca, we need to factor out the common terms from all the terms. In this case, the common term is 'a'. So, we can write:
a2 ab bc ca = a(a b c + b c a)
Now, we need to factor the expression inside the parentheses. We can see that it contains two terms, 'abc' and 'bca', which have a common factor of 'bc'. So, we can write:
a(a b c + b c a) = a(bc(a + c))
Finally, we can factor out the common factor of 'a' and 'bc', which gives us:
a(bc(a + c)) = ab(a + c) ac(a + c)
Therefore, the factors of a2 ab bc ca are (a b) (a c).
To find the factors of a2 ab bc ca, we need to factor out the common terms from all the terms. In this case, the common term is 'a'. So, we can write:
a2 ab bc ca = a(a b c + b c a)
Now, we need to factor the expression inside the parentheses. We can see that it contains two terms, 'abc' and 'bca', which have a common factor of 'bc'. So, we can write:
a(a b c + b c a) = a(bc(a + c))
Finally, we can factor out the common factor of 'a' and 'bc', which gives us:
a(bc(a + c)) = ab(a + c) ac(a + c)
Therefore, the factors of a2 ab bc ca are (a b) (a c).
What is the value of p if x-2 is a factor of x2 – 6x + p ?
- a)4
- b)-4
- c)8
- d)-8
Correct answer is option 'C'. Can you explain this answer?
What is the value of p if x-2 is a factor of x2 – 6x + p ?
a)
4
b)
-4
c)
8
d)
-8
|
Prachi Rathore answered |
X-2=0,=x=2,Now,x²-6x+p=2²-6×2+p=4-12+p=-8+p=p=8 the real ans is c
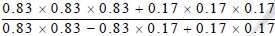is equal to :-
- a)1
- b)(0.83)3 + (0.17)3
- c)0
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
is equal to :-a)
1
b)
(0.83)3 + (0.17)3
c)
0
d)
None of these
|
Shalini Shahi answered |
Yes answer is 1. a=0.83 ,b=0.17 0.83×0.83×0.83 +0.17×0.17×0.17. / 0.83× 0.83+0.83× 0.17+0.17×0.17. = formula = a³+b³=( a+b)(a²-ab +b²) / a²-ab+ b² = cut the up and down a²-ab +b² = only a+b here ,add a+b =0.83+0.17= 1.00= 1, correct answer a) 1..
The value of the polynomial 5x−4x2+3, when x = −1 is
- a)- 6
- b)1
- c)9
- d)-1
Correct answer is option 'A'. Can you explain this answer?
The value of the polynomial 5x−4x2+3, when x = −1 is
a)
- 6
b)
1
c)
9
d)
-1
|
Zachary Foster answered |
It is given that
p(x) = 5x - 4x² + 3
We have to find the value when x = -1
p(-1) = 5(-1) - 4(-1)² + 3
By further calculation
p(-1) = -5 - 4 + 3
So we get
p(-1) = -9 + 3
p(-1) = -6
Therefore, the value is -6.
Find the value of the polynomial 6 – 4x + 3x2 at x = 3
- a)– 24
- b)21
- c)18
- d)-21
Correct answer is option 'B'. Can you explain this answer?
Find the value of the polynomial 6 – 4x + 3x2 at x = 3
a)
– 24
b)
21
c)
18
d)
-21
|
Bibek Ghoshal answered |
The value of the polynomial 6 is 6.
A linear polynomial will have how many zeroes.- a)2
- b)1
- c)0
- d)3
Correct answer is option 'B'. Can you explain this answer?
A linear polynomial will have how many zeroes.
a)
2
b)
1
c)
0
d)
3
|
Rahul Kumar answered |
A linear polynomial has 1 zero.
A quadratic polynomial has 2 zeroes.
A cubic polynomial has 3 zeroes.
In general, any polynomial has as many zeroes as its degree.
What are the two factors of quadratic polynomial x2-16x+64?- a)(x-16) and (x-64)
- b)(x+8) and (x-8)
- c)(x+16) and (x-4)
- d)(x-8) and (x-8)
Correct answer is option 'D'. Can you explain this answer?
What are the two factors of quadratic polynomial x2-16x+64?
a)
(x-16) and (x-64)
b)
(x+8) and (x-8)
c)
(x+16) and (x-4)
d)
(x-8) and (x-8)
|
Niharika Kapoor answered |
Solution:
To find the factors of the quadratic polynomial x2-16x+64, we can use the factorization formula for perfect square trinomials.
Formula: (a-b)2 = a2-2ab+b2
Comparing x2-16x+64 with the formula, we can see that a = x and b = 8.
Therefore, (x-8)2 = x2-16x+64
Taking the square root of both sides, we get:
x-8 = ±√(x2-16x+64)
x-8 = ±(x-8)
Now, we can solve for x in each case:
Case 1: x-8 = x-8
Simplifying, we get 0 = 0, which is always true. Therefore, this case gives us only one factor.
Factor 1: x-8
Case 2: x-8 = -(x-8)
Simplifying, we get 2x = 16, which gives us x = 8. Therefore, this case gives us another factor.
Factor 2: x-8
Thus, the two factors of the quadratic polynomial x2-16x+64 are (x-8) and (x-8), which can be written as (x-8)2.
Therefore, the correct answer is option D, (x-8) and (x-8).
To find the factors of the quadratic polynomial x2-16x+64, we can use the factorization formula for perfect square trinomials.
Formula: (a-b)2 = a2-2ab+b2
Comparing x2-16x+64 with the formula, we can see that a = x and b = 8.
Therefore, (x-8)2 = x2-16x+64
Taking the square root of both sides, we get:
x-8 = ±√(x2-16x+64)
x-8 = ±(x-8)
Now, we can solve for x in each case:
Case 1: x-8 = x-8
Simplifying, we get 0 = 0, which is always true. Therefore, this case gives us only one factor.
Factor 1: x-8
Case 2: x-8 = -(x-8)
Simplifying, we get 2x = 16, which gives us x = 8. Therefore, this case gives us another factor.
Factor 2: x-8
Thus, the two factors of the quadratic polynomial x2-16x+64 are (x-8) and (x-8), which can be written as (x-8)2.
Therefore, the correct answer is option D, (x-8) and (x-8).
The factors of x3 – 2x2 – 13x – 10 are :-- a)(x – 1) (x + 2) (x + 5)
- b)(x – 1) (x – 2) (x – 5)
- c)(x + 1) (x – 2) (x + 5)
- d)(x + 1) (x + 2) (x – 5)
Correct answer is option 'D'. Can you explain this answer?
The factors of x3 – 2x2 – 13x – 10 are :-
a)
(x – 1) (x + 2) (x + 5)
b)
(x – 1) (x – 2) (x – 5)
c)
(x + 1) (x – 2) (x + 5)
d)
(x + 1) (x + 2) (x – 5)
|
Mehak Jaju answered |
_+1 +_2 +_5 +_10 are factors (x+1)=0 x=-1 put the value in equation and remainder is 0 now dived the question equation with x+1 u get a byquardtic equation and using splitting the middle term factorise it
If one of the factor of x2 + x – 20 is (x + 5). Find the other
- a)x – 4
- b)x + 2
- c)x + 4
- d)x – 5
Correct answer is option 'A'. Can you explain this answer?
If one of the factor of x2 + x – 20 is (x + 5). Find the other
a)
x – 4
b)
x + 2
c)
x + 4
d)
x – 5
|
Tara Singh answered |
We cannot complete the question as it is incomplete. Please provide the complete question.
If p(x) = 7 – 3x + 2x2 then value of p(-2) is:- a)22
- b)12
- c)21
- d)31
Correct answer is option 'C'. Can you explain this answer?
If p(x) = 7 – 3x + 2x2 then value of p(-2) is:
a)
22
b)
12
c)
21
d)
31
|
Indu Gupta answered |
p(-2) = 7 - 3(-2) + 2(-2)2
= 7+6+8 = 21
A polynomial containing three nonzero terms is called a ________.- a)monomial
- b)binomial
- c)trinomial
- d)none of these
Correct answer is option 'B'. Can you explain this answer?
A polynomial containing three nonzero terms is called a ________.
a)
monomial
b)
binomial
c)
trinomial
d)
none of these
|
|
Ananya Sharma answered |
A polynomial containing two nonzero terms is called a binomial .For example :- (3+6x) , (x-5y)
If p(x) = x + 3, then p(x) + p(-x) is equal to- a)0
- b)3
- c)6
- d)2x
Correct answer is option 'C'. Can you explain this answer?
If p(x) = x + 3, then p(x) + p(-x) is equal to
a)
0
b)
3
c)
6
d)
2x
|
Sanjana answered |
we have , p(x) = x+3.........(1) Replacing x by -x ,we get p(-x)= -x+3 ............(2) adding the corresponding sides of (1)and (2),we get p(x)+p(-x) = 6
Factorise the quadratic polynomial by splitting the middle term:
x2 + 14x + 45- a)(x + 9) (x+5)
- b)(x - 9) (x+5)
- c)(x-9) ( x-5)
- d)(x+9) ( x-5)
Correct answer is option 'A'. Can you explain this answer?
Factorise the quadratic polynomial by splitting the middle term:
x2 + 14x + 45
x2 + 14x + 45
a)
(x + 9) (x+5)
b)
(x - 9) (x+5)
c)
(x-9) ( x-5)
d)
(x+9) ( x-5)
|
|
Shashwat Singh answered |
=x^2+14x+45
=x^2+9x+5x+45
=x(x+9)+5(x+9)
=(x+9)(x+5)
Which of the following is not a quadratic polynomial?
- a)1 + z2
- b)y + y2 + 4
- c)x – x3
- d)x2 + x
Correct answer is option 'C'. Can you explain this answer?
Which of the following is not a quadratic polynomial?
a)
1 + z2
b)
y + y2 + 4
c)
x – x3
d)
x2 + x
|
Harsh Kyada answered |
When we solve the polynomial it left one var iabla and one number I. e y^2+4. if a polynomial leave one no. after solving it is linear , if a polynomial leave two no. or two variable it is quadratic and if a polynomial leave three no. or three. variable after solving itbis cubic.
The number of zeros of x2 + 4x + 2- a) 1
- b) 2
- c) 3
- d) none of these
Correct answer is option 'B'. Can you explain this answer?
The number of zeros of x2 + 4x + 2
a)
1
b)
2
c)
3
d)
none of these
|
Shreya Singh answered |
The answer will be option d non of these.
When the polynomial x3 + 3x2 + 3x + 1 is divided by x + 1, the remainder is :-- a)1
- b)8
- c)0
- d)- 6
Correct answer is option 'C'. Can you explain this answer?
When the polynomial x3 + 3x2 + 3x + 1 is divided by x + 1, the remainder is :-
a)
1
b)
8
c)
0
d)
- 6
|
Hansa Sharma answered |
The zero of x + 1 is –1
And by remainder theorem, when
p(x) = x3 + 3x2 + 3x + 1 is divided by x + 1, then remainder is p(–1).
∴ p(–1) = (–1)3 + 3 (–1)2 + 3(–1) + 1
= –1 + (3 × 1) + (–3) + 1
= –1 + 3 – 3 + 1
= 0
Thus, the required = 0
What is the value 83 – 33 (without solving the cubes)?
- a)485
- b)845
- c)458
- d)854
Correct answer is option 'A'. Can you explain this answer?
What is the value 83 – 33 (without solving the cubes)?
a)
485
b)
845
c)
458
d)
854
|
Pranab Datta answered |
The value 83 is a positive integer that represents a quantity or amount. It is a prime number and comes after 82 and before 84 in the number sequence.
If P(x) = 10x−4x2−3, then the value of p(0)+p(1) is
- a)1
- b)3
- c)-3
- d)0
Correct answer is option 'D'. Can you explain this answer?
If P(x) = 10x−4x2−3, then the value of p(0)+p(1) is
a)
1
b)
3
c)
-3
d)
0
|
|
Madhurima Ahuja answered |
I'm sorry, your question is incomplete. Please provide more details or context for me to understand and respond accurately.
- a)2p = r
- b)p = 2r
- c)p = r
- d)none of these
Correct answer is option 'C'. Can you explain this answer?
a)
2p = r
b)
p = 2r
c)
p = r
d)
none of these
|
|
Amit Sharma answered |
Let f(x) = px2 + 5 x + r
If (x - 2) is a factor of f (x), then by factor theorem
f(2) = 0 | x - 2 = 0 ⇒ x = 2
⇒ p(2)2 + 5(2) + r = 0
⇒ 4p + r + 10 = 0 ...(1)
If (x - 2) is a factor of f (x), then by factor theorem
f(2) = 0 | x - 2 = 0 ⇒ x = 2
⇒ p(2)2 + 5(2) + r = 0
⇒ 4p + r + 10 = 0 ...(1)
If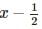 
is a factor of f (x), then by factor theorem,
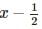is a factor of f (x), then by factor theorem,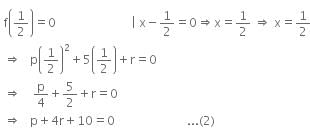
Subtracting (2) from (1), we get
3p - 3r = 0
⇒ p = r
3p - 3r = 0
⇒ p = r
The zero of the polynomial (x−2)2−(x+2)2 is- a)1
- b)-2
- c)2
- d)0
Correct answer is option 'D'. Can you explain this answer?
The zero of the polynomial (x−2)2−(x+2)2 is
a)
1
b)
-2
c)
2
d)
0
|
|
Sanjana answered |
{(x)² + (2)² - 2× x ×2} - {(x)² + (2)² + 2 × x×2} = (x² + 4 - 4x) - (x² + 4 + 4x) = x² + 4 - 4x - x²- 4 - 4x = 0.........ans
Can you explain the answer of this question below:Factorise : 8a3+ b3 + 12a2b + 6a b2
- A:
(2a + b) (2a + b) (2a + b)
- B:
(2a – b) (2a – b) (2a + b)
- C:
(2a-b)(2a-b)(2a-b)
- D:
(-2a + b) (-2a + b) (2a + b)
The answer is a.
Factorise : 8a3+ b3 + 12a2b + 6a b2
(2a + b) (2a + b) (2a + b)
(2a – b) (2a – b) (2a + b)
(2a-b)(2a-b)(2a-b)
(-2a + b) (-2a + b) (2a + b)
|
Ishan Nair answered |
8a3 + b3+ 12a2b + 6ab2
8a3 + b3+ 12a2b + 6ab2
= (2a)3 + (b)3 + 3(2a)(b) (2a + b)
= (2a + b)3 | Using Identity VI
= (2a + b)(2a + b)(2a + b)
8a3 + b3+ 12a2b + 6ab2
= (2a)3 + (b)3 + 3(2a)(b) (2a + b)
= (2a + b)3 | Using Identity VI
= (2a + b)(2a + b)(2a + b)
√2 is a polynomial of degree- a)1
- b)0
- c)2
- d)√2
Correct answer is option 'B'. Can you explain this answer?
√2 is a polynomial of degree
a)
1
b)
0
c)
2
d)
√2
|
Vivek Rana answered |
The highest power of the variable is known as the degree of the polynomial.
√2x^0 = √2
hence the degree of the polynomial is zero.
√2x^0 = √2
hence the degree of the polynomial is zero.
Find the zero of the polynomial of p (x) = ax + b ; a ≠ 0- a)b/a
- b)a/b
- c)-b/a
- d)-a/b
Correct answer is 'C'. Can you explain this answer?
Find the zero of the polynomial of p (x) = ax + b ; a ≠ 0
a)
b/a
b)
a/b
c)
-b/a
d)
-a/b
|
|
Saranya Nair answered |
Finding the Zero of a Polynomial
To find the zero of a polynomial, we need to solve for x when p(x) = 0. In other words, we need to find the value of x that makes the polynomial equal to zero.
Given p(x) = ax^b, where a ≠ 0 and b ≥ 1, we need to find the zero of the polynomial.
Solution
To find the zero of the polynomial, we need to solve for x when p(x) = 0. Substituting the given polynomial, we get:
ax^b = 0
Since a ≠ 0 and b ≥ 1, we know that the only value of x that satisfies the equation is x = 0. Therefore, the zero of the polynomial is x = 0.
Option (c) is the correct answer, as it corresponds to x = 0.
Explanation
The given polynomial p(x) = ax^b has only one term, which is ax^b. This term can only equal zero if x = 0, since any non-zero value of x raised to a positive power will be non-zero.
Therefore, the zero of the polynomial is x = 0, which corresponds to option (c).
To find the zero of a polynomial, we need to solve for x when p(x) = 0. In other words, we need to find the value of x that makes the polynomial equal to zero.
Given p(x) = ax^b, where a ≠ 0 and b ≥ 1, we need to find the zero of the polynomial.
Solution
To find the zero of the polynomial, we need to solve for x when p(x) = 0. Substituting the given polynomial, we get:
ax^b = 0
Since a ≠ 0 and b ≥ 1, we know that the only value of x that satisfies the equation is x = 0. Therefore, the zero of the polynomial is x = 0.
Option (c) is the correct answer, as it corresponds to x = 0.
Explanation
The given polynomial p(x) = ax^b has only one term, which is ax^b. This term can only equal zero if x = 0, since any non-zero value of x raised to a positive power will be non-zero.
Therefore, the zero of the polynomial is x = 0, which corresponds to option (c).
If the polynomial 2x3 – 3x2 + 2x – 4 is divided by x – 2, then the remainder is :-- a)- 4
- b)4
- c)-40
- d)2
Correct answer is option 'B'. Can you explain this answer?
If the polynomial 2x3 – 3x2 + 2x – 4 is divided by x – 2, then the remainder is :-
a)
- 4
b)
4
c)
-40
d)
2
|
|
Mahi Sharma answered |
3x2 - 5x + 7 is divided by x - 2, the remainder is:
To find the remainder, we can use the remainder theorem which states that if a polynomial f(x) is divided by x - a, the remainder is equal to f(a).
Therefore, if we substitute x = 2 in the given polynomial, we get:
2(2)3 - 3(2)2 - 5(2) + 7 = 16 - 12 - 10 + 7 = 1
Hence, the remainder when the polynomial 2x3 + 3x2 - 5x + 7 is divided by x - 2 is 1.
To find the remainder, we can use the remainder theorem which states that if a polynomial f(x) is divided by x - a, the remainder is equal to f(a).
Therefore, if we substitute x = 2 in the given polynomial, we get:
2(2)3 - 3(2)2 - 5(2) + 7 = 16 - 12 - 10 + 7 = 1
Hence, the remainder when the polynomial 2x3 + 3x2 - 5x + 7 is divided by x - 2 is 1.
(x + 1) is a factor of the polynomial- a)x4+3x3+3x2+x+1
- b)x3+x2−x+1
- c)x4+x3+x2+1
- d)x3+x2+x+1
Correct answer is option 'D'. Can you explain this answer?
(x + 1) is a factor of the polynomial
a)
x4+3x3+3x2+x+1
b)
x3+x2−x+1
c)
x4+x3+x2+1
d)
x3+x2+x+1
|
|
Krishna Singi answered |
Option d is the best answer in india reason is given in r d sharma and r s aggrawal or Google search
(a – b)3 + (b – c)3 + (c – a)3 is equal to :-- a)3abc
- b)3a3b3c3
- c)3(a – b) (b – c) (c – a)
- d)[a – (b + c)]3
Correct answer is option 'C'. Can you explain this answer?
(a – b)3 + (b – c)3 + (c – a)3 is equal to :-
a)
3abc
b)
3a3b3c3
c)
3(a – b) (b – c) (c – a)
d)
[a – (b + c)]3
|
|
Indu Gupta answered |
Let x = (a – b), y = (b – c) and z = (c – a)
Consider, x + y + z = (a – b) + (b – c) + (c – a) = 0
⇒ x3 + y3 + z3 = 3xyz
That is (a – b)3 + (b – c)3 + (c – a)3 = 3(a – b)(b – c)(c – a)
Consider, x + y + z = (a – b) + (b – c) + (c – a) = 0
⇒ x3 + y3 + z3 = 3xyz
That is (a – b)3 + (b – c)3 + (c – a)3 = 3(a – b)(b – c)(c – a)
Factorize the quadratic polynomial by splitting the middle term: y2 – 4 y –21
- a)(y – 7) (y – 3)
- b)(y – 7) (y + 3)
- c)(y + 7) (y – 3)
- d)(y + 7) (y + 3)
Correct answer is option 'B'. Can you explain this answer?
Factorize the quadratic polynomial by splitting the middle term: y2 – 4 y –21
a)
(y – 7) (y – 3)
b)
(y – 7) (y + 3)
c)
(y + 7) (y – 3)
d)
(y + 7) (y + 3)
|
|
Ujwal Das answered |
+ 7y + 10
To factorize this quadratic polynomial, we need to find two numbers that multiply to give the constant term (10) and add to give the coefficient of the middle term (7).
The factors of 10 are:
1 x 10
2 x 5
Since we need the sum of the factors to be 7, we can see that 2 and 5 are the two numbers we are looking for.
So, we can rewrite the middle term as 2y + 5y:
y2 + 2y + 5y + 10
Now, we can group the first two terms and the last two terms together and factorize each group separately:
y(y + 2) + 5(y + 2)
Notice that both groups have a common factor of (y + 2), so we can factorize it out:
(y + 2)(y + 5)
Therefore, the factored form of the quadratic polynomial y2 + 7y + 10 is (y + 2)(y + 5).
To factorize this quadratic polynomial, we need to find two numbers that multiply to give the constant term (10) and add to give the coefficient of the middle term (7).
The factors of 10 are:
1 x 10
2 x 5
Since we need the sum of the factors to be 7, we can see that 2 and 5 are the two numbers we are looking for.
So, we can rewrite the middle term as 2y + 5y:
y2 + 2y + 5y + 10
Now, we can group the first two terms and the last two terms together and factorize each group separately:
y(y + 2) + 5(y + 2)
Notice that both groups have a common factor of (y + 2), so we can factorize it out:
(y + 2)(y + 5)
Therefore, the factored form of the quadratic polynomial y2 + 7y + 10 is (y + 2)(y + 5).
The quadratic polynomial whose sum of zeroes is 3 and the product of zeroes is –2 is :
- a)x2 + 3x – 2
- b)x2 – 2x + 3
- c)x2 – 3x + 2
- d)x2 – 3x – 2
Correct answer is option 'D'. Can you explain this answer?
The quadratic polynomial whose sum of zeroes is 3 and the product of zeroes is –2 is :
a)
x2 + 3x – 2
b)
x2 – 2x + 3
c)
x2 – 3x + 2
d)
x2 – 3x – 2
|
Meha nair answered |
Sum of zeros = 3/1
-b/a = 3/1 .....................(1)
-b/a = 3/1 .....................(1)
Product of zeros = -2/1
c/a = -2/1 ...................(2)
c/a = -2/1 ...................(2)
From equation (1) and (2)
a = 1
-b = 3, b = -3
c = -2
a = 1
-b = 3, b = -3
c = -2
The required quadratic equation is
ax2+b
= x2-3x-2
ax2+b
x
+c= x2-3x-2
Expansion and simplification of 8(3h - 4) + 5(h - 2) gives- a)24h - 37
- b)36 - 4h
- c)29h - 42
- d)38 + 42h
Correct answer is option 'C'. Can you explain this answer?
Expansion and simplification of 8(3h - 4) + 5(h - 2) gives
a)
24h - 37
b)
36 - 4h
c)
29h - 42
d)
38 + 42h
|
C K Academy answered |
Now, 8 (3h - 4) + 5 (h - 2)
= 24h - 32 + 5h - 10, the expansion
= 24h + 5h - 32 - 10
= 29h - 42, the simplification
Degree of the polynomial 4x4 + 0x3 + 0x5 + 5x + 7 is:- a)5
- b)4
- c)6
- d)7
Correct answer is option 'B'. Can you explain this answer?
Degree of the polynomial 4x4 + 0x3 + 0x5 + 5x + 7 is:
a)
5
b)
4
c)
6
d)
7
|
Kanta Jindal answered |
Because x^5 is multiplied by 0
and therefore x^5 is not considered
and therefore x^5 is not considered
Evaluate (104)3- a)1142864
- b)1124864
- c)1124844
- d)1142846
Correct answer is option 'B'. Can you explain this answer?
Evaluate (104)3
a)
1142864
b)
1124864
c)
1124844
d)
1142846
|
Nipun S answered |
Hey mate here is Your answer
Given to -- Evaluate (104)^3
Solution --
We can Write (104)^3 As (100+4)^3
Now By Using Identity (a+b)^3 = a^3 + b^3 +3ab (a + b)
here a = 100 and b = 4
Therefore we Have...
(100+4)^3 = 100^3 + 4^3 + 3(100)(4) ( 100+4 )
That is...
1000000+64+1200 ( 104 )
That is
1000064+124800
So We Have
(104)^3 = 1124864
Hope It's helpful
Follow For More answers....
Given to -- Evaluate (104)^3
Solution --
We can Write (104)^3 As (100+4)^3
Now By Using Identity (a+b)^3 = a^3 + b^3 +3ab (a + b)
here a = 100 and b = 4
Therefore we Have...
(100+4)^3 = 100^3 + 4^3 + 3(100)(4) ( 100+4 )
That is...
1000000+64+1200 ( 104 )
That is
1000064+124800
So We Have
(104)^3 = 1124864
Hope It's helpful
Follow For More answers....
Factorize: (x – y)3 + (y – z)3 + (z – x)3- a)(2x – y) (y – z) ( z – x)
- b)3(x – y) (y – z) (z – x)
- c)(x – y) (2y – 3z) (z – x)
- d)(2x – 3y) (5x + 3y) (6x – 7y)
Correct answer is option 'B'. Can you explain this answer?
Factorize: (x – y)3 + (y – z)3 + (z – x)3
a)
(2x – y) (y – z) ( z – x)
b)
3(x – y) (y – z) (z – x)
c)
(x – y) (2y – 3z) (z – x)
d)
(2x – 3y) (5x + 3y) (6x – 7y)
|
|
Suyash Rane answered |
Sorry, the expression is incomplete. Please provide the complete expression for me to factorize.
If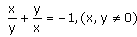 
, the value of x3 – y3 is :
- a)1
- b)1/2
- c)0
- d)-1
Correct answer is option 'C'. Can you explain this answer?
If 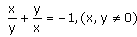, the value of x3 – y3 is :
, the value of x3 – y3 is :a)
1
b)
1/2
c)
0
d)
-1
|
|
Zachary Foster answered |
- Create a common denominator:
- Multiply both sides of the equation by xy to get rid of the fractions:
- x² + y² = -xy
- Multiply both sides of the equation by xy to get rid of the fractions:
- Rearrange the equation:
- Add xy to both sides:
- x² + xy + y² = 0
- Add xy to both sides:
- Substitute the values in: x³ - y³ = (x - y)(x² + xy + y²)
- We also know x² + xy + y² = 0 from step 2.Substitute these values into the identity:
- x³ - y³ = (x-y)(0) = 0
Therefore, the value of x³ - y³ is 0.
So, the correct answer is option 3.
The expanded form of (3x−5)3 is- a)27x3−135x2+225x−125
- b)27x3+135x2−225x−125
- c)27x3+135x2+225x−125
- d)none of these
Correct answer is option 'A'. Can you explain this answer?
The expanded form of (3x−5)3 is
a)
27x3−135x2+225x−125
b)
27x3+135x2−225x−125
c)
27x3+135x2+225x−125
d)
none of these
|
Raghav Bansal answered |
Let a = 3x and b = 5 (3x)³ - (5)³ - 3×3x×5(3x- 5) = 27x³ - 125 -45x ( 3x -5) = 27x³ -125 - 135x² + 225x = 27x³ - 135x² + 225x - 125
Factorise:(3x- 5y)3+ (5y – 2z)3 + (2z – 3x)3
- a)3(3x + 5y) (5y + 2z) (2z + 3x)
- b)2(3x + 5y) (5y + 2z) (2z + 3x)
- c)3(3x - 5y) (5y – 2z) (2z – 3x)
- d)2(3x – 5y) (5y – 2z) (2z – 3x)
Correct answer is option 'C'. Can you explain this answer?
Factorise:(3x- 5y)3+ (5y – 2z)3 + (2z – 3x)3
a)
3(3x + 5y) (5y + 2z) (2z + 3x)
b)
2(3x + 5y) (5y + 2z) (2z + 3x)
c)
3(3x - 5y) (5y – 2z) (2z – 3x)
d)
2(3x – 5y) (5y – 2z) (2z – 3x)
|
|
Hridoy Iyer answered |
The given expression is (3x - 5y)^3 (5y).
To factorize this expression, we can start by separating the expression inside the parentheses, (3x - 5y), and then factorize it using the binomial formula:
(3x - 5y)^3 (5y) = (3x - 5y)(3x - 5y)(3x - 5y)(5y)
Now, we can factor out the common factor (3x - 5y) from each term:
(3x - 5y)(3x - 5y)(3x - 5y)(5y) = (3x - 5y)^3 (3x - 5y)(5y)
Therefore, the factored form of the expression is (3x - 5y)^3 (3x - 5y)(5y).
To factorize this expression, we can start by separating the expression inside the parentheses, (3x - 5y), and then factorize it using the binomial formula:
(3x - 5y)^3 (5y) = (3x - 5y)(3x - 5y)(3x - 5y)(5y)
Now, we can factor out the common factor (3x - 5y) from each term:
(3x - 5y)(3x - 5y)(3x - 5y)(5y) = (3x - 5y)^3 (3x - 5y)(5y)
Therefore, the factored form of the expression is (3x - 5y)^3 (3x - 5y)(5y).
The degree of the polynomial 4x4+0x3+0x5+5x+74x4+0x3+0x5+5x+7 is- a)4
- b)6
- c)7
- d)5
Correct answer is option 'A'. Can you explain this answer?
The degree of the polynomial 4x4+0x3+0x5+5x+74x4+0x3+0x5+5x+7 is
a)
4
b)
6
c)
7
d)
5
|
|
Vikram Khanna answered |
Degree of 4x4 + Ox3 + Ox5 + 5x + 7 is equal to the highest power of variable x. Here, the highest power of x is 4, Hence, the degree of a polynomial is 4.
If p(x) = x + 3, then p(x) + p(-x) is equal to- a)0
- b)3
- c)6
- d)2x
Correct answer is option 'C'. Can you explain this answer?
If p(x) = x + 3, then p(x) + p(-x) is equal to
a)
0
b)
3
c)
6
d)
2x
|
Manisha Soni answered |
P(x) = x 3 = 0 (because (x 3) is a factor of p(x)) therefore, p(-x) = -(x 3) = 0 = -x -3 =0 therefore,. -x = 3 x =. -3. ---------result 1 therefore, p(-x) = 3. --------------- result 2 now, p(x) p(-x) = x 3 3 = -3 3 3. ( by result 1 & 2) =. -3 6 =. 3
The expanded form of (2x – 3y – z)2 is :-- a)4x2 + 9y2 + z2 – 6xy + 3yz – 2zx
- b)4x2 + 9y2 + z2 + 6xy + 6yz – 2zx
- c)4x2 + 9y2 + z2 – 12xy – 6yz – 4zx
- d)4x2 + 9y2 + z2 – 12xy + 6yz – 4zx
Correct answer is option 'D'. Can you explain this answer?
The expanded form of (2x – 3y – z)2 is :-
a)
4x2 + 9y2 + z2 – 6xy + 3yz – 2zx
b)
4x2 + 9y2 + z2 + 6xy + 6yz – 2zx
c)
4x2 + 9y2 + z2 – 12xy – 6yz – 4zx
d)
4x2 + 9y2 + z2 – 12xy + 6yz – 4zx
|
|
Sachi Menon answered |
The expanded form of (2x) is 2 times x, which can also be written as 2x. There is no further expansion needed as it is already in its simplest form.
Chapter doubts & questions for Polynomials - Mathematics (Maths) Class 9 2025 is part of Class 9 exam preparation. The chapters have been prepared according to the Class 9 exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Class 9 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Polynomials - Mathematics (Maths) Class 9 in English & Hindi are available as part of Class 9 exam.
Download more important topics, notes, lectures and mock test series for Class 9 Exam by signing up for free.
Mathematics (Maths) Class 9
40 videos|471 docs|57 tests
|
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup