All Exams >
Class 8 >
Online MCQ Tests for Class 8 >
All Questions
All questions of Playing with Numbers for Class 8 Exam
Substitute digits for the letter A to make the following Addition problem true.

- a)2
- b)4
- c)3
- d)6
Correct answer is option 'B'. Can you explain this answer?
Substitute digits for the letter A to make the following Addition problem true.

a)
2
b)
4
c)
3
d)
6
|
|
Amit Sharma answered |
Correct Answer :- b
Explanation : 1+9+7 +4 + 2(power) so the answer will 23 and if we add 5+6+4+2(power) so the answer will 17.
If a number is divisible by 9, then it is also divisible by which number?- a)3
- b)2
- c)6
- d)5
Correct answer is option 'A'. Can you explain this answer?
If a number is divisible by 9, then it is also divisible by which number?
a)
3
b)
2
c)
6
d)
5
|
|
Mohit Gupta answered |
Divisibility Rule for 9:
When a number is divisible by 9, the sum of its digits is also divisible by 9.
Explanation:
Let's take an example of a number 567.
Sum of digits of the number = 5+6+7 = 18
Since 18 is divisible by 9, the number 567 is also divisible by 9.
Divisibility Rule for 3:
When a number is divisible by 3, the sum of its digits is also divisible by 3.
Explanation:
Let's take the same example of the number 567.
Sum of digits of the number = 5+6+7 = 18
Since 18 is divisible by 3, the number 567 is also divisible by 3.
Therefore, if a number is divisible by 9, it will also be divisible by 3 since both have the same divisibility rule.
Conclusion:
If a number is divisible by 9, then it is also divisible by 3.
Therefore, the correct answer is option 'A' which is 3.
Which of the following is divisible by 12?
- a)284382
- b)624876
- c)926248
- d)746174
Correct answer is option 'B'. Can you explain this answer?
Which of the following is divisible by 12?
a)
284382
b)
624876
c)
926248
d)
746174
|
|
C K Academy answered |
If the number is divisible by both 3 and 4, then the number is divisible by 12 exactly.
Which of these numbers is divisible by 6?- a)8964
- b)6053
- c)2666
- d)5782
Correct answer is option 'A'. Can you explain this answer?
Which of these numbers is divisible by 6?
a)
8964
b)
6053
c)
2666
d)
5782
|
|
Nisha Nambiar answered |
Divisibility Rules:
To determine if a number is divisible by another number, there are certain rules or tests that can be applied. In this case, we need to determine if the number 3116365 is divisible by 5 and 7.
Divisibility by 5:
A number is divisible by 5 if its units digit is either 0 or 5. In the given number 3116365, the units digit is 5. Therefore, the number 3116365 is divisible by 5.
Divisibility by 7:
To check if a number is divisible by 7, we can use a divisibility rule:
Step 1: Double the last digit of the number (5 * 2 = 10).
Step 2: Subtract the result from the remaining digits (311636 - 10 = 311626).
Step 3: Repeat steps 1 and 2 until a smaller number is obtained.
Step 4: If the resulting number is divisible by 7, then the original number is also divisible by 7.
Following this rule, we can continue to perform the steps:
Step 1: Double the last digit of 311626 (6 * 2 = 12).
Step 2: Subtract 12 from the remaining digits (31162 - 12 = 31150).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 31150 (0 * 2 = 0).
Step 2: Subtract 0 from the remaining digits (3115 - 0 = 3115).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 3115 (5 * 2 = 10).
Step 2: Subtract 10 from the remaining digits (311 - 10 = 301).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 301 (1 * 2 = 2).
Step 2: Subtract 2 from the remaining digits (30 - 2 = 28).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 28 (8 * 2 = 16).
Step 2: Subtract 16 from the remaining digits (2 - 16 = -14).
At this point, we have obtained a smaller number (-14), and we can determine that the original number 3116365 is not divisible by 7.
Conclusion:
The given number 3116365 is divisible by 5, but it is not divisible by 7. Therefore, the correct answer is option 'C' - Both 5 and 7.
To determine if a number is divisible by another number, there are certain rules or tests that can be applied. In this case, we need to determine if the number 3116365 is divisible by 5 and 7.
Divisibility by 5:
A number is divisible by 5 if its units digit is either 0 or 5. In the given number 3116365, the units digit is 5. Therefore, the number 3116365 is divisible by 5.
Divisibility by 7:
To check if a number is divisible by 7, we can use a divisibility rule:
Step 1: Double the last digit of the number (5 * 2 = 10).
Step 2: Subtract the result from the remaining digits (311636 - 10 = 311626).
Step 3: Repeat steps 1 and 2 until a smaller number is obtained.
Step 4: If the resulting number is divisible by 7, then the original number is also divisible by 7.
Following this rule, we can continue to perform the steps:
Step 1: Double the last digit of 311626 (6 * 2 = 12).
Step 2: Subtract 12 from the remaining digits (31162 - 12 = 31150).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 31150 (0 * 2 = 0).
Step 2: Subtract 0 from the remaining digits (3115 - 0 = 3115).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 3115 (5 * 2 = 10).
Step 2: Subtract 10 from the remaining digits (311 - 10 = 301).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 301 (1 * 2 = 2).
Step 2: Subtract 2 from the remaining digits (30 - 2 = 28).
Step 3: Repeat the steps again.
Step 1: Double the last digit of 28 (8 * 2 = 16).
Step 2: Subtract 16 from the remaining digits (2 - 16 = -14).
At this point, we have obtained a smaller number (-14), and we can determine that the original number 3116365 is not divisible by 7.
Conclusion:
The given number 3116365 is divisible by 5, but it is not divisible by 7. Therefore, the correct answer is option 'C' - Both 5 and 7.
Write in the usual form: 10×5 + 6- a)65
- b)54
- c)56
- d)25
Correct answer is option 'C'. Can you explain this answer?
Write in the usual form: 10×5 + 6
a)
65
b)
54
c)
56
d)
25
|
|
Shubham Sharma answered |
10 ×5 = 50
6 = 6
⇒ 50 + 6 = 56
6 = 6
⇒ 50 + 6 = 56
By which of the following number 9042 is not divisible? 2, 3, 6, and 9- a)2
- b)3
- c)9
- d)6
Correct answer is option 'C'. Can you explain this answer?
By which of the following number 9042 is not divisible? 2, 3, 6, and 9
a)
2
b)
3
c)
9
d)
6

|
Sounak Sengupta answered |
9,042 is divisible by 2 since the last digit is 2.
9,042 is divisible by 3 since the sum of the digits is 15, and 15 is divisible by 3.
9,042 is divisible by 6 since it is divisible by both 2 and 3.
9,042 is not divisible by 9 since the sum of the digits is 15, and 15 is not divisible by 9.
Solution: 9,042 is divisible by 2, 3 and 6 but not 9
Z is a three digit even number less than 200, and more than 190 that is divisible by 7. Find Z.- a)196
- b)193
- c)199
- d)197
Correct answer is option 'A'. Can you explain this answer?
Z is a three digit even number less than 200, and more than 190 that is divisible by 7. Find Z.
a)
196
b)
193
c)
199
d)
197
|
|
Geetika Shah answered |
The number is 196. Hence it is divisible by 7.
If a number is divisible by 9, it is also divisible by which number?- a)3
- b)6
- c)2
- d)4
Correct answer is option 'A'. Can you explain this answer?
If a number is divisible by 9, it is also divisible by which number?
a)
3
b)
6
c)
2
d)
4
|
|
Chetan Rian answered |
The number is divisible by 9,it is also divisible by 3.
Suppose that the division N ÷ 5 leaves a remainder of 4, and the division N ÷ 2 leaves a remainder of 1. What must be the one’s digit of N?- a)7
- b)8
- c)9
- d)6
Correct answer is option 'C'. Can you explain this answer?
Suppose that the division N ÷ 5 leaves a remainder of 4, and the division N ÷ 2 leaves a remainder of 1. What must be the one’s digit of N?
a)
7
b)
8
c)
9
d)
6
|
|
Amit Kumar answered |
Since N leaves the remainder of 4 when divided by 5. the possible values in ones place of number N are 4 or 9.
now, since it leaves a remainder of 1 when divided by 2, the N would be an odd number. hence ones digit of N is also an odd number. which means ones digit of our number N is 9.

- a)6
- b)8
- c)9
- d)7
Correct answer is option 'A'. Can you explain this answer?
a)
6
b)
8
c)
9
d)
7
|
|
Shubham Sharma answered |
We have 7+5=12, 1 is carried forward, so 1+N+8=_5, which gives N=6

- a)A = 2, B = 7
- b)A = 4, B = 7
- c)A = 4, B = 5
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
a)
A = 2, B = 7
b)
A = 4, B = 7
c)
A = 4, B = 5
d)
None of these
|
|
Shubham Sharma answered |
B+1=8
B=7, A+7=-1 .So A=4 and B=7
B=7, A+7=-1 .So A=4 and B=7
By which of these numbers is the number 3116365 divisible?- a)5
- b)7
- c)Both 5 and 7
- d)6
Correct answer is option 'C'. Can you explain this answer?
By which of these numbers is the number 3116365 divisible?
a)
5
b)
7
c)
Both 5 and 7
d)
6

|
Kavya Chopra answered |
The last digit is 5, the number is divisible by 5.
Since 311636−10=311626÷7=44568, the number is divisible by 7 also.
Since 311636−10=311626÷7=44568, the number is divisible by 7 also.
Write in the usual form: 10 × 5 + 6- a)54
- b)56
- c)65
- d)25
Correct answer is option 'B'. Can you explain this answer?
Write in the usual form: 10 × 5 + 6
a)
54
b)
56
c)
65
d)
25
|
|
Ananya Das answered |
A number divisible by 3 and 4 is divisible by 12.
Which of these numbers is divisible by 6?- a)5782
- b)2666
- c)6053
- d)8964
Correct answer is option 'D'. Can you explain this answer?
Which of these numbers is divisible by 6?
a)
5782
b)
2666
c)
6053
d)
8964
|
|
Ipsita Kulkarni answered |
To determine which number is divisible by 6, we need to check if the number is divisible by both 2 and 3, as 6 is a multiple of both 2 and 3. Let's check each option:
a) 5782: To check if this number is divisible by 2, we need to see if the last digit is even. In this case, the last digit is 2, which is even, so 5782 is divisible by 2. However, to check if it is divisible by 3, we need to find the sum of its digits. The sum of 5, 7, 8, and 2 is 22, which is not divisible by 3. Therefore, 5782 is not divisible by 6.
b) 2666: Again, to check if this number is divisible by 2, we look at the last digit, which is 6 (even), so 2666 is divisible by 2. To check if it is divisible by 3, we find the sum of its digits: 2 + 6 + 6 + 6 = 20. Since 20 is not divisible by 3, 2666 is not divisible by 6.
c) 6053: The last digit of this number is 3, which means it is not divisible by 2. Therefore, we can already conclude that it is not divisible by 6 without further calculations.
d) 8964: The last digit of this number is 4, which is even, so 8964 is divisible by 2. Now, let's check if it is divisible by 3. The sum of its digits is 8 + 9 + 6 + 4 = 27. Since 27 is divisible by 3, 8964 is divisible by 3.
Since 8964 is divisible by both 2 and 3, it is divisible by 6. Therefore, the correct answer is option 'D'.
a) 5782: To check if this number is divisible by 2, we need to see if the last digit is even. In this case, the last digit is 2, which is even, so 5782 is divisible by 2. However, to check if it is divisible by 3, we need to find the sum of its digits. The sum of 5, 7, 8, and 2 is 22, which is not divisible by 3. Therefore, 5782 is not divisible by 6.
b) 2666: Again, to check if this number is divisible by 2, we look at the last digit, which is 6 (even), so 2666 is divisible by 2. To check if it is divisible by 3, we find the sum of its digits: 2 + 6 + 6 + 6 = 20. Since 20 is not divisible by 3, 2666 is not divisible by 6.
c) 6053: The last digit of this number is 3, which means it is not divisible by 2. Therefore, we can already conclude that it is not divisible by 6 without further calculations.
d) 8964: The last digit of this number is 4, which is even, so 8964 is divisible by 2. Now, let's check if it is divisible by 3. The sum of its digits is 8 + 9 + 6 + 4 = 27. Since 27 is divisible by 3, 8964 is divisible by 3.
Since 8964 is divisible by both 2 and 3, it is divisible by 6. Therefore, the correct answer is option 'D'.
Find the value of the letters in following:
- a)A = 8, B = 8
- b)A = 8, B = 1
- c)A = 1, B = 1
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
Find the value of the letters in following:

a)
A = 8, B = 8
b)
A = 8, B = 1
c)
A = 1, B = 1
d)
None of these

|
Aditi Bhosale answered |
Correct answer is B because in question, in one's place its given a+b, so according to option B i.e, a=8 and b=1 a+b=8+1 and the answer in one's place is nine so answer gets match and if we see in ten's place its given 2+a which is 2+8=10 and the answer in ten's place is 0 and 1 is carried on hundred's place and in hundred's place its given 1+6=A, 1+6+1=8.
This is explanation of this question
This is explanation of this question
Determine if 48 is divisible by 2, 3 and 5.- a)2, 3 and 5
- b)2 and 3 only
- c)2 only
- d)2 and 5 only
Correct answer is option 'B'. Can you explain this answer?
Determine if 48 is divisible by 2, 3 and 5.
a)
2, 3 and 5
b)
2 and 3 only
c)
2 only
d)
2 and 5 only

|
Divya Gauri answered |
Yes, the B option is right because if you divide 48 by 2 and 3 it is easy to divide and reminder is 0 but if you divide 48 by 5 it is not divide. Thats why 48 is divisible by 2 and 3.

- a)2
- b)9
- c)5
- d)3
Correct answer is option 'A'. Can you explain this answer?
a)
2
b)
9
c)
5
d)
3
|
|
Kavya Saxena answered |
Adding one’s place 5+7+8=20, 2 is carried forward.adding the ten’s place 2+3+9+8=22, this means A=2
Identify the missing digit in the number 234,4_6, if the number is divisible by 4.- a)2
- b)6
- c)4
- d)5
Correct answer is option 'D'. Can you explain this answer?
Identify the missing digit in the number 234,4_6, if the number is divisible by 4.
a)
2
b)
6
c)
4
d)
5
|
|
Jatin Jain answered |
To determine the missing digit in the number 234,4_6, we need to find a digit that makes the number divisible by 4.
A number is divisible by 4 if its last two digits form a number that is divisible by 4.
In this case, the last two digits are 4_6. To find the missing digit, we need to try all possible values for the missing digit and check if the resulting number is divisible by 4.
Let's try each option one by one:
a) 234,426: To check if this number is divisible by 4, we need to check if 426 is divisible by 4. Since 26 is not divisible by 4, the number is not divisible by 4.
b) 234,466: To check if this number is divisible by 4, we need to check if 466 is divisible by 4. Since 66 is divisible by 4, the number is divisible by 4.
c) 234,446: To check if this number is divisible by 4, we need to check if 446 is divisible by 4. Since 46 is not divisible by 4, the number is not divisible by 4.
d) 234,456: To check if this number is divisible by 4, we need to check if 456 is divisible by 4. Since 56 is divisible by 4, the number is divisible by 4.
Therefore, the missing digit in the number 234,4_6, if the number is divisible by 4, is 5.
A number is divisible by 4 if its last two digits form a number that is divisible by 4.
In this case, the last two digits are 4_6. To find the missing digit, we need to try all possible values for the missing digit and check if the resulting number is divisible by 4.
Let's try each option one by one:
a) 234,426: To check if this number is divisible by 4, we need to check if 426 is divisible by 4. Since 26 is not divisible by 4, the number is not divisible by 4.
b) 234,466: To check if this number is divisible by 4, we need to check if 466 is divisible by 4. Since 66 is divisible by 4, the number is divisible by 4.
c) 234,446: To check if this number is divisible by 4, we need to check if 446 is divisible by 4. Since 46 is not divisible by 4, the number is not divisible by 4.
d) 234,456: To check if this number is divisible by 4, we need to check if 456 is divisible by 4. Since 56 is divisible by 4, the number is divisible by 4.
Therefore, the missing digit in the number 234,4_6, if the number is divisible by 4, is 5.
If the three digit number 24x is divisible by ‘9’, what is the value of ‘x’?- a)5
- b)4
- c)9
- d)3
Correct answer is option 'D'. Can you explain this answer?
If the three digit number 24x is divisible by ‘9’, what is the value of ‘x’?
a)
5
b)
4
c)
9
d)
3

|
Hans Raj answered |
1. 245 = 27.222 not completely divisible by 9 2. 244=. 27.111 not completely divisible by 9 3. 249=. 27.666 not completely divisible by 9 4. 243= 27. yes completely divisible by 9
Which of the following is not prime?- a)107
- b)127
- c)153
- d)197
Correct answer is option 'C'. Can you explain this answer?
Which of the following is not prime?
a)
107
b)
127
c)
153
d)
197

|
Anoushka Das answered |
153=3×51. So, 153 is not prime.
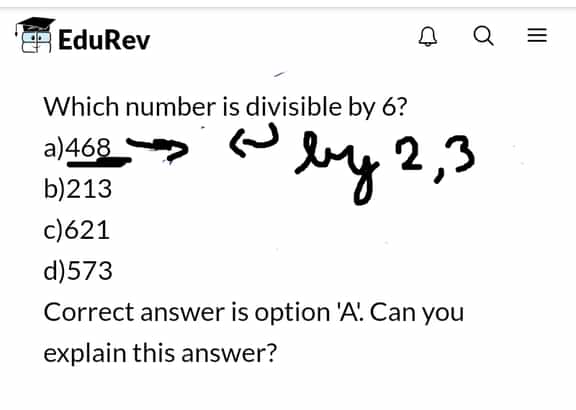
Which number is divisible by 6?- a)468
- b)213
- c)621
- d)573
Correct answer is option 'A'. Can you explain this answer?
Which number is divisible by 6?
a)
468
b)
213
c)
621
d)
573

|
Mouriya Saravanan answered |
The no which divisible by both 2 and 3 is divisible by 6
468 is divisible by 2
4+6+8=18 divisible by 3 so it is divisible by 6 also

How many prime numbers are there between 100 and 200?- a)25
- b)19
- c)21
- d)20
Correct answer is option 'C'. Can you explain this answer?
How many prime numbers are there between 100 and 200?
a)
25
b)
19
c)
21
d)
20
|
|
Raina Kapoor answered |
The number of prime numbers from 100 to 200 is 21.
101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179, 181, 191, 193, 197, 199.
Substitute digits for the letter A to make the following Multiplication problem true. 
- a)2
- b)6
- c)3
- d)5
Correct answer is option 'A'. Can you explain this answer?
Substitute digits for the letter A to make the following Multiplication problem true.

a)
2
b)
6
c)
3
d)
5
|
|
Kavya Saxena answered |
We have A*4=_8 so A can be 2 or 7.now if A is 7,then we have 2 as carried forward so 4*3+2=14_7A,when A=2, we have no carried forward so4*3=12=_2=A. Hence A=2
Which is the least number of 4 digits that is exactly divisible by 13?- a)1052
- b)1039
- c)1032
- d)1001
Correct answer is option 'D'. Can you explain this answer?
Which is the least number of 4 digits that is exactly divisible by 13?
a)
1052
b)
1039
c)
1032
d)
1001

|
Pallabi Choudhury answered |
1001 is divisible by 13, as 1001=13×77.
Identify the multiple of 8.- a)487718
- b)505672
- c)216428
- d)763121
Correct answer is option 'B'. Can you explain this answer?
Identify the multiple of 8.
a)
487718
b)
505672
c)
216428
d)
763121

|
Kavya Chopra answered |
672÷8=84
∴ 505672 is a multiple of 8.

- a)P = 5 and Q = 0
- b)P = 0 and Q = 1
- c)P = 0 and Q = 5
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
a)
P = 5 and Q = 0
b)
P = 0 and Q = 1
c)
P = 0 and Q = 5
d)
None of these
|
|
Ananya Das answered |
As we see the addition part,we have 23PPQ, this means that we don’t have any carried forward for the thousand’s place this means we have only single digit by adding Q and P, so Q+P =P only when Q=0,and now seeing the multiplication we have 3*P=_P which is only possible when P=5.
Write in the usual form: 100 × a + 10 × c + b- a)abc
- b)acb
- c)bac
- d)bca
Correct answer is option 'B'. Can you explain this answer?
Write in the usual form: 100 × a + 10 × c + b
a)
abc
b)
acb
c)
bac
d)
bca
|
|
Amit Sharma answered |
We can write acb=100*a+10*c+b which say that a is at hundred’s place, c is at ten’s place and b is at one’s place.
If the division N ÷2 leaves no remainder (i.e., zero remainder), what might be the one’s digit of N?- a)The one’s digit must be 0, 2, 4, 6 or 8.
- b)The one’s digit must be 1, 3, 5, 7 or 9.
- c)The one’s digit must be 5 or 0.
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
If the division N ÷2 leaves no remainder (i.e., zero remainder), what might be the one’s digit of N?
a)
The one’s digit must be 0, 2, 4, 6 or 8.
b)
The one’s digit must be 1, 3, 5, 7 or 9.
c)
The one’s digit must be 5 or 0.
d)
None of these
|
|
Pooja Shah answered |
Since every multiple of 2 leaves no remainder when divided by 2.Therefore N can be ,0,2,4,6 or 8
Which of the following statements is true?- a)A number is divisible by 4 if it is divisible by 2.
- b)A number is divisible by 9 if it is divisible by 3.
- c)A number is divisible by 11 if the number formed by its last two digits is divisible by 11.
- d)A number is divisible by 8 if the number formed by its last three digits is divisible by 8.
Correct answer is option 'D'. Can you explain this answer?
Which of the following statements is true?
a)
A number is divisible by 4 if it is divisible by 2.
b)
A number is divisible by 9 if it is divisible by 3.
c)
A number is divisible by 11 if the number formed by its last two digits is divisible by 11.
d)
A number is divisible by 8 if the number formed by its last three digits is divisible by 8.

|
Siddharth Mukherjee answered |
The statement in option (D) is true according to the test of divisibility by 8.
What value should be given to * so that the number 653∗47 is divisible by 11?- a)1
- b)6
- c)2
- d)9
Correct answer is option 'A'. Can you explain this answer?
What value should be given to * so that the number 653∗47 is divisible by 11?
a)
1
b)
6
c)
2
d)
9

|
Rounak Sen answered |
Adding the digits at odd places of the given number 653∗ 47,we get 13. Now if the number is divisible by 11, the sum of digits at even places should also be 13, so that 13−13=0 is divisible by 11. We have 7+∗+5=13.
∴ ∗=13−12=1 is the required value.
X is the least composite number between 85 and 100. What is the value of X?- a)85
- b)99
- c)100
- d)86
Correct answer is option 'D'. Can you explain this answer?
X is the least composite number between 85 and 100. What is the value of X?
a)
85
b)
99
c)
100
d)
86
|
|
Rutuja Reddy answered |
**Answer:**
To find the least composite number between 85 and 100, we need to understand what a composite number is.
A composite number is a positive integer greater than 1 that has at least one positive divisor other than 1 and itself. In other words, a composite number can be divided evenly by numbers other than 1 and itself.
Now, let's analyze the numbers between 85 and 100 to determine the least composite number.
- 85: This number can be divided evenly by 1 and 85, but it cannot be divided evenly by any other number. Therefore, it is not a composite number.
- 86: This number can be divided evenly by 1, 2, 43, and 86. Since it has divisors other than 1 and itself, it is a composite number.
- 87: This number can be divided evenly by 1, 3, 29, and 87. Since it has divisors other than 1 and itself, it is a composite number.
- 88: This number can be divided evenly by 1, 2, 4, 8, 11, 22, 44, and 88. Since it has divisors other than 1 and itself, it is a composite number.
- 89: This number is a prime number because it can only be divided evenly by 1 and 89. Therefore, it is not a composite number.
- 90: This number can be divided evenly by 1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, and 90. Since it has divisors other than 1 and itself, it is a composite number.
- 91: This number can be divided evenly by 1, 7, 13, and 91. Since it has divisors other than 1 and itself, it is a composite number.
- 92: This number can be divided evenly by 1, 2, 4, 23, 46, and 92. Since it has divisors other than 1 and itself, it is a composite number.
- 93: This number can be divided evenly by 1, 3, 31, and 93. Since it has divisors other than 1 and itself, it is a composite number.
- 94: This number can be divided evenly by 1, 2, 47, and 94. Since it has divisors other than 1 and itself, it is a composite number.
- 95: This number can be divided evenly by 1, 5, 19, and 95. Since it has divisors other than 1 and itself, it is a composite number.
- 96: This number can be divided evenly by 1, 2, 3, 4, 6, 8, 12, 16, 24, 32, 48, and 96. Since it has divisors other than 1 and itself, it is a composite number.
- 97: This number is a prime number because it can only be divided evenly by 1 and 97. Therefore, it is not a composite number.
- 98: This number can be divided evenly by 1, 2,
To find the least composite number between 85 and 100, we need to understand what a composite number is.
A composite number is a positive integer greater than 1 that has at least one positive divisor other than 1 and itself. In other words, a composite number can be divided evenly by numbers other than 1 and itself.
Now, let's analyze the numbers between 85 and 100 to determine the least composite number.
- 85: This number can be divided evenly by 1 and 85, but it cannot be divided evenly by any other number. Therefore, it is not a composite number.
- 86: This number can be divided evenly by 1, 2, 43, and 86. Since it has divisors other than 1 and itself, it is a composite number.
- 87: This number can be divided evenly by 1, 3, 29, and 87. Since it has divisors other than 1 and itself, it is a composite number.
- 88: This number can be divided evenly by 1, 2, 4, 8, 11, 22, 44, and 88. Since it has divisors other than 1 and itself, it is a composite number.
- 89: This number is a prime number because it can only be divided evenly by 1 and 89. Therefore, it is not a composite number.
- 90: This number can be divided evenly by 1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, and 90. Since it has divisors other than 1 and itself, it is a composite number.
- 91: This number can be divided evenly by 1, 7, 13, and 91. Since it has divisors other than 1 and itself, it is a composite number.
- 92: This number can be divided evenly by 1, 2, 4, 23, 46, and 92. Since it has divisors other than 1 and itself, it is a composite number.
- 93: This number can be divided evenly by 1, 3, 31, and 93. Since it has divisors other than 1 and itself, it is a composite number.
- 94: This number can be divided evenly by 1, 2, 47, and 94. Since it has divisors other than 1 and itself, it is a composite number.
- 95: This number can be divided evenly by 1, 5, 19, and 95. Since it has divisors other than 1 and itself, it is a composite number.
- 96: This number can be divided evenly by 1, 2, 3, 4, 6, 8, 12, 16, 24, 32, 48, and 96. Since it has divisors other than 1 and itself, it is a composite number.
- 97: This number is a prime number because it can only be divided evenly by 1 and 97. Therefore, it is not a composite number.
- 98: This number can be divided evenly by 1, 2,

- a)A = 4 and B = 4
- b)A = 6 and B = 6
- c)A = 4 and B = 6
- d)A = 6 and B = 4
Correct answer is option 'D'. Can you explain this answer?
a)
A = 4 and B = 4
b)
A = 6 and B = 6
c)
A = 4 and B = 6
d)
A = 6 and B = 4
|
|
Ananya Das answered |
Looking at the addition of the hundred’s place we have 7+7+2=_A which gives A=6. Coming back to the multiplication which is A*B=B where A=6 ,so B can be 4 or 6, but when you take B=6, we have 3 as carried forward and 7*6+3 is not equal to 0. Hence B=4 and A=6.
32 + m is a prime number. What is the least value of 'm'?- a)3
- b)5
- c)6
- d)4
Correct answer is option 'B'. Can you explain this answer?
32 + m is a prime number. What is the least value of 'm'?
a)
3
b)
5
c)
6
d)
4

|
Maya Nambiar answered |
The prime number after 32 is 37 which is got by adding 5 to 32.
Which of the following statements is false?- a)If a number is divisible by 8, it must be divisible by 4.
- b)If a number is divisible by both 9 and 10, it is divisible by 90.
- c)The sum of two consecutive odd numbers is always divisible by 4.
- d)If a number is not divisible by both 3 and 4, it is divisible by 12.
Correct answer is option 'D'. Can you explain this answer?
Which of the following statements is false?
a)
If a number is divisible by 8, it must be divisible by 4.
b)
If a number is divisible by both 9 and 10, it is divisible by 90.
c)
The sum of two consecutive odd numbers is always divisible by 4.
d)
If a number is not divisible by both 3 and 4, it is divisible by 12.

|
Siddharth Mukherjee answered |
A number divisible by 3 and 4 is divisible by 12.
If the division N ÷ 5 leaves a remainder of 0, what might be the one’s digit of N?- a)5
- b)7
- c)2
- d)Either 5 or 0
Correct answer is option 'B'. Can you explain this answer?
If the division N ÷ 5 leaves a remainder of 0, what might be the one’s digit of N?
a)
5
b)
7
c)
2
d)
Either 5 or 0
|
|
Stuti Chakraborty answered |
Since every multiple of 5 ends with either 5 or 0. Therefore, the unit digit of N must be either 0+3=3 or 5+3=8. Hence the answer is either 3 or 8.
Write in generalised form: 25- a)10 × 5 + 2
- b)10 × 5 + 3
- c)10 × 2 + 5
- d)10 × 3 + 5
Correct answer is option 'C'. Can you explain this answer?
Write in generalised form: 25
a)
10 × 5 + 2
b)
10 × 5 + 3
c)
10 × 2 + 5
d)
10 × 3 + 5

|
P. Aparajitha answered |
10 × 2 = 20
20 + 5 = 25
:: Hence the answer is 25 !:)
20 + 5 = 25
:: Hence the answer is 25 !:)
Which of the given numbers is composite?- a)137
- b)147
- c)157
- d)167
Correct answer is option 'B'. Can you explain this answer?
Which of the given numbers is composite?
a)
137
b)
147
c)
157
d)
167

|
Rounak Sen answered |
147=7×21 is a composite number.
A prime number is greater than 30 and lesser than 60. What is the greatest possible such number?- a)37
- b)53
- c)43
- d)59
Correct answer is option 'D'. Can you explain this answer?
A prime number is greater than 30 and lesser than 60. What is the greatest possible such number?
a)
37
b)
53
c)
43
d)
59

|
Pranab Chawla answered |
The prime numbers between 30 and 60 are 31, 37, 41, 43, 47, 53 and 59. The greatest of these is 59.
N is a 5-digit number divisible by 5. If N is bigger than 10000 and smaller than 10010, what is the value of N?- a)10000
- b)10010
- c)10005
- d)10001
Correct answer is option 'C'. Can you explain this answer?
N is a 5-digit number divisible by 5. If N is bigger than 10000 and smaller than 10010, what is the value of N?
a)
10000
b)
10010
c)
10005
d)
10001

|
Anoushka Das answered |
According to the problem, a five-digit number between 10000 and 10010 divisible by 5 is 10005.
When is a number always divisible by 90?- a)If it is divisible by both 2 and 45.
- b)If it is not divisible by both 5 and 18.
- c)If it is not divisible by both 9 and 10.
- d)If it is divisible by 3 and 20.
Correct answer is option 'A'. Can you explain this answer?
When is a number always divisible by 90?
a)
If it is divisible by both 2 and 45.
b)
If it is not divisible by both 5 and 18.
c)
If it is not divisible by both 9 and 10.
d)
If it is divisible by 3 and 20.
|
|
Sarita Verma answered |
A number is always divisible by 90 if it is divisible by both 2 and 45. This is because 90 is the product of 2335, so a number that is divisible by both 2 and 45 (which is the product of 33*5) is also divisible by 90.
Chapter doubts & questions for Playing with Numbers - Online MCQ Tests for Class 8 2025 is part of Class 8 exam preparation. The chapters have been prepared according to the Class 8 exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Class 8 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Playing with Numbers - Online MCQ Tests for Class 8 in English & Hindi are available as part of Class 8 exam.
Download more important topics, notes, lectures and mock test series for Class 8 Exam by signing up for free.
Signup to see your scores go up within 7 days!
Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Forgot Password
OR
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup














