Test: Problem Solving- 2 - UPSC MCQ
15 Questions MCQ Test CSAT Preparation - Test: Problem Solving- 2
Six points lie on a circle. How many quadrilaterals can be drawn joining these points?
There are 3 children of a lady. In how many ways is it possible to dress them for a party if the first child likes 3 dresses, second likes 4 and the third likes 5 but the third child has out grown one of them? Each child has a different set of clothes.
How many three-digit odd numbers can be formed from the digits 1, 3, 5, 0 and 8? [repetition is allowed ]
Find the number of words formed by permuting all the letters of the word INDEPENDENCE.
There are 12 children in a party. For a game they have to be paired up. How many different pairs can be made for the game?
How many different differences can be obtained by taking only 2 numbers at a time from 3, 5,2,10 and 15?
In a fruit market, watermelon costs Rs 25 per fruit, mango costs Rs 20 per fruit, an apple costs Rs 15 per fruit, and orange costs Rs 10 per fruit. If Indu bought 2 watermelons, 5 mangoes, 3 apples, and some oranges and paid a bill of Rs 285, what was the number of oranges purchased by her?
ow many five digit numbers can be formed using the digits 0, 2, 3,4and 5, when repetition is allowed such that the number formed is divisible by 2 and 5?
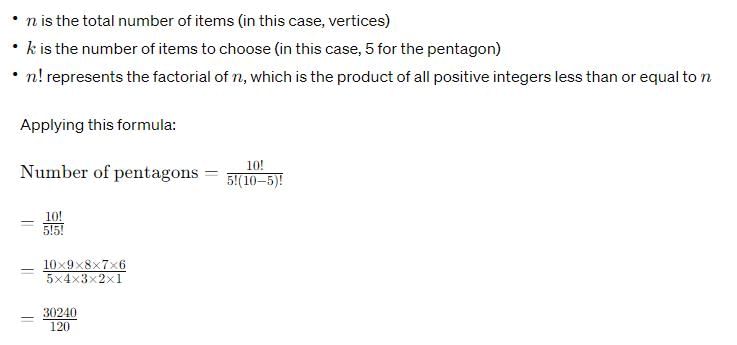
How many pentagons can be drawn by joining the vertices of a polygon with 10 sides?
Find the number of words formed by permuting all the letters of the word INDEPENDENCE such that the E???s do not come together.
Ten different letters of an alphabet are given. Words with 6 letters are formed with these alphabets. How many such words can be formed when repetition is not allowed in any word?
A polygon has 20 diagonals. How many sides does it have?
A box contains 7 red, 6 white and 4 blue balls. In how many ways can a selection of 3 balls be made,if all the balls are red ?
|
208 videos|138 docs|138 tests
|