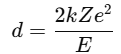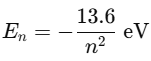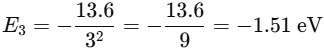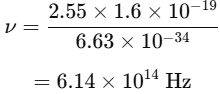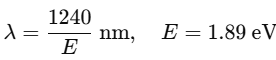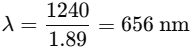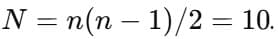The size of a hydrogen atom is about 10⁻¹⁰ m, but its nucleus is only about 10⁻¹⁵ m. What does this tell us?

Most of the atom is empty space!This size difference supports the Bohr model and quantum mechanical models, which describe electrons as occupying a large region around the nucleus rather than being inside it.

What is the approximate speed of an electron in a hydrogen atom's first orbit?

Around 2.2 × 10⁶ m/s—that’s about 1% the speed of light!

Each element has a unique spectral fingerprint. What do we call the visible set of hydrogen’s spectral lines?

The Balmer Series—these are the bright emission lines we see from hydrogen.

In Rutherford’s gold foil experiment, what fraction of α-particles were deflected by more than 90°?

Only 1 in 8000! That’s why the discovery of the nucleus was such a surprise—no one expected such strong repulsion.

According to Bohr, why don’t electrons spiral into the nucleus?

They can only exist in specific quantized orbits—no energy loss unless they jump between levels!

An electron in a hydrogen atom drops from n = 3 to n = 2. What’s the wavelength of the emitted photon?
(λ=1240/E, where 𝐸 = 1.89 E=1.89 eV.)

656 nm—this is the famous red line of hydrogen’s spectrum! 

If Bohr’s quantization rule applied to planets, what would the Earth’s quantum number be in its orbit around the Sun?

Around 10⁷⁴—so large that it behaves classically!

What happens if an electron in a hydrogen atom jumps to n = ∞?

The atom ionizes—the electron completely escapes, and the atom is no longer neutral!

De Broglie suggested electrons act like waves. What must be true for an orbit to exist?

The electron’s wavelength must fit perfectly in the orbit, forming a standing wave.

If an electron absorbs a photon, what happens?

It jumps to a higher energy level—but only if the photon has exactly the right energy!

If most of the atom is empty space, where is nearly all its mass located?

In the nucleus—it contains protons and neutrons, which are about 2000 times heavier than electrons!

What’s faster: an electron jumping between energy levels or the blink of an eye?

Electron jumps happen in about a billionth of a second (10^-9 s), much faster than blinking (~0.1 s).

If an alpha particle is positively charged, why did some of them bounce back in Rutherford’s experiment?

They collided with the tiny, dense, positively charged nucleus, experiencing strong repulsion.

How much energy does a hydrogen atom need to completely ionize from its ground state?

13.6 eV—this is the energy needed to remove the electron from n = 1 to n = ∞.

Why don’t electrons exist between energy levels?

Energy levels are quantized, meaning electrons can only exist in fixed orbits where their wave fits perfectly.

The Balmer series appears in the visible spectrum. What’s the color of the longest wavelength transition?

Red—at 656 nm, when an electron falls from n = 3 to n = 2.

An alpha particle (charge = +2e) moves toward a gold nucleus (Z = 79) with 7.7 MeV energy. 
Find its closest approach distance using:

3.0 × 10^-14 m (or 30 fm)

Find the energy of an electron in the third orbit of hydrogen. Use:

An electron in hydrogen jumps from n = 4 to n = 2. The energy released is 2.55 eV. Find the emitted photon's frequency using:
E = hν, h = 6.63 × 10⁻³⁴Js, 1eV = 1.6 × 10⁻¹⁹J

Find the wavelength of the emitted photon for the n = 3 to n = 2 transition. Use:

What if Bohr’s quantization applied to planetary motion? What would Earth’s quantum number be?

~10⁷⁴ so huge that planets behave classically, unlike electrons!

What happens to a hydrogen atom inside a star like the Sun?

At extreme temperatures, electrons are stripped away, forming a plasma!

If a hydrogen atom emits 10 photons, how many electrons changed energy levels?

At least 1 electron! A single electron can emit multiple photons as it cascades down energy levels.

How much energy is needed to completely ionize a hydrogen atom from n = 3?