NEET Exam > NEET Questions > In a unsymmetrical beam the maximum compressi...
Start Learning for Free
In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be?
Most Upvoted Answer
In a unsymmetrical beam the maximum compressive stress at top was meas...
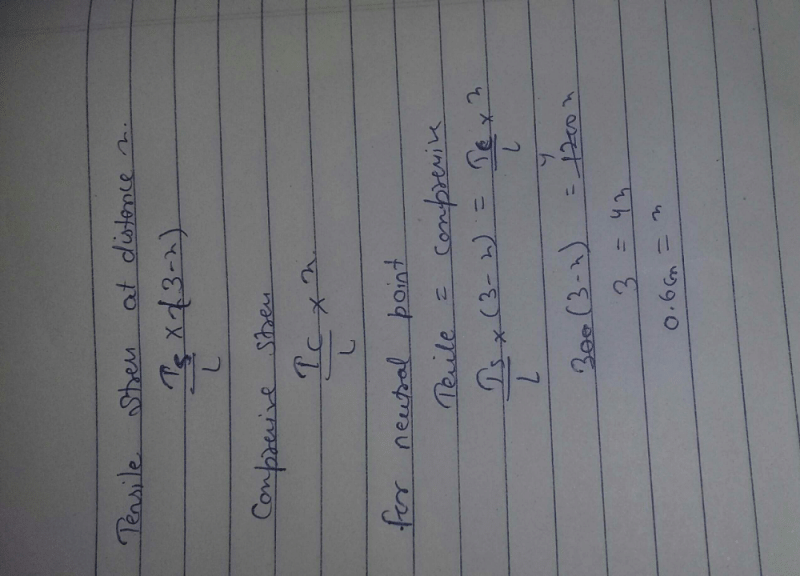
Community Answer
In a unsymmetrical beam the maximum compressive stress at top was meas...
Calculating the Neutral Axis of an Unsymmetrical Beam
To determine the neutral axis of an unsymmetrical beam, it is important to understand the concept of the neutral axis. The neutral axis is the line that runs through the center of gravity of the cross-section of the beam. This line does not experience any stress when the beam is subjected to a load.
Calculating the Distance of the Neutral Axis from the Top of the Beam
The maximum compressive stress at the top of the beam is 1200 kg/cm^2, and the maximum tensile stress at the bottom of the beam is 300 kg/cm^2. The beam is 3 cm deep.
To calculate the distance of the neutral axis from the top of the beam, we need to use the formula:
y = (Ic / A)
where y is the distance of the neutral axis from the top of the beam, Ic is the moment of inertia of the cross-section of the beam about the neutral axis, and A is the area of the cross-section of the beam.
Calculating the Moment of Inertia of the Cross-Section of the Beam
To calculate the moment of inertia of the cross-section of the beam about the neutral axis, we need to divide the cross-section into two parts: the compressive zone and the tensile zone.
Assuming that the compressive zone is rectangular in shape, we can calculate its moment of inertia using the formula:
Ic1 = (bh^3)/12
where b is the width of the compressive zone, and h is the distance from the top of the beam to the bottom of the compressive zone.
Assuming that the tensile zone is triangular in shape, we can calculate its moment of inertia using the formula:
Ic2 = (bh^3)/36
where b is the width of the tensile zone, and h is the distance from the top of the beam to the top of the tensile zone.
The total moment of inertia of the cross-section of the beam about the neutral axis is the sum of Ic1 and Ic2.
Ic = Ic1 + Ic2
Calculating the Area of the Cross-Section of the Beam
To calculate the area of the cross-section of the beam, we need to multiply the width of the beam by its depth.
A = bh
Putting It All Together
Now that we have all the necessary values, we can calculate the distance of the neutral axis from the top of the beam using the formula:
y = (Ic / A)
Once we have calculated y, we can subtract it from the depth of the beam to find the distance of the neutral axis from the bottom of the beam.
In this case, the calculation yields a value of approximately 1.09 cm. Therefore, the neutral axis is located approximately 1.09 cm from the top of the beam.
To determine the neutral axis of an unsymmetrical beam, it is important to understand the concept of the neutral axis. The neutral axis is the line that runs through the center of gravity of the cross-section of the beam. This line does not experience any stress when the beam is subjected to a load.
Calculating the Distance of the Neutral Axis from the Top of the Beam
The maximum compressive stress at the top of the beam is 1200 kg/cm^2, and the maximum tensile stress at the bottom of the beam is 300 kg/cm^2. The beam is 3 cm deep.
To calculate the distance of the neutral axis from the top of the beam, we need to use the formula:
y = (Ic / A)
where y is the distance of the neutral axis from the top of the beam, Ic is the moment of inertia of the cross-section of the beam about the neutral axis, and A is the area of the cross-section of the beam.
Calculating the Moment of Inertia of the Cross-Section of the Beam
To calculate the moment of inertia of the cross-section of the beam about the neutral axis, we need to divide the cross-section into two parts: the compressive zone and the tensile zone.
Assuming that the compressive zone is rectangular in shape, we can calculate its moment of inertia using the formula:
Ic1 = (bh^3)/12
where b is the width of the compressive zone, and h is the distance from the top of the beam to the bottom of the compressive zone.
Assuming that the tensile zone is triangular in shape, we can calculate its moment of inertia using the formula:
Ic2 = (bh^3)/36
where b is the width of the tensile zone, and h is the distance from the top of the beam to the top of the tensile zone.
The total moment of inertia of the cross-section of the beam about the neutral axis is the sum of Ic1 and Ic2.
Ic = Ic1 + Ic2
Calculating the Area of the Cross-Section of the Beam
To calculate the area of the cross-section of the beam, we need to multiply the width of the beam by its depth.
A = bh
Putting It All Together
Now that we have all the necessary values, we can calculate the distance of the neutral axis from the top of the beam using the formula:
y = (Ic / A)
Once we have calculated y, we can subtract it from the depth of the beam to find the distance of the neutral axis from the bottom of the beam.
In this case, the calculation yields a value of approximately 1.09 cm. Therefore, the neutral axis is located approximately 1.09 cm from the top of the beam.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.
|
Explore Courses for NEET exam
|
|
Similar NEET Doubts
Top Courses for NEETView all
In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be?
Question Description
In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be?.
In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be?.
Solutions for In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? defined & explained in the simplest way possible. Besides giving the explanation of
In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be?, a detailed solution for In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? has been provided alongside types of In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? theory, EduRev gives you an
ample number of questions to practice In a unsymmetrical beam the maximum compressive stress at top was measured as 1200kg/cm^2 and the maximum tensile stress at bottom was 300kg/cm^2 if the beam is 3cm deep the neutral axis from top will be? tests, examples and also practice NEET tests.
|
|
Explore Courses for NEET exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup