Class 9 Exam > Class 9 Questions > Class 9 Chapter 1 ex 1.2 Question 3 Show that...
Start Learning for Free
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line?
Most Upvoted Answer
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be repres...
Community Answer
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be repres...
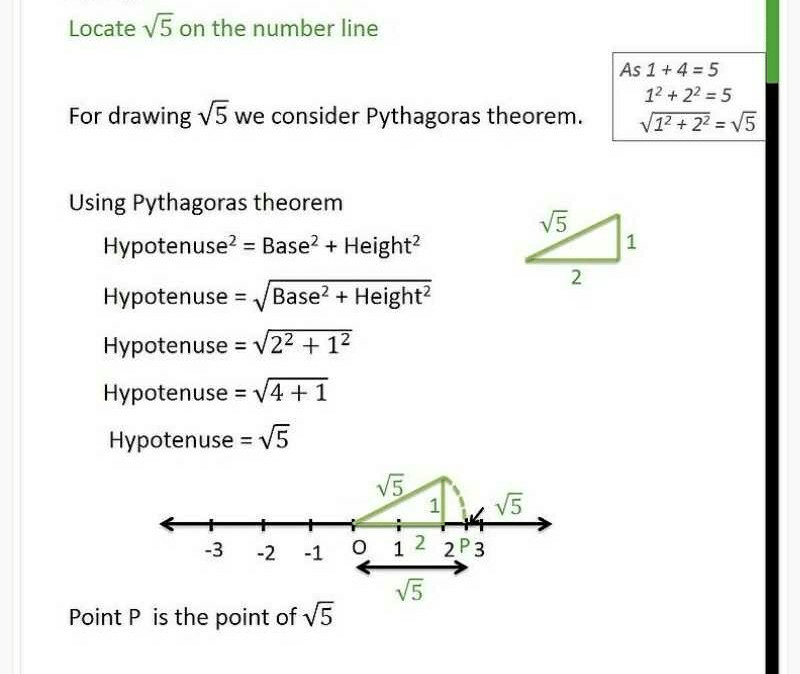
Introduction:
Representing irrational numbers on a number line is a common way to visualize them. In this question, we will show how the irrational number underoot 5 can be represented on a number line.
Method:
To represent underoot 5 on a number line, we will follow the steps below:
1. Draw a horizontal number line and mark a point as 0.
2. Mark another point as 1 to the right of 0.
3. Draw a line perpendicular to the number line at point 1.
4. Mark a point on this perpendicular line at a distance of underoot 5 units from point 1.
5. Label this point as underoot 5.
Explanation:
Underoot 5 is an irrational number that lies between the integers 2 and 3. To represent it on a number line, we start by drawing a horizontal line and marking a point as 0. We then mark another point as 1 to the right of 0. This represents the integer 1.
Next, we draw a line perpendicular to the number line at point 1. This line represents the square root of 5 units. To find the length of this line, we use the Pythagorean theorem:
a^2 + b^2 = c^2
Here, a = 1 unit, b = underoot 5 units, and c = the length of the perpendicular line. We can solve for c as follows:
1^2 + underoot 5^2 = c^2
1 + 5 = c^2
6 = c^2
c = underoot 6 units
We then mark a point on this perpendicular line at a distance of underoot 5 units from point 1. This point represents underoot 5 on the number line.
Conclusion:
In conclusion, underoot 5 can be represented on a number line by drawing a perpendicular line at point 1 and marking a point on this line at a distance of underoot 5 units from point 1. This point represents the irrational number underoot 5.
Representing irrational numbers on a number line is a common way to visualize them. In this question, we will show how the irrational number underoot 5 can be represented on a number line.
Method:
To represent underoot 5 on a number line, we will follow the steps below:
1. Draw a horizontal number line and mark a point as 0.
2. Mark another point as 1 to the right of 0.
3. Draw a line perpendicular to the number line at point 1.
4. Mark a point on this perpendicular line at a distance of underoot 5 units from point 1.
5. Label this point as underoot 5.
Explanation:
Underoot 5 is an irrational number that lies between the integers 2 and 3. To represent it on a number line, we start by drawing a horizontal line and marking a point as 0. We then mark another point as 1 to the right of 0. This represents the integer 1.
Next, we draw a line perpendicular to the number line at point 1. This line represents the square root of 5 units. To find the length of this line, we use the Pythagorean theorem:
a^2 + b^2 = c^2
Here, a = 1 unit, b = underoot 5 units, and c = the length of the perpendicular line. We can solve for c as follows:
1^2 + underoot 5^2 = c^2
1 + 5 = c^2
6 = c^2
c = underoot 6 units
We then mark a point on this perpendicular line at a distance of underoot 5 units from point 1. This point represents underoot 5 on the number line.
Conclusion:
In conclusion, underoot 5 can be represented on a number line by drawing a perpendicular line at point 1 and marking a point on this line at a distance of underoot 5 units from point 1. This point represents the irrational number underoot 5.

|
Explore Courses for Class 9 exam
|

|
Similar Class 9 Doubts
Question Description
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? for Class 9 2025 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? covers all topics & solutions for Class 9 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line?.
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? for Class 9 2025 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? covers all topics & solutions for Class 9 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line?.
Solutions for Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? in English & in Hindi are available as part of our courses for Class 9.
Download more important topics, notes, lectures and mock test series for Class 9 Exam by signing up for free.
Here you can find the meaning of Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? defined & explained in the simplest way possible. Besides giving the explanation of
Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line?, a detailed solution for Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? has been provided alongside types of Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? theory, EduRev gives you an
ample number of questions to practice Class 9 Chapter 1 ex 1.2 Question 3 Show that Underoot 5 can be represented on the number line? tests, examples and also practice Class 9 tests.

|
Explore Courses for Class 9 exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.






















