Class 12 Exam > Class 12 Questions > Derivation of electric field due to a linear ...
Start Learning for Free
Derivation of electric field due to a linear charge distribution?
Most Upvoted Answer
Derivation of electric field due to a linear charge distribution?
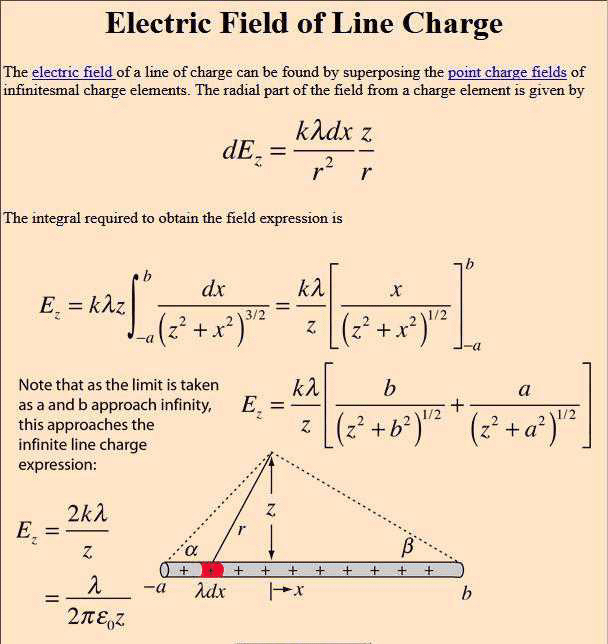
Community Answer
Derivation of electric field due to a linear charge distribution?
**Derivation of Electric Field due to a Linear Charge Distribution**
To derive the electric field due to a linear charge distribution, we can consider a small element of the distribution and calculate the electric field produced by that element. We can then integrate over all the elements to obtain the total electric field.
**1. Consider a small element of the linear charge distribution:**
Let's consider a small element of the linear charge distribution with length dl and charge dq. We can assume this element is located at a distance r from the point where we want to calculate the electric field.
**2. Calculate the electric field produced by the small element:**
The electric field produced by the small element can be calculated using Coulomb's law. Coulomb's law states that the electric field produced by a point charge is given by:
E = k * (dq) / r^2
where E is the electric field, k is Coulomb's constant (k = 1 / (4πε₀) where ε₀ is the permittivity of free space), dq is the charge of the small element, and r is the distance between the small element and the point where we want to calculate the electric field.
**3. Integrate over all the elements:**
To find the total electric field due to the linear charge distribution, we need to integrate the electric field produced by each small element over the entire length of the distribution.
∫ dE = ∫ k * (dq) / r^2
**4. Convert the integration variable:**
We can express the integration variable in terms of the position along the linear charge distribution. Let's assume the linear charge density of the distribution is λ, which is defined as the charge per unit length.
dq = λ * dl
Substituting this into the integral, we have:
∫ dE = ∫ k * λ * dl / r^2
**5. Solve the integral:**
Integrating both sides of the equation, we have:
E = k * λ * ∫ dl / r^2
The integral of dl over the length of the linear charge distribution is simply the length of the distribution L. Therefore, the equation becomes:
E = k * λ * L / r^2
**6. Final Result:**
The final expression for the electric field due to a linear charge distribution is:
E = k * λ * L / r^2
where E is the electric field, λ is the linear charge density, L is the length of the distribution, r is the distance between the point where we want to calculate the electric field and the linear charge distribution, and k is Coulomb's constant.
This derivation allows us to calculate the electric field at any point in space due to a linear charge distribution, given the linear charge density and the distance from the distribution.
To derive the electric field due to a linear charge distribution, we can consider a small element of the distribution and calculate the electric field produced by that element. We can then integrate over all the elements to obtain the total electric field.
**1. Consider a small element of the linear charge distribution:**
Let's consider a small element of the linear charge distribution with length dl and charge dq. We can assume this element is located at a distance r from the point where we want to calculate the electric field.
**2. Calculate the electric field produced by the small element:**
The electric field produced by the small element can be calculated using Coulomb's law. Coulomb's law states that the electric field produced by a point charge is given by:
E = k * (dq) / r^2
where E is the electric field, k is Coulomb's constant (k = 1 / (4πε₀) where ε₀ is the permittivity of free space), dq is the charge of the small element, and r is the distance between the small element and the point where we want to calculate the electric field.
**3. Integrate over all the elements:**
To find the total electric field due to the linear charge distribution, we need to integrate the electric field produced by each small element over the entire length of the distribution.
∫ dE = ∫ k * (dq) / r^2
**4. Convert the integration variable:**
We can express the integration variable in terms of the position along the linear charge distribution. Let's assume the linear charge density of the distribution is λ, which is defined as the charge per unit length.
dq = λ * dl
Substituting this into the integral, we have:
∫ dE = ∫ k * λ * dl / r^2
**5. Solve the integral:**
Integrating both sides of the equation, we have:
E = k * λ * ∫ dl / r^2
The integral of dl over the length of the linear charge distribution is simply the length of the distribution L. Therefore, the equation becomes:
E = k * λ * L / r^2
**6. Final Result:**
The final expression for the electric field due to a linear charge distribution is:
E = k * λ * L / r^2
where E is the electric field, λ is the linear charge density, L is the length of the distribution, r is the distance between the point where we want to calculate the electric field and the linear charge distribution, and k is Coulomb's constant.
This derivation allows us to calculate the electric field at any point in space due to a linear charge distribution, given the linear charge density and the distance from the distribution.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
Question Description
Derivation of electric field due to a linear charge distribution? for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Derivation of electric field due to a linear charge distribution? covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derivation of electric field due to a linear charge distribution?.
Derivation of electric field due to a linear charge distribution? for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Derivation of electric field due to a linear charge distribution? covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derivation of electric field due to a linear charge distribution?.
Solutions for Derivation of electric field due to a linear charge distribution? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of Derivation of electric field due to a linear charge distribution? defined & explained in the simplest way possible. Besides giving the explanation of
Derivation of electric field due to a linear charge distribution?, a detailed solution for Derivation of electric field due to a linear charge distribution? has been provided alongside types of Derivation of electric field due to a linear charge distribution? theory, EduRev gives you an
ample number of questions to practice Derivation of electric field due to a linear charge distribution? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.




















