Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > A sand layer of 15 m is overlain by 7.5 m cla...
Start Learning for Free
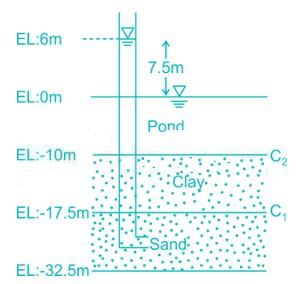
A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.
Take γsat for clay and sand layer to be 20 kN/m3 and 19 kN/m3 respectively and permeability of clay layer is 1.5 × 10–5 cm/sec.
Use γw =10 kN/m3
Correct answer is '12.7-13'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A sand layer of 15 m is overlain by 7.5 m clay layer which is further ...
Concept:
Using Darcy’s law
q = kiA
k → permeability of soil
i → hydraulic gradient
A → Area of Soil Sample
Calculation:
hydraulic gradient (i) is = 

h = head causing flow in the clay layer
Take C1 as datum
Total head at C1 = Datum head + Pressure head
= 0 + (7.5 + 10 + 7.5) = 25 m
Total head at C2 = Datum head + Pressure head
= 7.5 + 10
= 17.5 m
(T.H)C1 > (T.H)C2
Flow is occurring from C1 to C2 (upward)
Head causing the flow = (T.H)c1 − (T.H)c2
= 25 – 17.5
HL = 7.5m
L = 7.5m

A = 1 m2
k = 1.5 × 10–5 cm/sec
k = 1.5 × 10–7 m/sec
q = 1.5 × 10–7 × 1 × 1
q = 1.5 × 10–7 × 60 × 60 × 24 × 1 × 1
q = 12. 96 × 10–3 m3/day
q = 12.96 litres/day
Most Upvoted Answer
A sand layer of 15 m is overlain by 7.5 m clay layer which is further ...
To calculate the quantity of flow into the pond from the clay layer, we need to determine the flow rate through the clay layer.
The flow rate through a porous medium can be calculated using Darcy's law:
Q = K * A * h / L
Where:
Q = flow rate (in cubic meters per second)
K = hydraulic conductivity of the medium (in meters per second)
A = cross-sectional area of flow (in square meters)
h = hydraulic head difference (in meters)
L = length of flow path (in meters)
In this case, the flow path is the thickness of the clay layer (7.5 m), and the hydraulic head difference is the rise in elevation observed in the standpipe (7.5 m).
First, we need to convert the flow rate from cubic meters per second to liters per day. There are 86,400 seconds in a day, and 1 cubic meter is equal to 1,000 liters.
Q = (K * A * h / L) * 86,400 * 1,000
To calculate the cross-sectional area of flow (A), we need to know the dimensions of the pond. Let's assume the pond is a square with a side length of S meters.
A = S^2
Now we can calculate the flow rate per square meter of the pond bed:
Flow rate per square meter = Q / (S^2)
Substituting the given values:
Flow rate per square meter = (K * A * h / L) * 86,400 * 1,000 / (S^2)
We still need the value of the hydraulic conductivity (K) for the clay layer. Unfortunately, that information is not provided in the question. Without this value, we cannot calculate the flow rate accurately.
The flow rate through a porous medium can be calculated using Darcy's law:
Q = K * A * h / L
Where:
Q = flow rate (in cubic meters per second)
K = hydraulic conductivity of the medium (in meters per second)
A = cross-sectional area of flow (in square meters)
h = hydraulic head difference (in meters)
L = length of flow path (in meters)
In this case, the flow path is the thickness of the clay layer (7.5 m), and the hydraulic head difference is the rise in elevation observed in the standpipe (7.5 m).
First, we need to convert the flow rate from cubic meters per second to liters per day. There are 86,400 seconds in a day, and 1 cubic meter is equal to 1,000 liters.
Q = (K * A * h / L) * 86,400 * 1,000
To calculate the cross-sectional area of flow (A), we need to know the dimensions of the pond. Let's assume the pond is a square with a side length of S meters.
A = S^2
Now we can calculate the flow rate per square meter of the pond bed:
Flow rate per square meter = Q / (S^2)
Substituting the given values:
Flow rate per square meter = (K * A * h / L) * 86,400 * 1,000 / (S^2)
We still need the value of the hydraulic conductivity (K) for the clay layer. Unfortunately, that information is not provided in the question. Without this value, we cannot calculate the flow rate accurately.
Attention Civil Engineering (CE) Students!
To make sure you are not studying endlessly, EduRev has designed Civil Engineering (CE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Civil Engineering (CE).

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer?
Question Description
A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer?.
A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer?.
Solutions for A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer?, a detailed solution for A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? has been provided alongside types of A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A sand layer of 15 m is overlain by 7.5 m clay layer which is further overlain by 10 m deep pond. A standpipe is inserted in the sand layer at the bottom of clay stratum and rise of Elevation 7.5 m is observed in the standpipe due to artesian pressure in the sand stratum. Calculate the quantity of flow into the pond in litres per day from the clay layer per meter square of the pond bed.Take γsatfor clay and sand layer to be 20 kN/m3and 19 kN/m3respectively and permeability of clay layer is 1.5 × 10–5cm/sec.Use γw=10 kN/m3Correct answer is '12.7-13'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























