Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > A reinforced Concrete column of size 450mm &t...
Start Learning for Free
A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.
Use M-20 concrete and Fe-415 steel.
Correct answer is '2500-2520'. Can you explain this answer?
Verified Answer
A reinforced Concrete column of size 450mm × 550mm (overall dept...
Follow step by step method:
(i) check for short or long column
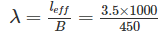
λ = 7.77 < 12
it is a short column
(ii) check for Minimum eccentricity
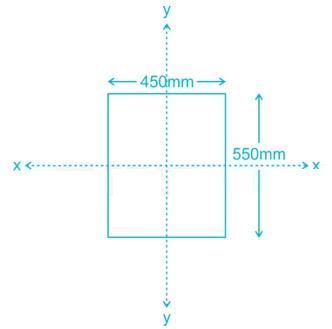
Minimum eccentricity (ex-x) is greater of

(ii) 20 mm
So, ex-x = 25.33 mm
ex-x < 0.05 × 550
25.33 < 27.5 mm
(O.K)
For ey−y = 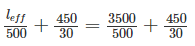 = 22mm
= 22mm
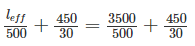 = 22mm
= 22mmey-y < 0.05 × 450
22 mm < 22.5 mm
(O.K)
So, Load carrying capacity of the column will be
Pu = 0.4 fck Ac + 0.67fy Asc
fck = 20 N/mm2
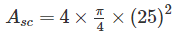
= 1963.50mm2
fy = 415 N/mm2
Pu = 0.4 × 20 × [450 × 550 – 1963.50] + 0.67 × 415 × 1963.50
Pu = 2510.24 kN
Most Upvoted Answer
A reinforced Concrete column of size 450mm × 550mm (overall dept...
X 450mm is designed to carry a compressive load of 2000kN. The column is reinforced with four bars of 25mm diameter. The concrete strength is assumed to be 30MPa and the steel strength is assumed to be 415MPa.
The first step in designing the column is to calculate the effective length of the column. The effective length is the length at which the column will buckle under the applied load. For a fixed-fixed column, the effective length is equal to the actual length of the column.
The next step is to calculate the slenderness ratio of the column. The slenderness ratio is the ratio of the effective length to the radius of gyration of the column cross-section. The radius of gyration is a measure of the distribution of material around the centroid of the cross-section. For a square column, the radius of gyration can be calculated as:
r = (b/2) x (1/√3)
where b is the width of the column.
For a 450mm x 450mm column, the radius of gyration is:
r = (450/2) x (1/√3) = 129.9mm
The effective length of the column is equal to the actual length of the column, which is assumed to be 3m.
Therefore, the slenderness ratio of the column is:
λ = L/r = 3000/129.9 = 23.09
The next step is to calculate the design compressive strength of the column. The design compressive strength is the compressive strength of the concrete multiplied by a factor that takes into account the slenderness ratio of the column. The factor is called the slenderness ratio factor and is given by the following equation:
φ = 0.65 + (0.35/λ) = 0.65 + (0.35/23.09) = 0.665
The design compressive strength of the column is therefore:
fcd = φ x fck = 0.665 x 30 = 19.95MPa
The next step is to calculate the area of steel required to resist the compressive load. The area of steel required can be calculated using the following equation:
As = (0.85 x fcd x Ac) / fyk
where Ac is the cross-sectional area of the column and fyk is the yield strength of the steel.
The cross-sectional area of the column is:
Ac = b x h = 450 x 450 = 202,500mm²
The yield strength of the steel is assumed to be 415MPa.
Therefore, the area of steel required is:
As = (0.85 x 19.95 x 202,500) / 415 = 8,159mm²
The area of steel required is divided equally between the four bars of 25mm diameter. The area of each bar is:
Abar = (π/4) x d² = (π/4) x 25² = 490.9mm²
Therefore, the number of bars required is:
n = As / Abar = 8,159 / 490.9 = 16.6
Since the number of bars must be a whole number, 17 bars are required.
The final step is to check the spacing of the bars. The minimum spacing
The first step in designing the column is to calculate the effective length of the column. The effective length is the length at which the column will buckle under the applied load. For a fixed-fixed column, the effective length is equal to the actual length of the column.
The next step is to calculate the slenderness ratio of the column. The slenderness ratio is the ratio of the effective length to the radius of gyration of the column cross-section. The radius of gyration is a measure of the distribution of material around the centroid of the cross-section. For a square column, the radius of gyration can be calculated as:
r = (b/2) x (1/√3)
where b is the width of the column.
For a 450mm x 450mm column, the radius of gyration is:
r = (450/2) x (1/√3) = 129.9mm
The effective length of the column is equal to the actual length of the column, which is assumed to be 3m.
Therefore, the slenderness ratio of the column is:
λ = L/r = 3000/129.9 = 23.09
The next step is to calculate the design compressive strength of the column. The design compressive strength is the compressive strength of the concrete multiplied by a factor that takes into account the slenderness ratio of the column. The factor is called the slenderness ratio factor and is given by the following equation:
φ = 0.65 + (0.35/λ) = 0.65 + (0.35/23.09) = 0.665
The design compressive strength of the column is therefore:
fcd = φ x fck = 0.665 x 30 = 19.95MPa
The next step is to calculate the area of steel required to resist the compressive load. The area of steel required can be calculated using the following equation:
As = (0.85 x fcd x Ac) / fyk
where Ac is the cross-sectional area of the column and fyk is the yield strength of the steel.
The cross-sectional area of the column is:
Ac = b x h = 450 x 450 = 202,500mm²
The yield strength of the steel is assumed to be 415MPa.
Therefore, the area of steel required is:
As = (0.85 x 19.95 x 202,500) / 415 = 8,159mm²
The area of steel required is divided equally between the four bars of 25mm diameter. The area of each bar is:
Abar = (π/4) x d² = (π/4) x 25² = 490.9mm²
Therefore, the number of bars required is:
n = As / Abar = 8,159 / 490.9 = 16.6
Since the number of bars must be a whole number, 17 bars are required.
The final step is to check the spacing of the bars. The minimum spacing
Free Test
FREE
| Start Free Test |
Community Answer
A reinforced Concrete column of size 450mm × 550mm (overall dept...
Follow step by step method:
(i) check for short or long column
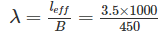
λ = 7.77 < 12
it is a short column
(ii) check for Minimum eccentricity

Minimum eccentricity (ex-x) is greater of

(ii) 20 mm
So, ex-x = 25.33 mm
ex-x < 0.05 × 550
25.33 < 27.5 mm
(O.K)
For ey−y = 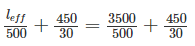 = 22mm
= 22mm
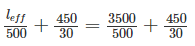 = 22mm
= 22mmey-y < 0.05 × 450
22 mm < 22.5 mm
(O.K)
So, Load carrying capacity of the column will be
Pu = 0.4 fck Ac + 0.67fy Asc
fck = 20 N/mm2
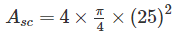
= 1963.50mm2
fy = 415 N/mm2
Pu = 0.4 × 20 × [450 × 550 – 1963.50] + 0.67 × 415 × 1963.50
Pu = 2510.24 kN

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer?
Question Description
A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer?.
A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer?.
Solutions for A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer?, a detailed solution for A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? has been provided alongside types of A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A reinforced Concrete column of size 450mm × 550mm (overall depth) is provided with area of steel in compression as 4 bars of 25mm diameter. If the effective length of the column is 3.5m, the ultimate load carrying capacity of the column will be _______kN.Use M-20 concrete and Fe-415 steel.Correct answer is '2500-2520'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















