Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > A cantilever beam of rectangular cross-sectio...
Start Learning for Free
A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)
Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.
Correct answer is '4.4-4.6'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A cantilever beam of rectangular cross-section having 20 m span is sub...
As per IS 456: 2000, clause 23.2.1
For cantilever beam:
For span ≤ 10m
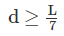
For, span > 10m, the limit of deflection criteria is modified.
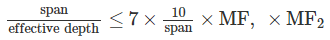
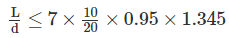
Where MF1 = Modification factor for tension = 0.95
MF2 = Modification factor for compression = 1.345
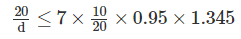
d ≥ 4.47m
Most Upvoted Answer
A cantilever beam of rectangular cross-section having 20 m span is sub...
Given Data:
- Span of the cantilever beam (L) = 20 m
- Uniformly distributed load (w)
- Modification factor for tension reinforcement (m_t) = 0.95
- Modification factor for compression reinforcement (m_c) = 1.345
Minimum Effective Depth for Deflection Control:
The minimum effective depth required to control deflection in a cantilever beam can be determined using the following formula:
d_min = (k * L^4 * w) / (384 * E * m)
Where:
- d_min is the minimum effective depth
- k is a constant depending on the type of load and support conditions (For a uniformly distributed load on a simply supported beam, k = 5)
- L is the span of the beam
- w is the uniformly distributed load
- E is the modulus of elasticity of the material (assumed to be constant)
- m is the modification factor for reinforcement
Calculation:
Substituting the given values into the formula:
d_min = (5 * 20^4 * w) / (384 * E * m)
To determine the range of minimum effective depth, we need to assume a value for the uniformly distributed load (w) and the modulus of elasticity (E). Let's assume:
- w = 10 kN/m (uniformly distributed load)
- E = 200 GPa (modulus of elasticity)
Substituting these values into the formula:
d_min = (5 * 20^4 * 10) / (384 * 200 * m)
d_min = 4166.67 / m
Considering the Modification Factors:
To satisfy the deflection criteria as per IS 456: 2000, the minimum effective depth should be within the range of 4.4 to 4.6 meters. Since the modification factor for tension reinforcement (m_t) is less than 1, it reduces the effective depth requirement. On the other hand, the modification factor for compression reinforcement (m_c) is greater than 1, which increases the effective depth requirement.
Therefore, the correct answer is a range of 4.4-4.6 meters for the minimum effective depth required to prevent failure in deflection.
Attention Civil Engineering (CE) Students!
To make sure you are not studying endlessly, EduRev has designed Civil Engineering (CE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Civil Engineering (CE).
|
Explore Courses for Civil Engineering (CE) exam
|
|
Similar Civil Engineering (CE) Doubts
Top Courses for Civil Engineering (CE)View all
A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer?
Question Description
A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer?.
A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer?.
Solutions for A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer?, a detailed solution for A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? has been provided alongside types of A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A cantilever beam of rectangular cross-section having 20 m span is subjected to uniformly distributed load throughout. The minimum effective depth required as per IS 456: 2000, so that the beam will not fail in deflection will be _______m. (up to two decimal places)Use Modification factor for tension and compression reinforcement to be 0.95 and 1.345 respectively.Correct answer is '4.4-4.6'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.
|
|
Explore Courses for Civil Engineering (CE) exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup