JEE Exam > JEE Questions > The work done by electric field during the di...
Start Learning for Free
The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance?
Most Upvoted Answer
The work done by electric field during the displacement of a negative ...
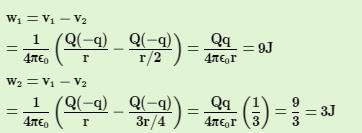
Community Answer
The work done by electric field during the displacement of a negative ...
The Work Done by Electric Field
The work done by an electric field on a charged particle can be calculated using the formula:
W = q * E * d * cos(theta)
Where:
W is the work done
q is the charge of the particle
E is the electric field strength
d is the displacement of the particle
theta is the angle between the direction of the electric field and the direction of displacement.
In this problem, we are given that the work done by the electric field is 9J. Let's assume that the charge of the particle is q and the electric field strength is E.
Work Done Over First Half of Distance
The problem states that the distance between the charges has been decreased by half. Let's denote the initial distance between the charges as d1 and the final distance as d2.
Since the distance has been halved, we can write:
d2 = d1/2
Now, we need to find the work done by the electric field over the first half of the distance, which means the displacement of the particle is d1/2.
Using the formula for work done, we have:
W1 = q * E * (d1/2) * cos(theta)
But we don't know the value of theta, so we can't calculate the exact value of W1. However, we can make some observations based on the given information.
Since the work done is positive (9J), we can conclude that the angle theta must be less than 90 degrees. This is because the cosine of an angle between 90 and 180 degrees is negative, which would result in a negative work done.
Therefore, we can say that the work done over the first half of the distance is positive, but we cannot determine the exact value without knowing the angle theta.
Summary:
The work done by the electric field over the first half of the distance is positive, but the exact value cannot be determined without knowing the angle theta.
The work done by an electric field on a charged particle can be calculated using the formula:
W = q * E * d * cos(theta)
Where:
W is the work done
q is the charge of the particle
E is the electric field strength
d is the displacement of the particle
theta is the angle between the direction of the electric field and the direction of displacement.
In this problem, we are given that the work done by the electric field is 9J. Let's assume that the charge of the particle is q and the electric field strength is E.
Work Done Over First Half of Distance
The problem states that the distance between the charges has been decreased by half. Let's denote the initial distance between the charges as d1 and the final distance as d2.
Since the distance has been halved, we can write:
d2 = d1/2
Now, we need to find the work done by the electric field over the first half of the distance, which means the displacement of the particle is d1/2.
Using the formula for work done, we have:
W1 = q * E * (d1/2) * cos(theta)
But we don't know the value of theta, so we can't calculate the exact value of W1. However, we can make some observations based on the given information.
Since the work done is positive (9J), we can conclude that the angle theta must be less than 90 degrees. This is because the cosine of an angle between 90 and 180 degrees is negative, which would result in a negative work done.
Therefore, we can say that the work done over the first half of the distance is positive, but we cannot determine the exact value without knowing the angle theta.
Summary:
The work done by the electric field over the first half of the distance is positive, but the exact value cannot be determined without knowing the angle theta.

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance?
Question Description
The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance?.
The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance?.
Solutions for The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? defined & explained in the simplest way possible. Besides giving the explanation of
The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance?, a detailed solution for The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? has been provided alongside types of The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? theory, EduRev gives you an
ample number of questions to practice The work done by electric field during the displacement of a negative charged particle towards the fixed positively charged particle is 9j .as a result the distance between the charges has been decreased by half.what is work done by electric field over first half of distance? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























