Quant Exam > Quant Questions > A Cistern has an inlet pipe and outlet pipe. ...
Start Learning for Free
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?
- a)3 hours
- b)2 hours
- c)5 hours
- d)4 hours
- e)None of the Above
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the ...
Inlet pipe Efficiency = 100/(8/6) = 75%
Outlet pipe Efficiency = 100/(4) = 25%
Net Efficiency = 75 – 25 = 50%(1/3)filled
2/3 filled = 100%
Required time = 100/50 = 2 hours
Outlet pipe Efficiency = 100/(4) = 25%
Net Efficiency = 75 – 25 = 50%(1/3)filled
2/3 filled = 100%
Required time = 100/50 = 2 hours
Most Upvoted Answer
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the ...
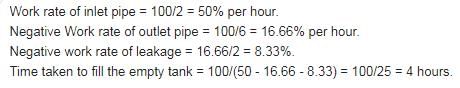
Free Test
FREE
| Start Free Test |
Community Answer
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the ...
Problem Solving Strategy:
- Determine the filling rate of the inlet pipe
- Determine the draining rate of the outlet pipe
- Determine the net filling rate when both pipes are opened
- Use the net filling rate to determine the time to fill the tank
Solution:
Let the total capacity of the cistern be C.
When the inlet pipe is plugged, the outlet pipe can empty the cistern in 4 hours. Therefore, the draining rate of the outlet pipe is:
outlet pipe draining rate = C/4
When the outlet pipe is plugged, the inlet pipe can fill the cistern in 1 hour 20 minutes, which is equivalent to 4/3 hours. Therefore, the filling rate of the inlet pipe is:
inlet pipe filling rate = C/(4/3) = 3C/4
When both pipes are opened simultaneously, the net filling rate is:
net filling rate = inlet pipe filling rate - outlet pipe draining rate
= 3C/4 - C/4
= C/2
This means that the cistern will fill at a rate of C/2 per hour.
At the start, the cistern is one-third filled, which is equivalent to C/3. Therefore, the remaining capacity to be filled is:
remaining capacity = C - C/3 = 2C/3
Using the net filling rate, the time to fill the remaining capacity is:
time to fill = remaining capacity / net filling rate
= (2C/3) / (C/2)
= 4/3 hours
= 1 hour 20 minutes
Therefore, the cistern will fill completely in 1 hour 20 minutes after both pipes are opened simultaneously.
The correct answer is option 'B', which is 2 hours.
- Determine the filling rate of the inlet pipe
- Determine the draining rate of the outlet pipe
- Determine the net filling rate when both pipes are opened
- Use the net filling rate to determine the time to fill the tank
Solution:
Let the total capacity of the cistern be C.
When the inlet pipe is plugged, the outlet pipe can empty the cistern in 4 hours. Therefore, the draining rate of the outlet pipe is:
outlet pipe draining rate = C/4
When the outlet pipe is plugged, the inlet pipe can fill the cistern in 1 hour 20 minutes, which is equivalent to 4/3 hours. Therefore, the filling rate of the inlet pipe is:
inlet pipe filling rate = C/(4/3) = 3C/4
When both pipes are opened simultaneously, the net filling rate is:
net filling rate = inlet pipe filling rate - outlet pipe draining rate
= 3C/4 - C/4
= C/2
This means that the cistern will fill at a rate of C/2 per hour.
At the start, the cistern is one-third filled, which is equivalent to C/3. Therefore, the remaining capacity to be filled is:
remaining capacity = C - C/3 = 2C/3
Using the net filling rate, the time to fill the remaining capacity is:
time to fill = remaining capacity / net filling rate
= (2C/3) / (C/2)
= 4/3 hours
= 1 hour 20 minutes
Therefore, the cistern will fill completely in 1 hour 20 minutes after both pipes are opened simultaneously.
The correct answer is option 'B', which is 2 hours.

|
Explore Courses for Quant exam
|

|
Question Description
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? for Quant 2025 is part of Quant preparation. The Question and answers have been prepared according to the Quant exam syllabus. Information about A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Quant 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer?.
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? for Quant 2025 is part of Quant preparation. The Question and answers have been prepared according to the Quant exam syllabus. Information about A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Quant 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Quant.
Download more important topics, notes, lectures and mock test series for Quant Exam by signing up for free.
Here you can find the meaning of A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A Cistern has an inlet pipe and outlet pipe. The inlet pipe fills the cistern completely in 1 hour 20 minutes when the outlet pipe is plugged. The outlet pipe empties the tank completely in 4 hours when the inlet pipe is plugged. If both pipes are opened simultaneously at a time when the tank was one-third filled, when will the tank fill thereafter?a)3 hoursb)2 hoursc)5 hoursd)4 hourse)None of the AboveCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Quant tests.

|
Explore Courses for Quant exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.