JEE Exam > JEE Questions > A sphere with some cavity has outer radius R....
Start Learning for Free
A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere is
- a)3R/4
- b)3R/2
- c)R/4
- d)R/2
Correct answer is option 'A'. Can you explain this answer?
Verified Answer
A sphere with some cavity has outer radius R. It rolls down an incline...
Let k be the radius of gyration and M the mass of the sphere. The kinetic energy should be the same in both the cases
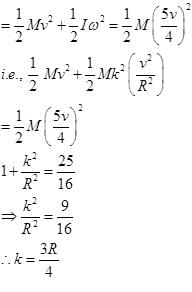
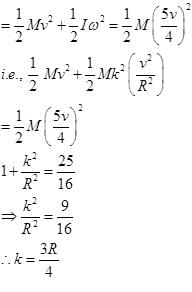
Most Upvoted Answer
A sphere with some cavity has outer radius R. It rolls down an incline...
To understand why the correct answer is option 'A', let's break down the problem into two parts: the rolling motion and the sliding motion of the sphere.
Rolling Motion:
- The sphere is rolling down the inclined plane without slipping, which means that the point of contact between the sphere and the plane is at rest.
- In this case, the sphere's linear speed is related to its angular speed (or rotational speed) by the equation v = ωR, where v is the linear speed, ω is the angular speed, and R is the radius of the sphere.
- Since the sphere is rolling without slipping, the frictional force between the sphere and the plane provides the necessary torque to keep the sphere rolling.
- The moment of inertia of a solid sphere about an axis passing through its center is given by I = (2/5)MR², where M is the mass of the sphere and R is the radius.
- The radius of gyration (k) is defined as the distance from the axis of rotation at which the entire mass of the object can be considered to be concentrated to calculate the moment of inertia.
- For a solid sphere, the radius of gyration is related to the radius by the equation k = R/√5.
- Using the equation v = ωR and the radius of gyration, we can write v = (k√5)ω.
Sliding Motion:
- Now, let's consider the sphere sliding down the same inclined plane without rolling.
- In this case, the sphere is purely sliding, and there is no rotational motion.
- The speed of the sphere at the bottom of the incline is given by v' = √(2gh), where g is the acceleration due to gravity and h is the height of the incline.
- Given that the speed at the bottom is 5v/4, we have v' = 5v/4.
- Substituting the value of v = (k√5)ω from the rolling motion, we can write 5v/4 = √(2gh).
Comparing the speeds:
- From the rolling motion, we have v = (k√5)ω.
- From the sliding motion, we have 5v/4 = √(2gh).
- Equating these two expressions, we get (k√5)ω = √(2gh).
- Simplifying this equation, we find ω = (4√5/5√2)(√h/R).
- Comparing this with the equation v = ωR, we can conclude that v = (4√5/5√2)(√hR/R).
- Simplifying further, we have v = (4√5/5√2)√h.
- Comparing this expression with v' = 5v/4 = √(2gh), we can equate them and solve for h.
Calculating the radius of gyration:
- By comparing the expressions for v and v', we can solve for h.
- After solving for h, we find h = (16/5)(R²/2k²).
- Substituting the value of k = R/√5, we get h = (16/5)(R²/2(R²/5)).
- Simplifying this equation, we
Rolling Motion:
- The sphere is rolling down the inclined plane without slipping, which means that the point of contact between the sphere and the plane is at rest.
- In this case, the sphere's linear speed is related to its angular speed (or rotational speed) by the equation v = ωR, where v is the linear speed, ω is the angular speed, and R is the radius of the sphere.
- Since the sphere is rolling without slipping, the frictional force between the sphere and the plane provides the necessary torque to keep the sphere rolling.
- The moment of inertia of a solid sphere about an axis passing through its center is given by I = (2/5)MR², where M is the mass of the sphere and R is the radius.
- The radius of gyration (k) is defined as the distance from the axis of rotation at which the entire mass of the object can be considered to be concentrated to calculate the moment of inertia.
- For a solid sphere, the radius of gyration is related to the radius by the equation k = R/√5.
- Using the equation v = ωR and the radius of gyration, we can write v = (k√5)ω.
Sliding Motion:
- Now, let's consider the sphere sliding down the same inclined plane without rolling.
- In this case, the sphere is purely sliding, and there is no rotational motion.
- The speed of the sphere at the bottom of the incline is given by v' = √(2gh), where g is the acceleration due to gravity and h is the height of the incline.
- Given that the speed at the bottom is 5v/4, we have v' = 5v/4.
- Substituting the value of v = (k√5)ω from the rolling motion, we can write 5v/4 = √(2gh).
Comparing the speeds:
- From the rolling motion, we have v = (k√5)ω.
- From the sliding motion, we have 5v/4 = √(2gh).
- Equating these two expressions, we get (k√5)ω = √(2gh).
- Simplifying this equation, we find ω = (4√5/5√2)(√h/R).
- Comparing this with the equation v = ωR, we can conclude that v = (4√5/5√2)(√hR/R).
- Simplifying further, we have v = (4√5/5√2)√h.
- Comparing this expression with v' = 5v/4 = √(2gh), we can equate them and solve for h.
Calculating the radius of gyration:
- By comparing the expressions for v and v', we can solve for h.
- After solving for h, we find h = (16/5)(R²/2k²).
- Substituting the value of k = R/√5, we get h = (16/5)(R²/2(R²/5)).
- Simplifying this equation, we

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer?
Question Description
A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer?.
A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer?.
Solutions for A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer?, a detailed solution for A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A sphere with some cavity has outer radius R. It rolls down an inclined plane without slipping and attains a speed v at the bottom. When this sphere slides down without rolling on the frictionless inclined plane of same height its speed at the bottom is 5v/4. The radius of gyration of the sphere isa)3R/4b)3R/2c)R/4d)R/2Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























