Chemistry Exam > Chemistry Questions > The degeneracy of a 3-D S.H.O having energy 9...
Start Learning for Free
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).
Correct answer is '10'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer...


Most Upvoted Answer
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer...
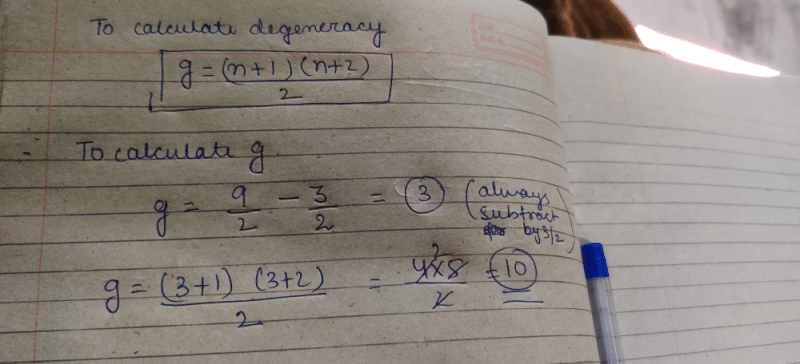
Free Test
FREE
| Start Free Test |
Community Answer
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer...
Explanation:
To understand the degeneracy of a 3-D Simple Harmonic Oscillator (S.H.O) having energy 9/2hv, let's break down the components and concepts involved.
1. Simple Harmonic Oscillator (S.H.O):
A simple harmonic oscillator is a system that exhibits periodic motion around a stable equilibrium position. In the case of a 3-D S.H.O, the oscillator can move in three mutually perpendicular directions, which we can refer to as x, y, and z.
2. Energy Levels of a 3-D S.H.O:
The energy levels of a 3-D S.H.O are quantized, meaning they can only take certain discrete values. The energy of a 3-D S.H.O is given by the equation E = (nx + ny + nz + 3/2)hv, where nx, ny, and nz are the quantum numbers along the x, y, and z directions, respectively. hv represents the energy quantum, which is the product of Planck's constant (h) and the frequency (v) of the oscillator.
3. Determining the Degeneracy:
Degeneracy refers to the number of different quantum states that have the same energy level. To determine the degeneracy of a specific energy level, we need to find the number of different combinations of quantum numbers (nx, ny, nz) that satisfy the energy equation.
4. Energy Level with E = 9/2hv:
Given that the energy of the system is 9/2hv, we can substitute this value into the energy equation and rearrange it to solve for the quantum numbers.
9/2hv = nx + ny + nz + 3/2
Multiplying both sides by 2/3hv, we get:
3/2hv = nx + ny + nz
Since the quantum numbers nx, ny, and nz must be non-negative integers, we can proceed to find all the possible combinations that satisfy the equation.
5. Combinations of Quantum Numbers:
To find the combinations, we can list all the possible values of nx, ny, and nz that satisfy the equation 3/2hv = nx + ny + nz.
- (0, 0, 3)
- (0, 1, 2)
- (0, 2, 1)
- (0, 3, 0)
- (1, 0, 2)
- (1, 1, 1)
- (1, 2, 0)
- (2, 0, 1)
- (2, 1, 0)
- (3, 0, 0)
6. Counting the Combinations:
By counting the number of combinations, we can determine the degeneracy of the energy level.
In this case, there are 10 different combinations, which means the degeneracy of the energy level 9/2hv is 10.
Conclusion:
The degeneracy of a 3-D S.H.O having energy 9/2hv is 10. This means there are 10 different quantum states that have the same energy level.
To understand the degeneracy of a 3-D Simple Harmonic Oscillator (S.H.O) having energy 9/2hv, let's break down the components and concepts involved.
1. Simple Harmonic Oscillator (S.H.O):
A simple harmonic oscillator is a system that exhibits periodic motion around a stable equilibrium position. In the case of a 3-D S.H.O, the oscillator can move in three mutually perpendicular directions, which we can refer to as x, y, and z.
2. Energy Levels of a 3-D S.H.O:
The energy levels of a 3-D S.H.O are quantized, meaning they can only take certain discrete values. The energy of a 3-D S.H.O is given by the equation E = (nx + ny + nz + 3/2)hv, where nx, ny, and nz are the quantum numbers along the x, y, and z directions, respectively. hv represents the energy quantum, which is the product of Planck's constant (h) and the frequency (v) of the oscillator.
3. Determining the Degeneracy:
Degeneracy refers to the number of different quantum states that have the same energy level. To determine the degeneracy of a specific energy level, we need to find the number of different combinations of quantum numbers (nx, ny, nz) that satisfy the energy equation.
4. Energy Level with E = 9/2hv:
Given that the energy of the system is 9/2hv, we can substitute this value into the energy equation and rearrange it to solve for the quantum numbers.
9/2hv = nx + ny + nz + 3/2
Multiplying both sides by 2/3hv, we get:
3/2hv = nx + ny + nz
Since the quantum numbers nx, ny, and nz must be non-negative integers, we can proceed to find all the possible combinations that satisfy the equation.
5. Combinations of Quantum Numbers:
To find the combinations, we can list all the possible values of nx, ny, and nz that satisfy the equation 3/2hv = nx + ny + nz.
- (0, 0, 3)
- (0, 1, 2)
- (0, 2, 1)
- (0, 3, 0)
- (1, 0, 2)
- (1, 1, 1)
- (1, 2, 0)
- (2, 0, 1)
- (2, 1, 0)
- (3, 0, 0)
6. Counting the Combinations:
By counting the number of combinations, we can determine the degeneracy of the energy level.
In this case, there are 10 different combinations, which means the degeneracy of the energy level 9/2hv is 10.
Conclusion:
The degeneracy of a 3-D S.H.O having energy 9/2hv is 10. This means there are 10 different quantum states that have the same energy level.

|
Explore Courses for Chemistry exam
|

|
Similar Chemistry Doubts
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer?
Question Description
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer?.
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer?.
Solutions for The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemistry.
Download more important topics, notes, lectures and mock test series for Chemistry Exam by signing up for free.
Here you can find the meaning of The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer?, a detailed solution for The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? has been provided alongside types of The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The degeneracy of a 3-D S.H.O having energy 9/2hv is _________ (answer should be an integer).Correct answer is '10'. Can you explain this answer? tests, examples and also practice Chemistry tests.

|
Explore Courses for Chemistry exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















