CA Foundation Exam > CA Foundation Questions > The ratio of two quantities is 3:4. if the an...
Start Learning for Free
The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent is
- a)75
- b)1200
- c)120
- d)None of these
Correct answer is option 'A'. Can you explain this answer?
Verified Answer
The ratio of two quantities is 3:4. if the antecedent of its inverse r...
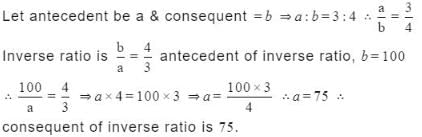
Most Upvoted Answer
The ratio of two quantities is 3:4. if the antecedent of its inverse r...
To solve this problem, we can use the concept of ratios and proportions. Let's break it down step by step:
Step 1: Understanding the given information
The ratio of two quantities is given as 3:4. This means that for every 3 units of the first quantity, there are 4 units of the second quantity.
Step 2: Finding the antecedent of the inverse ratio
The antecedent of the inverse ratio refers to the first value in the inverse ratio. In other words, it is the value that comes after the colon in the inverse ratio.
In this case, the antecedent of the inverse ratio is given as 100. This means that the inverse ratio is 100:something.
Step 3: Finding the consequent of the inverse ratio
To find the consequent of the inverse ratio, we need to determine the value that corresponds to the inverse ratio.
Since the ratio of the two quantities is 3:4, the inverse ratio is 4:3. This means that for every 4 units of the first quantity, there are 3 units of the second quantity.
Given that the antecedent of the inverse ratio is 100, we can set up the following proportion:
4/3 = 100/x
Cross-multiplying, we get:
4x = 3 * 100
4x = 300
Dividing both sides by 4, we find:
x = 300/4
x = 75
Therefore, the consequent of the inverse ratio is 75.
Step 4: Determining the correct answer
The question asks for the value of the consequent of the inverse ratio. We have found that the consequent is 75.
Among the given options (a) 75, (b) 1200, (c) 120, and (d) None of these, the correct answer is option (a) 75.
So, the correct answer is option 'A'.
Step 1: Understanding the given information
The ratio of two quantities is given as 3:4. This means that for every 3 units of the first quantity, there are 4 units of the second quantity.
Step 2: Finding the antecedent of the inverse ratio
The antecedent of the inverse ratio refers to the first value in the inverse ratio. In other words, it is the value that comes after the colon in the inverse ratio.
In this case, the antecedent of the inverse ratio is given as 100. This means that the inverse ratio is 100:something.
Step 3: Finding the consequent of the inverse ratio
To find the consequent of the inverse ratio, we need to determine the value that corresponds to the inverse ratio.
Since the ratio of the two quantities is 3:4, the inverse ratio is 4:3. This means that for every 4 units of the first quantity, there are 3 units of the second quantity.
Given that the antecedent of the inverse ratio is 100, we can set up the following proportion:
4/3 = 100/x
Cross-multiplying, we get:
4x = 3 * 100
4x = 300
Dividing both sides by 4, we find:
x = 300/4
x = 75
Therefore, the consequent of the inverse ratio is 75.
Step 4: Determining the correct answer
The question asks for the value of the consequent of the inverse ratio. We have found that the consequent is 75.
Among the given options (a) 75, (b) 1200, (c) 120, and (d) None of these, the correct answer is option (a) 75.
So, the correct answer is option 'A'.
Free Test
FREE
| Start Free Test |
Community Answer
The ratio of two quantities is 3:4. if the antecedent of its inverse r...
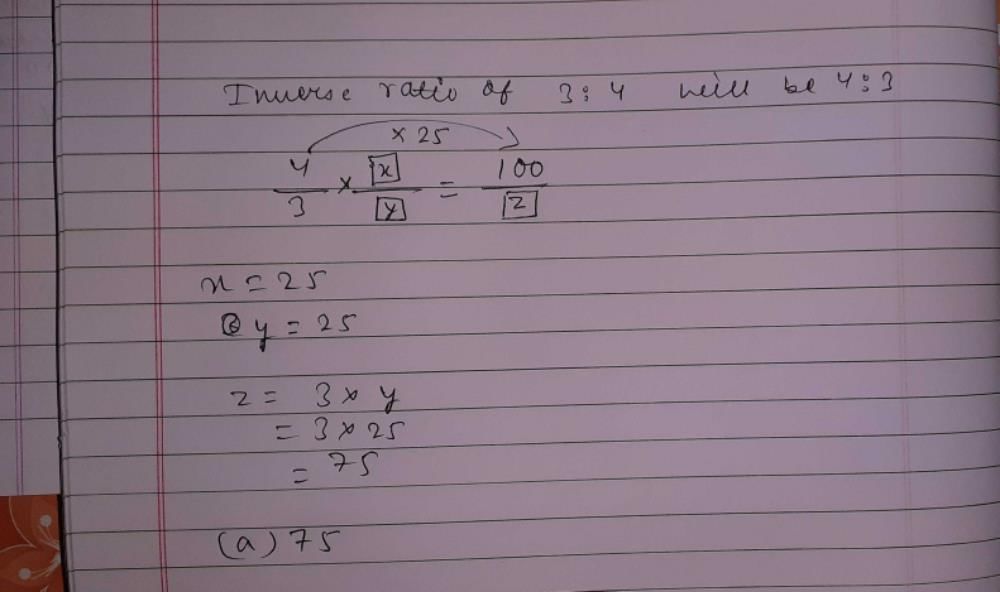

|
Explore Courses for CA Foundation exam
|

|
Similar CA Foundation Doubts
The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer?
Question Description
The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer?.
The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? for CA Foundation 2025 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer?.
Solutions for The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for CA Foundation.
Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free.
Here you can find the meaning of The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The ratio of two quantities is 3:4. if the antecedent of its inverse ratio is 100, then its consequent isa)75b)1200c)120d)None of theseCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice CA Foundation tests.

|
Explore Courses for CA Foundation exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























