Mathematics Exam > Mathematics Questions > The two planes represented by ax2- by2 + cz2+...
Start Learning for Free
The two planes represented by ax2 - by2 + cz2 + 2fyz + 2gzx + 2 hxy = 0 are perpendicular if
- a)abc + 2fgh + af2 + bg- + ch2 = 0
- b)af + bg + ch - 0
- c)a + b + c = 0
- d)f2 + g2 + h2 - bc - ca - ab = 0
Correct answer is option 'C'. Can you explain this answer?
Most Upvoted Answer
The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 ...
Let the two planes r epresented by
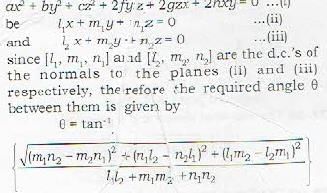
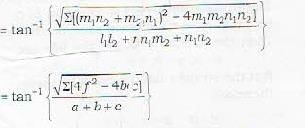
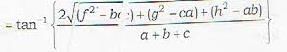
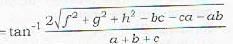
Condition of perpendicularity.
The two planes are perpendicular if
θ = 90° or tan θ = ∞
or a + b + c = 0
Remark: since the two planes pass through the origin, therefore the two planes are never parallel. The two planes can however coincide.
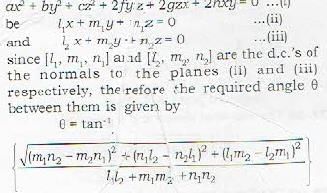
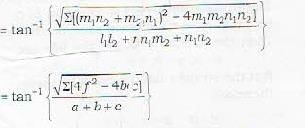
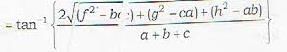
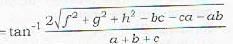
Condition of perpendicularity.
The two planes are perpendicular if
θ = 90° or tan θ = ∞
or a + b + c = 0
Remark: since the two planes pass through the origin, therefore the two planes are never parallel. The two planes can however coincide.
Free Test
FREE
| Start Free Test |
Community Answer
The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 ...
To determine whether the two planes represented by the given equation are perpendicular, we can use the dot product of their normal vectors.
The equation of a plane in general form is given by ax + by + cz + d = 0, where (a, b, c) is the normal vector to the plane.
First, let's rewrite the given equation in the standard form of a plane:
ax^2 - by^2 + cz^2 + 2fyz + 2gzx + 2hxy = 0
Dividing the equation by the coefficient of x^2, we get:
x^2 - (b/a)y^2 + (c/a)z^2 + (2f/a)yz + (2g/a)zx + (2h/a)xy = 0
Comparing this equation with the general form of a plane equation, we can determine the normal vector to the plane:
(a, b/a, c/a)
Similarly, for the second plane, the normal vector is given by (a, f/b, h/b).
To check if the two planes are perpendicular, we need to find the dot product of their normal vectors. If the dot product is zero, the planes are perpendicular.
Taking the dot product of the two normal vectors, we have:
(a)(a) + (b/a)(f/b) + (c/a)(h/b) = a^2 + f + h
The dot product is zero if and only if the equation a^2 + f + h = 0 is satisfied.
Therefore, the correct answer is option 'C': a, b, c = 0.
This means that the two planes represented by the given equation are perpendicular if a^2 + f + h = 0.
The equation of a plane in general form is given by ax + by + cz + d = 0, where (a, b, c) is the normal vector to the plane.
First, let's rewrite the given equation in the standard form of a plane:
ax^2 - by^2 + cz^2 + 2fyz + 2gzx + 2hxy = 0
Dividing the equation by the coefficient of x^2, we get:
x^2 - (b/a)y^2 + (c/a)z^2 + (2f/a)yz + (2g/a)zx + (2h/a)xy = 0
Comparing this equation with the general form of a plane equation, we can determine the normal vector to the plane:
(a, b/a, c/a)
Similarly, for the second plane, the normal vector is given by (a, f/b, h/b).
To check if the two planes are perpendicular, we need to find the dot product of their normal vectors. If the dot product is zero, the planes are perpendicular.
Taking the dot product of the two normal vectors, we have:
(a)(a) + (b/a)(f/b) + (c/a)(h/b) = a^2 + f + h
The dot product is zero if and only if the equation a^2 + f + h = 0 is satisfied.
Therefore, the correct answer is option 'C': a, b, c = 0.
This means that the two planes represented by the given equation are perpendicular if a^2 + f + h = 0.

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
Question Description
The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? for Mathematics 2025 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mathematics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer?.
The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? for Mathematics 2025 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mathematics 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer?.
Solutions for The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer?, a detailed solution for The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The two planes represented by ax2- by2 + cz2+ 2fyz + 2gzx + 2 hxy = 0 are perpendicular ifa)abc+ 2fgh + af2 + bg- + ch2 = 0b)af + bg + ch - 0c)a + b + c = 0d)f2 + g2 + h2 - bc- ca - ab= 0Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

















