Mechanical Engineering Exam > Mechanical Engineering Questions > In an epicyclic gear train with a sun gear, p...
Start Learning for Free
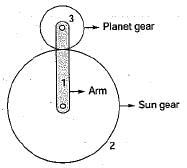
In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate with
- a)50 RPM
- b)100 RPM
- c)150 RPM
- d)200 RPM
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
In an epicyclic gear train with a sun gear, planet gear and a moving a...
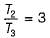
If
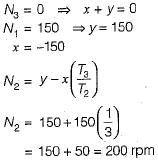
Most Upvoted Answer
In an epicyclic gear train with a sun gear, planet gear and a moving a...
Epicyclic Gear Train
An epicyclic gear train is a gear system consisting of one or more outer gears, or planet gears, revolving about a central, or sun gear. The planet gears are mounted on a movable arm which can rotate about its own axis. The gear train can be used to transmit power, change torque or direction of rotation, or to increase or decrease speed.
Given Data
- Sun Gear Teeth, Ts = ?
- Planet Gear Teeth, Tp = Ts/3
- Arm Angular Velocity, ω = 150 RPM
Solution
1. Find the Ratio of Gear Train
The ratio of an epicyclic gear train is given by the equation:
Ratio = (Ts + Tp) / Tp
Substituting Tp = Ts/3, we get:
Ratio = (Ts + Ts/3) / (Ts/3) = 4
Therefore, the gear train has a ratio of 4.
2. Find the Angular Velocity of Sun Gear
The angular velocity of the sun gear, ωs, is given by the equation:
ωs = ω / (1 + Ratio)
Substituting ω = 150 RPM and Ratio = 4, we get:
ωs = 150 / (1 + 4) = 30 RPM
3. Find the Rotational Speed of Sun Gear
The rotational speed of the sun gear, Ns, is related to its angular velocity by the equation:
Ns = ωs / (2π)
Substituting ωs = 30 RPM, we get:
Ns = 30 / (2π) = 4.77 RPM
4. Find the Rotational Speed of the Arm
The rotational speed of the arm, Na, is related to the rotational speed of the sun gear by the equation:
Na = Ns / Ratio
Substituting Ns = 4.77 RPM and Ratio = 4, we get:
Na = 4.77 / 4 = 1.19 RPM
5. Find the Rotational Speed of the Sun Gear
The rotational speed of the sun gear, Np, when the planet gear is fixed, is related to the rotational speed of the arm by the equation:
Np = Ratio × Na
Substituting Ratio = 4 and Na = 1.19 RPM, we get:
Np = 4 × 1.19 = 4.76 RPM
Therefore, the rotational speed of the sun gear when the planet gear is fixed is approximately 4.76 RPM or 200 RPM (rounded off to the nearest 10 RPM).
Answer
Therefore, the correct answer is option (d) 200 RPM.
An epicyclic gear train is a gear system consisting of one or more outer gears, or planet gears, revolving about a central, or sun gear. The planet gears are mounted on a movable arm which can rotate about its own axis. The gear train can be used to transmit power, change torque or direction of rotation, or to increase or decrease speed.
Given Data
- Sun Gear Teeth, Ts = ?
- Planet Gear Teeth, Tp = Ts/3
- Arm Angular Velocity, ω = 150 RPM
Solution
1. Find the Ratio of Gear Train
The ratio of an epicyclic gear train is given by the equation:
Ratio = (Ts + Tp) / Tp
Substituting Tp = Ts/3, we get:
Ratio = (Ts + Ts/3) / (Ts/3) = 4
Therefore, the gear train has a ratio of 4.
2. Find the Angular Velocity of Sun Gear
The angular velocity of the sun gear, ωs, is given by the equation:
ωs = ω / (1 + Ratio)
Substituting ω = 150 RPM and Ratio = 4, we get:
ωs = 150 / (1 + 4) = 30 RPM
3. Find the Rotational Speed of Sun Gear
The rotational speed of the sun gear, Ns, is related to its angular velocity by the equation:
Ns = ωs / (2π)
Substituting ωs = 30 RPM, we get:
Ns = 30 / (2π) = 4.77 RPM
4. Find the Rotational Speed of the Arm
The rotational speed of the arm, Na, is related to the rotational speed of the sun gear by the equation:
Na = Ns / Ratio
Substituting Ns = 4.77 RPM and Ratio = 4, we get:
Na = 4.77 / 4 = 1.19 RPM
5. Find the Rotational Speed of the Sun Gear
The rotational speed of the sun gear, Np, when the planet gear is fixed, is related to the rotational speed of the arm by the equation:
Np = Ratio × Na
Substituting Ratio = 4 and Na = 1.19 RPM, we get:
Np = 4 × 1.19 = 4.76 RPM
Therefore, the rotational speed of the sun gear when the planet gear is fixed is approximately 4.76 RPM or 200 RPM (rounded off to the nearest 10 RPM).
Answer
Therefore, the correct answer is option (d) 200 RPM.
|
Explore Courses for Mechanical Engineering exam
|
|
Similar Mechanical Engineering Doubts
Top Courses for Mechanical EngineeringView all
In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer?
Question Description
In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer?.
In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer?.
Solutions for In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In an epicyclic gear train with a sun gear, planet gear and a moving arm. The ratio of number of teeth of the sun gear to that of the planet gear is 3. If the planet gear is fixed and the arm has an angular velocity of 150 RPM the sun gear will rotate witha)50 RPMb)100 RPMc)150 RPMd)200 RPMCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.
|
|
Explore Courses for Mechanical Engineering exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup